B 01 CENCO Compound Pendulum Manual

User Manual:

Open the PDF directly: View PDF .
Page Count: 4

Copyright, 2003, Sargent-Welch Scientific Company No. 71990-154

THE COMPOUND PENDULUM

OBJECT: To study the properties of a compound pendulum,

and to determine the acceleration due to gravity by the use

of such a pendulum.

and the application of Newton's second law of motion for

rotating bodies

L



I

a

METHOD: An experimental pendulum is suspended

successively about several axes at different points along its

length and the period about each axis is observed. A graph

is plotted of the period versus the distance of the axis of

suspension from one end of the pendulum. The nature of the

graph shows the physical properties of the compound

pendulum. From values of the period and the corresponding

length of the equivalent simple pendulum as determined

from the graph, the acceleration due to gravity is calculated.

From the mass of the pendulum and its radius of gyration as

obtained from the curve, the rotational inertia of the

pendulum is computed.

where



is the angular displacement,



the angular

acceleration, L the torque and I the rotational inertia of the

body.

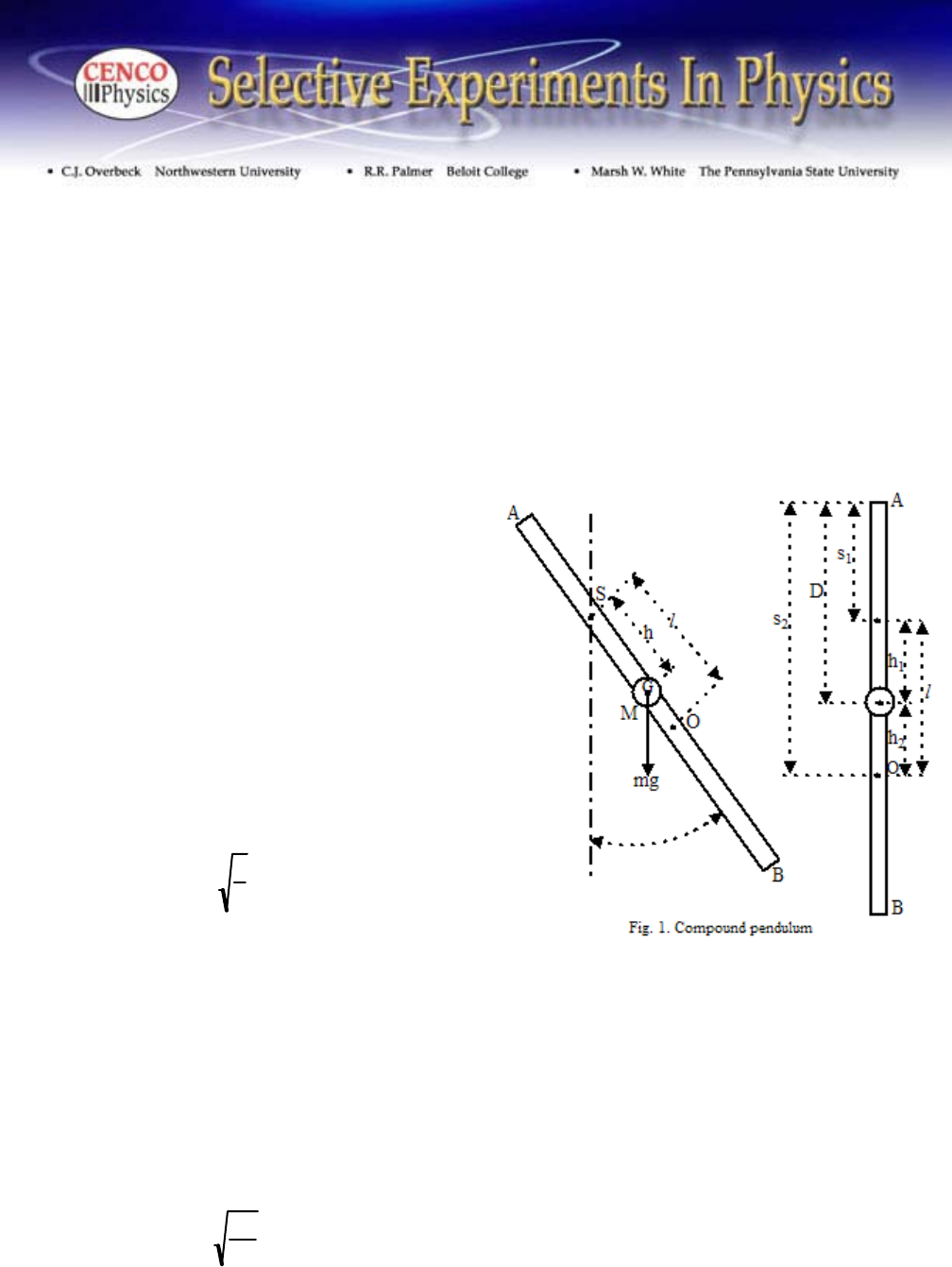
Figure 1 represents a compound pendulum of mass m,

consisting of a rectangular bar AB to which a cylindrical

THEORY: A simple pendulum consists of a small body

called a "bob" (usually a sphere) attached to the end of a

string the length of which is great compared with the

dimensions of the bob and the mass of which is negligible in

comparison with that of the bob. Under these conditions the

mass of the bob may be regarded as concentrated at its

center of gravity, and the length l of the pendulum is the

distance of this point from the axis of suspension. When a

simple pendulum swings through a small arc, it executes

linear simple harmonic motion of period T, given by the

equation

T2



l

g (1)

where g is the acceleration due to gravity. This relation-ship

affords one of the simplest and most satisfactory methods of

determining g experimentally.

mass M is attached. The pendulum is suspended on a

transverse axis through the point S. In the diagram, the

cylindrical mass M is represented as being exactly in the

middle of the bar, thereby making a symmetrical system in

which the center of gravity G is at the geometrical center.

Obviously, this particular condition is a very special case,

and has nothing whatever to do with the general treatment of

the problem.

When the dimensions of the suspended body are not

negligible in comparison with the distance from the axis of

suspension to the center of gravity, the pendulum is called a

compound, or physical, pendulum. Any body mounted upon

a horizontal axis so as to vibrate under the force of gravity is

a compound pendulum. The motion of such a body is an

angular vibration about the axis of suspension. The

expression for the period of a compound pendulum may be

deduced from the general expression for the period of any

angular simple harmonic motion

In the equilibrium position, the center of gravity G is vertically

below the axis of suspension S. When the body is rotated

through an angle



, the weight of the system mg, which

may be regarded as concentrated at the center of gravity,

exerts a restoring torque about S given by

T2







a (2)

L



mgh sin



(4)

where h is the distance from the axis of suspension to the

center of gravity. If a minus sign is used to indicate the tact

that the torque L is opposite in sign to the displacement



,

Eqs. (3) and (4) yield

I

amgh sin



(5)

When the angular displacement



is sufficiently small, sin



is approximately equal to



measured in radians. With this

restriction Eq. (5) may be written

amgh

I



(6)

Since m, g, h and I are all numerically constant for any given

case, Eq. (6), may be written simply

ak



(7)

where k is a constant. Equation (7) is the defining equation

of angular simple harmonic motion, i.e., motion in which the

angular acceleration is directly proportional to the angular

displacement and oppositely directed. Since the system

executes angular simple harmonic motion, substitution of the

expression for a from Eq. (6) in Eq. (2) yields the equation

for the period of a compound pendulum

T2



I

mgh (8)

where I is the rotational inertia of the pendulum about the

axis of suspension S. It is convenient to express I in terms of

I0, the rotational inertia of the body about an axis through its

center of gravity G. If the mass of the body is m,

(9) Iomko

2

where ko is the radius of gyration about an axis through G.

For any regular body, ko can be computed by means of the

appropriate formula (see any handbook of physics or

engineering); for an irregular body it must be determined

experimentally. The rotational inertia about any axis parallel

to the one through the center of gravity is given by

(10) IIomh2

where h is the distance between the two axes. Substitution

of the relationships of Eqs. (9) and (10) in Eq. (8) yields

T2



ko

2h2

gh (11)

This equation expresses the period in terms of the geometry

of the body. It shows that the period is independent of the

mass, depending only upon the distribution of the mass (as

measured by k

o) and upon the location of the axis of

suspension (as specified by h). Since the radius of gyration

of any given body is a constant, the period of any given

pendulum is a function of h only. Comparison of Eq. (1) and

Eq. (11) shows that the period of a compound pendulum

suspended on an axis at a distance h from its center of

gravity is equal to the period of a simple pendulum having a

length given by

lko

2



h2

hhko

2

h (12)

The simple pendulum whose period is the same as that of a

given compound pendulum is called the "equivalent simple

pendulum."

It is sometimes convenient to specify the location of the axis

of suspension S by its distance s from one end of the bar,

instead of by its distance h from the center of gravity G. If the

distances s1, s2 and D (Fig. 1) are measured from the end A,

the distance h1 must be considered negative, since h is

measured from G. Thus, if D is the fixed distance from A to

G, s1 = D + h1, s2 = D + h2 and, in general, s = D + h.

Substitution of this relationship in Eq. (11) yields

T2



ko

2sD

2

gsD

(13)

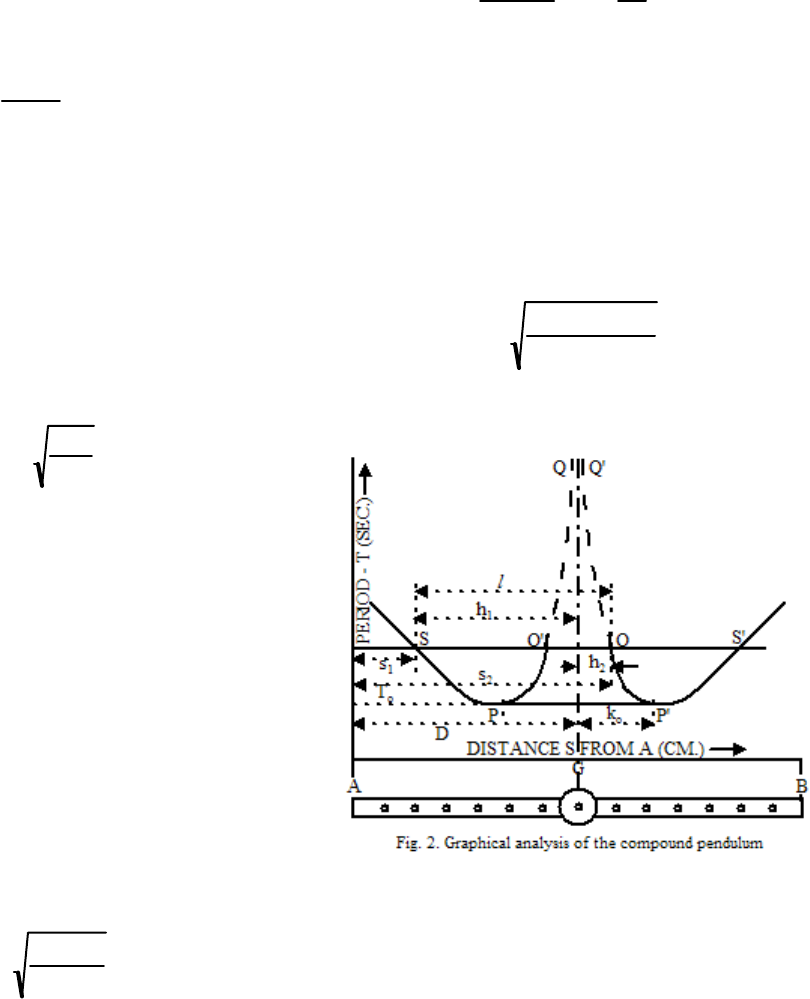
The relationships between T and s expressed by Eq. (l3) can

best be shown graphically. When T is plotted as a function of

s, a pair of identical curves SPQ and S'P'Q' are obtained as

illustrated in Fig. 2. (The dotted portions represent

extrapolations over apart of the body where it is difficult to

obtain experimental data with this particular pendulum.)

Analysis of these curves reveals several interesting and

remarkable properties of the compound pendulum.

Beginning at the end A, as the axis is displaced from A

toward B the period diminishes, reaching a minimum value

at P, after which it increases as S approaches G. The two

curves are asymptotic to a perpendicular line through G,

indicating that the period becomes infinitely great for an axis

through the center of gravity. As the axis is displaced still

2

Thus

farther from A (to the other side of G), the period again

diminishes to the same minimum value at a second point P',

after which it again increases.

ho



k

o (20)

A horizontal line SS', corresponding to a chosen value of T,

intersects the, graph in four points, indicating that there are

four positions of the axis, two on each side of the center of

gravity, for which the periods are the same. These positions

are symmetrically located with respect to G. There are,

therefore, two numerical values of h for which the period is

the same, represented by h1 and h2 (Figs. 1 and 2). Thus, for

any chosen axis of suspension S there is a conjugate point

O on the opposite side of the center of gravity such that the

periods about parallel axes through S and O are equal. The

point O is called the center of oscillation with respect to the

particular axis of suspension S. Consequently, if the center

of oscillation of any compound pendulum is located, the

pendulum may be inverted and supported at O without

altering its period. This so-called reversibility is one of the

unique properties of the compound pendulum and one that

has been made the basis of a very precise method of

measuring g (Kater's reversible pendulum).

Substituting in Eq. (12) yields

l

o



2

k

o (21)

Thus, the shortest simple pendulum to which the compound

pendulum can be made equivalent has a length lo equal to

twice the radius of gyration of the body about a parallel axis

through the center of gravity. This is indicated in Fig. 2 by

the line PP'. Inspection of the figure further shows that, of the

two values of h for other than minimum period, one is less

than and one greater than ko. From the foregoing it is evident

that if two asymmetrical points S and O can be found such

that the periods of vibration are identical, the length of the

equivalent simple pendulum is the distance between the two

points, and the necessity for locating the center of gravity is

eliminated. Thus, by making use of the reversible property of

the compound pendulum, a simplicity is, achieved similar to

that of the simple pendulum, the experimental

determinations being reduced to one measurement of length

and one of period.

It can be shown that the distance between S and O is equal

to l, the length of the equivalent simple pendulum. Equating

the expressions for the squares of the periods about S and

O, respectively, from Eq. (11)

APPARATUS: The apparatus used in this experiment is very

simple, consisting merely of a rectangular steel bar

approximately 1 meter long carrying a heavy cylindrical

mass, and supported on a horizontal axis (Fig. 3). The bar

T24



2

g

ko

2h1

2

h

1







 



4



2

g

ko

2h2

2

h2







 



 (14)

from which

T24



2

gh1h2

  (15)

or T2



h

1h2

g (16)

Comparison of Eqs. (1) and (16) shows that the length l of

the equivalent simple pendulum is

l

h1h2 (17)

Thus, the length of the equivalent simple pendulum is SO

(Figs. 1 and 2).

S' and O' are a second pair of conjugate points

symmetrically located with respect to S and O respectively,

i.e., having the same numerical values of h1 and h2.

Fig. 3. Experimental Pendulum

has a series of holes distributed along its length to provide

several axes of suspension. In use the pendulum is

supported successively at the various holes on a hardened

steel knife-edge in a wall bracket, and its period of vibration

determined with the aid of a stopwatch. A meter stick and a

platform balance with a set of weights are the only other

apparatus required.

Further consideration of Fig. 2 reveals the fact that the

period of vibration of a given body cannot be less than a

certain minimum value To, for which the four points of equal

period reduce to two, S and O' merging into P, and S' and O

merging into P' as h1 becomes numerically equal to h2. The

value of ho corresponding to minimum period can be

deduced by solving Eq. (14) for k02, which yields

PROCEDURE:

k (18)

o

2h1h2Experimental: Support the pendulum on the knife-edge at

the hole nearest to one end of the bar, making sure that it

swings freely in a vertical plane. With the aid of a stopwatch,

and setting

h (19)

oh1h2

3

4

observe the time of 50 full vibrations and determine the

period. In making this determination, begin with the count of

"zero" as the pendulum passes through its central position,

count "one" as it makes its next transit through center going

in the same direction, etc. In a like manner determine the

period about an axis through each of the several holes.

Remove the pendulum from its support and with a meter

stick (preferably one equipped with caliper jaws) measure

the distances s1, s2, etc., of the various points of suspension

from one end of the bar. Record these lengths opposite the

corresponding periods.

Weigh the pendulum on the platform balance and record its

mass m.

Analysis of Data: 1. Plot the data in a graph similar to Fig.

2. Draw any horizontal line SS'. From the corresponding

period T as determined by the ordinate of this line, and the

length l of the corresponding equivalent simple pendulum as

given by the average of the values of SO and S'O', calculate

the acceleration g due to gravity, by means of Eq. (1) .

Compare with the accepted value and record the percentage

difference.

2. From the mass m of the pendulum and the radius of

gyration ko as determined from the graph, compute the

rotational inertia Io about the axis G by Eq. (9). Compute the

rotational inertia I about the axis S by Eq. (10).

QUESTIONS: 1. What is the minimum period with which this

pendulum can vibrate? What is the length of a simple

pendulum having the same period?

2. Describe how Fig. 2 would be altered if the cylindrical

mass M were near one end, say the end B.

3. With a given, axis of suspension, say S, discuss the

effect upon the period of (a) increasing the mass of the

cylindrical body; (b) moving it nearer to S.

4. How would the value of the minimum period To be

affected by moving the mass M in either direction from the

middle?

5. With the mass M near the end B and the pendulum

suspended about an axis S near A, how could the vibration

of the system about the axis S' be experimentally observed?

6. Does the center of oscillation of a solid body, such as a

rod or bar, lie within the body for any transverse axis of

suspension? Explain.

7. Locate the center of oscillation of a meter stick

suspended about a transverse axis at the 10cm mark. At

what other positions could the meter stick be suspended and

have the same period?

8. Prove that the period of a thin ring hanging on a peg is

the same as that of a simple pendulum whose length is to

the diameter of the ring.