Experiment 4 Lab Manual Phys 115L Spring 2018

User Manual: Pdf

Open the PDF directly: View PDF .
Page Count: 15

4. Coupled oscillations

A. Objectives

 Observe the vibrations of a coupled oscillator consisting of two masses hanging on

springs. Measure the frequencies of the two normal modes of this system, and see if you

can verify the theoretical result for these frequencies.

 Individually excite each of the two normal modes of two masses hanging on springs.

Observe the relative displacements of the two masses for each mode. See if you can

verify the theoretical results for these relative displacements.

B. Equipment required

1. 80-20 frame with eyebolts for suspending masses

2. Three ringstands, each consisting of a ½" diameter, 24" tall stainless steel post on a 5" x 8"

stainless steel base

3. White cardboard sheet to set behind frame

4. Ball and spring assembly consisting of two stainless steel balls with tapped 8-32 holes, two

extension springs, three 8-32 eyebolts, and a 1/4" diameter magnet in a ½" diameter holder

5. Stopwatch

6. Drive coil assembly and two patch cables with banana plug ends

7. Machine vision camera with varifocal lens on ½" post and USB 3.0 cable

8. Incandescent light bulb with power supply on ½" post

9. 4" square plate on ½" post with taped-on cardboard strip

10. Five ½" right-angle post clamps and one additional ½" post

11. Computer data acquisition system including Pasco 850 interface and Pasco Capstone,

XiCamTool, and Tracker software

C. Introduction

Often in physics, we encounter multiple oscillators with motions that are coupled to each other.

Many mechanical structures exhibit them. Even seemingly isolated oscillators, such as two

identical pendulum clocks set near each other in the same room, can show effects of coupling.

Atoms in molecules sometimes vibrate as coupled oscillators. And, there are many examples of

coupled oscillators in electronic circuits.

1. Two masses hanging on springs

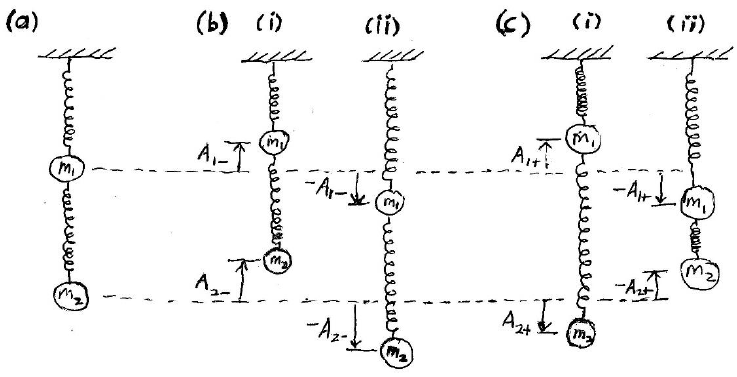
In this lab, we'll study two masses hanging on springs, as illustrated in Figure 4.1. Specifically, a

mass

hangs from a support on a spring of spring constant

1,k

and a mass

hangs from the

first mass on a spring of spring constant

2.k

If we allow the masses to move undisturbed for

some time, eventually they'll settle down into their static equilibrium positions shown in Figure

4.1(a). In this configuration, each spring exerts just enough force to cancel the downward

gravitational force on the objects below.

Figure 4.1. Two masses hanging on springs. (a) Configuration of the masses when hanging in static

equilibrium. (b) Configuration of the masses when displaced from equilibrium.

Now suppose that the masses are displaced from their equilibrium positions as shown in Figure

4.1(b). Let

be the displacement of mass

and

the displacement of mass

, both

measured relative to the equilibrium positions. With this definition of displacement, the

equations of motion of the two masses are

1 1 1 2 1 2

2()

m k y k y y

dt    

(4.1)

2 2 2 1

2()

m k y y

dt   

(4.2)

We can rewrite these as

 

1 1 2 1 2 2

m k k y k y

dt   

(4.3)

2 2 2 2 1

m k y k y

dt 

(4.4)

If the right hand side of each of the equations (4.3) and (4.4) were zero, then each equation

would be that of a single harmonic oscillator – one with mass

and spring constant

kk

and another with mass

and spring constant

2.k

However on the right-hand side of equation

(4.3) for the first oscillator there is a term proportional to the displacement of the second

oscillator. Similarly on the right-hand side of equation (4.4) for the second oscillator, there is a

term proportional to the displacement of the first oscillator. These terms couple the motion of the

first oscillator to the second, and vice-versa. In other words, we have a system of two coupled

oscillators.

In order to solve for the motion of the two oscillators, we'll guess that there is a solution in which

both oscillators have a sinusoidal oscillation at the same frequency



 

1 1 1

cos

i i i

y A t





(4.5)

 

2 2 2

cos

i i i

y A t





(4.6)

In these equations, we're anticipating that there might be more than one solution for the

frequency, so we label the different solutions with an index i. Substituting these expressions into

the equations of motion (4.3) and (4.4), we find a solution only if

12 ,

i i i

  



and

21 2 2

i i i

k k k







  





(4.7)

222

i i i





  





(4.8)

Equations (4.7) and (4.8) can each be solved for the ratio

if these equations are both

satisfied the ratio must be the same, so that

212

Akk m











   











(4.9)

From the second equality in equation (4.9) we deduce that (4.5) and (4.6) are solutions of (4.3)

and (4.4) if and only if

2 1 2 2 1 2 2 1 2 2

2 1 2 1 1 2

()

k k k k k k k k k k

m m m m m m





   

   

     

   

   

(4.10)

2 1 2 2 1 2 2 1 2 2

2 1 2 1 1 2

()

k k k k k k k k k k

m m m m m m





   

   

     

   

   

(4.11)

So for this coupled oscillator, there are solutions of the form (4.5) and (4.6) for two and only two

specific frequencies, which we label as



with

i

and

.i

Oscillations of this form, in

which all components of the system vibrate at the same frequency, are called normal modes.

The two normal modes differ not only in frequency, but in the displacements

A

and

2.A

turns out that the ratio

/AA



is always positive, so for the "minus" mode the two masses are

always moving in the same direction, as illustrated in Figure 4.2(b). On the other hand, for the

"plus" mode the ratio

/AA



is always negative, so the masses are always moving in the

opposite direction, as illustrated in Figure 4.2(c).

Figure 4.2. (a) Illustration of the masses when in equilibrium and at rest. (b) Displaced positions of the

masses when oscillating only in the "minus" frequency mode, at (i) the time when the displacement of

mass

is a maximum, and (ii) one-half cycle of oscillation later. (c) Displaced positions of the masses

when oscillating only in the "plus" frequency mode, at (i) the time when the displacement of mass

is a

maximum, and (ii) one-half cycle of oscillation later.

In the minus mode, the two masses vibrate up and down in the same direction. There is also a

vibration in the distance between two masses, but the vibration of the center of mass position is

more pronounced. The plus mode consists of a "stretching mode" vibration, in which the two

masses vibrate in opposite directions. There is also a vibration of the center-of-mass, but the

vibration in the distance between the masses is more pronounced.

From Figure 4.2, it's easy to see why the "plus" mode has the higher frequency. In this mode,

spring

is stretched to a much greater degree. Therefore the restoring forces are larger and the

frequency is higher in the “plus mode” than in the "minus" mode.

Since our system is linear, any superposition of solutions is a solution. This allows us to write

down the general solution to the equations of motion (4.3) and (4.4) as a superposition of the two

normal mode vibrations:

   

1 1 1

( ) cos cosy t A t A t

   

     

   

(4.12)

   

2 2 2

( ) cos cosy t A t A t

   

     

   

(4.13)

As expected, this general solution to a system of two coupled second order differential equations

contains four arbitrary constants:

, , , and AA



   

. The other two parameters,

and AA



, are

not arbitrary constants, because once

and AA



are specified,

and AA



are given by

equation (4.9) i.e.

AA k







 







(4.14)

AA k







 







(4.15)

If the coupling were absent, we'd have a system with just two frequencies – a frequency



for

mass 1 and a frequency



for mass 2. With the coupling, we find that the system still oscillates

with only two frequencies, but these frequencies are





and





. Both are different from the

uncoupled frequencies



and



If we displace just one of the masses and let go, we'll generally find that both

A

and

A

are

different from zero. The resulting oscillation will not be a pure sinusoid of the displaced mass.

Instead, both masses will oscillate in superpositions (4.12) and (4.13) of the two normal modes.

Each mass will oscillate at both frequencies.

It is possible to excite just one of the two normal modes. We could do this by exciting the

oscillation with a drive at just one of the frequencies





. Or, we could set the initial

displacements of the two masses to match the amplitude ratio (4.14). In either case, we would

produce an oscillation with

10.A

Then the displacements would be given by

 

cosy A t



  



(4.16)

 

cosy A t



  



(4.17)

and the masses would oscillate at just the one frequency





This is referred to as oscillation in a

single mode. It is still a coupled oscillation: both masses oscillate at that frequency, with a

definite amplitude ratio given by equation (4.14). Of course, it would also be possible to excite

an oscillation with

10,A

in which both masses oscillate only at the frequency





D. Experimental Procedure

The apparatus is illustrated in Figure 4.3. It contains a frame made of 80-20. This is an extruded

aluminum framing material that can be ordered with standardized brackets, nuts, and bolts, and is

commonly used to make frames and supports for scientific apparatus. Your frame includes nine

¼-20 eyebolts that can be used to support springs. You can easily loosen the eyebolts by turning

them slightly counterclockwise, move them to a desired position, and tightening them back

down. For this experiment you'll only use the top center eyebolt as shown in Figure 4.3(b). This

will support the system of two masses hanging on springs that we analyzed in the introduction.

Figure 4.3. Experimental apparatus (a) "Ringstand" with machine vision camera, varifocal lens, and light

bulb. (b) Additional components including 80-20 frame, masses and springs, coil, and cardboard flap.

In this experiment we'll measure the displacement of the masses with video analysis. We'll take

data with a machine vision camera. This type of camera is generally capable of high frame rates

of the order of a few hundred frames per second, comes with support for detailed control of the

camera parameters, and produces video that is compatible with sophisticated video analysis

software. These are most often used for industrial applications to monitor manufacturing

processes, but they're sometimes also used in scientific applications such as high-speed video

microscopy. Your camera is a Ximea model MQ013MG-ON, which is a black-and-white 1280 x

1024 pixel camera that can record up to 170 frames per second.

Your camera is equipped with a varifocal lens. This is similar to a zoom lens, in that the focal

length (i.e. the magnification) can be set within some range of values. Both a zoom and a

varifocal lens have two focusing adjustments. The difference is that one of the adjustments on a

zoom lens adjusts the focal length only – so that the image remains in focus as the focal length is

adjusted. On a varifocal lens the two adjustments are not orthogonal, so you always have to

move both adjustments if you want to change the focal length. The camera and lens will be

supported on a tall "ring-stand" consisting of a tall ½" post on a 5"x 7" base.

1. Set up your masses and springs

To begin the experiment, take three ring stands, one 80-20 frame, and one of the large white

cardboard sheets. Set the frame on your lab bench with the square part of the frame towards the

back of the bench. Set two of the ringstands behind the frame, with their bases tucked under the

square frame. Set the third ringstand in the position labeled (*) in Figure 4.3(b). Finally, place

the white cardboard sheet at the very back, behind the ringstands. The purpose of this sheet is to

provide a uniform background for the video recording.

Next, select one of the mass-and-spring sets. Each set has a number. Take a note of this number

– write it down in your lab book, and include it in your report. This is important because we want

to see if you got the correct results for your particular mass-and-spring set.

The masses consist of stainless steel balls of two different sizes from the set 1-1/2", 1-1/4", 1-

1/8", or 1" diameter. Use the larger ball for mass

and the smaller ball for mass

2.m

Use the

stronger spring for spring

and the weaker spring for spring

2.k

Make sure that two 8-32

eyebolts are screwed into opposite sides of your ball

. Make sure that one 8-32 eyebolt is

screwed into your ball

2,m

and a magnet into the opposite side of your ball

2.m

The balls,

springs, and magnet should be assembled as shown in Figure 4.3(b). You won't use the magnet in

the first part of the experiment, but the magnet should be placed on the ball anyway, because it

will contribute to the mass

2,m

and you want that mass to remain constant throughout the

experiment.

For each set, we have selected springs and masses that should work well together. The

requirements are:

(i) When mass

hangs by itself from spring

, the extension of spring

should be greater

than 1 cm and less than 5 cm.

(ii) When mass

hangs by itself from spring

, the extension of spring

should be greater

than 1 cm and less than 5 cm.

(iii) When mass

, spring

and mass

hangs from spring

1,k

the extension of spring

should be greater than 2 cm and less than 8 cm. Also, when this combination hangs from the

central ¼-20 eyebolt as shown in Figure 4.3(b), you should have at least 3" of clearance between

the magnet and the bottom of the frame. Consult with your instructor if you notice these

conditions aren’t satisfied.

2. Measure the masses and spring constants

Next, measure each of your two masses

and

on the electronic scale at the front of the lab.

To keep the masses from rolling around, you can place them in a pyrex dish. (If you turn the

scale on with just the dish, it will zero out the mass of the dish.) When you measure the masses,

make sure that the 8-32 eyebolts and magnet are installed onto the masses, because the mass of

the eyebolts and magnet is a part of the mass that oscillates. (The springs also contribute a little

to the oscillating mass, but this is a tricky thing to get right for coupled oscillations, so just leave

the springs off for your mass measurements, and ignore the effect of the spring masses for this

lab.)

Next, we want to determine the spring constants

and

2.k

You might think we could use

Hooke's law

mg kx

for this, where x is the extension, but that turns out to be a poor strategy.

One problem is that x is difficult to measure accurately. Another is that it's not true that

mg kx

for these springs. The reason is that they are under a non-zero compression force

when

unloaded. It takes a force greater than

to get a non-zero extension, so there is an offset in

Hooke's law for these springs. The correct force law is

0.mg F kx

We'll use a different strategy: to measure the frequency



of the oscillation of each mass on its

own spring. This works much better because the frequency can be measured quite accurately,

and then we can use the result





To get started with this, hang spring

from the central eyebolt on the frame, and hang mass

from spring

1.k

Make sure that both eyebolts are installed on

1,m

because we want this mass to

remain constant throughout the experiment. Now,

and

constitute a simple harmonic

oscillator. (Leave

and

set aside.)

Then, pull the mass

down slightly and let go, so that you set it into oscillation. Using your

stopwatch, measure the time it takes to complete 50 periods of oscillation, and from that

measurement determine the oscillation frequency. Remember that

2 / ,T





where T is the

period, since there are



radians in one cycle. You should be able to measure



to better than

1% accuracy in this way. (Say, a relative accuracy better than ½ period/50 periods.)

Repeat this for mass

suspended from the center support by spring

2.k

(Mass

and spring

should be set aside while you do this.) Also make sure that the magnet is attached to mass

2,m

since you want that mass to be constant throughout the experiment.

At this point, you should have good measurements for

1 1 2 2

, , , and .m k m k

3. Learn to record and track a video of an oscillating mass, and re-measure

Next, remove mass

and spring

from the frame, and hang mass

from spring

again.

Then, attach the post-mounted light bulb to the ringstand with a right angle clamp as shown in

Figure 4.3(a). Plug the light bulb supply into an electrical outlet, and make sure the bulb

illuminates. Next, set up your camera and lens on the ringstand as shown in the figure. You

should use two right angle clamps and posts, so that the camera is oriented as shown. Then

position the ringstand so that the camera faces your masses. Next, connect the micro-B end of

the USB 3.0 cable into the camera, and the A end of the cable into the back of the Mac computer.

Please be gentle when making the connection to the camera so that you don't stress or

damage the connector.

Next, log in to the computer, and start the program "XiCamTool", which you can do via its

orange-colored icon along the bottom row of your screen. This is the program to operate and take

video from the camera. When the program comes up, it should automatically detect the camera.

If this has happened, you should see an orange arrow in the upper left corner of the window, and

you should see the camera model "MQ013MG-ON" indicated in the right hand column. If you

do not see these, try unplugging the USB cable and plugging it back in. If that doesn't work,

consult with your instructor. Next, press the orange arrow. This will take in a continuous stream

of images from the camera and display it.

The camera lens has three adjustments. The front two adjust the lens focal parameters (i.e. focal

length (magnification) and focus). The rear one adjusts the aperture, (i.e the amount of light

collected by the camera). Note that the little levers screw in and out in order to secure or loosen

the lens adjustments. Adjust the vertical and horizontal position of the camera and the two focus

adjustments until you see a clear image of your mass. For tracking, bigger (more magnification)

is not necessarily better. The reason is that the more pixels the tracker has to deal with, the

slower it goes. For this reason, you may want to select a relatively low magnification.

Next, uncheck the "Auto exposure" box under the "Settings" tab. Then, select “Frame Rate” from

the drop down menu next to “Control FPS” under the “Performance” tab. At this point, you can

independently adjust the frame rate using the "Control FPS" slider, and the exposure time using

the "Exposure" slider. (This is subject to the constraint that the exposure time must be less than

the inverse of the frame rate, which is enforced automatically.) Another slider allows you to

adjust the electronic gain of the camera.

Below, you'll be using automatic video tracking. One of the most difficult parts of this

experiment is to get the automatic tracking working well. The most effective strategy is to track a

small feature that has a consistent shape from frame to frame, and has very high contrast.

Tracking the entire ball has the problem that the tracking tends to be very slow, due to the

relatively large number of pixels involved. We have come up with the following strategy which



works reasonably well. We've chosen masses that are polished, reflective spheres, and we've

placed a small light bulb in front of them. You should see the reflection of this bulb in your video

as a compact, bright spot near the center of your ball. This spot is nearly ideal for the video

tracking. And, since the ball is spherical, the spot doesn't change its appearance with rotations of

the ball.

You now have manual control over the brightness of the image, using three controls: (1) the

camera aperture, (2) the exposure time, and (3) the gain of the camera (this controls an electronic

amplifier that boosts the signal from each pixel). Keep the exposure time set somewhere in the

range from 2 ms to 5 ms. We don’t want longer exposures, because that can result in blurring of

the bright spot due to the ball’s motion. On the other hand, exposures less than 2 ms result in a

loss of brightness without any real benefit.

After setting the exposure time, adjust the camera aperture and gain in some combination that

produces a clear image of the ball that has good contrast. You especially want to see good

contrast between the bright spot and the surrounding ball surface.

When you record video, you would like the frame rate to be fast enough to record something like

10 frames per cycle of oscillation or more. On the other hand, you'd like to follow a number of

cycles of oscillation without accumulating too many frames, because the tracker may take a long

time to complete tracking of a large number of frames. For this reason, it is best to select a frame

rate that is neither large nor small compared to 10 frames per cycle of oscillation. Your

oscillation frequency is likely to be between 1 Hz and 4 Hz for the single masses, so 40 fps

would be a maximum frame rate at this point. For now, set the frame rate to about 30 fps using

the Control FPS slider. Later, you can adjust this if you want more or fewer frames per second.

At this point, you should be looking at mass

hanging from spring

, and mass

and

spring

should be set aside. Return to this condition if you aren’t. Make sure none of the

tapped holes on the sides of the ball faces the camera. Then, record and save a video of the

oscillation of the mass. To do this, click on the "Record Loop" icon along the top row of the

window. Set the number of frames to 200 to start with. Pull down slightly on your mass and let

go, so as to set it into oscillation. Click "Start". When the circle on the left has completed, check

"Stop." This will record a video with 200 frames into memory. To save this video, select the

diskette icon. Under "Files of type", select “.MOV". Type in a descriptive filename, then click

"Save." Note where the file is saved (probably in your “Documents” folder).

Throughout this experiment, during any file save or file conversion, wait until the save or

conversion completes before moving on. If you don’t, you may end up with a truncated video

file.

Next, open the folder with your video, and keep it open throughout the experiment. This is the

easiest way to work with these files.

Next, start the program "Tracker" by clicking on its light blue, pink, and purple icon. Then, go to

Edit → Preferences → Video. Under “Video Engine”, select “Xuggle,” and click “Save.” (You

have to make this selection if you want Tracker to be able to work well with your .MOV format

movies.)

Drag and drop the .MOV file you just produced into the open Tracker window. If you get a

warning about frame durations, ignore it. You can now view the video with the video player

controls at the bottom of the window. Check that the video plays properly in Tracker. Then

return your movie to its first frame in the player window before proceeding to the next step.

We'll next use Tracker's Autotracker feature. To do this, click on "Create" and select "Point

Mass". Then press and hold cntrl-shift. You will see a cross-hair pattern on the screen. While

holding down cntrl-shift, position the exact center of the cross hair on the bright spot on your ball

and click. This will open up the Autotracker window. (If cntrl-shift doesn’t work, you can also

open Autotracker from Tracker’s menus.) Move this window so you can see both it and your

ball. On your ball, you'll see a small red circle in the middle of a red square. The small red circle

selects the video feature that is to be tracked. The red square selects the area of the video that is

to be searched for this feature. You can see the appearance of the tracked feature in the

"Template" image in the Autotracker window. For each successive frame, the Autotracker

searches for an image that matches this template. To save time, it searches only within the square

search window, not in the entire video frame.

If Tracker finds the feature, then it records the x (horizontal) and y (vertical) coordinate of the

feature as the location of the "point mass" that you created. If it can't find the feature, it stops and

asks you what to do. For this lab, we'll try to avoid this by producing videos that reliably

autotrack.

You can enlarge or shrink the video template area and search area by clicking and dragging on

them. There is a compromise to be struck. Increasing one or both areas may (or may not) result

in more reliable autotracking, but at the cost of increased tracking time. The default for the area

tracked might seem too small, but often it works just fine. That is because Tracker moves this

area as needed to keep up with the moving object.

The main thing you want is a highly distinct bright spot, surrounded by some dark area on all

sides, in the Template window. As long as you have this, the tracking will probably work well.

Adjust the Autotracker until you do have this condition. Then press "Search". Hopefully, the

program will then track through your entire video. If it doesn't, one or more of the following

steps might help:

* Check that you have a 2 to 5 ms exposure time, so that you don't get smearing out of the spot

due to motion.

* Make sure that the tapped holes in the sides of the ball are off to the side of the camera image.

If a hole is near the front of the ball, it may interfere with the reflected light bulb spot.

* Increase or decrease the template area or search area.

* Block out or turn off lights other than your light bulb that give stray reflections. (For instance,

try placing a cardboard sheet above your camera and oscillators.)

* Take a video with less brightness, in order to improve contrast. Or, try a video with more

brightness, so as to saturate the bright spot (and reduce variation in its appearance).

* Try the other side of the ball, or switch your ball for one of the same size with a better polish.

Again, your goal is to have a template consisting of a bright spot in the middle of a dark area,

that is reasonably consistent throughout the video. If you have difficulty in getting good tracking

after some effort, consult with your instructor.

At this point, you will be presented with a graph of x vs. time t. This is not what you want since

x is the horizontal coordinate. Click on the x, and you'll be presented with a series of choices for

that axis of the graph. Select "y". Now you have a graph of the oscillation in displacement y vs

time t. It should look like an accurate sinusoid. You also have a table of data values for x, y, and

t. At this point you can maximize the size of the graph by clicking on the up arrow, or similarly

you can return the graph to its smaller size.

Next, right-click on the graph and select "Analyze." Then, click on "Analyze" and select "Fourier

Spectrum." You should see a Fourier Power spectrum with one peak at non-zero frequency, both

in the graph and in the data. To make it clear in the graph, you can reset the vertical scale by

clicking on the top entry on the vertical scale, and similarly for the horizontal scale. You'll

probably want to reduce the maximum plotted vertical value because there is usually a large but

uninteresting zero-frequency peak associated with the offset of your sine wave. You'll probably

also want to reduce the maximum plotted frequency.

Check that the frequency of the peak in the Fourier spectrum agrees with the value you measured

earlier for



If it doesn’t, figure out where you’ve gone wrong. Remember that frequency in

radians per second is equal to



times frequency in Hz.

4. Measure the frequencies of the coupled oscillation.

Next, set up the double mass and spring oscillator illustrated in Figure 4.3. View the double

oscillator with XiCamTool. Adjust the camera field of view and light bulb so that you can see

both balls clearly, with a bright spot in the middle of each. Then record a video of the coupled

oscillation using the method discussed in the last section. For this video, start with 1000 frames

and a frame rate of about 50 frames per second. (You can adjust either of these in a second video

if you want.) The reason for the increased frame rate is that the highest mode frequency will be

larger than the frequencies you measured earlier. The reason to have a video with more frames is

that you will get higher frequency resolution from the Fourier Transform of the ball’s track

position data. A good method to get the coupled oscillation going is to displace

upward

slightly while holding

stationary, and then let go of both.

Then, save the video as discussed above, and load it into Tracker. Make a track of one of the

two balls, and display y vs. t for that ball. (The tracking might take a few minutes.) You’re now

looking at coupled oscillation of the two masses, that is a superposition of both of its modes,

mathematically described by equations (4.12) and (4.13). Take note of the qualitative appearance

of this data. Does it look like a sine wave? Or completely chaotic? Or does it differ from a sine

wave, yet still have come regularities?

Next, use the Fourier Transform tool to calculate and display the Fourier power spectrum of y vs.

t. You should see a power spectrum with two peaks at non-zero frequency. Once you can see

both peaks, determine their centroids with good accuracy from the actual data values, which are

tabulated as "frequency" and "power". (Here, "power" means the value of the Fourier power

spectrum.) One way to do this is to copy a set of data points for "frequency" and "power"

extending from five to ten data points below a peak to five to ten points above the peak from

Tracker’s table of data values, and to paste them into an Excel spreadsheet. (Use command-c and

command-v to cut and paste on a Mac.) Once you have the data in Excel, you should be able to

calculate the centroid (weighted average frequency) of the peak relatively easily. Use this

centroid for your measured mode frequency.

Once you've determined the frequency of these two modes, check whether they agree with what

you'd predict based on your measured values of

1 2 1 2

, , , and ,m m k k

using equations (4.10) and

(4.11).

5. Excite one mode at a time, observe the single-mode oscillations, and measure

the relative displacements for single mode oscillation

Next, observe single mode vibrations of your coupled oscillator. To do this, connect your coil to

Output 1 of the Pasco 850 interface. Make sure that your current passes through both the 5



resistor and the coil in series. Position the coil just below the magnet. This seems to work best

with a gap of perhaps 4 to 8 mm between the coil and the magnet. Using Pasco Capstone, set the

Pasco 850 to 1 V voltage amplitude, and frequency of about 0.5 Hz. You should be able to see a

small mechanical response of your coupled oscillator to this current. Once you’re sure it’s

working, reduce the voltage amplitude to 0.3 V. Next, use the Capstone controls to tune the

frequency of the oscillator near your measured frequency





Don't forget that you probably got





in units of radians per second, whereas the Capstone frequency is specified in Hz, which

differs by a factor of 1/



See if you can excite just one mode of vibration, so that both masses

vibrate only with frequency





When you do this, you should press the cardboard strip lightly

against one mass. The friction of the ball against the cardboard will reduce the Q of the

oscillation. Without this, the decay time of the oscillation can easily be several minutes. Thus,

when you make a change to the system in the absence of damping, it can take several minutes for

the system to "forget" its previous condition of oscillation, and come into equilibrium for the

new condition. In other words, more damping means the system will respond more quickly to

any changes that you make. A damping time of something like 10 to 20 seconds would be

reasonable. You will probably also want to reduce the drive amplitude when you are close to

resonance.

When completing this part of the lab, make sure you're really seeing a resonance. If you are, you

should be able to tune something like 0.3 Hz away from the mode frequency, and the vibrations

of the masses should decrease substantially. If they don't, then you are not driving a single mode

oscillation on resonance. There are two reasons why you might see oscillations, but not

resonance: (i) you don't have enough damping, so the masses continue to oscillate for a long time

regardless of what you do with the frequency, or (ii) you have your voltage turned up way too

high. (If you have your amplitude set to 1 V or more, that is too high.)

When you have succeeded in getting a single mode vibration going at frequency





record a

video of the oscillation, load it into Tracker, and autotrack the displacement of one of the two

balls. You don't need lots of frames for this part, 200 frames would be plenty. Then autotrack the

displacement of the other ball. You can do this by pressing "create" and selecting "point mass" a

second time, and then running Autotrack a second time on the second ball. This will give you

one set of data labelled "mass A" and one labelled "mass B", which you can select for any of the

plotting or analysis functions.

Next, measure the amplitudes

A

and

A

of the vibrations of the two balls. Ideally, you’ll do

this with a fit to your data as discussed below. However the fitting in Tracker is pretty finicky, so

to start, just measure the amplitudes from the screen display, or by printing out your graphs on

paper and measuring amplitudes with a ruler (relative to the graph scale) (better). It would be a

little better to obtain your amplitudes from a fit as discussed below, but for this lab just

measuring amplitudes from the graph will be acceptable. (We’ll take off only a small bit of credit

if you don’t do the full fitting.)

[Fitting your data: To carry out a fit with Tracker, right-click on the graph and select "Analyze."

Click on "Analyze" and select "Curve Fits". Under "Fit Name," select "Sinusoid". This fit

function won't work because it lacks an offset from zero. To correct this, click on "Fit Builder."

Under "Parameters", click "Add." Edit the variable field "param" to read "D". Scroll Down and

double-click the expression. Edit it to read "A*sin(B*t+C)+D". Then press close.

Next select the data that you want to fit by clicking in the data field on the right. Selected data

points are yellow. You can select all or only part of the data. To select all points, right click on

the data area and select "Select All". Then, get a good fit to the data by some combination of

adjusting fit parameters by hand, and by checking "Autofit". Unfortunately, this fitting routine is

not good at automatically finding the best fit parameters. So, in order to get the Autofit to work,

you'll have to leave the Autofit unchecked, tweak the fit parameters by hand until the fit function

is quite close to the data, and then check Autofit. You'll know you have a good fit when the pink

curve goes through the data points.

An alternative would be to fit your data in another numerical environment such as Mathematica,

Matlab, or Python. ]

Check that your frequency





is the same as the frequency





that you measured earlier with the

Fourier transform. Verify that the two masses vibrate with the same phase. Finally, check

whether your measured ratio

/AA



agrees with the theoretical ratio (4.15).

Repeat the previous procedure for the single mode with frequency





Again check whether





is the same as the frequency you measured earlier. Check how well your measured amplitude

ratio

/AA



agrees with theory. In your report, give a qualitative description of the difference

between the two modes.

Experiment 4 Lab Manual Phys 115L Spring 2018

Navigation menu

Versions of this User Manual:

Views

Navigation