Instructor Solutions Manual For Physics Volume 1

Instructor%20Solutions%20Manual%20for%20Physics%20Volume%201

User Manual: Pdf

Open the PDF directly: View PDF .
Page Count: 313 [warning: Documents this large are best viewed by clicking the View PDF Link!]

Instructor Solutions Manual

for

Physics

Halliday, Resnick, and Krane

Paul Stanley

Beloit College

Volume 1: Chapters 1-24

A Note To The Instructor...

The solutions here are somewhat brief, as they are designed for the instructor, not for the student.

Check with the publishers before electronically posting any part of these solutions; website, ftp, or

server access must be restricted to your students.

I have been somewhat casual about subscripts whenever it is obvious that a problem is one

dimensional, or that the choice of the coordinate system is irrelevant to the numerical solution.

Although this does not change the validity of the answer, it will sometimes obfuscate the approach

if viewed by a novice.

There are some traditional formula, such as

x=v2

0x+ 2axx,

which are not used in the text. The worked solutions use only material from the text, so there may

be times when the solution here seems unnecessarily convoluted and drawn out. Yes, I know an

easier approach existed. But if it was not in the text, I did not use it here.

I also tried to avoid reinventing the wheel. There are some exercises and problems in the text

which build upon previous exercises and problems. Instead of rederiving expressions, I simply refer

you to the previous solution.

I adopt a diﬀerent approach for rounding of signiﬁcant ﬁgures than previous authors; in partic-

ular, I usually round intermediate answers. As such, some of my answers will diﬀer from those in

the back of the book.

Exercises and Problems which are enclosed in a box also appear in the Student’s Solution Manual

with considerably more detail and, when appropriate, include discussion on any physical implications

of the answer. These student solutions carefully discuss the steps required for solving problems, point

out the relevant equation numbers, or even specify where in the text additional information can be

found. When two almost equivalent methods of solution exist, often both are presented. You are

encouraged to refer students to the Student’s Solution Manual for these exercises and problems.

However, the material from the Student’s Solution Manual must not be copied.

Paul Stanley

Beloit College

stanley@clunet.edu

E1-1 (a) Megaphones; (b) Microphones; (c) Decacards (Deck of Cards); (d) Gigalows (Gigolos);

(e) Terabulls (Terribles); (f) Decimates; (g) Centipedes; (h) Nanonanettes (?); (i) Picoboos (Peek-a-

Boo); (j) Attoboys (’atta boy); (k) Two Hectowithits (To Heck With It); (l) Two Kilomockingbirds

(To Kill A Mockingbird, or Tequila Mockingbird).

E1-2 (a) $36,000/52 week = $692/week. (b) $10,000,000/(20 ×12 month) = $41,700/month. (c)

30 ×109/8 = 3.75 ×109.

E1-3 Multiply out the factors which make up a century.

1 century = 100 years 365 days

1 year 24 hours

1 day 60 minutes

1 hour 

This gives 5.256 ×107minutes in a century, so a microcentury is 52.56 minutes.

The percentage diﬀerence from Fermi’s approximation is (2.56 min)/(50 min) ×100% or 5.12%.

E1-4 (3000 mi)/(3 hr) = 1000 mi/timezone-hour. There are 24 time-zones, so the circumference

is approximately 24 ×1000 mi = 24,000 miles.

E1-5 Actual number of seconds in a year is

(365.25 days) 24 hr

1 day 60 min

1 hr  60 s

1 min= 3.1558 ×107s.

The percentage error of the approximation is then

3.1416 ×107s−3.1558 ×107s

3.1558 ×107s=−0.45 %.

E1-6 (a) 10−8seconds per shake means 108shakes per second. There are

365 days

1 year 24 hr

1 day 60 min

1 hr  60 s

1 min= 3.1536 ×107s/year.

This means there are more shakes in a second.

(b) Humans have existed for a fraction of

106years/1010 years = 10−4.

That fraction of a day is

10−4(24 hr) 60 min

1 hr  60 s

1 min= 8.64 s.

E1-7 We’ll assume, for convenience only, that the runner with the longer time ran exactly one

mile. Let the speed of the runner with the shorter time be given by v1, and call the distance actually

ran by this runner d1. Then v1=d1/t1. Similarly, v2=d2/t2for the other runner, and d2= 1 mile.

We want to know when v1> v2. Substitute our expressions for speed, and get d1/t1> d2/t2.

Rearrange, and d1/d2> t1/t2or d1/d2>0.99937. Then d1>0.99937 mile ×(5280 feet/1 mile) or

d1>5276.7 feet is the condition that the ﬁrst runner was indeed faster. The ﬁrst track can be no

more than 3.3 feet too short to guarantee that the ﬁrst runner was faster.

E1-8 We will wait until a day’s worth of minutes have been gained. That would be

(24 hr) 60 min

1 hr = 1440 min.

The clock gains one minute per day, so we need to wait 1,440 days, or almost four years. Of course,

if it is an older clock with hands that only read 12 hours (instead of 24), then after only 720 days

the clock would be correct.

E1-9 First ﬁnd the “logarithmic average” by

log tav =1

2log(5 ×1017) + log(6 ×10−15),

2log 5×1017 ×6×10−15,

2log 3000 = log √3000.

Solve, and tav = 54.8 seconds.

E1-10 After 20 centuries the day would have increased in length by a total of 20 ×0.001 s = 0.02 s.

The cumulative eﬀect would by the product of the average increase and the number of days; that

average is half of the maximum, so the cumulative eﬀect is 1

2(2000)(365)(0.02 s) = 7300 s. That’s

about 2 hours.

E1-11 Lunar months are based on the Earth’s position, and as the Earth moves around the orbit

the Moon has farther to go to complete a phase. In 27.3 days the Moon may have orbited through

360◦, but since the Earth moved through (27.3/365) ×360◦= 27◦the Moon needs to move 27◦

farther to catch up. That will take (27◦/360◦)×27.3 days = 2.05 days, but in that time the Earth

would have moved on yet farther, and the moon will need to catch up again. How much farther?

(2.05/365) ×360◦= 2.02◦which means (2.02◦/360◦)×27.3 days = 0.153 days. The total so far is

2.2 days longer; we could go farther, but at our accuracy level, it isn’t worth it.

E1-12 (1.9 m)(3.281 ft/1.000 m) = 6.2 ft, or just under 6 feet, 3 inches.

E1-13 (a) 100 meters = 328.1 feet (Appendix G), or 328.1/3 = 10.9 yards. This is 28 feet longer

than 100 yards, or (28 ft)(0.3048 m/ft) = 8.5 m. (b) A metric mile is (1500 m)(6.214×10−4mi/m) =

0.932 mi.I’d rather run the metric mile.

E1-14 There are

300,000 years 365.25 days

1 year 24 hr

1 day 60 min

1 hr  60 s

1 min= 9.5×1012s

that will elapse before the cesium clock is in error by 1 s. This is almost 1 part in 1013. This kind

of accuracy with respect to 2572 miles is

10−13(2572 mi) 1609 m

1 mi = 413 nm.

E1-15 The volume of Antarctica is approximated by the area of the base time the height; the

area of the base is the area of a semicircle. Then

V=Ah =1

2πr2h.

The volume is

V=1

2(3.14)(2000 ×1000 m)2(3000 m) = 1.88 ×1016 m3

= 1.88 ×1016 m3×100 cm

1 m 3

= 1.88 ×1022 cm3.

E1-16 The volume is (77×104m2)(26 m) = 2.00×107m3. This is equivalent to

(2.00×107m3)(10−3km/m)3= 0.02 km3.

E1-17 (a) C= 2πr = 2π(6.37 ×103km) = 4.00 ×104km. (b) A= 4πr2= 4π(6.37 ×103km)2=

5.10 ×108km. (c) V=4

3π(6.37 ×103km)3= 1.08 ×1012 km3.

E1-18 The conversions: squirrel, 19 km/hr(1000 m/km)/(3600 s/hr) = 5.3 m/s;

rabbit, 30 knots(1.688ft/s/knot)(0.3048 m/ft) = 15 m/s;

snail, 0.030 mi/hr(1609 m/mi)/(3600 s/hr) = 0.013 m/s;

spider, 1.8 ft/s(0.3048 m/ft) = 0.55 m/s;

cheetah, 1.9 km/min(1000 m/km)/(60 s/min) = 32 m/s;

human, 1000 cm/s/(100 cm/m) = 10 m/s;

fox, 1100 m/min/(60 s/min) = 18 m/s;

lion, 1900 km/day(1000 m/km)/(86,400 s/day) = 22 m/s.

The order is snail, spider, squirrel, human, rabbit, fox, lion, cheetah.

E1-19 One light-year is the distance traveled by light in one year, or (3 ×108m/s) ×(1 year).

Then

19,200mi

hr light-year

(3 ×108m/s) ×(1 year) 1609 m

1 mi  1 hr

3600 s100 year

1 century ,

which is equal to 0.00286 light-year/century.

E1-20 Start with the British units inverted,

gal

30.0 mi 231 in3

gal 1.639 ×10−2L

in3 mi

1.609 km= 7.84 ×10−2L/km.

E1-21 (b) A light-year is

(3.00 ×105km/s) 3600 s

1 hr 24 hr

1 day (365 days) = 9.46 ×1012 km.

A parsec is

1.50 ×108km

0◦00100 360◦

2πrad=1.50 ×108km

(1/3600)◦360◦

2πrad= 3.09 ×1013 km.

(a) (1.5×108km)/(3.09 ×1013 km/pc = 4.85 ×10−6pc. (1.5×108km)/(9.46 ×1012 km/ly) =

1.59 ×10−5ly.

E1-22 First ﬁnd the “logarithmic average” by

log dav =1

2log(2 ×1026) + log(1 ×10−15),

2log 2×1026 ×1×10−15,

2log 2 ×1011 = log p2×1011.

Solve, and dav = 450 km.

E1-23 The number of atoms is given by (1 kg)/(1.00783 ×1.661 ×10−27 kg), or 5.974 ×1026

atoms.

E1-24 (a) (2 ×1.0 + 16)u(1.661 ×10−27kg) = 3.0×10−26kg.

(b) (1.4×1021kg)/(3.0×10−26kg) = 4.7×1046 molecules.

E1-25 The coﬀee in Paris costs $18.00 per kilogram, or

$18.00 kg−10.4536 kg

1 lb = $8.16 lb−1.

It is cheaper to buy coﬀee in New York (at least according to the physics textbook, that is.)

E1-26 The room volume is (21 ×13 ×12)ft3(0.3048 m/ft)3= 92.8 m3. The mass contained in the

room is

(92.8 m3)(1.21 kg/m3) = 112 kg.

E1-27 One mole of sugar cubes would have a volume of NA×1.0 cm3, where NAis the Avogadro

constant. Since the volume of a cube is equal to the length cubed, V=l3, then l=3

√NAcm

= 8.4×107cm.

E1-28 The number of seconds in a week is 60 ×60 ×24 ×7 = 6.05 ×105. The “weight” loss per

second is then

(0.23 kg)/(6.05 ×105s) = 3.80 ×10−1mg/s.

E1-29 The deﬁnition of the meter was wavelengths per meter; the question asks for meters per

wavelength, so we want to take the reciprocal. The deﬁnition is accurate to 9 ﬁgures, so the reciprocal

should be written as 1/1,650,763.73 = 6.05780211 ×10−7m = 605.780211 nm.

E1-30 (a) 37.76 + 0.132 = 37.89. (b) 16.264 −16.26325 = 0.001.

E1-31 The easiest approach is to ﬁrst solve Darcy’s Law for K, and then substitute the known

SI units for the other quantities. Then

K=V L

AHt has units of m3(m)

(m2) (m) (s)

which can be simpliﬁed to m/s.

E1-32 The Planck length, lP, is found from

[lP] = [ci][Gj][hk],

L = (LT−1)i(L3T−2M−1)j(ML2T−1)k,

= Li+3j+2kT−i−2j−kM−j+k.

Equate powers on each side,

L: 1 = i+ 3j+ 2k,

T: 0 = −i−2j−k,

M: 0 = −j+k.

Then j=k, and i=−3k, and 1 = 2k; so k= 1/2, j= 1/2, and i=−3/2. Then

[lP] = [c−3/2][G1/2][h1/2],

= (3.00 ×108m/s)−3/2(6.67 ×10−11 m3/s2·kg)1/2(6.63 ×10−34 kg ·m2/s)1/2,

= 4.05 ×10−35 m.

E1-33 The Planck mass, mP, is found from

[mP] = [ci][Gj][hk],

M = (LT−1)i(L3T−2M−1)j(ML2T−1)k,

= Li+3j+2kT−i−2j−kM−j+k.

Equate powers on each side,

L: 0 = i+ 3j+ 2k,

T: 0 = −i−2j−k,

M: 1 = −j+k.

Then k=j+ 1, and i=−3j−1, and 0 = −1+2k; so k= 1/2, and j=−1/2, and i= 1/2. Then

[mP] = [c1/2][G−1/2][h1/2],

= (3.00 ×108m/s)1/2(6.67 ×10−11 m3/s2·kg)−1/2(6.63 ×10−34 kg ·m2/s)1/2,

= 5.46 ×10−8kg.

P1-1 There are 24 ×60 = 1440 traditional minutes in a day. The conversion plan is then fairly

straightforward

822.8 dec. min 1440 trad. min

1000 dec. min = 1184.8 trad. min.

This is traditional minutes since midnight, the time in traditional hours can be found by dividing

by 60 min/hr, the integer part of the quotient is the hours, while the remainder is the minutes. So

the time is 19 hours, 45 minutes, which would be 7:45 pm.

P1-2 (a) By similar triangles, the ratio of the distances is the same as the ratio of the diameters—

390:1.

(b) Volume is proportional to the radius (diameter) cubed, or 3903= 5.93 ×107.

3500 km.

P1-3 (a) The circumference of the Earth is approximately 40,000 km; 0.5 seconds of an arc is

0.5/(60 ×60 ×360) = 3.9×10−7of a circumference, so the north-south error is ±(3.9×10−7)(4 ×

107m) = ±15.6 m. This is a range of 31 m.

(b) The east-west range is smaller, because the distance measured along a latitude is smaller than

the circumference by a factor of the cosine of the latitude. Then the range is 31 cos 43.6◦= 22 m.

P1-4 Your position is determined by the time it takes for your longitude to rotate ”underneath”

the sun (in fact, that’s the way longitude was measured originally as in 5 hours west of the Azores...)

the rate the sun sweep over at equator is 25,000 miles/86,400 s = 0.29 miles/second. The correction

factor because of latitude is the cosine of the latitude, so the sun sweeps overhead near England at

approximately 0.19 mi/s. Consequently a 30 mile accuracy requires an error in time of no more than

(30 mi)/(0.19 mi/s) = 158 seconds.

Trip takes about 6 months, so clock accuracy needs to be within (158 s)/(180 day) = 1.2 sec-

onds/day.

(b) Same, except 0.5 miles accuracy requires 2.6 s accuracy, so clock needs to be within 0.007

s/day!

P1-5 Let Bbe breaths/minute while sleeping. Each breath takes in (1.43 g/L)(0.3 L) = 0.429 g;

and lets out (1.96 g/L)(0.3 L) = 0.288 g. The net loss is 0.141 g. Multiply by the number of breaths,

(8 hr)(60 min./hr)B(0.141 g) = B(67.68 g). I’ll take a short nap, and count my breaths, then ﬁnish

the problem.

I’m back now, and I found my breaths to be 8/minute. So I lose 541 g/night, or about 1 pound.

P1-6 The mass of the water is (1000 kg/m3)(5700 m3) = 5.7×106kg. The rate that water leaks

drains out is (5.7×106kg)

(12 hr)(3600 s/hr) = 132 kg/s.

P1-7 Let the radius of the grain be given by rg. Then the surface area of the grain is Ag= 4πr2

and the volume is given by Vg= (4/3)πr3

If Ngrains of sand have a total surface area equal to that of a cube 1 m on a edge, then

NAg= 6 m2. The total volume Vtof this number of grains of sand is NVg. Eliminate Nfrom these

two expressions and get

Vt=NVg=(6 m2)

Vg=(6 m2)rg

Then Vt= (2 m2)(50 ×10−6m) = 1 ×10−4m3.

The mass of a volume Vtis given by

1×10−4m32600 kg

1 m3= 0.26 kg.

P1-8 For a cylinder V=πr2h, and A= 2πr2+ 2πrh. We want to minimize Awith respect to

changes in r, so

dr =d

dr 2πr2+ 2πr V

πr2,

= 4πr −2V

r2.

Set this equal to zero; then V= 2πr3. Notice that h= 2rin this expression.

P1-9 (a) The volume per particle is

(9.27 ×10−26kg)/(7870 kg/m3) = 1.178 ×10−28m3.

The radius of the corresponding sphere is

r=3

r3(1.178 ×10−28m3)

4π= 1.41 ×10−10m.

Double this, and the spacing is 282 pm.

(b) The volume per particle is

(3.82 ×10−26kg)/(1013 kg/m3) = 3.77 ×10−29m3.

The radius of the corresponding sphere is

r=3

r3(3.77 ×10−29m3)

4π= 2.08 ×10−10m.

Double this, and the spacing is 416 pm.

P1-10 (a) The area of the plate is (8.43 cm)(5.12 cm) = 43.2 cm2. (b) (3.14)(3.7 cm)2= 43 cm2.

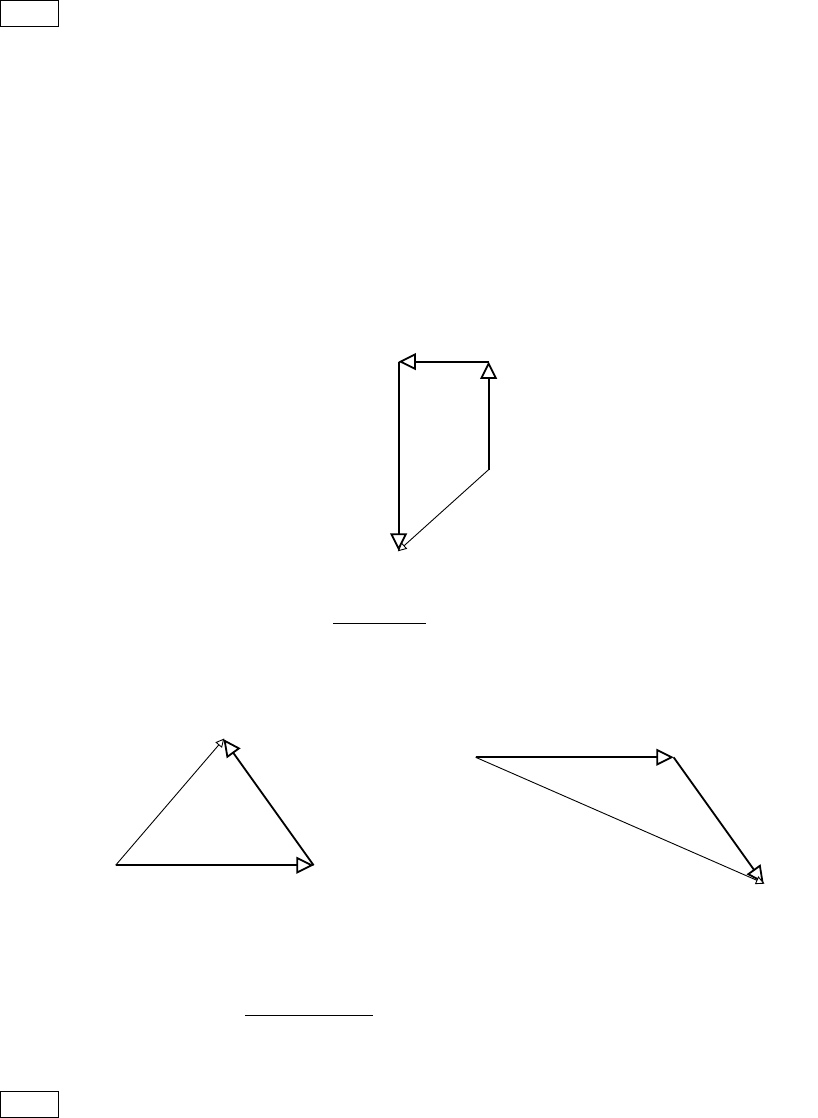
E2-1 Add the vectors as is shown in Fig. 2-4. If ~

ahas length a= 4 m and ~

bhas length b= 3 m

then the sum is given by ~

s. The cosine law can be used to ﬁnd the magnitude sof ~

s2=a2+b2−2ab cos θ,

where θis the angle between sides aand bin the ﬁgure.

(a) (7 m)2= (4 m)2+ (3 m)2−2(4 m)(3 m) cos θ, so cos θ=−1.0, and θ= 180◦. This means

that ~

aand ~

bare pointing in the same direction.

(b) (1 m)2= (4 m)2+ (3 m)2−2(4 m)(3 m) cos θ, so cos θ= 1.0, and θ= 0◦. This means that

aand ~

bare pointing in the opposite direction.

and ~

bare pointing at right angles to each other.

E2-2 (a) Consider the ﬁgures below.

(b) Net displacement is 2.4 km west, (5.2−3.1 = 2.1) km south. A bird would ﬂy

p2.42+ 2.12km = 3.2 km.

E2-3 Consider the ﬁgure below.

ba+b

-b

a-b

E2-4 (a) The components are (7.34) cos(252◦) = −2.27ˆ

iand (7.34) sin(252◦) = −6.98ˆ

(b) The magnitude is p(−25)2+ (43)2= 50; the direction is θ= tan−1(43/−25) = 120◦. We

did need to choose the correct quadrant.

E2-5 The components are given by the trigonometry relations

O=Hsin θ= (3.42 km) sin 35.0◦= 1.96 km

and

A=Hcos θ= (3.42 km) cos 35.0◦= 2.80 km.

The stated angle is measured from the east-west axis, counter clockwise from east. So Ois measured

against the north-south axis, with north being positive; Ais measured against east-west with east

being positive.

Since her individual steps are displacement vectors which are only north-south or east-west, she

must eventually take enough north-south steps to equal 1.96 km, and enough east-west steps to

equal 2.80 km. Any individual step can only be along one or the other direction, so the minimum

total will be 4.76 km.

E2-6 Let ~

rf= 124ˆ

ikm and ~

ri= (72.6ˆ

i+ 31.4ˆ

j) km. Then the ship needs to travel

∆~

r=~

rf−~

ri= (51.4ˆ

i+ 31.4ˆ

j) km.

Ship needs to travel √51.42+ 31.42km = 60.2 km in a direction θ= tan−1(31.4/51.4) = 31.4◦west

of north.

E2-7 (a) In unit vector notation we need only add the components; ~

a+~

b= (5ˆ

i+3ˆ

j)+(−3ˆ

i+2ˆ

j) =

(5 −3)ˆ

i+ (3 + 2)ˆ

j= 2ˆ

i+ 5ˆ

(b) If we deﬁne ~

c=~

a+~

band write the magnitude of ~

cas c, then c=qc2

x+c2

y=√22+ 52=

5.39. The direction is given by tan θ=cy/cxwhich gives an angle of 68.2◦, measured counterclock-

wise from the positive x-axis.

E2-8 (a) ~

a+~

b= (4 −1)ˆ

i+ (−3 + 1)ˆ

j+ (1 + 4)ˆ

k= 3ˆ

i−2ˆ

j+ 5ˆ

(b) ~

a−~

b= (4 − −1)ˆ

i+ (−3−1)ˆ

j+ (1 −4)ˆ

k= 5ˆ

i−4ˆ

j−3ˆ

c=~

b−~

a, or ~

b−~

a= (−1−4)ˆ

i+ (1 − −3)ˆ

j+ (4 −1)ˆ

k=−5ˆ

i+ 4ˆ

j+ 3ˆ

E2-9 (a) The magnitude of ~

ais p4.02+ (−3.0)2= 5.0; the direction is θ= tan−1(−3.0/4.0) =

323◦.

(b) The magnitude of ~

bis √6.02+ 8.03= 10.0; the direction is θ= tan−1(6.0/8.0) = 36.9◦.

a+~

b= (4.0 + 6.0)ˆ

i+ (−3.0 + 8.0)ˆ

j. The magnitude of ~

a+~

bis

p(10.0)2+ (5.0)2= 11.2; the direction is θ= tan−1(5.0/10.0) = 26.6◦.

(d) The resultant vector is ~

a−~

b= (4.0−6.0)ˆ

i+ (−3.0−8.0)ˆ

j. The magnitude of ~

a−~

bis

p(−2.0)2+ (−11.0)2= 11.2; the direction is θ= tan−1(−11.0/−2.0) = 260◦.

(e) The resultant vector is ~

b−~

a= (6.0−4.0)ˆ

i+ (8.0− −3.0)ˆ

j. The magnitude of ~

b−~

ais

p(2.0)2+ (11.0)2= 11.2; the direction is θ= tan−1(11.0/2.0) = 79.7◦.

E2-10 (a) Find components of ~

a;ax= (12.7) cos(28.2◦) = 11.2, ay= (12.7) sin(28.2◦)=6.00.

Find components of ~

b;bx= (12.7) cos(133◦) = −8.66, by= (12.7) sin(133◦) = 9.29. Then

r=~

a+~

b= (11.2−8.66)ˆ

i+ (6.00 + 9.29)ˆ

j= 2.54ˆ

i+ 15.29ˆ

(b) The magnitude of ~

ris √2.542+ 15.292= 15.5.

E2-11 Consider the ﬁgure below.

E2-12 Consider the ﬁgure below.

E2-13 Our axes will be chosen so that ˆ

ipoints toward 3 O’clock and ˆ

jpoints toward 12 O’clock.

(a)

The two relevant positions are ~

ri= (11.3 cm)ˆ

iand ~

rf= (11.3 cm)ˆ

j. Then

∆~

r=~

rf−~

= (11.3 cm)ˆ

j−(11.3 cm)ˆ

(b)

The two relevant positions are now ~

ri= (11.3 cm)ˆ

jand ~

rf= (−11.3 cm)ˆ

j. Then

∆~

r=~

rf−~

= (11.3 cm)ˆ

j−(−11.3 cm)ˆ

= (22.6 cm)ˆ

(c)

The two relevant positions are now ~

ri= (−11.3 cm)ˆ

jand ~

rf= (−11.3 cm)ˆ

j. Then

∆~

r=~

rf−~

= (−11.3 cm)ˆ

j−(−11.3 cm)ˆ

= (0 cm)ˆ

E2-14 (a) The components of ~

r1are

r1x= (4.13 m) cos(225◦) = −2.92 m

and

r1y= (4.13 m) sin(225◦) = −2.92 m.

The components of ~

r2are

r1x= (5.26 m) cos(0◦) = 5.26 m

and

r1y= (5.26 m) sin(0◦) = 0 m.

The components of ~

r3are

r1x= (5.94 m) cos(64.0◦) = 2.60 m

and

r1y= (5.94 m) sin(64.0◦) = 5.34 m.

(b) The resulting displacement is

h(−2.92 + 5.26 + 2.60)ˆ

i+ (−2.92+0+5.34)ˆ

jim = (4.94ˆ

i+ 2.42ˆ

j) m.

the resulting displacement is θ= tan−1(2.42/4.94) = 26.1◦. (d) To bring the particle back to the

starting point we need only reverse the answer to (c); the magnitude will be the same, but the angle

will be 206◦.

E2-15 The components of the initial position are

r1x= (12,000 ft) cos(40◦) = 9200 ft

and

r1y= (12,000 ft) sin(40◦) = 7700 ft.

The components of the ﬁnal position are

r2x= (25,8000 ft) cos(163◦) = −24,700 ft

and

r2y= (25,800 ft) sin(163◦) = 7540 ft.

The displacement is

r=~

r2−~

r1=h(−24,700 −9,200)ˆ

i+ (7,540 −9,200)ˆ

j)i= (−33900ˆ

i−1660ˆ

j) ft.

E2-16 (a) The displacement vector is ~

r= (410ˆ

i−820ˆ

j) mi, where positive xis east and positive

yis north. The magnitude of the displacement is p(410)2+ (−820)2mi = 920 mi. The direction is

θ= tan−1(−820/410) = 300◦.

(b) The average velocity is the displacement divided by the total time, 2.25 hours. Then

vav = (180ˆ

i−360ˆ

j) mi/hr.

E2-17 (a) Evaluate ~

rwhen t= 2 s.

r= [(2 m/s3)t3−(5 m/s)t]ˆ

i+ [(6 m) −(7 m/s4)t4]ˆ

= [(2 m/s3)(2 s)3−(5 m/s)(2 s)]ˆ

i+ [(6 m) −(7 m/s4)(2 s)4]ˆ

= [(16 m) −(10 m)]ˆ

i+ [(6 m) −(112 m)]ˆ

= [(6 m)]ˆ

i+ [−(106 m)]ˆ

(b) Evaluate:

v=d~

dt = [(2 m/s3)3t2−(5 m/s)]ˆ

i+ [−(7 m/s4)4t3]ˆ

= [(6 m/s3)t2−(5 m/s)]ˆ

i+ [−(28 m/s4)t3]ˆ

Into this last expression we now evaluate ~

v(t= 2 s) and get

v= [(6 m/s3)(2 s)2−(5 m/s)]ˆ

i+ [−(28 m/s4)(2 s)3]ˆ

= [(24 m/s) −(5 m/s)]ˆ

i+ [−(224 m/s)]ˆ

= [(19 m/s)]ˆ

i+ [−(224 m/s)]ˆ

for the velocity ~

vwhen t= 2 s.

a=d~

dt = [(6 m/s3)2t]ˆ

i+ [−(28 m/s4)3t2]ˆ

= [(12 m/s3)t]ˆ

i+ [−(84 m/s4)t2]ˆ

Into this last expression we now evaluate ~

a(t= 2 s) and get

a= [(12 m/s3)(2 s)]ˆ

i+ [−(84 m/s4)(2 2)2]ˆ

= [(24 m/s2)]ˆ

i+ [−(336 m/s2)]ˆ

E2-18 (a) Let ui point north, ˆ

jpoint east, and ˆ

kpoint up. The displacement is (8.7ˆ

i+ 9.7ˆ

2.9ˆ

k) km. The average velocity is found by dividing each term by 3.4 hr; then

vav = (2.6ˆ

i+ 2.9ˆ

j+ 0.85) km/hr.

The magnitude of the average velocity is √2.62+ 2.92+ 0.852km/hr = 4.0 km/hr.

(b) The horizontal velocity has a magnitude of √2.62+ 2.92km/hr = 3.9 km/hr. The angle with

the horizontal is given by θ= tan−1(0.85/3.9) = 13◦.

E2-19 (a) The derivative of the velocity is

a= [(6.0 m/s2)−(8.0 m/s3)t]ˆ

so the acceleration at t= 3 s is ~

a= (−18.0 m/s2)ˆ

i. (b) The acceleration is zero when (6.0 m/s2)−

(8.0 m/s3)t= 0, or t= 0.75 s. (c) The velocity is never zero; there is no way to “cancel” out the

ycomponent. (d) The speed equals 10 m/s when 10 = pv2

x+ 82, or vx=±6.0 m/s. This happens

when (6.0 m/s2)−(8.0 m/s3)t=±6.0 m/s, or when t= 0 s.

E2-20 If vis constant then so is v2=v2

x+v2

y. Take the derivative;

2vx

dtvx+ 2vy

dtvy= 2(vxax+vyay).

But if the value is constant the derivative is zero.

E2-21 Let the actual ﬂight time, as measured by the passengers, be T. There is some time

diﬀerence between the two cities, call it ∆T= Namulevu time - Los Angeles time. The ∆Twill be

positive if Namulevu is east of Los Angeles. The time in Los Angeles can then be found from the

time in Namulevu by subtracting ∆T.

The actual time of ﬂight from Los Angeles to Namulevu is then the diﬀerence between when the

plane lands (LA times) and when the plane takes oﬀ (LA time):

T= (18:50 −∆T)−(12:50)

= 6:00 −∆T,

where we have written times in 24 hour format to avoid the AM/PM issue. The return ﬂight time

can be found from

T= (18:50) −(1:50 −∆T)

= 17:00 + ∆T,

where we have again changed to LA time for the purpose of the calculation.

(b) Now we just need to solve the two equations and two unknowns.

17:00 + ∆T= 6:00 −∆T

2∆T= 6:00 −17:00

∆T=−5:30.

Since this is a negative number, Namulevu is located west of Los Angeles.

(a) T= 6:00 −∆T= 11 : 30, or eleven and a half hours.

We’ll draw a circle around Los Angeles with a radius of 5980 mi, and then we look for where it

intersects with longitudes that would belong to a time zone ∆Taway from Los Angeles. Since the

Earth rotates once every 24 hours and there are 360 longitude degrees, then each hour corresponds

to 15 longitude degrees, and then Namulevu must be located approximately 15◦×5.5 = 83◦west of

Los Angeles, or at about longitude 160 east. The location on the globe is then latitude 5◦, in the

vicinity of Vanuatu.

When this exercise was originally typeset the times for the outbound and the inbound ﬂights were

inadvertently switched. I suppose that we could blame this on the airlines; nonetheless, when the answers

were prepared for the back of the book the reversed numbers put Namulevu east of Los Angeles. That would

put it in either the North Atlantic or Brazil.

E2-22 There is a three hour time zone diﬀerence. So the ﬂight is seven hours long, but it takes

3 hr 51 min for the sun to travel same distance. Look for when the sunset distance has caught up

with plane:

dsunset =dplane,

vsunset(t−1:35) = vplanet,

(t−1:35)/3:51 = t/7:00,

so t= 3:31 into ﬂight.

E2-23 The distance is

d=vt = (112 km/hr)(1 s)/(3600 s/hr) = 31 m.

E2-24 The time taken for the ball to reach the plate is

t=d

v=(18.4 m)

(160 km/hr)(3600 s/hr)/(1000 m/km) = 0.414 s.

E2-25 Speed is distance traveled divided by time taken; this is equivalent to the inverse of the

slope of the line in Fig. 2-32. The line appears to pass through the origin and through the point

(1600 km, 80 ×106y), so the speed is v= 1600 km/80 ×106y= 2 ×10−5km/y. Converting,

v= 2 ×10−5km/y 1000 m

1 km 100 cm

1 m = 2 cm/y

E2-26 (a) For Maurice Greene vav = (100 m)/(9.81 m) = 10.2 m/s. For Khalid Khannouchi,

vav =(26.219 mi)

(2.0950 hr) 1609 m

1 mi  1hr

3600 s= 5.594 m/s.

(b) If Maurice Greene ran the marathon with an average speed equal to his average sprint speed

then it would take him

t=(26.219 mi)

10.2 m/s1609 m

1 mi  1hr

3600 s= 1.149 hr,

or 1 hour, 9 minutes.

E2-27 The time saved is the diﬀerence,

∆t=(700 km)

(88.5 km/hr) −(700 km)

(104.6 km/hr) = 1.22 hr,

which is about 1 hour 13 minutes.

E2-28 The ground elevation will increase by 35 m in a horizontal distance of

x= (35.0 m)/tan(4.3◦) = 465 m.

The plane will cover that distance in

t=(0.465 km)

(1300 km/hr) 3600 s

1hr = 1.3 s.

E2-29 Let v1= 40 km/hr be the speed up the hill, t1be the time taken, and d1be the distance

traveled in that time. We similarly deﬁne v2= 60 km/hr for the down hill trip, as well as t2and d2.

Note that d2=d1.

v1=d1/t1and v2=d2/t2.vav =d/t, where dtotal distance and tis the total time. The total

distance is d1+d2= 2d1. The total time tis just the sum of t1and t2, so

vav =d

=2d1

t1+t2

=2d1

d1/v1+d2/v2

1/v1+ 1/v2

Take the reciprocal of both sides to get a simpler looking expression

vav

Then the average speed is 48 km/hr.

E2-30 (a) Average speed is total distance divided by total time. Then

vav =(240 ft) + (240 ft)

(240 ft)/(4.0 ft/s) + (240 ft)/(10 ft/s) = 5.7 ft/s.

(b) Same approach, but diﬀerent information given, so

vav =(60 s)(4.0 ft/s) + (60 s)(10 ft/s)

(60 s) + (60 s) = 7.0 ft/s.

E2-31 The distance traveled is the total area under the curve. The “curve” has four regions: (I)

a triangle from 0 to 2 s; (II) a rectangle from 2 to 10 s; (III) a trapezoid from 10 to 12 s; and (IV)

a rectangle from 12 to 16 s.

The area underneath the curve is the sum of the areas of the four regions.

d=1

2(2 s)(8 m/s) + (8.0 s)(8 m/s) + 1

2(2 s)(8 m/s + 4 m/s) + (4.0 s)(4 m/s) = 100 m.

E2-32 The acceleration is the slope of a velocity-time curve,

a=(8 m/s) −(4 m/s)

(10 s) −(12 s) =−2 m/s2.

E2-33 The initial velocity is ~

vi= (18 m/s)ˆ

i, the ﬁnal velocity is ~

vf= (−30 m/s)ˆ

i. The average

acceleration is then

aav =∆~

∆t=~

vf−~

∆t=(−30 m/s)ˆ

i−(18 m/s)ˆ

2.4 s ,

which gives ~

aav = (−20.0 m/s2)ˆ

E2-34 Consider the ﬁgure below.

12345

-5

-10

E2-35 (a) Up to A vx>0 and is constant. From Ato B vxis decreasing, but still positive. From

Bto C vx= 0. From Cto D vx<0, but |vx|is decreasing.

(b) No. Constant acceleration would appear as (part of) a parabola; but it would be challenging

to distinguish between a parabola and an almost parabola.

E2-36 (a) Up to A vx>0 and is decreasing. From Ato B vx= 0. From Bto C vx>0 and is

increasing. From Cto D vx>0 and is constant.

(b) No. Constant acceleration would appear as (part of) a parabola; but it would be challenging

to distinguish between a parabola and an almost parabola.

E2-37 Consider the ﬁgure below.

E2-38 Consider the ﬁgure below.

−4

0123456t(s)

−4

0123456t(s)

x(cm)

v(cm/s)

The acceleration

is a constant

2 cm/s/s during

the entire time

interval.

E2-39 (a) Amust have units of m/s2.Bmust have units of m/s3.

(b) The maximum positive xposition occurs when vx= 0, so

vx=dx

dt = 2At −3Bt2

implies vx= 0 when either t= 0 or t= 2A/3B= 2(3.0 m/s2)/3(1.0 m/s3) = 2.0 s.

x= (3.0 m/s2)(2.0 s)2−(1.0 m/s3)(2.0 s)3= 4.0 m.

Then the particle moves back to a ﬁnal position of

x= (3.0 m/s2)(4.0 s)2−(1.0 m/s3)(4.0 s)3=−16.0 m.

The total path followed was 4.0 m + 4.0 m + 16.0 m = 24.0 m.

(d) The displacement is −16.0 m as was found in part (c).

(e) The velocity is vx= (6.0 m/s2)t−(3.0 m/s3)t2. When t= 0, vx= 0.0 m/s. When t= 1.0 s,

vx= 3.0 m/s. When t= 2.0 s, vx= 0.0 m/s. When t= 3.0 s, vx=−9.0 m/s. When t= 4.0 s,

vx=−24.0 m/s.

(f) The acceleration is the time derivative of the velocity,

ax=dvx

dt = (6.0 m/s2)−(6.0 m/s3)t.

When t= 0 s, ax= 6.0 m/s2. When t= 1.0 s, ax= 0.0 m/s2. When t= 2.0 s, ax=−6.0 m/s2.

When t= 3.0 s, ax=−12.0 m/s2. When t= 4.0 s, ax=−18.0 m/s2.

(g) The distance traveled was found in part (a) to be −20 m The average speed during the time

interval is then vx,av = (−20 m)/(2.0 s) = −10 m/s.

E2-40 v0x= 0, vx= 360 km/hr = 100 m/s. Assuming constant acceleration the average velocity

will be

vx,av =1

2(100 m/s + 0) = 50 m/s.

The time to travel the distance of the runway at this average velocity is

t= (1800 m)/(50 m/s) = 36 s.

The acceleration is

ax= 2x/t2= 2(1800 m)/(36.0 s)2= 2.78 m/s2.

E2-41 (a) Apply Eq. 2-26,

vx=v0x+axt,

(3.0×107m/s) = (0) + (9.8 m/s2)t,

3.1×106s = t.

(b) Apply Eq. 2-28 using an initial position of x0= 0,

x=x0+v0x+1

2axt2,

x= (0) + (0) + 1

2(9.8 m/s2)(3.1×106s)2,

x= 4.7×1013 m.

E2-42 v0x= 0 and vx= 27.8 m/s. Then

t= (vx−v0x)/a = ((27.8 m/s) −(0)) /(50 m/s2) = 0.56 s.

I want that car.

E2-43 The muon will travel for tseconds before it comes to a rest, where tis given by

t= (vx−v0x)/a =(0) −(5.20 ×106m/s)/(−1.30 ×1014m/s2) = 4 ×10−8s.

The distance traveled will be

x=1

2axt2+v0xt=1

2(−1.30 ×1014m/s2)(4 ×10−8s)2+ (5.20 ×106m/s)(4 ×10−8s) = 0.104 m.

E2-44 The average velocity of the electron was

vx,av =1

2(1.5×105m/s + 5.8×106m/s) = 3.0×106m/s.

The time to travel the distance of the runway at this average velocity is

t= (0.012 m)/(3.0×106m/s) = 4.0×10−9s.

The acceleration is

ax= (vx−v0x)/t = ((5.8×106m/s) −(1.5×105m/s))/(4.0×10−9s) = 1.4×1015m/s2.

E2-45 It will be easier to solve the problem if we change the units for the initial velocity,

v0x= 1020km

hr 1000 m

km  hr

3600 s= 283 m

and then applying Eq. 2-26,

vx=v0x+axt,

(0) = (283 m/s) + ax(1.4 s),

−202 m/s2=ax.

The problem asks for this in terms of g, so

−202 m/s2 g

9.8 m/s2!= 21g.

E2-46 Change miles to feet and hours to seconds. Then vx= 81 ft/s and v0x= 125 ft/s. The

time is then

t= ((81 ft/s) −(125 ft/s)) /(−17 ft/s2) = 2.6 s.

E2-47 (a) The time to stop is

t= ((0 m/s) −(24.6 m/s)) /(−4.92 m/s2) = 5.00 s.

(b) The distance traveled is

x=1

2axt2+v0xt=1

2(−4.92 m/s2)(5.00 s)2+ (24.6 m/s)(5.00 s) = 62 m.

E2-48 Answer part (b) ﬁrst. The average velocity of the arrow while decelerating is

vy,av =1

2((0) + (260 ft/s)) = 130 ft/s.

The time for the arrow to travel 9 inches (0.75 feet) is

t= (0.75 ft)/(130 ft/s) = 5.8×10−3s.

(a) The acceleration of the arrow is then

ay= (vy−v0y)/t = ((0) −(260 ft/s))/(5.8×10−3s) = −4.5×104ft/s2.

E2-49 The problem will be somewhat easier if the units are consistent, so we’ll write the maxi-

mum speed as

1000 ft

min min

60 s= 16.7ft

(a) We can ﬁnd the time required for the acceleration from Eq. 2-26,

vx=v0x+axt,

(16.7 ft/s) = (0) + (4.00 ft/s2)t,

4.18 s = t.

And from this and Eq 2-28 we can ﬁnd the distance

x=x0+v0x+1

2axt2,

x= (0) + (0) + 1

2(4.00 ft/s2)(4.18 s)2,

x= 34.9 ft.

(b) The motion of the elevator is divided into three parts: acceleration from rest, constant speed

motion, and deceleration to a stop. The total distance is given at 624 ft and in part (a) we found

the distance covered during acceleration was 34.9 ft. By symmetry, the distance traveled during

deceleration should also be 34.9 ft. The distance traveled at constant speed is then (624 −34.9−

34.9) ft = 554 ft. The time required for the constant speed portion of the trip is found from Eq.

2-22, rewritten as

∆t=∆x

v=554 ft

16.7 ft/s = 33.2 s.

The total time for the trip is the sum of times for the three parts: accelerating (4.18 s), constant

speed (33.2 s), and decelerating (4.18 s). The total is 41.6 seconds.

E2-50 (a) The deceleration is found from

ax=2

t2(x−v0t) = 2

(4.0 s)2((34 m) −(16 m/s)(4.0 s)) = −3.75 m/s2.

(b) The impact speed is

vx=v0x+axt= (16 m/s) + (−3.75 m/s2)(4.0 s) = 1.0 m/s.

E2-51 Assuming the drops fall from rest, the time to fall is

t=s2y

=s2(−1700 m)

(−9.8 m/s2)= 19 s.

The velocity of the falling drops would be

vy=ayt= (−9.8 m/s2)(19 s) = 190 m/s,

or about 2/3 the speed of sound.

E2-52 Solve the problem out of order.

(b) The time to fall is

t=s2y

=s2(−120 m)

(−9.8 m/s2)= 4.9 s.

(a) The speed at which the elevator hits the ground is

vy=ayt= (−9.8 m/s2)(4.9 s) = 48 m/s.

(d) The time to fall half-way is

t=s2y

=s2(−60 m)

(−9.8 m/s2)= 3.5 s.

vy=ayt= (−9.8 m/s2)(3.5 s) = 34 m/s.

E2-53 The initial velocity of the “dropped” wrench would be zero. I choose vertical to be along

the yaxis with up as positive, which is the convention of Eq. 2-29 and Eq. 2-30. It turns out that

it is much easier to solve part (b) before solving part (a).

(b) We solve Eq. 2-29 for the time of the fall.

vy=v0y−gt,

(−24.0 m/s) = (0) −(9.8 m/s2)t,

2.45 s = t.

(a) Now we can use Eq. 2-30 to ﬁnd the height from which the wrench fell.

y=y0+v0yt−1

2gt2,

(0) = y0+ (0)(2.45 s) −1

2(9.8 m/s2)(2.45 s)2,

0 = y0−29.4 m

We have set y= 0 to correspond to the ﬁnal position of the wrench: on the ground. This results in

an initial position of y0= 29.4 m; it is positive because the wrench was dropped from a point above

where it landed.

E2-54 (a) It is easier to solve the problem from the point of view of an object which falls from the

highest point. The time to fall from the highest point is

t=s2y

=s2(−53.7 m)

(−9.81 m/s2)= 3.31 s.

The speed at which the object hits the ground is

vy=ayt= (−9.81 m/s2)(3.31 s) = −32.5 m/s.

But the motion is symmetric, so the object must have been launched up with a velocity of vy=

32.5 m/s.

(b) Double the previous answer; the time of ﬂight is 6.62 s.

E2-55 (a) The time to fall the ﬁrst 50 meters is

t=s2y

=s2(−50 m)

(−9.8 m/s2)= 3.2 s.

(b) The total time to fall 100 meters is

t=s2y

=s2(−100 m)

(−9.8 m/s2)= 4.5 s.

The time to fall through the second 50 meters is the diﬀerence, 1.3 s.

E2-56 The rock returns to the ground with an equal, but opposite, velocity. The acceleration is

then

ay= ((−14.6 m/s) −(14.6 m/s))/(7.72 s) = 3.78 m/s2.

That would put them on Mercury.

E2-57 (a) Solve Eq. 2-30 for the initial velocity. Let the distances be measured from the ground

so that y0= 0.

y=y0+v0yt−1

2gt2,

(36.8 m) = (0) + v0y(2.25 s) −1

2(9.8 m/s2)(2.25 s)2,

36.8 m = v0y(2.25 s) −24.8 m,

27.4 m/s = v0y.

(b) Solve Eq. 2-29 for the velocity, using the result from part (a).

vy=v0y−gt,

vy= (27.4 m/s) −(9.8 m/s2)(2.25 s),

vy= 5.4 m/s.

long it takes to get there. So we ﬁrst solve Eq. 2-29, because we do know the velocity at the highest

point (vy= 0).

vy=v0y−gt,

(0) = (27.4 m/s) −(9.8 m/s2)t,

2.8 s = t.

And then we ﬁnd the height to which the object rises,

y=y0+v0yt−1

2gt2,

y= (0) + (27.4 m/s)(2.8 s) −1

2(9.8 m/s2)(2.8 s)2,

y= 38.3m.

This is the height as measured from the ground; so the ball rises 38.3−36.8 = 1.5 m above the point

speciﬁed in the problem.

E2-58 The time it takes for the ball to fall 2.2 m is

t=s2y

=s2(−2.2 m)

(−9.8 m/s2)= 0.67 s.

The ball hits the ground with a velocity of

vy=ayt= (−9.8 m/s2)(0.67 s) = −6.6 m/s.

The ball then bounces up to a height of 1.9 m. It is easier to solve the falling part of the motion,

and then apply symmetry. The time is would take to fall is

t=s2y

=s2(−1.9 m)

(−9.8 m/s2)= 0.62 s.

The ball hits the ground with a velocity of

vy=ayt= (−9.8 m/s2)(0.62 s) = −6.1 m/s.

But we are interested in when the ball moves up, so vy= 6.1 m/s.

The acceleration while in contact with the ground is

ay= ((6.1 m/s) −(−6.6 m/s))/(0.096 s) = 130 m/s2.

E2-59 The position as a function of time for the ﬁrst object is

y1=−1

2gt2,

The position as a function of time for the second object is

y2=−1

2g(t−1 s)2

The diﬀerence,

∆y=y2−y1=frac12g((2 s)t−1) ,

is the set equal to 10 m, so t= 1.52 s.

E2-60 Answer part (b) ﬁrst.

(b) Use the quadratic equation to solve

(−81.3 m) = 1

2(−9.81 m/s2)t2+ (12.4 m/s)t

for time. Get t=−3.0 s and t= 5.53 s. Keep the positive answer.

(a) Now ﬁnd ﬁnal velocity from

vy= (−9.8 m/s2)(5.53 s) + (12.4 m/s) = −41.8 m/s.

E2-61 The total time the pot is visible is 0.54 s; the pot is visible for 0.27 s on the way down.

We’ll deﬁne the initial position as the highest point and make our measurements from there. Then

y0= 0 and v0y= 0. Deﬁne t1to be the time at which the falling pot passes the top of the window

y1, then t2=t1+ 0.27 s is the time the pot passes the bottom of the window y2=y1−1.1 m. We

have two equations we can write, both based on Eq. 2-30,

y1=y0+v0yt1−1

2gt2

y1= (0) + (0)t1−1

2gt2

and

y2=y0+v0yt2−1

2gt2

y1−1.1 m = (0) + (0)t2−1

2g(t1+ 0.27 s)2,

Isolate y1in this last equation and then set the two expressions equal to each other so that we can

solve for t1,

−1

2gt2

1= 1.1 m −1

2g(t1+ 0.27 s)2,

−1

2gt2

1= 1.1 m −1

2g(t2

1+ [0.54 s]t1+ 0.073 s2),

0=1.1 m −1

2g([0.54 s]t1+ 0.073 s2).

This last line can be directly solved to yield t1= 0.28 s as the time when the falling pot passes the

top of the window. Use this value in the ﬁrst equation above and we can ﬁnd y1=−1

2(9.8 m/s2)(0.28

s)2=−0.38 m. The negative sign is because the top of the window is beneath the highest point, so

the pot must have risen to 0.38 m above the top of the window.

P2-1 (a) The net shift is p(22 m)2+ (17 m)2) = 28 m.

(b) The vertical displacement is (17 m) sin(52◦) = 13 m.

P2-2 Wheel “rolls” through half of a turn, or πr = 1.41 m. The vertical displacement is 2r=

0.90 m. The net displacement is

p(1.41 m)2+ (0.90 m)2= 1.67 m0.

The angle is

θ= tan−1(0.90 m)/(1.41 m) = 33◦.

P2-3 We align the coordinate system so that the origin corresponds to the starting position of

the ﬂy and that all positions inside the room are given by positive coordinates.

(a) The displacement vector can just be written,

∆~

r= (10 ft)ˆ

i+ (12 ft)ˆ

j+ (14 ft)ˆ

(b) The magnitude of the displacement vector is |∆~

r|=√102+ 122+ 142ft= 21 ft.

of the path taken by the ﬂy must be greater than or equal to 21 ft.

(d) If the ﬂy walks it will need to cross two faces. The shortest path will be the diagonal across

these two faces. If the lengths of sides of the room are l1,l2, and l3, then the diagonal length across

two faces will be given by

q(l1+l2)2+l2

where we want to choose the lifrom the set of 10 ft, 12 ft, and 14 ft that will minimize the length.

The minimum distance is when l1= 10 ft, l2= 12 ft, and l3= 14. Then the minimal distance the

ﬂy would walk is 26 ft.

P2-4 Choose vector ~

ato lie on the xaxis. Then ~

a=aˆ

iand ~

b=bxˆ

i+byˆ

jwhere bx=bcos θand

by=bsin θ. The sum then has components

rx=a+bcos θand ry=bsin θ.

Then

r2= (a+bcos θ)2+ (bsin θ)2,

=a2+ 2ab cos θ+b2.

P2-5 (a) Average speed is total distance divided by total time. Then

vav =(35.0 mi/hr)(t/2) + (55.0 mi/hr)(t/2)

(t/2) + (t/2) = 45.0 mi/hr.

(b) Average speed is total distance divided by total time. Then

vav =(d/2) + (d/2)

(d/2)/(35.0 mi/hr) + (d/2)/(55.0 mi/hr) = 42.8 mi/hr.

vav =d+d

(d)/(45.0 mi/hr) + (d)/(42.8 mi/hr) = 43.9 mi/hr

P2-6 (a) We’ll do just one together. How about t= 2.0 s?

x= (3.0 m/s)(2.0 s) + (−4.0 m/s2)(2.0 s)2+ (1.0 m/s3)(2.0 s)3=−2.0 m.

The rest of the values are, starting from t= 0, x= 0.0 m, 0.0 m, -2.0 m, 0.0 m, and 12.0 m.

(b) Always ﬁnal minus initial. The answers are xf−xi=−2.0 m −0.0 m = −2.0 m and xf−xi=

12.0 m −0.0 m = 12.0 m.

vav =(12.0 m) −(−2.0 m)

(4.0 s) −(2.0 s) = 7.0 m/s,

and

vav =(0.0 m) −(0.0 m)

(3.0 s) −(0.0 s) = 0.0 m/s.

P2-7 (a) Assume the bird has no size, the trains have some separation, and the bird is just

leaving one of the trains. The bird will be able to ﬂy from one train to the other before the two

trains collide, regardless of how close together the trains are. After doing so, the bird is now on the

other train, the trains are still separated, so once again the bird can ﬂy between the trains before

they collide. This process can be repeated every time the bird touches one of the trains, so the bird

will make an inﬁnite number of trips between the trains.

(b) The trains collide in the middle; therefore the trains collide after (51 km)/(34 km/hr) = 1.5

hr. The bird was ﬂying with constant speed this entire time, so the distance ﬂown by the bird is (58

km/hr)(1.5 hr) = 87 km.

P2-8 (a) Start with a perfect square:

(v1−v2)2>0,

1+v2

2>2v1v2,

(v2

1+v2

2)t1t2>2v1v2t1t2,

1+d2

2+ (v2

1+v2

2)t1t2> d2

1+d2

2+ 2v1v2t1t2,

(v2

1t1+v2

2t2)(t1+t2)>(d1+d2)2,

1t1+v2

2t2

d1+d2

>d1+d2

t1+t2

v1d1+v2d2

d1+d2

>v1t1+v2t2

t1+t2

Actually, it only works if d1+d2>0!

(b) If v1=v2.

P2-9 (a) The average velocity during the time interval is vav = ∆x/∆t, or

vav =(A+B(3 s)3)−(A+B(2 s)3)

(3 s) −(2 s) = (1.50 cm/s3)(19 s3)/(1 s) = 28.5 cm/s.

(b) v=dx/dt = 3Bt2= 3(1.50 cm/s3)(2 s)2= 18 cm/s.

(d) v=dx/dt = 3Bt2= 3(1.50 cm/s3)(2.5 s)2= 28.1 cm/s.

(e) The midway position is (xf+xi)/2, or

xmid =A+B[(3 s)3+ (2 s)3)]/2 = A+ (17.5 s3)B.

This occurs when t=3

p(17.5 s3). The instantaneous velocity at this point is

v=dx/dt = 3Bt2= 3(1.50 cm/s3)( 3

p(17.5 s3))2= 30.3 cm/s.

P2-10 Consider the ﬁgure below.

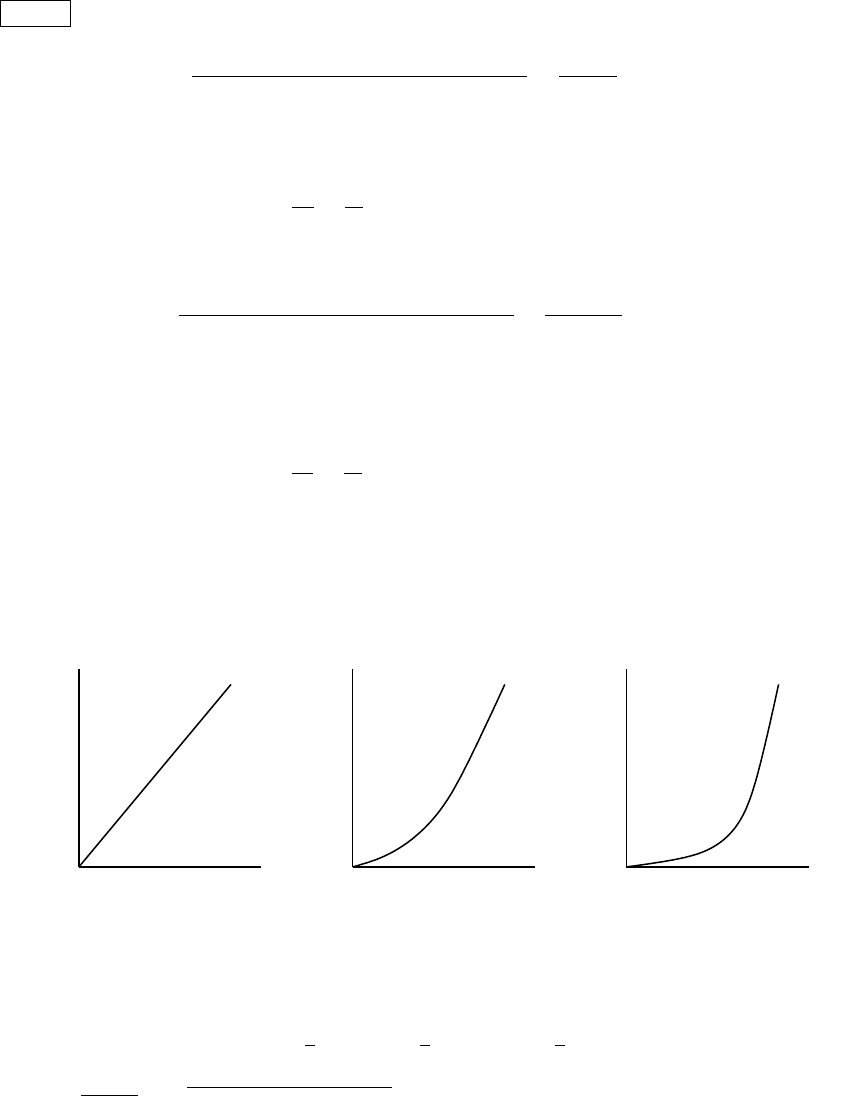
P2-11 (a) The average velocity is displacement divided by change in time,

vav =(2.0 m/s3)(2.0 s)3−(2.0 m/s3)(1.0 s)3

(2.0 s) −(1.0 s) =14.0 m

1.0 s = 14.0 m/s.

The average acceleration is the change in velocity. So we need an expression for the velocity, which

is the time derivative of the position,

v=dx

dt =d

dt(2.0 m/s3)t3= (6.0 m/s3)t2.

From this we ﬁnd average acceleration

aav =(6.0 m/s3)(2.0 s)2−(6.0 m/s3)(1.0 s)2

(2.0 s) −(1.0 s) =18.0 m/s

1.0 s = 18.0 m/s2.

(b) The instantaneous velocities can be found directly from v= (6.0 m/s2)t2, so v(2.0 s) = 24.0

m/s and v(1.0 s) = 6.0 m/s. We can get an expression for the instantaneous acceleration by taking

the time derivative of the velocity

a=dv

dt =d

dt(6.0 m/s3)t2= (12.0 m/s3)t.

Then the instantaneous accelerations are a(2.0 s) = 24.0 m/s2and a(1.0 s) = 12.0 m/s2

the instantaneous values at the endpoints of the time interval. Indeed, that is the case.

P2-12 Consider the ﬁgure below.

0246810

t(s)

0246810

t(s)

0246810

t(s)

a(m/s^2)

v(m/s) x(m)

P2-13 Start with vf=vi+at, but vf= 0, so vi=−at, then

x=1

2at2+vit=1

2at2−at2=−1

2at2,

so t=p−2x/a =q−2(19.2 ft)/(−32 ft/s2) = 1.10 s. Then vi=−(−32 ft/s2)(1.10 s) = 35.2 ft/s.

Converting,

35.2 ft/s(1/5280 mi/ft)(3600 s/h) = 24 mi/h.

P2-14 (b) The average speed during while traveling the 160 m is

vav = (33.0 m/s + 54.0 m/s)/2 = 43.5 m/s.

The time to travel the 160 m is t= (160 m)/(43.5 m/s) = 3.68 s.

(a) The acceleration is

a=2x

t2−2vi

t=2(160 m)

(3.68 s)2−2(33.0 m/s)

(3.68 s) = 5.69 m/s2.

t=v/a = (33.0 m/s)/(5.69 m/s2) = 5.80 s.

(d) The distance moved from start is

d=1

2at2=1

2(5.69 m/s2)(5.80 s)2= 95.7 m.

P2-15 (a) The distance traveled during the reaction time happens at constant speed; treac =d/v =

(15 m)/(20 m/s) = 0.75 s.

(b) The braking distance is proportional to the speed squared (look at the numbers!) and in

this case is dbrake =v2/(20 m/s2). Then dbrake = (25 m/s)2/(20 m/s2) = 31.25 m. The reaction time

distance is dreac = (25 m/s)(0.75 s) = 18.75 m. The stopping distance is then 50 m.

P2-16 (a) For the car xc=act2/2. For the truck xt=vtt. Set both xito the same value, and

then substitute the time from the truck expression:

x=act2/2 = ac(x/vt)2/2,

x= 2vt2/ac= 2(9.5 m/s)2/(2.2 m/s) = 82 m.

(b) The speed of the car will be given by vc=act, or

vc=act=acx/vt= (2.2 m/s)(82 m)/(9.5 m/s) = 19 m/s.

P2-17 The runner covered a distance d1in a time interval t1during the acceleration phase and

a distance d2in a time interval t2during the constant speed phase. Since the runner started from

rest we know that the constant speed is given by v=at1, where ais the runner’s acceleration.

The distance covered during the acceleration phase is given by

d1=1

2at2

The distance covered during the constant speed phase can also be found from

d2=vt2=at1t2.

We want to use these two expressions, along with d1+d2= 100 m and t2= (12.2 s) −t1, to get

100 m = d1+d2=1

2at2

1+at1(12.2 s −t1),

=−1

2at2

1+a(12.2 s)t1,

=−(1.40 m/s2)t2

1+ (34.2 m/s)t1.

This last expression is quadratic in t1, and is solved to give t1= 3.40 s or t1= 21.0 s. Since the race

only lasted 12.2 s we can ignore the second answer.

(b) The distance traveled during the acceleration phase is then

d1=1

2at2

1= (1.40 m/s2)(3.40 s)2= 16.2 m.

P2-18 (a) The ball will return to the ground with the same speed it was launched. Then the total

time of ﬂight is given by

t= (vf−vi)/g = (−25 m/s−25 m/s)/(9.8 m/s2) = 5.1 s.

(b) For small quantities we can think in terms of derivatives, so

δt = (δvf−δvi)/g,

or τ= 2/g.

P2-19 Use y=−gt2/2, but only keep the absolute value. Then y50 = (9.8 m/s2)(0.05 s)2/2 =

1.2 cm; y100 = (9.8 m/s2)(0.10 s)2/2 = 4.9 cm; y150 = (9.8 m/s2)(0.15 s)2/2 = 11 cm; y200 =

(9.8 m/s2)(0.20 s)2/2 = 20 cm; y250 = (9.8 m/s2)(0.25 s)2/2 = 31 cm.

P2-20 The truck will move 12 m in (12 m)/(55 km/h) = 0.785 s. The apple will fall y=−gt2/2 =

−(9.81 m/s2)(0.785 s)2/2 = −3.02 m.

P2-21 The rocket travels a distance d1=1

2at2

1=1

2(20 m/s2)(60 s)2= 36,000 m during the accel-

eration phase; the rocket velocity at the end of the acceleration phase is v=at = (20 m/s2)(60 s) =

1200 m/s. The second half of the trajectory can be found from Eqs. 2-29 and 2-30, with y0= 36,000

m and v0y= 1200 m/s.

(a) The highest point of the trajectory occurs when vy= 0, so

vy=v0y−gt,

(0) = (1200 m/s) −(9.8 m/s2)t,

122 s = t.

This time is used to ﬁnd the height to which the rocket rises,

y=y0+v0yt−1

2gt2,

= (36000 m) + (1200 m/s)(122s) −1

2(9.8 m/s2)(122 s)2= 110000 m.

(b) The easiest way to ﬁnd the total time of ﬂight is to solve Eq. 2-30 for the time when the

rocket has returned to the ground. Then

y=y0+v0yt−1

2gt2,

(0) = (36000 m) + (1200 m/s)t−1

2(9.8 m/s2)t2.

This quadratic expression has two solutions for t; one is negative so we don’t need to worry about

it, the other is t= 270 s. This is the free-fall part of the problem, to ﬁnd the total time we need to

add on the 60 seconds of accelerated motion. The total time is then 330 seconds.

P2-22 (a) The time required for the player to “fall” from the highest point a distance of y= 15 cm

is p2y/g; the total time spent in the top 15 cm is twice this, or 2p2y/g = 2p2(0.15 m)/(9.81 m/s) =

0.350 s.

(b) The time required for the player to “fall” from the highest point a distance of 76 cm is

p2(0.76 m)/(9.81 m/s) = 0.394 s, the time required for the player to fall from the highest point a

distance of (76 −15 = 61) cm is p2(0.61 m)/g = 0.353 s. The time required to fall the bottom 15

cm is the diﬀerence, or 0.041 s. The time spent in the bottom 15 cm is twice this, or 0.081 s.

P2-23 (a) The average speed between Aand Bis vav = (v+v/2)/2=3v/4. We can also write

vav = (3.0 m)/∆t= 3v/4. Finally, v/2 = v−g∆t. Rearranging, v/2 = g∆t. Combining all of the

above,

2=g4(3.0 m)

3vor v2= (8.0 m)g.

Then v=p(8.0 m)(9.8 m/s2) = 8.85 m/s.

(b) The time to the highest point above Bis v/2 = gt, so the distance above Bis

y=−g

2t2+v

2t=−g

2v

2g2

2v

2g=v2

8g.

Then y= (8.85 m/s)2/(8(9.8 m/s2)) = 1.00 m.

P2-24 (a) The time in free fall is t=p−2y/g =p−2(−145 m)/(9.81 m/s2) = 5.44 s.

(b) The speed at the bottom is v=−gt =−(9.81 m/s2)(5.44 s) = −53.4 m/s.

0.218 s. The distance through which deceleration occurred is

y=25g

2t2+vt = (123 m/s2)(0.218 s)2+ (−53.4 m/s)(0.218 s) = −5.80 m.

P2-25 Find the time she fell from Eq. 2-30,

(0 ft) = (144 ft) + (0)t−1

2(32 ft/s2)t2,

which is a simple quadratic with solutions t=±3.0 s. Only the positive solution is of interest. Use

this time in Eq. 2-29 to ﬁnd her speed when she hit the ventilator box,

vy= (0) −(32 ft/s2)(3.0 s) = −96 ft/s.

This becomes the initial velocity for the deceleration motion, so her average speed during deceleration

is given by Eq. 2-27,

vav,y =1

2(vy+v0y) = 1

2((0) + (−96 ft/s)) = −48 ft/s.

This average speed, used with the distance of 18 in (1.5 ft), can be used to ﬁnd the time of deceleration

vav,y = ∆y/∆t,

and putting numbers into the expression gives ∆t= 0.031 s. We actually used ∆y=−1.5 ft, where

the negative sign indicated that she was still moving downward. Finally, we use this in Eq. 2-26 to

ﬁnd the acceleration,

(0) = (−96 ft/s) + a(0.031 s),

which gives a= +3100 ft/s2. In terms of gthis is a= 97g, which can be found by multiplying

through by 1 = g/(32 ft/s2).

P2-26 Let the speed of the disk when it comes into contact with the ground be v1; then the

average speed during the deceleration process is v1/2; so the time taken for the deceleration process

is t1= 2d/v1, where d=−2 mm. But dis also give by d=at2

1/2 + v1t1, so

d=100g

22d

v12

+v12d

v1= 200gd2

+ 2d,

or v2

1=−200gd. The negative signs are necessary!.

The disk was dropped from a height h=−yand it ﬁrst came into contact with the ground when

it had a speed of v1. Then the average speed is v1/2, and we can repeat most of the above (except

a=−ginstead of 100g), and then the time to fall is t2= 2y/v1,

y=g

22y

v12

+v12y

v1= 2gy2

+ 2y,

or v2

1=−2gy. The negative signs are necessary!.

Equating, y= 100d= 100(−2 mm) = −0.2 m, so h= 0.2 m. Note that although 100g’s sounds

like plenty, you still shouldn’t be dropping your hard disk drive!

P2-27 Measure from the feet! Jim is 2.8 cm tall in the photo, so 1 cm on the photo is 60.7

cm in real-life. Then Jim has fallen a distance y1=−3.04 m while Clare has fallen a distance

y2=−5.77 m. Clare jumped ﬁrst, and the time she has been falling is t2; Jim jumped seconds, the

time he has been falling is t1=t2−∆t. Then y2=−gt2

2/2 and y1=−gt2

1/2, or t2=p−2y2/g =

p−2(−5.77 m)/(9.81 m/s2)=1.08 s and t1=p−2y1/g =p−2(3.04 m)/(9.81 m/s2)=0.79 s. So

Jim waited 0.29 s.

P2-28 (a) Assuming she starts from rest and has a speed of v1when she opens her chute, then

her average speed while falling freely is v1/2, and the time taken to fall y1=−52.0 m is t1= 2y1/v1.

Her speed v1is given by v1=−gt1, or v2

1=−2gy1. Then v1=−p−2(9.81 m/s2)(−52.0 m) =

−31.9 m/s. We must use the negative answer, because she fall down! The time in the air is then

t1=p−2y1/g =p−2(52.0 m)/(9.81 m/s2) = 3.26 s.

Her ﬁnal speed is v2=−2.90 m/s, so the time for the deceleration is t2= (v2−v1)/a, where

a= 2.10 m/s2. Then t2= (−2.90 m/s− −31.9 m/s)/(2.10 m/s2) = 13.8 s.

Finally, the total time of ﬂight is t=t1+t2= 3.26 s + 13.8 s = 17.1 s.

(b) The distance fallen during the deceleration phase is

y2=−g

2t2

2+v1t2=−(2.10 m/s2)

2(13.8 s)2+ (−31.9 m/s)(13.8 s) = −240 m.

The total distance fallen is y=y1+y2=−52.0 m −240 m = −292 m. It is negative because she was

falling down.

P2-29 Let the speed of the bearing be v1at the top of the windows and v2at the bottom.

These speeds are related by v2=v1−gt12, where t12 = 0.125 s is the time between when the

bearing is at the top of the window and at the bottom of the window. The average speed is

vav = (v1+v2)/2 = v1−gt12/2. The distance traveled in the time t12 is y12 =−1.20 m, so

y12 =vavt12 =v1t12 −gt2

12/2,

and expression that can be solved for v1to yield

v1=y12 +gt2

12/2

t12

=(−1.20 m) + (9.81 m/s2)(0.125 s)2/2

(0.125 s) =−8.99 m/s.

Now that we know v1we can ﬁnd the height of the building above the top of the window. The time

the object has fallen to get to the top of the window is t1=−v1/g =−(−8.99 m/s)/(9.81 m/s2) =

0.916 m.

The total time for falling is then (0.916 s) + (0.125 s) + (1.0 s) = 2.04 s. Note that we remembered

to divide the last time by two! The total distance from the top of the building to the bottom is then

y=−gt2/2 = −(9.81 m/s2)(2.04 s)2/2 = 20.4 m.

P2-30 Each ball falls from a highest point through a distance of 2.0 m in

t=p−2(2.0 m)/(9.8 m/s2) = 0.639 s.

The time each ball spends in the air is twice this, or 1.28 s. The frequency of tosses per ball is the

reciprocal, f= 1/T = 0.781 s−1. There are ﬁve ball, so we multiply this by 5, but there are two

hands, so we then divide that by 2. The tosses per hand per second then requires a factor 5/2, and

the tosses per hand per minute is 60 times this, or 117.

P2-31 Assume each hand can toss nobjects per second. Let τbe the amount of time that any

one object is in the air. Then 2nτ is the number of objects that are in the air at any time, where

the “2” comes from the fact that (most?) jugglers have two hands. We’ll estimate n, but τcan be

found from Eq. 2-30 for an object which falls a distance hfrom rest:

0 = h+ (0)t−1

2gt2,

solving, t=p2h/g. But τis twice this, because the object had to go up before it could come down.

So the number of objects that can be juggled is

4np2h/g

We estimate n= 2 tosses/second. So the maximum number of objects one could juggle to a height

hwould be

3.6ph/meters.

P2-32 (a) We need to look up the height of the leaning tower to solve this! If the height is h= 56 m,

then the time taken to fall a distance h=−y1is t1=p−2y1/g =p−2(−56 m)/(9.81 m/s2) = 3.4 s.

The second object, however, has only fallen a a time t2=t1−∆t= 3.3 s, so the distance the second

object falls is y2=−gt2

2/2 = −(9.81 m/s2)(3.3 s)2/2 = 53.4. The diﬀerence is y1−y2= 2.9 m.

(b) If the vertical separation is ∆y= 0.01 m, then we can approach this problem in terms of

diﬀerentials,

δy =at δt,

so δt = (0.01 m)/[((9.81 m/s2)(3.4 s)] = 3×10−4s.

P2-33 Use symmetry, and focus on the path from the highest point downward. Then ∆tU= 2tU,

where tUis the time from the highest point to the upper level. A similar expression exists for

the lower level, but replace Uwith L. The distance from the highest point to the upper level is

yU=−gt2

U/2 = −g(∆tU/2)2/2. The distance from the highest point to the lower level is yL=

−gt2

L/2 = −g(∆tL/2)2/2. Now H=yU−yL=−g∆t2

U/8− −g∆t2

L/8, which can be written as

g=8H

∆t2

L−∆t2

E3-1 The Earth orbits the sun with a speed of 29.8 km/s. The distance to Pluto is 5900×106km.

The time it would take the Earth to reach the orbit of Pluto is

t= (5900×106km)/(29.8 km/s) = 2.0×108s,

or 6.3 years!

E3-2 (a) a=F/m = (3.8 N)/(5.5 kg) = 0.69 m/s2.

(b) t=vf/a = (5.2 m/s)/(0.69 m/s2) = 7.5 s.

E3-3 Assuming constant acceleration we can ﬁnd the average speed during the interval from Eq.

2-27

vav,x =1

2(vx+v0x) = 1

2(5.8×106m/s) + (0)= 2.9×106m/s.

From this we can ﬁnd the time spent accelerating from Eq. 2-22, since ∆x=vav,x∆t. Putting in

the numbers ∆t= 5.17×10−9s. The acceleration is then

ax=∆vx

∆t=(5.8×106m/s) −(0)

(5.17×10−9s) = 1.1×1015m/s2.

The net force on the electron is from Eq. 3-5,

XFx=max= (9.11×10−31kg)(1.1×1015m/s2) = 1.0×10−15 N.

E3-4 The average speed while decelerating is vav = 0.7×107m/s. The time of deceleration is

t=x/vav = (1.0×10−14m)/(0.7×107m/s) = 1.4×10−21 s. The deceleration is a= ∆v/t = (−1.4×

107m/s)/(1.4×10−21 s) = −1.0×1028m/s2. The force is F=ma = (1.67×10−27kg)(1.0×1028m/s2) =

17 N.

E3-5 The net force on the sled is 92 N−90 N= 2 N; subtract because the forces are in opposite

directions. Then

ax=PFx

m=(2 N)

(25 kg) = 8.0×10−2m/s2.

E3-6 53 km/hr is 14.7 m/s. The average speed while decelerating is vav = 7.4 m/s. The time

of deceleration is t=x/vav = (0.65 m)/(7.4 m/s) = 8.8×10−2s. The deceleration is a= ∆v/t =

(−14.7 m/s)/(8.8×10−2s) = −17×102m/s2. The force is F=ma = (39kg)(1.7×102m/s2) = 6600 N.

E3-7 Vertical acceleration is a=F/m = (4.5×10−15N)/(9.11×10−31kg) = 4.9×1015m/s2. The

electron moves horizontally 33 mm in a time t=x/vx= (0.033 m)/(1.2×107m/s) = 2.8×10−9s.

The vertical distance deﬂected is y=at2/2 = (4.9×1015m/s2)(2.8×10−9s)2/2 = 1.9×10−2m.

E3-8 (a) a=F/m = (29 N)/(930 kg) = 3.1×10−2m/s2.

(b) x=at2/2 = (3.1×10−2m/s2)(86400 s)2/2 = 1.2×108m.

E3-9 Write the expression for the motion of the ﬁrst object as PFx=m1a1xand that of the

second object as PFx=m2a2x. In both cases there is only one force, F, on the object, so PFx=F.

We will solve these for the mass as m1=F/a1and m2=F/a2. Since a1> a2we can conclude that

m2> m1

(a) The acceleration of and object with mass m2−m1under the inﬂuence of a single force of

magnitude Fwould be

a=F

m2−m1

F/a2−F/a1

1/(3.30 m/s2)−1/(12.0 m/s2),

which has a numerical value of a= 4.55 m/s2.

(b) Similarly, the acceleration of an object of mass m2+m1under the inﬂuence of a force of

magnitude Fwould be

a=1

1/a2+ 1/a1

1/(3.30 m/s2)+1/(12.0 m/s2),

which is the same as part (a) except for the sign change. Then a= 2.59 m/s2.

E3-10 (a) The required acceleration is a=v/t = 0.1c/t. The required force is F=ma = 0.1mc/t.

Then

F= 0.1(1200×103kg)(3.00×108m/s)/(2.59×105s) = 1.4×108N,

and

F= 0.1(1200×103kg)(3.00×108m/s)/(5.18×106s) = 6.9×106N,

(b) The distance traveled during the acceleration phase is x1=at2

1/2, the time required to

travel the remaining distance is t2=x2/v where x2=d−x1.dis 5 light-months, or d= (3.00×

108m/s)(1.30×107s) = 3.90×1015m. Then

t=t1+t2=t1+d−x1

v=t1+2d−at2

2v=t1+2d−vt1

2v.

If t1is 3 days, then

t= (2.59×105s) + 2(3.90×1015m) −(3.00×107m/s)(2.59×105s)

2(3.00×107m/s) = 1.30×108s=4.12 yr,

if t1is 2 months, then

t= (5.18×106s) + 2(3.90×1015m) −(3.00×107m/s)(5.18×106s)

2(3.00×107m/s) = 1.33×108s=4.20 yr,

E3-11 (a) The net force on the second block is given by

XFx=m2a2x= (3.8 kg)(2.6 m/s2) = 9.9 N.

There is only one (relevant) force on the block, the force of block 1 on block 2.

(b) There is only one (relevant) force on block 1, the force of block 2 on block 1. By Newton’s

third law this force has a magnitude of 9.9 N. Then Newton’s second law gives PFx=−9.9

N= m1a1x= (4.6 kg)a1x. So a1x=−2.2 m/s2at the instant that a2x= 2.6 m/s2.

E3-12 (a) W= (5.00 lb)(4.448 N/lb) = 22.2 N; m=W/g = (22.2 N)/(9.81 m/s2) = 2.26 kg.

(b) W= (240 lb)(4.448 N/lb) = 1070 N; m=W/g = (1070 N)/(9.81 m/s2) = 109 kg.

E3-13 (a) W= (1420.00 lb)(4.448 N/lb) = 6320 N; m=W/g = (6320 N)/(9.81 m/s2) = 644 kg.

(b) m= 412 kg; W=mg = (412 kg)(9.81 m/s2) = 4040 N.

E3-14 (a) W=mg = (75.0 kg)(9.81 m/s2) = 736 N.

(b) W=mg = (75.0 kg)(3.72 m/s2) = 279 N.

(d) The mass is 75.0 kg at all locations.

E3-15 If g= 9.81 m/s2, then m=W/g = (26.0 N)/(9.81 m/s2) = 2.65 kg.

(a) Apply W=mg again, but now g= 4.60 m/s2, so at this point W= (2.65 kg)(4.60 m/s2) =

12.2 N.

(b) If there is no gravitational force, there is no weight, because g= 0. There is still mass,

however, and that mass is still 2.65 kg.

E3-16 Upward force balances weight, so F=W=mg = (12000 kg)(9.81 m/s2) = 1.2×105N.

E3-17 Mass is m=W/g; net force is F=ma, or F=W a/g. Then

F= (3900 lb)(13 ft/s2)/(32 ft/s2) = 1600 lb.

E3-18 a= ∆v/∆t= (450 m/s)/(1.82 s) = 247 m/s2. Net force is F=ma = (523 kg)(247 m/s2) =

1.29×105N.

E3-19 PFx= 2(1.4×105N) = max. Then m= 1.22×105kg and

W=mg = (1.22×105kg)(9.81 m/s2) = 1.20×106N.

E3-20 Do part (b) ﬁrst; there must be a 10 lb force to support the mass. Now do part (a), but

cover up the left hand side of both pictures. If you can’t tell which picture is which, then they must

both be 10 lb!

E3-21 (b) Average speed during deceleration is 40 km/h, or 11 m/s. The time taken to stop the

car is then t=x/vav = (61 m)/(11 m/s) = 5.6 s.

(a) The deceleration is a= ∆v/∆t= (22 m/s)/(5.6 s) = 3.9 m/s2. The braking force is F=

ma =W a/g = (13,000 N)(3.9 m/s2)/(9.81 m/s2) = 5200 N.

(d) The deceleration is same; the time to stop the car is then ∆t= ∆v/a = (11 m/s)/(3.9 m/s2) =

2.8s.

E3-22 Assume acceleration of free fall is 9.81 m/s2at the altitude of the meteor. The net force is

Fnet =ma = (0.25 kg)(9.2m/s2) = 2.30 N. The weight is W=mg = (0.25 kg)(9.81 m/s2) = 2.45 N.

The retarding force is Fnet −W= (2.3 N) −(2.45 N) = −0.15 N.

E3-23 (a) Find the time during the “jump down” phase from Eq. 2-30.

(0 m) = (0.48 m) + (0)t−1

2(9.8 m/s2)t2,

which is a simple quadratic with solutions t=±0.31 s. Use this time in Eq. 2-29 to ﬁnd his speed

when he hit ground,

vy= (0) −(9.8 m/s2)(0.31 s) = −3.1 m/s.

This becomes the initial velocity for the deceleration motion, so his average speed during deceleration

is given by Eq. 2-27,

vav,y =1

2(vy+v0y) = 1

2((0) + (−3.1 m/s)) = −1.6 m/s.

This average speed, used with the distance of -2.2 cm (-0.022 m), can be used to ﬁnd the time of

deceleration

vav,y = ∆y/∆t,

and putting numbers into the expression gives ∆t= 0.014 s. Finally, we use this in Eq. 2-26 to ﬁnd

the acceleration,

(0) = (−3.1 m/s) + a(0.014 s),

which gives a= 220 m/s2.

(b) The average net force on the man is

XFy=may= (83 kg)(220 m/s2) = 1.8×104N.

E3-24 The average speed of the salmon while decelerating is 4.6 ft/s. The time required to stop

the salmon is then t=x/vav = (0.38 ft)/(4.6 ft/s) = 8.3×10−2s. The deceleration of the salmon

is a= ∆v/∆t= (9.2 ft/s)/(8.2-2s) = 110 ft/s2. The force on the salmon is then F=W a/g =

(19 lb)(110 ft/s2)/(32 ft/s2) = 65 lb.

E3-25 From appendix G we ﬁnd 1 lb = 4.448 N; so the weight is (100 lb)(4.448 N/1 lb) = 445

N; similarly the cord will break if it pulls upward on the object with a force greater than 387 N.

The mass of the object is m=W/g = (445 N)/(9.8 m/s2) = 45 kg.

There are two vertical forces on the 45 kg object, an upward force from the cord FOC (which has a

maximum value of 387 N) and a downward force from gravity FOG. Then PFy=FOC −FOG = (387

N) −(445 N) = −58 N. Since the net force is negative, the object must be accelerating downward

according to

ay=XFy/m = (−58 N)/(45 kg) = −1.3 m/s2.

E3-26 (a) Constant speed means no acceleration, hence no net force; this means the weight is

balanced by the force from the scale, so the scale reads 65 N.

(b) Net force on mass is Fnet =ma =W a/g = (65 N)(−2.4 m/s2)/(9.81 m/s2) = −16 N.. Since

the weight is 65 N, the scale must be exerting a force of (−16 N) −(−65 N) = 49 N.

E3-27 The magnitude of the net force is W−R= (1600 kg)(9.81 m/s2)−(3700 N) = 12000 N.

The acceleration is then a=F/m = (12000 N)/(1600 kg) = 7.5 m/s2. The time to fall is

t=p2y/a =p2(−72 m)/(−7.5 m/s2) = 4.4 s.

The ﬁnal speed is v=at = (−7.5 m/s2)(4.4 s) = 33 m/s. Get better brakes, eh?

E3-28 The average speed during the acceleration is 140 ft/s. The time for the plane to travel 300

ft is

t=x/vav = (300 ft)/(140 ft/s) = 2.14 s.

The acceleration is then

a= ∆v/∆t= (280 ft/s)/(2.14 s) = 130 ft/s2.

The net force on the plane is F=ma =W a/g = (52000 lb)(130 ft/s2)/(32 ft/s2) = 2.1×105lb.

The force exerted by the catapult is then 2.1×105lb −2.4×104lb = 1.86×105lb.

E3-29 (a) The acceleration of a hovering rocket is 0, so the net force is zero; hence the thrust must

equal the weight. Then T=W=mg = (51000 kg)(9.81 m/s2) = 5.0×105N.

(b) If the rocket accelerates upward then the net force is F=ma = (51000 kg)(18 m/s2) =

9.2×105N. Now Fnet =T−W, so T= 9.2×105N+5.0×105N = 1.42×106N.

E3-30 (a) Net force on parachute + person system is Fnet =ma = (77 kg + 5.2 kg)(−2.5 s2) =

−210 N. The weight of the system is W=mg = (77 kg+5.2 kg)(9.81 s2) = 810 N. If Pis the upward

force of the air on the system (parachute) then P=Fnet +W= (−210 N) + (810 N) = 600 N.

(b) The net force on the parachute is Fnet =ma = (5.2 kg)(−2.5 s2) = −13 N. The weight of the

parachute is W=mg = (5.2 kg)(9.81 m/s2) = 51 N. If Dis the downward force of the person on

the parachute then D=−Fnet −W+P=−(−13 N) −(51 N) + 600 N = 560 N.

E3-31 (a) The total mass of the helicopter+car system is 19,500 kg; and the only other force

acting on the system is the force of gravity, which is

W=mg = (19,500 kg)(9.8 m/s2) = 1.91×105N.

The force of gravity is directed down, so the net force on the system is PFy=FBA −(1.91×105

N). The net force can also be found from Newton’s second law: PFy=may= (19,500 kg)(1.4

m/s2)=2.7×104N. Equate the two expressions for the net force, FBA −(1.91×105N) = 2.7×104

N, and solve; FBA = 2.2×105N.

(b) Repeat the above steps except: (1) the system will consist only of the car, and (2) the upward

force on the car comes from the supporting cable only FCC . The weight of the car is W=mg = (4500

kg)(9.8 m/s2)=4.4×104N. The net force is PFy=FCC −(4.4×104N), it can also be written as

PFy=may= (4500 kg)(1.4 m/s2) = 6300 N. Equating, FCC = 50,000 N.

P3-1 (a) The acceleration is a=F/m = (2.7×10−5N)/(280 kg) = 9.64×10−8m/s2. The displace-

ment (from the original trajectory) is

y=at2/2 = (9.64×10−8m/s2)(2.4 s)2/2 = 2.8×10−7m.

(b) The acceleration is a=F/m = (2.7×10−5N)/(2.1 kg) = 1.3×10−5m/s2. The displacement

(from the original trajectory) is

y=at2/2 = (1.3×10−5m/s2)(2.4 s)2/2 = 3.7×10−5m.

P3-2 (a) The acceleration of the sled is a=F/m = (5.2 N)/(8.4 kg) = 0.62 m/s2.

(b) The acceleration of the girl is a=F/m = (5.2 N)/(40 kg) = 0.13 m/s2.

The two meet when x1+x2= 15 m. This happens when (a1+a2)t2= 30 m. They then meet when

t=p(30 m)/(0.13 m/s2+ 0.62 m/s2) = 6.3 s. The girl moves x1= (0.13 m/s2)(6.3 s)2/2 = 2.6 m.

P3-3 (a) Start with block one. It starts from rest, accelerating through a distance of 16 m in a

time of 4.2 s. Applying Eq. 2-28,

x=x0+v0xt+1

2axt2,

−16 m = (0) + (0)(4.2 s) + 1

2ax(4.2 s)2,

ﬁnd the acceleration to be ax=−1.8 m/s2.

Now for the second block. The acceleration of the second block is identical to the ﬁrst for much

the same reason that all objects fall with approximately the same acceleration.

(b) The initial and ﬁnal velocities are related by a sign, then vx=−v0xand Eq. 2-26 becomes

vx=v0x+axt,

−v0x=v0x+axt,

−2v0x= (−1.8 m/s2)(4.2 s).

which gives an initial velocity of v0x= 3.8 m/s.

The distance traveled is found from Eq. 2-28,

x= (0) + (0)(2.1 s) + 1

2(−1.8 m/s2)(2.1 s)2=−4.0 m.

P3-4 (a) The weight of the engine is W=mg = (1400 kg)(9.81 m/s2)=1.37×104N. If each bolt

supports 1/3 of this, then the force on a bolt is 4600 N.

(b) If engine accelerates up at 2.60 m/s2, then net force on the engine is

Fnet =ma = (1400 kg)(2.60 m/s2) = 3.64×103N.

The upward force from the bolts must then be

B=Fnet +W= (3.64×103N) + (1.37×104N) = 1.73×104N.

The force per bolt is one third this, or 5800 N.

P3-5 (a) If craft descends with constant speed then net force is zero, so thrust balances weight.

The weight is then 3260 N.

(b) If the thrust is 2200 N the net force is 2200 N −3260 N = −1060 N. The mass is then

m=F/a = (−1060 N)/(−0.390 m/s2) = 2720 kg.

P3-6 The weight is originally Mg. The net force is originally −Ma. The upward force is then

originally B=Mg −Ma. The goal is for a net force of (M−m)aand a weight (M−m)g. Then

(M−m)a=B−(M−m)g=Mg −Ma −Mg +mg =mg −Ma,

or m= 2Ma/(a+g).

P3-7 (a) Consider all three carts as one system. Then

XFx=mtotalax,

6.5 N = (3.1 kg + 2.4 kg + 1.2 kg)ax,

0.97 m/s2=ax.

(b) Now choose your system so that it only contains the third car. Then

XFx=F23 =m3ax= (1.2 kg)(0.97 m/s2).

The unknown can be solved to give F23 = 1.2 N directed to the right.

applied to the system gives

F12 = (m2+m3)ax= (2.4 kg + 1.2 kg)(0.97 m/s2) = 3.5 N.

P3-8 (a) F=ma = (45.2 kg + 22.8 kg + 34.3 kg)(1.32 m/s2) = 135 N.

(b) Consider only m3. Then F=ma = (34.3 kg)(1.32 m/s2) = 45.3 N.

P3-9 (c) The net force on each link is the same, Fnet =ma = (0.100 kg)(2.50 m/s2) = 0.250 N.

(a) The weight of each link is W=mg = (0.100 kg)(9.81 m/s2) = 0.981 N. On each link (except

the top or bottom link) there is a weight, an upward force from the link above, and a downward force

from the link below. Then Fnet =U−D−W. Then U=Fnet +W+D= (0.250 N)+(0.981 N)+D=

1.231 N + D. For the bottom link D= 0. For the bottom link, U= 1.23 N. For the link above,

U= 1.23 N + 1.23 N = 2.46 N. For the link above, U= 1.23 N + 2.46 N = 3.69 N. For the link above,

U= 1.23 N + 3.69 N = 4.92 N.

(b) For the top link, the upward force is U= 1.23 N + 4.92 N = 6.15 N.

P3-10 (a) The acceleration of the two blocks is a=F/(m1+m2) The net force on block 2 is from

the force of contact, and is

P=m2a=F m2/(m1+m2) = (3.2 N)(1.2 kg)/(2.3 kg + 1.2 kg) = 1.1 N.

(b) The acceleration of the two blocks is a=F/(m1+m2) The net force on block 1 is from the

force of contact, and is

P=m1a=F m1/(m1+m2) = (3.2 N)(2.3 kg)/(2.3 kg + 1.2 kg) = 2.1 N.

Not a third law pair, eh?

P3-11 (a) Treat the system as including both the block and the rope, so that the mass of

the system is M+m. There is one (relevant) force which acts on the system, so PFx=P. Then

Newton’s second law would be written as P= (M+m)ax. Solve this for axand get ax=P/(M+m).

(b) Now consider only the block. The horizontal force doesn’t act on the block; instead, there is

the force of the rope on the block. We’ll assume that force has a magnitude R, and this is the only

(relevant) force on the block, so PFx=Rfor the net force on the block.. In this case Newton’s

second law would be written R=Max. Yes, axis the same in part (a) and (b); the acceleration of

the block is the same as the acceleration of the block + rope. Substituting in the results from part

(a) we ﬁnd

R=M

M+mP.

E4-1 (a) The time to pass between the plates is t=x/vx= (2.3 cm)/(9.6×108cm/s) = 2.4×10−9s.

(b) The vertical displacement of the beam is then y=ayt2/2 == (9.4×1016 cm/s2)(2.4×

10−9s)2/2 = 0.27 cm.

10−9s) = 2.3×108cm/s.

E4-2 ~

a= ∆~

v/∆t=−(6.30ˆ

i−8.42ˆ

j)(m/s)/(3 s) = (−2.10ˆ

i+ 2.81ˆ

j)(m/s2).

E4-3 (a) The velocity is given by

dt =d

dt Aˆ

i+d

dt Bt2ˆ

j+d

dt Ctˆ

k,

v= (0) + 2Btˆ

j+Cˆ

(b) The acceleration is given by

dt =d

dt 2Btˆ

j+d

dt Cˆ

k,

a= (0) + 2Bˆ

j+ (0).

in this plane is a parabola.

E4-4 (a) Maximum xis when vx= 0. Since vx=axt+vx,0,vx= 0 when t=−vx,0/ax=

−(3.6 m/s)/(−1.2 m/s2) = 3.0 s.

(b) Since vx= 0 we have |~

v|=|vy|. But vy=ayt+vy,0=−(1.4 m/s)(3.0 s) + (0) = −4.2 m/s.

Then |~

v|= 4.2 m/s.

r=~

at2/2 + ~

v0t, so

r= [−(0.6 m/s2)ˆ

i−(0.7 m/s2)ˆ

j](3.0 s)2+ [(3.6 m/s)ˆ

i](3.0 s) = (5.4 m)ˆ

i−(6.3 m)ˆ

E4-5 ~

F=~

F1+~

F2= (3.7 N)ˆ

j+ (4.3 N)ˆ

i. Then ~

a=~

F/m = (0.71 m/s2)ˆ

j+ (0.83 m/s2)ˆ

E4-6 (a) The acceleration is ~

a=~

F/m = (2.2 m/s2)ˆ

j. The velocity after 15 seconds is ~

v=~

at+~

v0,

v= [(2.2 m/s2)ˆ

j](15 s) + [(42 m/s)ˆ

i] = (42 m/s)ˆ

i+ (33 m/s)ˆ

(b) ~

r=~

at2/2 + ~

v0t, so

r= [(1.1 m/s2)ˆ

j](15 s)2+ [(42 m/s)ˆ

i](15 s) = (630 m)ˆ

i+ (250 m)ˆ

E4-7 The block has a weight W=mg = (5.1 kg)(9.8 m/s2) = 50 N.

(a) Initially P= 12 N, so Py= (12 N) sin(25◦) = 5.1 N and Px= (12 N) cos(25◦) = 11 N. Since

the upward component is less than the weight, the block doesn’t leave the ﬂoor, and a normal force

will be present which will make PFy= 0. There is only one contribution to the horizontal force,

so PFx=Px. Newton’s second law then gives ax=Px/m = (11 N)/(5.1 kg) = 2.2 m/s2.

(b) As Pis increased, so is Py; eventually Pywill be large enough to overcome the weight of the

block. This happens just after Py=W= 50 N, which occurs when P=Py/sin θ= 120 N.

is ax=Px/m = 22 m/s2.

E4-8 (a) The block has weight W=mg = (96.0 kg)(9.81 m/s2) = 942 N. Px= (450 N) cos(38◦) =

355 N; Py= (450 N) sin(38◦) = 277 N. Since Py< W the crate stays on the ﬂoor and there is a

normal force N=W−Py. The net force in the xdirection is Fx=Px−(125 N) = 230 N. The

acceleration is ax=Fx/m = (230 N)/(96.0 kg) = 2.40 m/s2.

(b) The block has mass m=W/g = (96.0 N)/(9.81 m/s2)=9.79 kg. Px= (450 N) cos(38◦) =

355 N; Py= (450 N) sin(38◦) = 277 N. Since Py> W the crate lifts oﬀ of the ﬂoor! The net

force in the xdirection is Fx=Px−(125 N) = 230 N. The xacceleration is ax=Fx/m =

(230 N)/(9.79 kg) = 23.5 m/s2. The net force in the ydirection is Fy=Py−W= 181 N. The y

acceleration is ay=Fy/m = (181 N)/(9.79 kg) = 18.5 m/s2. Wow.

E4-9 Let ybe perpendicular and xbe parallel to the incline. Then P= 4600 N;

Px= (4600 N) cos(27◦) = 4100 N;

Py= (4600 N) sin(27◦) = 2090 N.

The weight of the car is W=mg = (1200 kg)(9.81 m/s2) = 11800 N;

Wx= (11800 N) sin(18◦) = 3650 N;

Wy= (11800 N) cos(18◦) = 11200 N.

Since Wy> Pythe car stays on the incline. The net force in the xdirection is Fx=Px−Wx= 450 N.

The acceleration in the xdirection is ax=Fx/m = (450 N)/(1200 kg) = 0.375 m/s2. The distance

traveled in 7.5 s is x=axt2/2 = (0.375 m/s2)(7.5 s)2/2 = 10.5 m.

E4-10 Constant speed means zero acceleration, so net force is zero. Let ybe perpendicular and xbe

parallel to the incline. The weight is W=mg = (110 kg)(9.81 m/s2) = 1080 N; Wx=Wsin(34◦);

Wy=Wcos(34◦). The push Fhas components Fx=Fcos(34◦) and Fy=−Fsin(34◦). The

ycomponents will balance after a normal force is introduced; the xcomponents will balance if

Fx=Wx, or F=Wtan(34◦) = (1080 N) tan(34◦) = 730 N.

E4-11 If the xaxis is parallel to the river and the yaxis is perpendicular, then ~

a= 0.12ˆ

im/s2.

The net force on the barge is

F=m~

a= (9500 kg)(0.12ˆ

im/s2) = 1100ˆ

iN.

The force exerted on the barge by the horse has components in both the xand ydirection. If

P= 7900 N is the magnitude of the pull and θ= 18◦is the direction, then ~

P=Pcos θˆ

i+Psin θˆ

(7500ˆ

i+ 2400ˆ

j) N.

Let the force exerted on the barge by the water be ~

Fw=Fw,xˆ

i+Fw,yˆ

j. Then PFx= (7500

N) + Fw,x and PFy= (2400 N) + Fw,y . But we already found P~

F, so

Fx= 1100 N = 7500 N + Fw,x,

Fy= 0 = 2400 N + Fw,y.

Solving, Fw,x =−6400 N and Fw,y =−2400 N. The magnitude is found by Fw=qF2

w,x +F2

w,y =

6800 N.

E4-12 (a) Let ybe perpendicular and xbe parallel to the direction of motion of the plane.

Then Wx=mg sin θ;Wy=mg cos θ;m=W/g. The plane is accelerating in the xdirection, so

ax= 2.62 m/s2; the net force is in the xdirection, where Fx=max. But Fx=T−Wx, so

T=Fx+Wx=Wax

g+Wsin θ= (7.93×104N) (2.62 m/s2)

(9.81 m/s2)+ sin(27◦)= 5.72×104N.

(b) There is no motion in the ydirection, so

L=Wy= (7.93×104N) cos(27◦) = 7.07×104N.

E4-13 (a) The ball rolled oﬀ horizontally so v0y= 0. Then

y=v0yt−1

2gt2,

(−4.23 ft) = (0)t−1

2(32.2 ft/s2)t2,

which can be solved to yield t= 0.514 s.

(b) The initial velocity in the xdirection can be found from x=v0xt; rearranging, v0x=x/t =

(5.11 ft)/(0.514 s) = 9.94 ft/s. Since there is no ycomponent to the velocity, then the initial speed

is v0= 9.94 ft/s.

E4-14 The electron travels for a time t=x/vx. The electron “falls” vertically through a distance

y=−gt2/2 in that time. Then

y=−g

2x

vx2

=−(9.81 m/s2)

2(1.0 m)

(3.0×107m/s)2

=−5.5×10−15 m.

E4-15 (a) The dart “falls” vertically through a distance y=−gt2/2 = −(9.81 m/s2)(0.19 s)2/2 =

−0.18 m.

(b) The dart travels horizontally x=vxt= (10 m/s)(0.19 s) = 1.9 m.

E4-16 The initial velocity components are

vx,0= (15 m/s) cos(20◦) = 14 m/s

and

vy,0=−(15 m/s) sin(20◦) = −5.1 m/s.

(a) The horizontal displacement is x=vxt= (14 m/s)(2.3 s) = 32 m.

(b) The vertical displacement is

y=−gt2/2 + vy,0t=−(9.81 m/s2)(2.3 s)2/2+(−5.1 m/s)(2.3 s) = −38 m.

E4-17 Find the time in terms of the the initial ycomponent of the velocity:

vy=v0y−gt,

(0) = v0y−gt,

t=v0y/g.

Use this time to ﬁnd the highest point:

y=v0yt−1

2gt2,

ymax =v0yv0y

g−1

2gv0y

g2

=v2

2g.

Finally, we know the initial ycomponent of the velocity from Eq. 2-6, so ymax = (v0sin φ0)2/2g.

E4-18 The horizontal displacement is x=vxt. The vertical displacement is y=−gt2/2. Combin-

ing, y=−g(x/vx)2/2. The edge of the nth step is located at y=−nw,x=nw, where w= 2/3 ft.

If |y|> nw when x=nw then the ball hasn’t hit the step. Solving,

g(nw/vx)2/2< nw,

gnw/v2

x<2,

n < 2v2

x/(gw) = 2(5.0 ft/s)2/[(32 ft/s2)(2/3 ft)] = 2.34.

Then the ball lands on the third step.

E4-19 (a) Start from the observation point 9.1 m above the ground. The ball will reach the

highest point when vy= 0, this will happen at a time tafter the observation such that t=vy,0/g =

(6.1 m/s)/(9.81 m/s2) = 0.62 s. The vertical displacement (from the ground) will be

y=−gt2/2 + vy,0t+y0=−(9.81 m/s2)(0.62 s)2/2 + (6.1 m/s)(0.62 s) + (9.1 m) = 11 m.

(b) The time for the ball to return to the ground from the highest point is t=p2ymax/g =

p2(11 m)/(9.81 m/s2) = 1.5 s. The total time of ﬂight is twice this, or 3.0 s. The horizontal distance

traveled is x=vxt= (7.6 m/s)(3.0 s) = 23 m.

v=~

at+~

v0= (−9.81 m/s2)ˆ

j(1.5 s) + (7.6 m/s)ˆ

i= 7.6 m/sˆ

i−15 m/suj.

The magnitude is √7.62+ 152(m/s) = 17 m/s. The direction is

θ= arctan(−15/7.6) = −63◦.

E4-20 Focus on the time it takes the ball to get to the plate, assuming it traveled in a straight

line. The ball has a “horizontal” velocity of 135 ft/s. Then t=x/vx= (60.5 ft)/(135 ft/s) = 0.448 s.

The ball will “fall” a vertical distance of y=−gt2/2 = −(32 ft/s2)(0.448 s)2/2 = −3.2 ft. That’s in

the strike zone.

E4-21 Since R∝1/g one can write R2/R1=g1/g2, or

∆R=R2−R1=R11−g1

g2= (8.09 m) 1−(9.7999 m/s2)

(9.8128 m/s2)= 1.06 cm.

E4-22 If initial position is ~

r0= 0, then ﬁnal position is ~

r= (13 ft)ˆ

i+ (3 ft)ˆ

j. The initial velocity

is ~

v0=vcos θˆ

i+vsin θˆ

j. The horizontal equation is (13 ft) = vcos θt; the vertical equation is

(3 ft) = −(g/2)t2+vsin θt. Rearrange the vertical equation and then divide by the horizontal

equation to get

3 ft + (g/2)t2

(13 ft) = tan θ,

t2= [(13 ft) tan(55◦)−(3 ft)][2/(32 m/s2)] = 0.973 s2,

or t= 0.986 s. Then v= (13 ft)/(cos(55◦)(0.986 s)) = 23 ft/s.

E4-23 vx=x/t = (150 ft)/(4.50 s) = 33.3 ft/s. The time to the highest point is half the hang

time, or 2.25 s. The vertical speed when the ball hits the ground is vy=−gt =−(32 ft/s2)(2.25 s) =

72.0 ft/s. Then the initial vertical velocity is 72.0 ft/s. The magnitude of the initial velocity is

√722+ 332(ft/s) = 79 ft/s. The direction is

θ= arctan(72/33) = 65◦.

E4-24 (a) The magnitude of the initial velocity of the projectile is v= 264 ft/s. The projectile

was in the air for a time twhere

t=x

vcos θ=(2300 ft)

(264 ft/s) cos(−27◦)= 9.8 s.

(b) The height of the plane was −ywhere

−y=gt2/2−vy,0t= (32 ft/s)(9.8 s)2/2−(264 ft/s) sin(−27◦)(9.8 s) = 2700 ft.

E4-25 Deﬁne the point the ball leaves the racquet as ~

r= 0.

(a) The initial conditions are given as v0x= 23.6 m/s and v0y= 0. The time it takes for the ball

to reach the horizontal location of the net is found from

x=v0xt,

(12 m) = (23.6 m/s)t,

0.51 s = t,

Find how far the ball has moved horizontally in this time:

y=v0yt−1

2gt2= (0)(0.51 s) −1

2(9.8 m/s2)(0.51 s)2=−1.3 m.

Did the ball clear the net? The ball started 2.37 m above the ground and “fell” through a distance

of 1.3 m by the time it arrived at the net. So it is still 1.1 m above the ground and 0.2 m above the

net.

(b) The initial conditions are now given by v0x= (23.6 m/s)(cos[−5.0◦]) = 23.5 m/s and v0y=

(23.6 m/s)(sin[−5.0◦]) = −2.1 m/s. Now ﬁnd the time to reach the net just as done in part (a):

t=x/v0x= (12.0 m)/(23.5 m/s) = 0.51 s.

Find the vertical position of the ball when it arrives at the net:

y=v0yt−1

2gt2= (−2.1 m/s)(0.51 s) −1

2(9.8 m/s2)(0.51 s)2=−2.3 m.

Did the ball clear the net? Not this time; it started 2.37 m above the ground and then passed the

net 2.3 m lower, or only 0.07 m above the ground.

E4-26 The initial speed of the ball is given by v=√gR =q(32 ft/s2)(350 ft) = 106 ft/s. The

time of ﬂight from the batter to the wall is

t=x/vx= (320 ft)/[(106 ft/s) cos(45◦)] = 4.3 s.

The height of the ball at that time is given by y=y0+v0yt−1

2gt2, or

y= (4 ft) + (106 ft/s) sin(45◦)(4.3 s) −(16 ft/s2)(4.3 s)2= 31 ft,

clearing the fence by 7 feet.

E4-27 The ball lands x= (358 ft) + (39 ft) cos(28◦) = 392 ft from the initial position. The ball

lands y= (39 ft) sin(28◦)−(4.60 ft) = 14 ft above the initial position. The horizontal equation

is (392 ft) = vcos θt; the vertical equation is (14 ft) = −(g/2)t2+vsin θt. Rearrange the vertical

equation and then divide by the horizontal equation to get

14 ft + (g/2)t2

(392 ft) = tan θ,

t2= [(392 ft) tan(52◦)−(14 ft)][2/(32 m/s2)] = 30.5 s2,

or t= 5.52 s. Then v= (392 ft)/(cos(52◦)(5.52 s)) = 115 ft/s.

E4-28 Since ball is traveling at 45◦when it returns to the same level from which it was thrown

for maximum range, then we can assume it actually traveled ≈1.6 m. farther than it would have

had it been launched from the ground level. This won’t make a big diﬀerence, but that means that

R= 60.0 m −1.6 m = 58.4 m. If v0is initial speed of ball thrown directly up, the ball rises to the

highest point in a time t=v0/g, and that point is ymax =gt2/2 = v2

0/(2g) above the launch point.

But v2

0=gR, so ymax =R/2 = (58.4 m)/2 = 29.2 m. To this we add the 1.60 m point of release to

get 30.8 m.

E4-29 The net force on the pebble is zero, so PFy= 0. There are only two forces on the

pebble, the force of gravity Wand the force of the water on the pebble FP W . These point in

opposite directions, so 0 = FP W −W. But W=mg = (0.150 kg)(9.81 m/s2) = 1.47 N. Since

FP W =Win this problem, the force of the water on the pebble must also be 1.47 N.

E4-30 Terminal speed is when drag force equal weight, or mg =bvT2. Then vT=pmg/b.

E4-31 Eq. 4-22 is

vy(t) = vT1−e−bt/m,

where we have used Eq. 4-24 to substitute for the terminal speed. We want to solve this equation

for time when vy(t) = vT/2, so

2vT=vT1−e−bt/m,

2=1−e−bt/m,

e−bt/m =1

bt/m =−ln(1/2)

t=m

bln 2

E4-32 The terminal speed is 7 m/s for a raindrop with r= 0.15 cm. The mass of this drop is

m= 4πρr3/3, so

b=mg

=4π(1.0×10−3kg/cm3)(0.15 cm)3(9.81 m/s2)

3(7 m/s) = 2.0×10−5kg/s.

E4-33 (a) The speed of the train is v= 9.58 m/s. The drag force on one car is f= 243(9.58) N =

2330 N. The total drag force is 23(2330 N) = 5.36×104N. The net force exerted on the cars (treated

as a single entity) is F=ma = 23(48.6×103kg)(0.182 m/s2) = 2.03×105N. The pull of the locomotive

is then P= 2.03×105N+5.36×104N = 2.57×105N.

(b) If the locomotive is pulling the cars at constant speed up an incline then it must exert a

force on the cars equal to the sum of the drag force and the parallel component of the weight. The

drag force is the same in each case, so the parallel component of the weight is W|| =Wsin θ=

2.03×105N = ma, where ais the acceleration from part (a). Then

θ= arcsin(a/g) = arcsin[(0.182 m/s2)/(9.81 m/s2)] = 1.06◦.

E4-34 (a) a=v2/r = (2.18×106m/s)2/(5.29×10−11m) = 8.98×1022m/s2.

(b) F=ma = (9.11×10−31kg)(8.98×1022m/s2) = 8.18×10−8N, toward the center.

E4-35 (a) v=√rac=q(5.2 m)(6.8)(9.8 m/s2) = 19 m/s.

(b) Use the fact that one revolution corresponds to a length of 2πr:

19m

s1 rev

2π(5.2 m) 60 s

1 min= 35 rev

min.

E4-36 (a) v= 2πr/T = 2π(15 m)/(12 s) = 7.85 m/s. Then a=v2/r = (7.85 m/s)2/(15 m) =

4.11 m/s2, directed toward center, which is down.

(b) Same arithmetic as in (a); direction is still toward center, which is now up.

The weight of the person is the same in both parts: W=mg = (75 kg)(9.81 m/s2) = 740 N. At

the top the net force is down, the weight is down, so the Ferris wheel is pushing up with a force of

P=W−F= (740 N) −(310 N) = 430 N. At the bottom the net force is up, the weight is down, so

the Ferris wheel is pushing up with a force of P=W+F= (740 N) + (310 N) = 1050 N.

E4-37 (a) v= 2πr/T = 2π(20×103m)/(1.0 s) = 1.26×105m/s.

(b) a=v2/r = (1.26×105m/s)2/(20×103m) = 7.9×105m/s2.

E4-38 (a) v= 2πr/T = 2π(6.37 ×106m)/(86400 s) = 463 m/s. a=v2/r = (463 m/s)2/(6.37 ×

106m) = 0.034 m/s2.

(b) The net force on the object is F=ma = (25.0 kg)(0.034 m/s2) = 0.85 N. There are two forces

on the object: a force up from the scale (S), and the weight down, W=mg = (25.0 kg)(9.80 m/s2) =

245 N. Then S=F+W= 245 N + 0.85 N = 246 N.

E4-39 Let ∆x= 15 m be the length; tw= 90 s, the time to walk the stalled Escalator; ts= 60

s, the time to ride the moving Escalator; and tm, the time to walk up the moving Escalator.

The walking speed of the person relative to a ﬁxed Escalator is vwe = ∆x/tw; the speed of the

Escalator relative to the ground is veg = ∆x/ts; and the speed of the walking person relative to the

ground on a moving Escalator is vwg = ∆x/tm. But these three speeds are related by vwg =vwe +veg .

Combine all the above:

vwg =vwe +veg,

∆x

=∆x

+∆x

Putting in the numbers, tm= 36 s.

E4-40 Let vwbe the walking speed, vsbe the sidewalk speed, and vm=vw+vsbe Mary’s speed

while walking on the moving sidewalk. All three cover the same distance x, so vi=x/ti, where iis

one of w, s, or m. Put this into the Mary equation, and

1/tm= 1/tw+ 1/ts= 1/(150 s) + 1/(70 s) = 1/48 s.

E4-41 If it takes longer to ﬂy westward then the speed of the plane (relative to the ground)

westward must be less than the speed of the plane eastward. We conclude that the jet-stream must

be blowing east. The speed of the plane relative to the ground is ve=vp+vjwhen going east and

vw=vp−vjwhen going west. In either case the distance is the same, so x=viti, where iis e or

w. Since tw−teis given, we can write

tw−te=x

vp−vj−x

vp+vj

=x2vj

vp2−vj2.

Solve the quadratic if you want, but since vjvpwe can neglect it in the denominator and

vj=vp2(0.83 h)/(2x) = (600 mi/h)2(0.417 h)/(2700 mi) = 56 mi/hr.

E4-42 The horizontal component of the rain drop’s velocity is 28 m/s. Since vx=vsin θ,v=

(28 m/s)/sin(64◦) = 31 m/s.

E4-43 (a) The position of the bolt relative to the elevator is ybe, the position of the bolt relative

to the shaft is ybs, and the position of the elevator relative to the shaft is yes. Zero all three positions

at t= 0; at this time v0,bs =v0,es = 8.0 ft/s.

The three equations describing the positions are

ybs =v0,bst−1

2gt2,

yes =v0,est+1

2at2,

ybe +res =rbs,

where a= 4.0 m/s2is the upward acceleration of the elevator. Rearrange the last equation and

solve for ybe; get ybe =−1

2(g+a)t2, where advantage was taken of the fact that the initial velocities

are the same.

Then

t=p−2ybe/(g+a) = q−2(−9.0 ft)/(32 ft/s2+ 4 ft/s2) = 0.71 s

(b) Use the expression for ybs to ﬁnd how the bolt moved relative to the shaft:

ybs =v0,bst−1

2gt2= (8.0 ft)(0.71 s) −1

2(32 ft/s2)(0.71 s)2=−2.4 ft.

E4-44 The speed of the plane relative to the ground is vpg = (810 km)/(1.9 h) = 426 km/h. The

velocity components of the plane relative to the air are vN= (480 km/h) cos(21◦) = 448 km/h and

vE= (480 km/h) sin(21◦) = 172 km/h. The wind must be blowing with a component of 172 km/h

to the west and a component of 448 −426 = 22 km/h to the south.

E4-45 (a) Let ˆ

ipoint east and ˆ

jpoint north. The velocity of the torpedo is ~

v= (50 km/h)ˆ

isin θ+

(50 km/h)ˆ

jcos θ. The initial coordinates of the battleship are then ~

r0= (4.0 km)ˆ

isin(20◦) +

(4.0 km)ˆ

jcos(20◦) = (1.37 km)ˆ

i+ (3.76 km)ˆ

j. The ﬁnal position of the battleship is ~

r= (1.37 km +

24 km/ht)ˆ

i+ (3.76 km)ˆ

j, where tis the time of impact. The ﬁnal position of the torpedo is the

same, so

[(50 km/h)ˆ

isin θ+ (50 km/h)ˆ

jcos θ]t= (1.37 km + 24 km/ht)ˆ

i+ (3.76 km)ˆ

[(50 km/h) sin θ]t−24 km/ht= 1.37 km

and

[(50 km/h) cos θ]t= 3.76 km.

Dividing the top equation by the bottom and rearranging,

50 sin θ−24 = 18.2 cos θ.

Use any trick you want to solve this. I used Maple and found θ= 46.8◦.

(b) The time to impact is then t= 3.76 km/[(50 km/h) cos(46.8◦)] = 0.110 h, or 6.6 minutes.

P4-1 Let ~

rAbe the position of particle of particle A, and ~

rBbe the position of particle B. The

equations for the motion of the two particles are then

rA=~

r0,A +~

vt,

=dˆ

j+vtˆ

rB=1

at2,

2a(sin θˆ

i+ cos θˆ

j)t2.

A collision will occur if there is a time when ~

rA=~

rB. Then

dˆ

j+vtˆ

i=1

2a(sin θˆ

i+ cos θˆ

j)t2,

but this is really two equations: d=1

2at2cos θand vt =1

2at2sin θ.

Solve the second one for tand get t= 2v/(asin θ). Substitute that into the ﬁrst equation, and

then rearrange,

d=1

2at2cos θ,

d=1

2a2v

asin θ2

cos θ,

sin2θ=2v

ad cos θ,

1−cos2θ=2v2

ad cos θ,

0 = cos2θ+2v2

ad cos θ−1.

This last expression is quadratic in cos θ. It simpliﬁes the solution if we deﬁne b= 2v/(ad) = 2(3.0

m/s)2/([0.4 m/s2][30 m]) = 1.5, then

cos θ=−b±√b2+ 4

2=−0.75 ±1.25.

Then cos θ= 0.5 and θ= 60◦.

P4-2 (a) The acceleration of the ball is ~

a= (1.20 m/s2)ˆ

i−(9.81 m/s2)ˆ

j. Since ~

ais constant the

trajectory is given by ~

r=~

at2/2, since ~

v0= 0 and we choose ~

r0= 0. This is a straight line trajectory,

with a direction given by ~

a. Then

θ= arctan(9.81/1.20) = 83.0◦.

and R= (39.0 m)/tan(83.0◦) = 4.79 m. It will be useful to ﬁnd H= (39.0 m)/sin(83.0◦) = 39.3 m.

(b) The magnitude of the acceleration of the ball is a=√9.812+ 1.202(m/s2) = 9.88 m/s2. The

time for the ball to travel down the hypotenuse of the ﬁgure is then t=p2(39.3 m)/(9.88 m/s2) =

2.82 s.

v=at = (9.88 m/s2)(2.82 s) = 27.9 m/s.

P4-3 (a) The rocket thrust is ~

T= (61.2 kN) cos(58.0◦)ˆ

i+ (61.2 kN) sin(58.0◦)ˆ

i= 32.4 kNˆ

51.9 kNˆ

j. The net force on the rocket is the ~

F=~

T+~

W, or

F= 32.4 kNˆ

i+ 51.9 kNˆ

j−(3030 kg)(9.81 m/s2)ˆ

j= 32.4 kNˆ

i+ 22.2 kNˆ

The acceleration (until rocket cut-oﬀ) is this net force divided by the mass, or

a= 10.7m/s2ˆ

i+ 7.33m/s2ˆ

The position at rocket cut-oﬀ is given by

r=~

at2/2 = (10.7m/s2ˆ

i+ 7.33m/s2ˆ

j)(48.0 s)2/2,

= 1.23×104mˆ

i+ 8.44×103mˆ

The altitude at rocket cut-oﬀ is then 8.44 km.

(b) The velocity at rocket cut-oﬀ is

v=~

at= (10.7m/s2ˆ

i+ 7.33m/s2ˆ

j)(48.0 s) = 514 m/sˆ

i+ 352 m/sˆ

this becomes the initial velocity for the “free fall” part of the journey. The rocket will hit the ground

after tseconds, where tis the solution to

0 = −(9.81 m/s2)t2/2 + (352 m/s)t+ 8.44×103m.

The solution is t= 90.7 s. The rocket lands a horizontal distance of x=vxt= (514 m/s)(90.7 s) =

4.66×104m beyond the rocket cut-oﬀ; the total horizontal distance covered by the rocket is 46.6 km+

12.3 km = 58.9 km.

P4-4 (a) The horizontal speed of the ball is vx= 135 ft/s. It takes

t=x/vx= (30.0 ft)/(135 ft/s) = 0.222 s

to travel the 30 feet horizontally, whether the ﬁrst 30 feet, the last 30 feet, or 30 feet somewhere in

the middle.

(b) The ball “falls” y=−gt2/2 = −(32 ft/s2)(0.222 s)2/2 = −0.789 ft while traveling the ﬁrst

30 feet.

the ﬁrst 60 feet, so during the last 30 feet it must have fallen (−3.15 ft) −(−0.789 ft) = −2.36 ft.

(d) The distance fallen because of acceleration is not linear in time; the distance moved horizon-

tally is linear in time.

P4-5 (a) The initial velocity of the ball has components

vx,0= (25.3 m/s) cos(42.0◦) = 18.8 m/s

and

vy,0= (25.3 m/s) sin(42.0◦) = 16.9 m/s.

The ball is in the air for t=x/vx= (21.8 m)/(18.8 m/s) = 1.16 s before it hits the wall.

(b) y=−gt2/2 + vy,0t=−(4.91 m/s2)(1.16 s)2+ (16.9 m/s)(1.16 s) = 13.0 m.

(d) Since vy>0 the ball is still heading up.

P4-6 (a) The initial vertical velocity is vy,0=v0sin φ0. The time to the highest point is t=vy,0/g.

The highest point is H=gt2/2. Combining,

H=g(v0sin φ0/g)2/2 = v2

0sin2φ0/(2g).

The range is R= (v2

0/g) sin 2φ0= 2(v2

0/g) sin φ0cos φ0. Since tan θ=H/(R/2), we have

tan θ=2H

R=v2

0sin2φ0/g

2(v2

0/g) sin φ0cos φ0

2tan φ0.

(b) θ= arctan(0.5 tan 45◦) = 26.6◦.

P4-7 The components of the initial velocity are given by v0x=v0cos θ= 56 ft/s and v0y=

v0sin θ= 106 ft/s where we used v0= 120 ft/s and θ= 62◦.

(a) To ﬁnd hwe need only ﬁnd out the vertical position of the stone when t= 5.5 s.

y=v0yt−1

2gt2= (106 ft/s)(5.5 s) −1

2(32 ft/s2)(5.5 s)2= 99 ft.

(b) Look at this as a vector problem:

v=~

v0+~

at,

=v0xˆ

i+v0yˆ

j−gˆ

jt,

=v0xˆ

i+ (v0y−gt)ˆ

= (56 ft/s)ˆ

i+(106 ft/s −(32 ft/s2)(5.5 s)ˆ

= (56 ft/s)ˆ

i+ (−70.0 ft/s)ˆ

The magnitude of this vector gives the speed when t= 5.5 s; v=p562+ (−70)2ft/s= 90 ft/s.

(106 ft/s) −(32 ft/s2)t;t= 3.31 s. Use this time in Eq. 4-10(b),

y=v0yt−1

2gt2= (106 ft/s)(3.31 s) −1

2(32 ft/s2)(3.31 s)2= 176 ft.

P4-8 (a) Since R= (v2

0/g) sin 2φ0, it is suﬃcient to prove that sin 2φ0is the same for both

sin 2(45◦+α) and sin 2(45◦−α).

sin 2(45◦±α) = sin(90◦±2α) = cos(±2α) = cos(2α).

Since the ±dropped out, the two quantities are equal.

(b) φ0= (1/2) arcsin(Rg/v2

0) = (1/2) arcsin((20.0 m)(9.81 m/s2)/(30.0 m/s)2) = 6.3◦. The other

choice is 90◦−6.3◦= 83.7◦.

P4-9 To score the ball must pass the horizontal distance of 50 m with an altitude of no less than

3.44 m. The initial velocity components are v0x=v0cos θand v0y=v0sin θwhere v0= 25 m/s,

and θis the unknown.

The time to the goal post is t=x/v0x=x/(v0cos θ).

The vertical motion is given by

y=v0yt−1

2gt2= (v0sin θ)x

v0cos θ−1

2gx

v0cos θ2

=xsin θ

cos θ−gx2

2v2

cos2θ.

In this last expression yneeds to be greater than 3.44 m. In this last expression use

cos2θ−1 + 1 = 1

cos2θ−cos2θ

cos2θ+ 1 = 1−cos2θ

cos2θ+ 1 = sin2θ

cos2θ+ 1 = tan2θ+ 1.

This gives for our yexpression

y=xtan θ−gx2

2v0tan2θ+ 1,

which can be combined with numbers and constraints to give

(3.44 m) ≤(50 m) tan θ−(9.8 m/s2)(50 m)2

2(25 m/s)2tan2θ+ 1,

3.44 ≤50 tan θ−20 tan2θ+ 1,

0≤ −20 tan2θ+ 50 tan θ−23

Solve, and tan θ= 1.25 ±0.65, so the allowed kicking angles are between θ= 31◦and θ= 62◦.

P4-10 (a) The height of the projectile at the highest point is H=Lsin θ. The amount of time

before the projectile hits the ground is t=p2H/g =p2Lsin θ/g. The horizontal distance covered

by the projectile in this time is x=vxt=vp2Lsin θ/g. The horizontal distance to the projectile

when it is at the highest point is x0=Lcos θ. The projectile lands at

D=x−x0=vp2Lsin θ/g −Lcos θ.

(b) The projectile will pass overhead if D > 0.

P4-11 v2=v2

x+v2

y. For a projectile vxis constant, so we need only evaluate d2(v2

y)/dt2. The ﬁrst

derivative is 2vydvy/dt =−2vyg. The derivative of this (the second derivative) is −2g dvy/dt = 2g2.

P4-12 |~

r|is a maximum when r2is a maximum. r2=x2+y2, or

r2= (vx,0t)2+ (−gt2/2 + vy,0t)2,

= (v0tcos φ0)2+v0tsin φ0−gt2/22,

=v2

0t2−v0gt3sin φ0+g2t4/4.

We want to look for the condition which will allow dr2/dt to vanish. Since

dr2/dt = 2v2

0t−3v0gt2sin φ0+g2t3

we can focus on the quadratic discriminant, b2−4ac, which is

9v2

0g2sin2φ0−8v2

0g2,

a quantity which will only be greater than zero if 9 sin2φ0>8. The critical angle is then

φc= arcsin(p8/9) = 70.5◦.

P4-13 There is a downward force on the balloon of 10.8 kN from gravity and an upward force

of 10.3 kN from the buoyant force of the air. The resultant of these two forces is 500 N down, but

since the balloon is descending at constant speed so the net force on the balloon must be zero. This

is possible because there is a drag force on the balloon of D=bv2, this force is directed upward.

The magnitude must be 500 N, so the constant bis

b=(500 N)

(1.88 m/s)2= 141 kg/m.

If the crew drops 26.5 kg of ballast they are “lightening” the balloon by

(26.5 kg)(9.81 m/s2) = 260 N.

This reduced the weight, but not the buoyant force, so the drag force at constant speed will now be

500 N −260 N = 240 N.

The new constant downward speed will be

v=pD/b =p(240 N)/(141 kg/m) = 1.30 m/s.

P4-14 The constant bis

b= (500 N)/(1.88 m/s) = 266 N ·s/m.

The drag force after “lightening” the load will still be 240 N. The new downward speed will be

v=D/b = (240 N)/(266 N ·s/m) = 0.902 m/s.

P4-15 (a) Initially v0= 0, so D= 0, the only force is the weight, so a=−g.

(b) After some time the acceleration is zero, then W=D, or bvT2=mg, or vT=pmg/b.

−3mg/4. The acceleration is the a=−3g/4.

P4-16 (a) The net force on the barge is F=−D=−bv, this results in a diﬀerential equation

m dv/dt =−bv, which can be written as

dv/v =−(b/m)dt,

Zdv/v =−(b/m)Zdt,

ln(vf/vi) = −bt/m.

Then t= (m/b) ln(vi/vf).

(b) t= [(970 kg)/(68 N ·s/m)] ln(32/8.3) = 19 s.

P4-17 (a) The acceleration is the time derivative of the velocity,

ay=dvy

dt =d

dt mg

b1−e−bt/m=mg

me−bt/m,

which can be simpliﬁed as ay=ge−bt/m. For large tthis expression approaches 0; for small tthe

exponent can be expanded to give

ay≈g1−bt

m=g−vTt,

where in the last line we made use of Eq. 4-24.

(b) The position is the integral of the velocity,

vydt =Zt

0mg

b1−e−bt/m dt,

dt dt =mg

bt−(−m/b)e−bt/m

dy =vTt+vT

ge−vTt/g −1,

y=vTt+vT

ge−vTt/g −1.

P4-18 (a) We have vy=vT(1 −e−bt/m) from Eq. 4-22; this can be substituted into the last line

of the solution for P4-17 to give

y95 =vTt−vy

g.

We can also rearrange Eq. 4-22 to get t=−(m/b) ln(1 −vy/vT), so

y95 =vT2/g −ln(1 −vy/vT)−vy

vT.

But vy/vT= 0.95, so

y95 =vT2/g (−ln(0.05) −0.95) = vT2/g (ln 20 −19/20) .

(b) y95 = (42 m/s)2/(9.81 m/s2)(2.05) = 370 m.

P4-19 (a) Convert units ﬁrst. v= 86.1 m/s, a= 0.05(9.81 m/s2) = 0.491 m/s2. The minimum

radius is r=v2/a = (86.1 m/s)/(0.491 m/s2) = 15 km.

(b) v=√ar =p(0.491 m/s2)(940 m) = 21.5 m/s. That’s 77 km/hr.

P4-20 (a) The position is given by ~

r=Rsin ωtˆ

i+R(1 −cos ωt)ˆ

j, where ω= 2π/(20 s) = 0.314 s−1

and R= 3.0 m. When t= 5.0 s ~

r= (3.0 m)ˆ

i+ (3.0 m)ˆ

j; when t= 7.5 s ~

r= (2.1 m)ˆ

i+ (5.1 m)ˆ

when t= 10 s ~

r= (6.0 m)ˆ

j. These vectors have magnitude 4.3 m, 5.5 m and 6.0 m, respectively. The

vectors have direction 45◦, 68◦and 90◦respectively.

(b) ∆~

r= (−3.0 m)ˆ

i+ (3.0 m)ˆ

j, which has magnitude 4.3 m and direction 135◦.

(d) The velocity is given by ~

v=Rω cos ωtˆ

i+Rω sin ωtˆ

j. At t= 5.0 s ~

v= (0.94 m/s)ˆ

j; at t= 10 s

v= (−0.94 m/s)ˆ

(e) The acceleration is given by ~

a=−Rω2sin ωtˆ

i+Rω2cos ωtˆ

j. At t= 5.0 s ~

a= (−0.30 m/s2)ˆ

at t= 10 s ~

a= (−0.30 m/s2)ˆ

P4-21 Start from where the stone lands; in order to get there the stone fell through a vertical

distance of 1.9 m while moving 11 m horizontally. Then

y=−1

2gt2which can be written as t=r−2y

Putting in the numbers, t= 0.62 s is the time of ﬂight from the moment the string breaks. From

this time ﬁnd the horizontal velocity,

vx=x

t=(11 m)

(0.62 s) = 18 m/s.

Then the centripetal acceleration is

ac=v2

r=(18 m/s)2

(1.4 m) = 230 m/s2.

P4-22 (a) The path traced out by her feet has circumference c1= 2πr cos 50◦, where ris the

radius of the earth; the path traced out by her head has circumference c2= 2π(r+h) cos 50◦, where

his her height. The diﬀerence is ∆c= 2πh cos 50◦= 2π(1.6 m) cos 50◦= 6.46 m.

(b) a=v2/r = (2πr/T )2/r = 4π2r/T 2. Then ∆a= 4π2∆r/T 2. Note that ∆r=hcos θ! Then

∆a= 4π2(1.6 m) cos 50◦/(86400 s)2= 5.44×10−9m/s2.

P4-23 (a) A cycloid looks something like this:

(b) The position of the particle is given by

r= (Rsin ωt +ωRt)ˆ

i+ (Rcos ωt +R)ˆ

The maximum value of yoccurs whenever cos ωt = 1. The minimum value of yoccurs whenever

cos ωt =−1. At either of those times sin ωt = 0.

The velocity is the derivative of the displacement vector,

v= (Rω cos ωt +ωR)ˆ

i+ (−Rω sin ωt)ˆ

When yis a maximum the velocity simpliﬁes to

v= (2ωR)ˆ

i+ (0)ˆ

When yis a minimum the velocity simpliﬁes to

v= (0)ˆ

i+ (0)ˆ

The acceleration is the derivative of the velocity vector,

a= (−Rω2sin ωt)ˆ

i+ (−Rω2cos ωt)ˆ

When yis a maximum the acceleration simpliﬁes to

a= (0)ˆ

i+ (−Rω2)ˆ

When yis a minimum the acceleration simpliﬁes to

a= (0)ˆ

i+ (Rω2)ˆ

P4-24 (a) The speed of the car is vc= 15.3 m/s. The snow appears to fall with an angle θ=

arctan(15.3/7.8) = 63◦.

(b) The apparent speed is p(15.3)2+ (7.8)2(m/s) = 17.2 m/s.

P4-25 (a) The decimal angles are 89.994250◦and 89.994278◦. The earth moves in the orbit

around the sun with a speed of v= 2.98×104m/s (Appendix C). The speed of light is then between

c= (2.98×104m/s) tan(89.994250◦)=2.97×108m/s and c= (2.98 ×104m/s) tan(89.994278◦) =

2.98×108m/s. This method is highly sensitive to rounding. Calculating the orbital speed from the

radius and period of the Earth’s orbit will likely result in diﬀerent answers!

P4-26 (a) Total distance is 2l, so t0= 2l/v.

(b) Assume wind blows east. Time to travel out is t1=l/(v+u), time to travel back is

t2=l/(v−u). Total time is sum, or

tE=l

v+u+l

v−u=2lv

v2−u2=t0

1−u2/v2.

If wind blows west the times reverse, but the result is otherwise the same.

it will need to ﬂy with a heading away from east. The speed of the plane relative to the ground will

be √v2−u2. This will be the speed even when it ﬂies west, so

tN=2l

√v2−u2=t0

p1−u2/v2.

(d) If u>vthe wind sweeps the plane along in one general direction only; it can never ﬂy back.

Sort of like a black hole event horizon.

P4-27 The velocity of the police car with respect to the ground is ~

vpg =−76km/hˆ

i. The velocity

of the motorist with respect the ground is ~

vmg =−62 km/hˆ

The velocity of the motorist with respect to the police car is given by solving

vmg =~

vmp +~

vpg,

so ~

vmp = 76km/hˆ

i−62 km/hˆ

j. This velocity has magnitude

vmp =p(76km/h)2+ (−62 km/h)2= 98 km/h.

The direction is

θ= arctan(−62 km/h)/(76km/h) = −39◦,

but that is relative to ˆ

i. We want to know the direction relative to the line of sight. The line of sight

α= arctan(57 m)/(41 m) = −54◦

relative to ˆ

i, so the answer must be 15◦.

P4-28 (a) The velocity of the plane with respect to the air is ~

vpa; the velocity of the air with

respect to the ground is ~

vag, the velocity of the plane with respect to the ground is ~

vpg. Then

vpg =~

vpa +~

vag. This can be represented by a triangle; since the sides are given we can ﬁnd the

angle between ~

vag and ~

vpg (points north) using the cosine law

θ= arccos (135)2−(135)2−(70)2

−2(135)(70) = 75◦.

(b) The direction of ~

vpa can also be found using the cosine law,

θ= arccos (70)2−(135)2−(135)2

−2(135)(135) = 30◦.

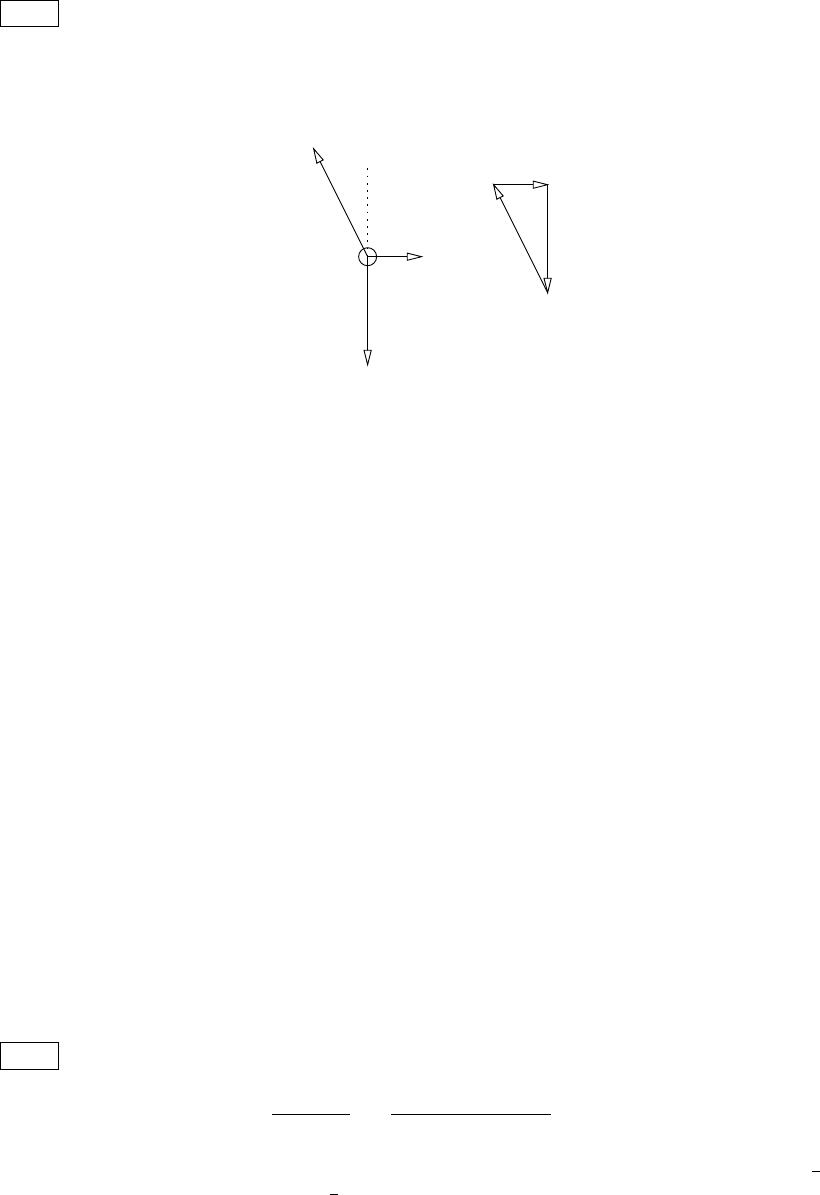
E5-1 There are three forces which act on the charged sphere— an electric force, FE, the force of

gravity, W, and the tension in the string, T. All arranged as shown in the ﬁgure on the right below.

(a) Write the vectors so that they geometrically show that the sum is zero, as in the ﬁgure

on the left below. Now W=mg = (2.8×10−4kg)(9.8 m/s2)=2.7×10−3N. The magnitude

of the electric force can be found from the tangent relationship, so FE=Wtan θ= (2.7×10−3

N) tan(33◦) = 1.8×10−3N.

(a)

T F Eθ

(b)

(b) The tension can be found from the cosine relation, so

T=W/ cos θ= (2.7×10−3N)/cos(33◦) = 3.2×10−3N.

E5-2 (a) The net force on the elevator is F=ma =W a/g = (6200 lb)(3.8 ft/s2)/(32 ft/s2) =

740 lb. Positive means up. There are two force on the elevator: a weight Wdown and a tension

from the cable Tup. Then F=T−Wor T=F+W= (740 lb) + (6200 lb) = 6940 lb.

(b) If the elevator acceleration is down then F=−740 lb; consequently T=F+W= (−740 lb)+

(6200 lb) = 5460 lb.

E5-3 (a) The tension Tis up, the weight Wis down, and the net force Fis in the direction of the

acceleration (up). Then F=T−W. But F=ma and W=mg, so

m=T/(a+g) = (89 N)/[(2.4 m/s2) + (9.8 m/s2)] = 7.3 kg.

(b) T= 89 N. The direction of velocity is unimportant. In both (a) and (b) the acceleration is

up.

E5-4 The average speed of the elevator during deceleration is vav = 6.0 m/s. The time to stop the

elevator is then t= (42.0 m)/(6.0 m/s) = 7.0 s. The deceleration is then a= (12.0 m/s)/(7.0 s) =

1.7 m/s2. Since the elevator is moving downward but slowing down, then the acceleration is up,

which will be positive.

The net force on the elevator is F=ma; this is equal to the tension Tminus the weight W.

Then

T=F+W=ma +mg = (1600 kg)[(1.7 m/s2) + (9.8 m/s2)] = 1.8×104N.

E5-5 (a) The magnitude of the man’s acceleration is given by

a=m2−m1

m2+m1

g=(110 kg) −(74 kg)

(110 kg) + (74 kg) g= 0.2g,

and is directed down. The time which elapses while he falls is found by solving y=v0yt+1

2ayt2,

or, with numbers, (−12 m) = (0)t+1

2(−0.2g)t2which has the solutions t=±3.5 s. The velocity

with which he hits the ground is then v=v0y+ayt= (0) + (−0.2g)(3.5 s) = −6.9 m/s.

(b) Reducing the speed can be accomplished by reducing the acceleration. We can’t change Eq.

5-4 without also changing one of the assumptions that went into it. Since the man is hoping to

reduce the speed with which he hits the ground, it makes sense that he might want to climb up the

rope.

E5-6 (a) Although it might be the monkey which does the work, the upward force to lift him still

comes from the tension in the rope. The minimum tension to lift the log is T=Wl=mlg. The net

force on the monkey is T−Wm=mma. The acceleration of the monkey is then

a= (ml−mm)g/mm= [(15 kg) −(11 kg)](9.8 m/s2)/(11 kg) = 3.6 m/s2.

(b) Atwood’s machine!

a= (ml−mm)g/(ml+mm) = [(15 kg) −(11 kg)](9.8 m/s2)/[(15 kg) + (11 kg)] = 1.5 m/s.

T= 2mlmmg/(ml+mm) = 2(15 kg)(11 kg)(9.8 m/s2)/[(15 kg) + (11 kg)] = 120 N.

E5-7 The weight of each car has two components: a component parallel to the cables W|| =Wsin θ

and a component normal to the cables W⊥.. The normal component is “balanced” by the supporting

cable. The parallel component acts with the pull cable.

In order to accelerate a car up the incline there must be a net force up with magnitude F=ma.

Then F=Tabove −Tbelow −W||, or

∆T=ma +mg sin θ= (2800 kg)[(0.81 m/s2) + (9.8 m/s2) sin(35◦)] = 1.8×104N.

E5-8 The tension in the cable is T, the weight of the man + platform system is W=mg, and the

net force on the man + platform system is F=ma =W a/g =T−W. Then

T=W a/g +W=W(a/g + 1) = (180 lb + 43 lb)[(1.2 ft/s2)/(32 ft/s2) + 1] = 231 lb.

E5-9 See Sample Problem 5-8. We need only apply the (unlabeled!) equation

µs= tan θ

to ﬁnd the egg angle. In this case θ= tan−1(0.04) = 2.3◦.

E5-10 (a) The maximum force of friction is F=µsN. If the rear wheels support half of the weight

of the automobile then N=W/2 = mg/2. The maximum acceleration is then

a=F/m =µsN/m =µsg/2.

(b) a= (0.56)(9.8 m/s2)/2 = 2.7 m/s2.

E5-11 The maximum force of friction is F=µsN. Since there is no motion in the ydirection the

magnitude of the normal force must equal the weight, N=W=mg. The maximum acceleration is

then

a=F/m =µsN/m =µsg= (0.95)(9.8 m/s2) = 9.3 m/s2.

E5-12 There is no motion in the vertical direction, so N=W=mg. Then µk=F/N =

(470 N)/[(9.8 m/s2)(79 kg)] = 0.61.

E5-13 A 75 kg mass has a weight of W= (75 kg)(9.8 m/s2) = 735 N, so the force of friction on

each end of the bar must be 368 N. Then

F≥fs

µs

=(368 N)

(0.41) = 900 N.

E5-14 (a) There is no motion in the vertical direction, so N=W=mg.

To get the box moving you must overcome static friction and push with a force of P≥µsN=

(0.41)(240 N) = 98 N.

(b) To keep the box moving at constant speed you must push with a force equal to the kinetic

friction, P=µkN= (0.32)(240 N) = 77 N.

the net force on the box is 21 N. The box will accelerate at

a=F/m =F g/W = (21 N)(9.8 m/s2)/(240 N) = 0.86 m/s2.

E5-15 (a) The maximum braking force is F=µsN. There is no motion in the vertical direction,

so N=W=mg. Then F=µsmg = (0.62)(1500 kg)(9.8 m/s2) = 9100 N.

(b) Although we still use F=µsN,N6=Won an incline! The weight has two components;

one which is parallel to the surface and the other which is perpendicular. Since there is no motion

perpendicular to the surface we must have N=W⊥=Wcos θ. Then

F=µsmg cos θ= (0.62)(1500 kg)(9.8 m/s2) cos(8.6◦) = 9000 N.

E5-16 µs= tan θis the condition for an object to sit without slipping on an incline. Then

θ= arctan(0.55) = 29◦. The angle should be reduced by 13◦.

E5-17 (a) The force of static friction is less than µsN, where Nis the normal force. Since the

crate isn’t moving up or down, PFy=0=N−W. So in this case N=W=mg = (136 kg)(9.81

m/s2) = 1330 N. The force of static friction is less than or equal to (0.37)(1330 N) = 492 N; moving

the crate will require a force greater than or equal to 492 N.

(b) The second worker could lift upward with a force L, reducing the normal force, and hence

reducing the force of friction. If the ﬁrst worker can move the block with a 412 N force, then

412 ≥µsN. Solving for N, the normal force needs to be less than 1110 N. The crate doesn’t move

oﬀ the table, so then N+L=W, or L=W−N= (1330 N) −(1110 N) = 220 N.

equal to 492 N. If the ﬁrst worker pushes with a force of 412 N, the second would need to push with

a force of 80 N.

E5-18 The coeﬃcient of static friction is µs= tan(28.0◦) = 0.532. The acceleration is a=

2(2.53 m)/(3.92 s)2=.329 m/s2. We will need to insert a negative sign since this is downward.

The weight has two components: a component parallel to the plane, W|| =mg sin θ; and a

component perpendicular to the plane, W⊥=mg cos θ. There is no motion perpendicular to the

plane, so N=W⊥. The kinetic friction is then f=µkN=µkmg cos θ. The net force parallel to

the plane is F=ma =f−W|| =µkmg cos θ−mg sin θ. Solving this for µk,

µk= (a+gsin θ)/(gcos θ),

= [(−0.329 m/s2) + (9.81 m/s2) sin(28.0◦)]/[((9.81 m/s2) cos(28.0◦)] = 0.494.

E5-19 The acceleration is a=−2d/t2, where d= 203 m is the distance down the slope and tis

the time to make the run.

The weight has two components: a component parallel to the incline, W|| =mg sin θ; and a

component perpendicular to the incline, W⊥=mg cos θ. There is no motion perpendicular tot he

plane, so N=W⊥. The kinetic friction is then f=µkN=µkmg cos θ. The net force parallel to

the plane is F=ma =f−W|| =µkmg cos θ−mg sin θ. Solving this for µk,

µk= (a+gsin θ)/(gcos θ),

= (gsin θ−2d/t2)/(gcos θ).

If t= 61 s, then

µk=(9.81 m/s2) sin(3.0◦)−2(203 m)/(61 s)2

(9.81 m/s2) cos(3.0◦)= 0.041;

if t= 42 s, then

µk=(9.81 m/s2) sin(3.0◦)−2(203 m)/(42 s)2

(9.81 m/s2) cos(3.0◦)= 0.029.

E5-20 (a) If the block slides down with constant velocity then a= 0 and µk= tan θ. Not only

that, but the force of kinetic friction must be equal to the parallel component of the weight, f=W||.

If the block is projected up the ramp then the net force is now 2W|| = 2mg sin θ. The deceleration

is a= 2gsin θ; the block will travel a time t=v0/a before stopping, and travel a distance

d=−at2/2 + v0t=−a(v0/a)2/2 + v0(v0/a) = v2

0/(2a) = v2

0/(4gsin θ)

before stopping.

(b) Since µk< µs, the incline is not steep enough to get the block moving again once it stops.

E5-21 Let a1be acceleration down frictionless incline of length l, and t1the time taken. The a2

is acceleration down “rough” incline, and t2= 2t1is the time taken. Then

l=1

2a1t2

1and l=1

2a2(2t1)2.

Equate and ﬁnd a1/a2= 4.

There are two force which act on the ice when it sits on the frictionless incline. The normal force

acts perpendicular to the surface, so it doesn’t contribute any components parallel to the surface.

The force of gravity has a component parallel to the surface, given by

W|| =mg sin θ,

and a component perpendicular to the surface given by

W⊥=mg cos θ.

The acceleration down the frictionless ramp is then

a1=W||

m=gsin θ.

When friction is present the force of kinetic friction is fk=µkN; since the ice doesn’t move

perpendicular to the surface we also have N=W⊥; and ﬁnally the acceleration down the ramp is

a2=W|| −fk

m=g(sin θ−µcos θ).

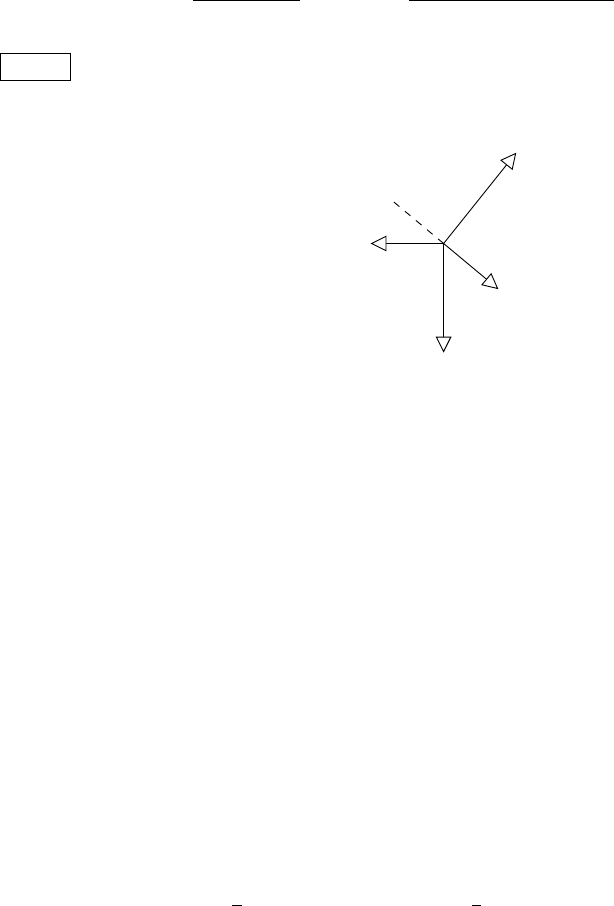
Previously we found the ratio of a1/a2, so we now have

sin θ= 4 sin θ−4µcos θ,

sin 33◦= 4 sin 33◦−4µcos 33◦,

µ= 0.49.

E5-22 (a) The static friction between Aand the table must be equal to the weight of block Bto

keep Afrom sliding. This means mBg=µs(mA+mC)g, or mc=mB/µs−mA= (2.6 kg)/(0.18) −

(4.4 kg) = 10 kg.

(b) There is no up/down motion for block A, so f=µkN=µkmAg. The net force on the system

containing blocks Aand Bis F=WB−f=mBg−µkmAg; the acceleration of this system is then

a=gmB−µkmA

mA+mB

= (9.m/s2)(2.6 kg) −(0.15)(4.4 kg)

(2.6 kg) + (4.4 kg) = 2.7 m/s2.

E5-23 There are four forces on the block— the force of gravity, W=mg; the normal force,

N; the horizontal push, P, and the force of friction, f. Choose the coordinate system so that

components are either parallel (x-axis) to the plane or perpendicular (y-axis) to it. θ= 39◦. Refer

to the ﬁgure below.

The magnitudes of the xcomponents of the forces are Wx=Wsin θ,Px=Pcos θand f; the

magnitudes of the ycomponents of the forces are Wy=Wcos θ,Py=Psin θ.

(a) We consider the ﬁrst the case of the block moving up the ramp; then fis directed down.

Newton’s second law for each set of components then reads as

XFx=Px−f−Wx=Pcos θ−f−Wsin θ=max,

XFy=N−Py−Wy=N−Psin θ−Wcos θ=may

Then the second equation is easy to solve for N

N=Psin θ+Wcos θ= (46 N) sin(39◦) + (4.8 kg)(9.8 m/s2) cos(39◦) = 66 N.

The force of friction is found from f=µkN= (0.33)(66 N) = 22 N. This is directed down the incline

while the block is moving up. We can now ﬁnd the acceleration in the xdirection.

max=Pcos θ−f−Wsin θ,

= (46 N) cos(39◦)−(22 N) −(4.8 kg)(9.8 m/s2) sin(39◦) = −16 N.

So the block is slowing down, with an acceleration of magnitude 3.3 m/s2.

(b) The block has an initial speed of v0x= 4.3 m/s; it will rise until it stops; so we can use

vy= 0 = v0y+aytto ﬁnd the time to the highest point. Then t= (vy−v0y)/ay=−(−4.3 m/s)/(3.3

m/s2= 1.3 s. Now that we know the time we can use the other kinematic relation to ﬁnd the

distance

y=v0yt+1

2ayt2= (4.3 m/s)(1.3 s) + 1

2(−3.3 m/s2)(1.3 s)2= 2.8 m

force, which is now directed up the ramp, must be suﬃciently small so that f+Px≤Wx. Solving

for fwe ﬁnd f≤Wx−Pxor, using our numbers from above, f≤ −6 N. Is this possible? No, so

the block will not slide back down the ramp, even if the ramp were frictionless, while the horizontal

force is applied.

E5-24 (a) The horizontal force needs to overcome the maximum static friction, so P≥µsN=

µsmg = (0.52)(12 kg)(9.8 m/s2) = 61 N.

(b) If the force acts upward from the horizontal then there are two components: a horizontal

component Px=Pcos θand a vertical component Py=Psin θ. The normal force is now given by

W=Py+N; consequently the maximum force of static friction is now µsN=µs(mg −Psin θ).

The block will move only if Pcos θ≥µs(mg −Psin θ), or

P≥µsmg

cos θ+µssin θ=(0.52)(12 kg)(9.8 m/s2)

cos(62◦) + (0.52) sin(62◦)= 66 N.

P≥µsmg

cos θ+µssin θ=(0.52)(12 kg)(9.8 m/s2)

cos(−62◦) + (0.52) sin(−62◦)= 5900 N.

E5-25 (a) If the tension acts upward from the horizontal then there are two components: a hori-

zontal component Tx=Tcos θand a vertical component Ty=Tsin θ. The normal force is now given

by W=Ty+N; consequently the maximum force of static friction is now µsN=µs(W−Tsin θ).

The crate will move only if Tcos θ≥µs(W−Tsin θ), or

P≥µsW

cos θ+µssin θ=(0.52)(150 lb)

cos(17◦) + (0.52) sin(17◦)= 70 lb.

(b) Once the crate starts to move then the net force on the crate is F=Tx−f. The acceleration

is then

a=g

W[Tcos θ−µk(W−Tsin θ)],

=(32 ft/s2)

(150 lb) {(70 lb) cos(17◦)−(0.35)[(150 lb) −(70 lb) sin(17◦)]},

= 4.6 ft/s2.

E5-26 If the tension acts upward from the horizontal then there are two components: a horizontal

component Tx=Tcos θand a vertical component Ty=Tsin θ. The normal force is now given by

W=Ty+N; consequently the maximum force of static friction is now µsN=µs(W−Tsin θ). The

crate will move only if Tcos θ≥µs(W−Tsin θ), or

W≤Tcos θ/µs+Tsin θ.

We want the maximum, so we ﬁnd dW/dθ,

dW/dθ =−(T/µs) sin θ+Tcos θ,

which equals zero when µs= tan θ. For this problem θ= arctan(0.35) = 19◦, so

W≤(1220 N) cos(19◦)/(0.35) + (1220 N) sin(19◦) = 3690 N.

E5-27 The three force on the know above Amust add to zero. Construct a vector diagram:

TA+~

TB+~

Td= 0, where ~

Tdrefers to the diagonal rope. TAand TBmust be related by TA=

TBtan θ, where θ= 41◦.

There is no up/down motion of block B, so N=WBand f=µsWB. Since block Bis at rest

f=TB. Since block Ais at rest WA=TA. Then

WA=WB(µstan θ) = (712 N)(0.25) tan(41◦) = 155 N.

E5-28 (a) Block 2 doesn’t move up/down, so N=W2=m2gand the force of friction on block 2

is f=µkm2g. Block 1 is on a frictionless incline; only the component of the weight parallel to the

surface contributes to the motion, and W|| =m1gsin θ. There are two relevant forces on the two

mass system. The eﬀective net force is the of magnitude W|| −f, so the acceleration is

a=gm1sin θ−µkm2

m1+m2

= (9.81 m/s2)(4.20 kg) sin(27◦)−(0.47)(2.30 kg)

(4.20 kg) + (2.30 kg) = 1.25 m/s2.

The blocks accelerate down the ramp.

(b) The net force on block 2 is F=m2a=T−f. The tension in the cable is then

T=m2a+µkm2g= (2.30 kg)[(1.25 m/s2) + (0.47)(9.81 m/s2)] = 13.5 N.

E5-29 This problem is similar to Sample Problem 5-7, except now there is friction which can act

on block B. The relevant equations are now for block B

N−mBgcos θ= 0

and

T−mBgsin θ±f=mBa,

where the sign in front of fdepends on the direction in which block Bis moving. If the block is

moving up the ramp then friction is directed down the ramp, and we would use the negative sign.

If the block is moving down the ramp then friction will be directed up the ramp, and then we will

use the positive sign. Finally, if the block is stationary then friction we be in such a direction as to

make a= 0.

For block Athe relevant equation is

mAg−T=mAa.

Combine the ﬁrst two equations with f=µN to get

T−mBgsin θ±µmBgcos θ=mBa.

Take some care when interpreting friction for the static case, since the static value of µyields the

maximum possible static friction force, which is not necessarily the actual static frictional force.

Combine this last equation with the block Aequation,

mAg−mAa−mBgsin θ±µmBgcos θ=mBa,

and then rearrange to get

a=gmA−mBsin θ±µmBcos θ

mA+mB

For convenience we will use metric units; then the masses are mA= 13.2 kg and mB= 42.6 kg. In

addition, sin 42◦= 0.669 and cos 42◦= 0.743.

(a) If the blocks are originally at rest then

mA−mBsin θ= (13.2 kg) −(42.6 kg)(0.669) = −15.3 kg

where the negative sign indicates that block Bwould slide downhill if there were no friction.

If the blocks are originally at rest we need to consider static friction, so the last term can be as

large as

µmBcos θ= (.56)(42.6 kg)(0.743) = 17.7 kg.

Since this quantity is larger than the ﬁrst static friction would be large enough to stop the blocks

from accelerating if they are at rest.

(b) If block Bis moving up the ramp we use the negative sign, and the acceleration is

a= (9.81 m/s2)(13.2 kg) −(42.6 kg)(0.669) −(.25)(42.6 kg)(0.743)

(13.2 kg) + (42.6 kg) =−4.08 m/s2.

where the negative sign means down the ramp. The block, originally moving up the ramp, will

slow down and stop. Once it stops the static friction takes over and the results of part (a) become

relevant.

a= (9.81 m/s2)(13.2 kg) −(42.6 kg)(0.669) + (.25)(42.6 kg)(0.743)

(13.2 kg) + (42.6 kg) =−1.30 m/s2.

where the negative sign means down the ramp. This means that if the block is moving down the

ramp it will continue to move down the ramp, faster and faster.

E5-30 The weight can be resolved into a component parallel to the incline, W|| =Wsin θand a

component that is perpendicular, W⊥=Wcos θ. There are two normal forces on the crate, one from

each side of the trough. By symmetry we expect them to have equal magnitudes; since they both

act perpendicular to their respective surfaces we expect them to be perpendicular to each other.

They must add to equal the perpendicular component of the weight. Since they are at right angles

and equal in magnitude, this yields N2+N2=W⊥2, or N=W⊥/√2.

Each surface contributes a frictional force f=µkN=µkW⊥/√2; the total frictional force is

then twice this, or √2µkW⊥. The net force on the crate is F=Wsin θ−√2µkWcos θdown the

ramp. The acceleration is then

a=g(sin θ−√2µkcos θ).

E5-31 The normal force between the top slab and the bottom slab is N=Wt=mtg. The force

of friction between the top and the bottom slab is f≤µN =µmtg. We don’t yet know if the slabs

slip relative to each other, so we don’t yet know what kind of friction to consider.

The acceleration of the top slab is

at= (110 N)/(9.7 kg) −µ(9.8 m/s2) = 11.3 m/s2−µ(9.8 m/s2).

The acceleration of the bottom slab is

ab=µ(9.8 m/s2)(9.7,kg)/(42 kg) = µ(2.3 m/s2).

Can these two be equal? Only if µ≥0.93. Since the static coeﬃcient is less than this, the block

slide. Then at= 7.6 m/s2and ab= 0.87 m/s2.

E5-32 (a) Convert the speed to ft/s: v= 88 ft/s. The acceleration is

a=v2/r = (88 ft/s)2/(25 ft) = 310 ft/s2.

(b) a= 310 ft/s2g/(32 ft/s2) = 9.7g.

E5-33 (a) The force required to keep the car in the turn is F=mv2/r =W v2/(rg), or

F= (10700 N)(13.4 m/s)2/[(61.0 m)(9.81 m/s2)] = 3210 N.

(b) The coeﬃcient of friction required is µs=F/W = (3210 N)/(10700 N) = 0.300.

E5-34 (a) The proper banking angle is given by

θ= arctan v2

Rg = arctan (16.7 m/s)2

(150 m)(9.8 m/s2)= 11◦.

(b) If the road is not banked then the force required to keep the car in the turn is F=mv2/r =

W v2/(Rg) and the required coeﬃcient of friction is

µs=F/W =v2

Rg =(16.7 m/s)2

(150 m)(9.8 m/s2)= 0.19.

E5-35 (a) This conical pendulum makes an angle θ= arcsin(0.25/1.4) = 10◦with the vertical.

The pebble has a speed of

v=pRg tan θ=p(0.25 m)(9.8 m/s2) tan(10◦) = 0.66 m/s.

(b) a=v2/r = (0.66 m/s)2/(0.25 m) = 1.7 m/s2.

E5-36 Ignoring air friction (there must be a forward component to the friction!), we have a nor-

mal force upward which is equal to the weight: N=mg = (85 kg)(9.8 m/s2) = 833 N. There

is a sideways component to the friction which is equal tot eh centripetal force, F=mv2/r =

(85 kg)(8.7 m/s)2/(25 m) = 257 N. The magnitude of the net force of the road on the person is

F=p(833 N)2+ (257 N)2= 870 N,

and the direction is θ= arctan(257/833) = 17◦oﬀ of vertical.

E5-37 (a) The speed is v= 2πrf = 2π(5.3×10−11 m)(6.6×1015/s) = 2.2×106m/s.

(b) The acceleration is a=v2/r = (2.2×106m/s)2/(5.3×10−11m) = 9.1×1022 m/s2.

E5-38 The basket has speed v= 2πr/t. The basket experiences a frictional force F=mv2/r =

m(2πr/t)2/r = 4π2mr/t2. The coeﬃcient of static friction is µs=F/N =F/W . Combining,

µs=4π2r

gt2=4π2(4.6 m)

(9.8 m/s2)(24 s)2= 0.032.

E5-39 There are two forces on the hanging cylinder: the force of the cord pulling up Tand the

force of gravity W=Mg. The cylinder is at rest, so these two forces must balance, or T=W.

There are three forces on the disk, but only the force of the cord on the disk Tis relevant here, since

there is no friction or vertical motion.

The disk undergoes circular motion, so T=mv2/r. We want to solve this for vand then express

the answer in terms of m,M,r, and G.

v=rT r

m=rMgr

E5-40 (a) The frictional force stopping the car is F=µsN=µsmg. The deceleration of the car

is then a=µsg. If the car is moving at v= 13.3 m/s then the average speed while decelerating

is half this, or vav = 6.7 m/s. The time required to stop is t=x/vav = (21 m)/(6.7 m/s) = 3.1 s.

The deceleration is a= (13.3 m/s)/(3.1 s) = 4.3 m/s2. The coeﬃcient of friction is µs=a/g =

(4.3 m/s2)/(9.8 m/s2) = 0.44.

(b) The acceleration is the same as in part (a), so r=v2/a = (13.3 m/s)2/(4.3 m/s2) = 41 m.

E5-41 There are three forces to consider: the normal force of the road on the car N; the force of

gravity on the car W; and the frictional force on the car f. The acceleration of the car in circular

motion is toward the center of the circle; this means the net force on the car is horizontal, toward

the center. We will arrange our coordinate system so that ris horizontal and zis vertical. Then the

components of the normal force are Nr=Nsin θand Nz=Ncos θ; the components of the frictional

force are fr=fcos θand fz=fsin θ.

The direction of the friction depends on the speed of the car. The ﬁgure below shows the two

force diagrams.

The turn is designed for 95 km/hr, at this speed a car should require no friction to stay on the

road. Using Eq. 5-17 we ﬁnd that the banking angle is given by

tan θb=v2

rg =(26 m/s)2

(210 m)(9.8 m/s2)= 0.33,

for a bank angle of θb= 18◦.

(a) On the rainy day traﬃc is moving at 14 m/s. This is slower than the rated speed, so any

frictional force must be directed up the incline. Newton’s second law is then

XFr=Nr−fr=Nsin θ−fcos θ=mv2

XFz=Nz+fz−W=Ncos θ+fsin θ−mg = 0.

We can substitute f=µsNto ﬁnd the minimum value of µswhich will keep the cars from slipping.

There will then be two equations and two unknowns, µsand N. Solving for N,

N(sin θ−µscos θ) = mv2

rand N(cos θ+µssin θ) = mg.

Combining,

(sin θ−µscos θ)mg = (cos θ+µssin θ)mv2

Rearrange,

µs=gr sin θ−v2cos θ

gr cos θ+v2sin θ.

We know all the numbers. Put them in and we’ll ﬁnd µs= 0.22

(b) Now the frictional force will point the other way, so Newton’s second law is now

XFr=Nr+fr=Nsin θ+fcos θ=mv2

XFz=Nz−fz−W=Ncos θ−fsin θ−mg = 0.

The bottom equation can be rearranged to show that

N=mg

cos θ−µssin θ.

This can be combined with the top equation to give

mg sin θ+µscos θ

cos θ−µssin θ=mv2

We can solve this ﬁnal expression for vusing all our previous numbers and get v= 35 m/s. That’s

about 130 km/hr.

E5-42 (a) The net force on the person at the top of the Ferris wheel is mv2/r =W−Nt, pointing

down. The net force on the bottom is still mv2/r, but this quantity now equals Nb−Wand is point

up. Then Nb= 2W−Nt= 2(150 lb) −(125 lb) = 175 lb.

(b) Doubling the speed would quadruple the net force, so the new scale reading at the top would

be (150 lb) −4[(150 lb) −(125 lb)] = 50 lb. Wee!

E5-43 The net force on the object when it is not sliding is F=mv2/r; the speed of the object is

v= 2πrf (fis rotational frequency here), so F= 4π2mrf2. The coeﬃcient of friction is then at

least µs=F/W = 4π2rf2/g. If the object stays put when the table rotates at 33 1

3rev/min then

µs≥4π2(0.13 m)(33.3/60 /s)2/(9.8 m/s2) = 0.16.

If the object slips when the table rotates at 45.0 rev/min then

µs≤4π2(0.13 m)(45.0/60 /s)2/(9.8 m/s2) = 0.30.

E5-44 This is eﬀectively a banked highway problem if the pilot is ﬂying correctly.

r=v2

gtan θ=(134 m/s)2

(9.8 m/s2) tan(38.2◦)= 2330 m.

E5-45 (a) Assume that frigate bird ﬂies as well as a pilot. Then this is a banked highway problem.

The speed of the bird is given by v2=gr tan θ. But there is also vt = 2πr, so 2πv2=gvt tan θ, or

v=gt tan θ

2π=(9.8 m/s2)(13 s) tan(25◦)

2π= 9.5 m/s.

(b) r=vt/(2π) = (9.5 m/s)(13 s)/(2π) = 20 m.

E5-46 (a) The radius of the turn is r=p(33 m)2−(18 m)2= 28 m. The speed of the plane is v=

2πrf = 2π(28 m)(4.4/60 /s) = 13 m/s. The acceleration is a=v2/r = (13 m/s)2/(28 m) = 6.0m/s2.

(b) The tension has two components: Tx=Tcos θand Ty=Tsin θ. In this case θ=

arcsin(18/33) = 33◦. All of the centripetal force is provided for by Tx, so

T= (0.75 kg)(6.0 m/s2)/cos(33◦) = 5.4 N.

Ty+W= (5.4 N) sin(33◦) + (0.75 kg)(9.8 m/s2) = 10 N.

E5-47 (a) The acceleration is a=v2/r = 4π2r/t2= 4π2(6.37×106m)/(8.64×104s)2= 3.37 ×

10−2m/s2. The centripetal force on the standard kilogram is F=ma = (1.00 kg)(3.37×10−2m/s2) =

0.0337 N.

(b) The tension in the balance would be T=W−F= (9.80 N) −(0.0337 N) = 9.77 N.

E5-48 (a) v= 4(0.179 m/s4)(7.18 s)3−2(2.08 m/s2)(7.18 s) = 235 m/s.

(b) a= 12(0.179 m/s4)(7.18 s)2−2(2.08 m/s2) = 107 m/s2.

E5-49 The force only has an xcomponent, so we can use Eq. 5-19 to ﬁnd the velocity.

vx=v0x+1

mZt

Fxdt =v0+F0

mZt

(1 −t/T )dt

Integrating,

vx=v0+a0t−1

2Tt2

Now put this expression into Eq. 5-20 to ﬁnd the position as a function of time

x=x0+Zt

vxdt =Zt

0v0x+a0t−1

2Tt2 dt

Integrating,

x=v0T+a01

2T2−1

6TT3=v0T+a0

Now we can put t=Tinto the expression for v.

vx=v0+a0T−1

2TT2=v0+a0T/2.

P5-1 (a) There are two forces which accelerate block 1: the tension, T, and the parallel component

of the weight, W||,1=m1gsin θ1. Assuming the block accelerates to the right,

m1a=m1gsin θ1−T.

There are two forces which accelerate block 2: the tension, T, and the parallel component of the

weight, W||,2=m2gsin θ2. Assuming the block 1 accelerates to the right, block 2 must also accelerate

to the right, and

m2a=T−m2gsin θ2.

Add these two equations,

(m1+m2)a=m1gsin θ1−m2gsin θ2,

and then rearrange:

a=m1gsin θ1−m2gsin θ2

m1+m2

Or, take the two net force equations, divide each side by the mass, and set them equal to each other:

gsin θ1−T/m1=T/m2−gsin θ2.

Rearrange,

T1

m2=gsin θ1+gsin θ2,

and then rearrange again:

T=m1m2g

m1+m2

(sin θ1+ sin θ2).

(b) The negative sign we get in the answer means that block 1 accelerates up the ramp.

a=(3.70 kg) sin(28◦)−(4.86 kg) sin(42◦)

(3.70 kg) + (4.86 kg) (9.81 m/s2) = −1.74 m/s2.

T=(3.70 kg)(4.86 kg)(9.81 m/s2)

(3.70 kg) + (4.86 kg) [sin(28◦) + sin(42◦)] = 23.5 N.

massive than this m1will accelerate up the plane; if m2is less massive than this m1will accelerate

down the plane.

P5-2 (a) Since the pulley is massless, F= 2T. The largest value of Tthat will allow block 2 to

remain on the ﬂoor is T≤W2=m2g. So F≤2(1.9 kg)(9.8 m/s2) = 37 N.

(b) T=F/2 = (110 N)/2 = 55 N.

an acceleration of a= (43 N)/(1.2 kg) = 36 m/s2.

P5-3 As the string is pulled the two masses will move together so that the conﬁguration will look

like the ﬁgure below. The point where the force is applied to the string is massless, so PF= 0 at

that point. We can take advantage of this fact and the ﬁgure below to ﬁnd the tension in the cords,

F/2 = Tcos θ. The factor of 1/2 occurs because only 1/2 of Fis contained in the right triangle that

has Tas the hypotenuse. From the ﬁgure we can ﬁnd the xcomponent of the force on one mass to

be Tx=Tsin θ. Combining,

Tx=F

sin θ

cos θ=F

2tan θ.

But the tangent is equal to

tan θ=Opposite

Adjacent =x

√L2−x2

And now we have the answer in the book.

θT

What happens when x=L? Well, axis inﬁnite according to this expression. Since that could

only happen if the tension in the string were inﬁnite, then there must be some other physics that

we had previously ignored.

P5-4 (a) The force of static friction can be as large as f≤µsN= (0.60)(12 lb) = 7.2 lb. That is

more than enough to hold the block up.

(b) The force of static friction is actually only large enough to hold up the block: f= 5.0 lb.

The magnitude of the force of the wall on the block is then Fbw =p(5.0)2+ (12.0)2lb = 13 lb.

P5-5 (a) The weight has two components: normal to the incline, W⊥=mg cos θand parallel to the

incline, W|| =mg sin θ. There is no motion perpendicular to the incline, so N=W⊥=mg cos θ. The

force of friction on the block is then f=µN =µmg cos θ, where we use whichever µis appropriate.

The net force on the block is F−f−W|| =F±µmg cos θ−mg sin θ.

To hold the block in place we use µsand friction will point up the ramp so the ±is +, and

F= (7.96 kg)(9.81 m/s2)[sin(22.0◦)−(0.25) cos(22.0◦)] = 11.2 N.

(b) To ﬁnd the minimum force to begin sliding the block up the ramp we still have static friction,

but now friction points down, so

F= (7.96 kg)(9.81 m/s2)[sin(22.0◦) + (0.25) cos(22.0◦)] = 47.4 N.

F= (7.96 kg)(9.81 m/s2)[sin(22.0◦) + (0.15) cos(22.0◦)] = 40.1 N.

P5-6 The sand will slide if the cone makes an angle greater than θwhere µs= tan θ. But

tan θ=h/R or h=Rtan θ. The volume of the cone is then

Ah/3 = πR2h/3 = πR3tan θ/3 = πµsR3/3.

P5-7 There are four forces on the broom: the force of gravity W=mg; the normal force of the

ﬂoor N; the force of friction f; and the applied force from the person P(the book calls it F). Then

XFx=Px−f=Psin θ−f=max,

XFy=N−Py−W=N−Pcos θ−mg =may= 0

Solve the second equation for N,

N=Pcos θ+mg.

(a) If the mop slides at constant speed f=µkN. Then

Psin θ−f=Psin θ−µk(Pcos θ+mg) = 0.

We can solve this for P(which was called Fin the book);

P=µmg

sin θ−µkcos θ.

This is the force required to push the broom at constant speed.

(b) Note that Pbecomes negative (or inﬁnite) if sin θ≤µkcos θ. This occurs when tan θc≤µk.

If this happens the mop stops moving, to get it started again you must overcome the static friction,

but this is impossible if tan θ0≤µs

P5-8 (a) The condition to slide is µs≤tan θ. In this case, (0.63) >tan(24◦) = 0.445.

(b) The normal force on the slab is N=W⊥=mg cos θ. There are three forces parallel to the

surface: friction, f=µsN=µsmg cos θ; the parallel component of the weight, W|| =mg sin θ, and

the force F. The block will slide if these don’t balance, or

F > µsmg cos θ−mg sin θ= (1.8×107kg)(9.8 m/s2)[(0.63) cos(24◦)−sin(24◦)] = 3.0×107N.

P5-9 To hold up the smaller block the frictional force between the larger block and smaller block

must be as large as the weight of the smaller block. This can be written as f=mg. The normal

force of the larger block on the smaller block is N, and the frictional force is given by f≤µsN. So

the smaller block won’t fall if mg ≤µsN.

There is only one horizontal force on the large block, which is the normal force of the small block

on the large block. Newton’s third law says this force has a magnitude N, so the acceleration of the

large block is N=Ma.

There is only one horizontal force on the two block system, the force F. So the acceleration of

this system is given by F= (M+m)a. The two accelerations are equal, otherwise the blocks won’t

stick together. Equating, then, gives N/M =F/(M+m).

We can combine this last expression with mg ≤µsNand get

mg ≤µsFM

M+m

F≥g(M+m)m

µsM=(9.81 m/s2)(88 kg + 16 kg)(16 kg)

(0.38)(88 kg) = 490 N

P5-10 The normal force on the ith block is Ni=migcos θ; the force of friction on the ith block

is then fi=µimigcos θ. The parallel component of the weight on the ith block is W||,i =migsin θ.

(a) The net force on the system is

F=X

mig(sin θ−µicos θ).

Then

a= (9.81 m/s2)(1.65 kg)(sin 29.5◦−0.226 cos 29.5◦) + (3.22 kg)(sin 29.5◦−0.127 cos 29.5◦)

(1.65 kg) + (3.22 kg) ,

= 3.46 m/s2.

(b) The net force on the lower mass is m2a=W||,2−f2−T, so the tension is

T= (9.81 m/s2)(3.22 kg)(sin 29.5◦−0.127 cos 29.5◦)−(3.22 kg)(3.46 m/s2) = 0.922 N.

of the masses can only result in a reversing of the tension: it is still 0.992 N, but is now negative,

meaning compression.

P5-11 The rope wraps around the dowel and there is a contribution to the frictional force ∆f

from each small segment of the rope where it touches the dowel. There is also a normal force ∆Nat

each point where the contact occurs. We can ﬁnd ∆Nmuch the same way that we solve the circular

motion problem.

In the ﬁgure on the left below we see that we can form a triangle with long side Tand short side

∆N. In the ﬁgure on the right below we see a triangle with long side rand short side r∆θ. These

triangles are similar, so r∆θ/r = ∆N/T .

r∆θ∆ N

Now ∆f=µ∆Nand T(θ) + ∆f≈T(θ+ ∆θ). Combining, and taking the limit as ∆θ→0,

dT =df

T=Zdθ

integrating both sides of this expression,

T=Zdθ,

µln T|T2

T1=π,

T2=T1eπµ.

In this case T1is the weight and T2is the downward force.

P5-12 Answer this out of order!

(b) The maximum static friction between the blocks is 12.0 N; the maximum acceleration of the

top block is then a=F/m = (12.0 N)/(4.40 kg) = 2.73 m/s2.

(a) The net force on a system of two blocks that will accelerate them at 2.73 m/s2is F=

(4.40 kg + 5.50 kg)(2.73 m/s2) = 27.0 N.

P5-13 The speed is v= 23.6 m/s.

(a) The average speed while stopping is half the initial speed, or vav = 11.8 m/s. The time to stop

is t= (62 m)/(11.8 m/s) = 5.25 s. The rate of deceleration is a= (23.6 m/s)/(5.25 s) = 4.50 m/s2.

The stopping force is F=ma; this is related to the frictional force by F=µsmg, so µs=a/g =

(4.50 m/s2)/(9.81 m/s2) = 0.46.

(b) Turning,

a=v2/r = (23.6 m/s)2/(62 m) = 8.98 m/s2.

Then µs=a/g = (8.98 m/s2)/(9.81 m/s2) = 0.92.

P5-14 (a) The net force on car as it travels at the top of a circular hill is Fnet =mv2/r =W−N;

in this case we are told N=W/2, so Fnet =W/2 = (16000 N)/2 = 8000 N. When the car travels

through the bottom valley the net force at the bottom is Fnet =mv2/r =N−W. Since the

magnitude of v,r, and hence Fnet is the same in both cases,

N=W/2 + W= 3W/2 = 3(16000 N)/2 = 24000 N

at the bottom of the valley.

(b) You leave the hill when N= 0, or

v=√rg =p(250 m)(9.81 m/s2) = 50 m/s.

P5-15 (a) v= 2πr/t = 2π(0.052 m)(3/3.3 s) = 0.30 m/s.

(b) a=v2/r = (0.30 m/s)2/(0.052 m) = 1.7 m/s2, toward center.

(d) If the coin can be as far away as rbefore slipping, then

µs=F/mg = (2πr/t)2/(rg) = 4π2r/(t2g) = 4π2(0.12 m)/[(3/3.3 s)2(9.8 m/s2)] = 0.59.

P5-16 (a) Whether you assume constant speed or constant energy, the tension in the string must

be the greatest at the bottom of the circle, so that’s where the string will break.

(b) The net force on the stone at the bottom is T−W=mv2/r. Then

v=prg[T /W −1] = q(2.9 ft)(32 ft/s2)[(9.2 lb)/(0.82 lb) −1] = 31 ft/s.

P5-17 (a) In order to keep the ball moving in a circle there must be a net centripetal force Fc

directed horizontally toward the rod. There are only three forces which act on the ball: the force

of gravity, W=mg = (1.34 kg)(9.81 m/s2) = 13.1 N; the tension in the top string T1= 35.0 N, and

the tension in the bottom string, T2.

The components of the force from the tension in the top string are

T1,x = (35.0 N) cos 30◦= 30.3 N and T1,y = (35.0 N) sin 30◦= 17.5 N.

The vertical components do balance, so

T1,y +T2,y =W,

or T2,y = (13.1 N) −(17.5 N) = −4.4 N. From this we can ﬁnd the tension in the bottom string,

T2=T2,y/sin(−30◦) = 8.8 N.

(b) The net force on the object will be the sum of the two horizontal components,

Fc= (30.3 N) + (8.8 N) cos 30◦= 37.9 N.

v=√acr=pFcr/m,

=p(37.9 m)(1.70 m) sin 60◦/(1.34 kg) = 6.45 m/s.

P5-18 The net force on the cube is F=mv2/r. The speed is 2πrω. (Note that we are using ωin

a non-standard way!) Then F= 4π2mrω2. There are three forces to consider: the normal force of

the funnel on the cube N; the force of gravity on the cube W; and the frictional force on the cube

f. The acceleration of the cube in circular motion is toward the center of the circle; this means the

net force on the cube is horizontal, toward the center. We will arrange our coordinate system so

that ris horizontal and zis vertical. Then the components of the normal force are Nr=Nsin θ

and Nz=Ncos θ; the components of the frictional force are fr=fcos θand fz=fsin θ.

The direction of the friction depends on the speed of the cube; it will point up if ωis small and

down if ωis large.

(a) If ωis small, Newton’s second law is

XFr=Nr−fr=Nsin θ−fcos θ= 4π2mrω2,

XFz=Nz+fz−W=Ncos θ+fsin θ−mg = 0.

We can substitute f=µsN. Solving for N,

N(cos θ+µssin θ) = mg.

Combining,

4π2rω2=gsin θ−µscos θ

cos θ+µssin θ.

Rearrange,

ω=1

2πsg

sin θ−µscos θ

cos θ+µssin θ.

This is the minimum value.

(b) Now the frictional force will point the other way, so Newton’s second law is now

XFr=Nr+fr=Nsin θ+fcos θ= 4π2mrω2,

XFz=Nz−fz−W=Ncos θ−fsin θ−mg = 0.

We’ve swapped + and - signs, so

ω=1

2πsg

sin θ+µscos θ

cos θ−µssin θ

is the maximum value.

P5-19 (a) The radial distance from the axis of rotation at a latitude Lis Rcos L. The speed

in the circle is then v= 2πR cos L/T . The net force on a hanging object is F=mv2/(Rcos L) =

4π2mR cos L/T 2. This net force is not directed toward the center of the earth, but is instead directed

toward the axis of rotation. It makes an angle Lwith the Earth’s vertical. The tension in the cable

must then have two components: one which is vertical (compared to the Earth) and the other which

is horizontal. If the cable makes an angle θwith the vertical, then T|| =Tsin θand T⊥=Tcos θ.

Then T|| =F|| and W−T⊥=F⊥. Written with a little more detail,

Tsin θ= 4π2mR cos Lsin L/T 2≈T θ,

and

Tcos θ= 4π2mR cos2L/T 2+mg ≈T.

But 4π2Rcos2L/T 2g, so it can be ignored in the last equation compared to g, and T≈mg.

Then from the ﬁrst equation,

θ= 2π2Rsin 2L/(gT 2).

(b) This is a maximum when sin 2Lis a maximum, which happens when L= 45◦. Then

θ= 2π2(6.37×106m)/[(9.8 m/s2)(86400 s)2] = 1.7×10−3rad.

P5-20 a= (F0/m)e−t/T . Then v=Rt

0a dt = (F0T/m)e−t/T , and x=Rt

0v dt = (F0T2/m)e−t/T .

(a) When t=T v = (F0T/m)e−1= 0.368(F0T/m).

(b) When t=T x = (F0T2/m)e−1= 0.368(F0T2/m).

E6-1 (a) v1= (m2/m1)v2= (2650 kg/816 kg)(16.0 km/h) = 52.0 km/h.

(b) v1= (m2/m1)v2= (9080 kg/816 kg)(16.0 km/h) = 178 km/h.

E6-2 ~

pi= (2000 kg)(40 km/h)ˆ

j= 8.00×104kg ·km/hˆ

j.~

pf= (2000 kg)(50 km/h)ˆ

i= 1.00×105kg ·

km/hˆ

i. ∆~

p=~

pf−~

pi= 1.00×105kg ·km/hˆ

i−8.00×104kg ·km/hˆ

j. ∆p=p(∆px)2+ (∆py)2=

1.28×105kg ·km/h. The direction is 38.7◦south of east.

E6-3 The ﬁgure below shows the initial and ﬁnal momentum vectors arranged to geometrically

show ~

pf−~

pi= ∆~

p. We can use the cosine law to ﬁnd the length of ∆~

-pi

∆p

The angle α= 42◦+ 42◦,pi=mv = (4.88 kg)(31.4 m/s) = 153 kg·m/s. Then the magnitude of

∆~

pis

∆p=p(153 kg·m/s)2+ (153 kg·m/s)2−2(153 kg·m/s)2cos(84◦) = 205 kg·m/s,

directed up from the plate. By symmetry it must be perpendicular.

E6-4 The change in momentum is ∆p=−mv =−(2300 kg)(15 m/s) = −3.5×104kg ·m/s. The

average force is F= ∆p/∆t= (−3.5×104kg ·m/s)/(0.54 s) = −6.5×104N.

E6-5 (a) The change in momentum is ∆p= (−mv)−mv; the average force is F= ∆p/∆t=

−2mv/∆t.

(b) F=−2(0.14 kg)(7.8 m/s)/(3.9×10−3s) = 560 N.

E6-6 (a) J= ∆p= (0.046 kg)(52.2 m/s) −0 = 2.4 N ·s.

(b) The impulse imparted to the club is opposite that imparted to the ball.

E6-7 Choose the coordinate system so that the ball is only moving along the xaxis, with away

from the batter as positive. Then pfx=mvfx= (0.150 kg)(61.5 m/s) = 9.23 kg·m/s and pix=

mvix= (0.150 kg)(−41.6 m/s) = −6.24 kg·m/s. The impulse is given by Jx=pfx−pix= 15.47

kg·m/s. We can ﬁnd the average force by application of Eq. 6-7:

Fav,x =Jx

∆t=(15.47 kg ·m/s)

(4.7×10−3s) = 3290 N.

E6-8 The magnitude of the impulse is J=F δt = (−984 N)(0.0270 s) = −26.6 N ·s. Then pf=

pi+ ∆p, so

vf=(0.420 kg)(13.8 m/s) + (−26.6 N ·s)

(0.420 kg) =−49.5 m/s.

The ball moves backward!

E6-9 The change in momentum of the ball is ∆p= (mv)−(−mv) = 2mv = 2(0.058 kg)(32 m/s) =

3.7 kg ·m/s. The impulse is the area under a force - time graph; for the trapezoid in the ﬁgure this

area is J=Fmax(2 ms + 6 ms)/2 = (4 ms)Fmax. Then Fmax = (3.7 kg ·m/s)/(4 ms) = 930 N.

E6-10 The ﬁnal speed of each object is given by vi=J/mi, where irefers to which object (as

opposed to “initial”). The object are going in diﬀerent directions, so the relative speed will be the

sum. Then

vrel =v1+v2= (300 N ·s)[1/(1200 kg)+1/(1800 kg)] = 0.42 m/s.

E6-11 Use Simpson’s rule. Then the area is given by

Jx=1

3h(f0+ 4f1+ 2f2+ 4f3+... + 4f13 + f14) ,

3(0.2 ms) (200 + 4 ·800 + 2 ·1200... N)

which gives Jx= 4.28 kg·m/s.

Since the impulse is the change in momentum, and the ball started from rest, pfx=Jx+pix= 4.28

kg·m/s. The ﬁnal velocity is then found from vx=px/m = 8.6 m/s.

E6-12 (a) The average speed during the the time the hand is in contact with the board is half of the

initial speed, or vav = 4.8 m/s. The time of contact is then t=y/vav = (0.028 m)/(4.8 m/s) = 5.8 ms.

(b) The impulse given to the board is the same as the magnitude in the change in momentum of

the hand, or J= (0.54 kg)(9.5 m/s) = 5.1 N ·s. Then Fav = (5.1 N ·s)/(5.8 ms) = 880 N.

E6-13 ∆p=J=F∆t= (3000 N)(65.0 s) = 1.95×105N·s. The direction of the thrust relative to

the velocity doesn’t matter in this exercise.

E6-14 (a) p=mv = (2.14×10−3kg)(483 m/s) = 1.03 kg ·m/s.

(b) The impulse imparted to the wall in one second is ten times the above momentum, or

J= 10.3 kg ·m/s. The average force is then Fav = (10.3 kg ·m/s)/(1.0 s) = 10.3 N.

E6-15 A transverse direction means at right angles, so the thrusters have imparted a momentum

suﬃcient to direct the spacecraft 100+3400 = 3500 km to the side of the original path. The spacecraft

is half-way through the six-month journey, so it has three months to move the 3500 km to the side.

This corresponds to a transverse speed of v= (3500×103m)/(90×86400 s) = 0.45 m/s.The required

time for the rocket to ﬁre is ∆t= (5400 kg)(0.45 m/s)/(1200 N) = 2.0 s.

E6-16 Total initial momentum is zero, so

vm=−ms

vs=−msg

mmgvs=−(0.158 lb)

(195 lb) (12.7 ft/s) = −1.0×10−2ft/s.

E6-17 Conservation of momentum:

pf,m+pf,c=pi,m+pi,c,

mmvf,m+mcvf,c=mmvi,m+mcvi,c,

vf,c−vi,c=mmvi,m−mmvf,m

∆vc=(75.2 kg)(2.33 m/s) −(75.2 kg)(0)

(38.6 kg) ,

= 4.54 m/s.

The answer is positive; the cart speed increases.

E6-18 Conservation of momentum:

pf,m+pf,c=pi,m+pi,c,

mm(vf,c−vrel) + mcvf,c= (mm+mc)vi,c,

(mm+mc)vf,c−mmvrel = (mm+mc)vi,c,

∆vc=mmvrel/(mm+mc),

=wvrel/(w+W).

E6-19 Conservation of momentum. Let mrefer to motor and crefer to command module:

pf,m+pf,c=pi,m+pi,c,

mm(vf,c−vrel) + mcvf,c= (mm+mc)vi,c,

(mm+mc)vf,c−mmvrel = (mm+mc)vi,c,

vf,c=mmvrel + (mm+mc)vi,c

(mm+mc),

=4mc(125 km/h) + (4mc+mc)(3860 km/h)

(4mc+mc)= 3960 km/h.

E6-20 Conservation of momentum. The block on the left is 1, the other is 2.

m1v1,f+m2v2,f=m1v1,i+m2v2,i,

v1,f=v1,i+m2

(v2,i−v2,f),

= (5.5 m/s) + (2.4 kg)

(1.6 kg) [(2.5 m/s) −(4.9 m/s)],

= 1.9 m/s.

E6-21 Conservation of momentum. The block on the left is 1, the other is 2.

m1v1,f+m2v2,f=m1v1,i+m2v2,i,

v1,f=v1,i+m2

(v2,i−v2,f),

= (5.5 m/s) + (2.4 kg)

(1.6 kg) [(−2.5 m/s) −(4.9 m/s)],

=−5.6 m/s.

E6-22 Assume a completely inelastic collision. Call the Earth 1 and the meteorite 2. Then

m1v1,f+m2v2,f=m1v1,i+m2v2,i,

v1,f=m2v2,i

m1+m2

=(5×1010kg)(7200 m/s)

(5.98×1024kg) + (5×1010kg) = 7×10−11m/s.

That’s 2 mm/y!

E6-23 Conservation of momentum is used to solve the problem:

Pf=Pi,

pf,bl +pf,bu =pi,bl +pi,bu,

mblvf,bl +mbuvf,bu =mblvi,bl +mbuvi,bu,

(715 g)vf,bl + (5.18 g)(428 m/s) = (715 g)(0) + (5.18 g)(672 m/s),

which has solution vf,bl = 1.77 m/s.

E6-24 The ycomponent of the initial momentum is zero; therefore the magnitudes of the ycom-

ponents of the two particles must be equal after the collision. Then

mαvαsin θα=mOvOsin θO,

vα=mOvOsin θO

mαvαsin θα

=(16 u)(1.20×105m/s) sin(51◦)

(4.00 u) sin(64◦)= 4.15×105m/s.

E6-25 The total momentum is

p= (2.0 kg)[(15 m/s)ˆ

i+ (30 m/s)ˆ

j] + (3.0 kg)[(−10 m/s)ˆ

i+ (5 m/s)ˆ

j],

= 75 kg ·m/sˆ

The ﬁnal velocity of Bis

vBf=1

p−mA~

vAf),

(3.0 kg) {(75 kg ·m/sˆ

j)−(2.0 kg)[(−6.0 m/s)ˆ

i+ (30 m/s)ˆ

j]},

= (4.0 m/s)ˆ

i+ (5.0 m/s)ˆ

E6-26 Assume electron travels in +xdirection while neutrino travels in +ydirection. Conservation

of momentum requires that

p=−(1.2×10−22kg ·m/s)ˆ

i−(6.4×10−23kg ·m/s)ˆ

be the momentum of the nucleus after the decay. This has a magnitude of p= 1.4×10−22kg ·m/s

and be directed 152◦from the electron.

E6-27 What we know:

p1,i= (1.50×105kg)(6.20 m/s)ˆ

i= 9.30×105kg ·m/sˆ

p2,i= (2.78×105kg)(4.30 m/s)ˆ

j= 1.20×106kg ·m/sˆ

p2,f= (2.78×105kg)(5.10 m/s)[sin(18◦)ˆ

i+ cos(18◦)ˆ

j],

= 4.38×105kg ·m/sˆ

i+ 1.35×106kg ·m/sˆ

Conservation of momentum then requires

p1,f= (9.30×105kg ·m/sˆ

i)−(4.38×105kg ·m/sˆ

+(1.20×106kg ·m/sˆ

j)−(1.35×106kg ·m/sˆ

j),

= 4.92×105kg ·m/sˆ

i−1.50×105kg ·m/sˆ

This corresponds to a velocity of

v1,f= 3.28 m/sˆ

i−1.00 m/sˆ

which has a magnitude of 3.43 m/s directed 17◦to the right.

E6-28 vf=−2.1 m/s.

E6-29 We want to solve Eq. 6-24 for m2given that v1,f= 0 and v1,i=−v2,i. Making these

substitutions

(0) = m1−m2

m1+m2

v1,i+2m2

m1+m2

(−v1,i),

0=(m1−m2)v1,i−(2m2)v1,i,

3m2=m1

so m2= 100 g.

E6-30 (a) Rearrange Eq. 6-27:

m2=m1

v1i −v1f

v1i +v1f

= (0.342 kg) (1.24 m/s) −(0.636 m/s)

(1.24 m/s) + (0.636 m/s) = 0.110 kg.

(b) v2f = 2(0.342 kg)(1.24 m/s)/(0.342 kg + 0.110 kg) = 1.88 m/s.

E6-31 Rearrange Eq. 6-27:

m2=m1

v1i −v1f

v1i +v1f

= (2.0 kg) v1i −v1i/4

v1i +v1i/4= 1.2 kg.

E6-32 I’ll multiply all momentum equations by g, then I can use weight directly without converting

to mass.

(a) vf= [(31.8 T)(5.20 ft/s) + (24.2 T)(2.90 ft/s)]/(31.8 T + 24.2 T) = 4.21 ft/s.

(b) Evaluate:

v1f =31.8 T −24.2 T

31.8 T + 24.2 T (5.20 ft/s) + 2(24.2 T)

31.8 T + 24.2 T (2.90 ft/s) = 3.21 ft/s.

v2f =−31.8 T −24.2 T

31.8 T + 24.2 T (2.90 ft/s) + 2(31.8 T)

31.8 T + 24.2 T (5.20 ft/s) = 5.51 ft/s.

E6-33 Let the initial momentum of the ﬁrst object be ~

p1,i=m~

v1,i, that of the second object be

p2,i=m~

v2,i, and that of the combined ﬁnal object be ~

pf= 2m~

vf. Then

p1,i+~

p2,i=~

pf,

implies that we can ﬁnd a triangle with sides of length p1,i,p2,i, and pf. These lengths are

p1,i=mvi,

p2,i=mvi,

pf= 2mvf= 2mvi/2 = mvi,

so this is an equilateral triangle. This means the angle between the initial velocities is 120◦.

E6-34 We need to change to the center of mass system. Since both particles have the same

mass, the conservation of momentum problem is eﬀectively the same as a (vector) conservation of

velocity problem. Since one of the particles is originally at rest, the center of mass moves with

speed vcm =v1i/2. In the ﬁgure below the center of mass velocities are primed; the transformation

velocity is vt.

v’

Note that since vt=v01i =v02i =v01f =v02f the entire problem can be inscribed in a rhombus.

The diagonals of the rhombus are the directions of v1f and v2f ; note that the diagonals of a rhombus

are necessarily at right angles!

(a) The target proton moves oﬀ at 90◦to the direction the incident proton moves after the

collision, or 26◦away from the incident protons original direction.

(b) The ycomponents of the ﬁnal momenta must be equal, so v2f sin(26◦) = v1f sin(64◦), or v2f =

v1f tan(64◦). The xcomponents must add to the original momentum, so (514 m/s) = v2f cos(26◦) +

v1f cos(64◦), or

v1f = (514 m/s)/{tan(64◦) cos(26◦) + cos(64◦)}= 225 m/s,

and

v2f = (225 m/s) tan(64◦) = 461 m/s.

E6-35 vcm ={(3.16 kg)(15.6 m/s)+(2.84 kg)(−12.2 m/s)}/{(3.16 kg)+(2.84 kg)}= 2.44 m/s, pos-

itive means to the left.

P6-1 The force is the change in momentum over change in time; the momentum is the mass time

velocity, so

F=∆p

∆t=m∆v

∆t= ∆vm

∆t= 2uµ,

since µis the mass per unit time.

P6-2 (a) The initial momentum is ~

pi= (1420 kg)(5.28 m/s)ˆ

j= 7500 kg ·m/sˆ

j. After making the

right hand turn the ﬁnal momentum is ~

pf= 7500 kg ·m/sˆ

i. The impulse is ~

J= 7500 kg ·m/sˆ

i−

7500 kg ·m/sˆ

j, which has magnitude J= 10600 kg ·m/s.

(b) During the collision the impulse is ~

J= 0−7500 kg·m/sˆ

i. The magnitude is J= 7500 kg·m/s.

(d) The average force is F=J/t = (7500 kg ·m/s)/(0.350 s) = 21400 N.

P6-3 (a) Only the component of the momentum which is perpendicular to the wall changes. Then

J= ∆~

p=−2(0.325 kg)(6.22 m/s) sin(33◦)ˆ

j=−2.20 kg ·m/sˆ

(b) ~

F=−~

J/t =−(−2.20 kg ·m/sˆ

j)/(0.0104 s) = 212 N.

P6-4 The change in momentum of one bullet is ∆p= 2mv = 2(0.0030 kg)(500 m/s) = 3.0 kg ·m/s.

The average force is the total impulse in one minute divided by one minute, or

Fav = 100(3.0 kg ·m/s)/(60 s) = 5.0 N.

P6-5 (a) The volume of a hailstone is V= 4πr3/3 = 4π(0.5 cm)3/3 = 0.524 cm3.The mass of a

hailstone is m=ρV = (9.2×10−4kg/cm3)(0.524 cm3) = 4.8×10−4kg.

(b) The change in momentum of one hailstone when it hits the ground is

∆p= (4.8×10−4kg)(25 m/s) = 1.2×10−2kg ·m/s.

The hailstones fall at 25 m/s, which means that in one second the hailstones in a column 25 m high

hit the ground. Over an area of 10 m×20 m then there would be (25 m)(10 m)(20 m) = 500 m3worth

of hailstones, or 6.00×105hailstones per second striking the surface. Then

Fav = 6.00×105(1.2×10−2kg ·m/s)/(1 s) = 7200 N.

P6-6 Assume the links are not connected once the top link is released. Consider the link that

starts habove the table; it falls a distance hin a time t=p2h/g and hits the table with a speed

v=gt =√2hg. When the link hits the table hof the chain is already on the table, and L−his yet

to come. The linear mass density of the chain is M/L, so when this link strikes the table the mass is

hitting the table at a rate dm/dt = (M/L)v= (M/L)√2hg. The average force required to stop the

falling link is then v dm/dt = (M/L)2hg = 2(M/L)hg. But the weight of the chain that is already

on the table is (M/L)hg, so the net force on the table is the sum of these two terms, or F= 3W.

P6-7 The weight of the marbles in the box after a time tis mgRt because Rt is the number of

marbles in the box.

The marbles fall a distance hfrom rest; the time required to fall this distance is t=p2h/g,

the speed of the marbles when they strike the box is v=gt =√2gh. The momentum each marble

imparts on the box is then m√2gh. If the marbles strike at a rate Rthen the force required to stop

them is Rm√2gh.

The reading on the scale is then

W=mR(p2gh +gt).

This will give a numerical result of

(4.60×10−3kg)(115 s−1)p2(9.81 m/s2)(9.62 m) + (9.81 m/s2)(6.50 s)= 41.0 N.

P6-8 (a) v= (108 kg)(9.74 m/s)/(108 kg + 1930 kg) = 0.516 m/s.

(b) Label the person as object 1 and the car as object 2. Then m1v1+m2v2= (108 kg)(9.74 m/s)

and v1=v2+ 0.520 m/s. Combining,

v2= [1050 kg ·m/s−(0.520 m/s)(108 kg)]/(108 kg + 1930 kg) = 0.488 m/s.

P6-9 (a) It takes a time t1=p2h/g to fall h= 6.5 ft. An object will be moving at a speed

v1=gt1=√2hg after falling this distance. If there is an inelastic collision with the pile then the

two will move together with a speed of v2=Mv1/(M+m) after the collision.

If the pile then stops within d= 1.5 inches, then the time of stopping is given by t2=d/(v2/2) =

2d/v2.

For inelastic collisions this corresponds to an average force of

Fav =(M+m)v2

=(M+m)v2

2d=M2v2

2(M+m)d=(gM)2

g(M+m)

Note that we multiply through by gto get weights. The numerical result is Fav = 130 t.

(b) For an elastic collision v2= 2M v1/(M+m); the time of stopping is still expressed by

t2= 2d/v2, but we now know Fav instead of d. Then

Fav =mv2

=mv2

2d=4Mmv2

(M+m)d=2(gM)(gm)

g(M+m)

d=2(gM)(gm)

g(M+m)

Fav

which has a numerical result of d= 0.51 inches.

But wait! The weight, which just had an elastic collision, “bounced” oﬀ of the pile, and then hit

it again. This drives the pile deeper into the earth. The weight hits the pile a second time with a

speed of v3= (M−m)/(M+m)v1; the pile will (in this second elastic collision) then have a speed of

v4= 2M(M+m)v3= [(M−m)/(M+m)]v2. In other words, we have an inﬁnite series of distances

traveled by the pile, and if α= [(M−m)/(M+m)] = 0.71, the depth driven by the pile is

df=d(1 + α2+α4+α6···) = d

1−α2,

or d= 1.03.

P6-10 The cat jumps oﬀ of sled A; conservation of momentum requires that MvA,1+m(vA,1+vc) =

0, or

vA,1=−mvc/(m+M) = −(3.63 kg)(3.05 m/s)/(22.7 kg + 3.63 kg) = −0.420 m/s.

The cat lands on sled B; conservation of momentum requires vB,1=m(vA,1+vc)/(m+M). The

cat jumps oﬀ of sled B; conservation of momentum is now

MvB,2+m(vB,2−vc) = m(vA,1+vc),

vB,2= 2mvc/(m+M) = (3.63 kg)[(−0.420 m/s) + 2(3.05 m/s)]/(22.7 kg + 3.63 kg) = 0.783 m/s.

The cat then lands on cart A; conservation of momentum requires that (M+m)vA,2=−MvB,2, or

vA,2=−(22.7 kg)(0.783 m/s)/(22.7 kg + 3.63 kg) = −0.675 m/s.

P6-11 We align the coordinate system so that west is +xand south is +y. The each car

contributes the following to the initial momentum

A: (2720 lb/g)(38.5 mi/h)ˆ

i= 1.05×105lb ·mi/h/gˆ

B: (3640 lb/g)(58.0 mi/h)ˆ

j= 2.11×105lb ·mi/h/g ˆ

These become the components of the ﬁnal momentum. The direction is then

θ= arctan 2.11×105lb ·mi/h/g

1.05×105lb ·mi/h/g = 63.5◦,

south of west. The magnitude is the square root of the sum of the squares,

2.36×105lb ·mi/h/g,

and we divide this by the mass (6360 lb/g) to get the ﬁnal speed after the collision: 37.1 mi/h.

P6-12 (a) Ball Amust carry oﬀ a momentum of ~

p=mBvˆ

i−mBv/2ˆ

j, which would be in a direction

θ= arctan(−0.5/1) = 27◦from the original direction of B, or 117◦from the ﬁnal direction.

(b) No.

P6-13 (a) We assume all balls have a mass m. The collision imparts a “sideways” momentum to

the cue ball of m(3.50 m/s) sin(65◦) = m(3.17 m/s). The other ball must have an equal, but opposite

“sideways” momentum, so −m(3.17 m/s) = m(6.75 m/s) sin θ, or θ=−28.0◦.

(b) The ﬁnal “forward” momentum is

m(3.50 m/s) cos(65◦) + m(6.75 m/s) cos(−28◦) = m(7.44 m/s),

so the initial speed of the cue ball would have been 7.44 m/s.

P6-14 Assuming Mm, Eq. 6-25 becomes

v2f = 2v1i −v1i = 2(13 km/s) −(−12 km/s) = 38 km/s.

P6-15 (a) We get

v2,f=2(220 g)

(220 g) + (46.0 g) (45.0 m/s) = 74.4 m/s.

(b) Doubling the mass of the clubhead we get

v2,f=2(440 g)

(440 g) + (46.0 g) (45.0 m/s) = 81.5 m/s.

v2,f=2(660 g)

(660 g) + (46.0 g) (45.0 m/s) = 84.1 m/s.

Although the heavier club helps some, the maximum speed to get out of the ball will be less than

twice the speed of the club.

P6-16 There will always be at least two collisions. The balls are a,b, and cfrom left to right.

After the ﬁrst collision between aand bone has

vb,1=v0and va,1= 0.

After the ﬁrst collision between band cone has

vc,1= 2mv0/(m+M) and vb,2= (m−M)v0/(m+M).

(a) If m≥Mthen ball bcontinue to move to the right (or stops) and there are no more collisions.

(b) If m < M then ball bbounces back and strikes ball awhich was at rest. Then

va,2= (m−M)v0/(m+M) and vb,3= 0.

P6-17 All three balls are identical in mass and radii? Then balls 2 and 3 will move oﬀ at 30◦to

the initial direction of the ﬁrst ball. By symmetry we expect balls 2 and 3 to have the same speed.

The problem now is to deﬁne an elastic three body collision. It is no longer the case that the

balls bounce oﬀ with the same speed in the center of mass. One can’t even treat the problem as two

separate collisions, one right after the other. No amount of momentum conservation laws will help

solve the problem; we need some additional physics, but at this point in the text we don’t have it.

P6-18 The original speed is v0in the lab frame. Let αbe the angle of deﬂection in the cm frame

and ~

1be the initial velocity in the cm frame. Then the velocity after the collision in the cm frame

is v0

1cos αˆ

i+v0

1sin αˆ

jand the velocity in the lab frame is (v0

1cos α+v)ˆ

i+v0

1sin αˆ

j, where vis the

speed of the cm frame. The deﬂection angle in the lab frame is

θ= arctan[(v0

1sin α)/(v0

1cos α+v)],

but v=m1v0/(m1+m2) and v0

1=v0−vso v0

1=m2v0/(m1+m2) and

θ= arctan[(m2sin α)/(m2cos α+m1)].

−m1/m2if m1≤m2. Then [(m2sin α)/(m2cos α+m1)] can have any value between −∞ and ∞,

so θcan be between 0 and π.

(a) If m1> m2then (cos α+m1/m2)/sin αis a minimum when cos α=−m2/m1, then

[(m2sin α)/(m2cos α+m1)] = m2/qm2

1−m2

If tan θ=m2/pm2

1−m2

2then m1is like a hypotenuse and m2the opposite side. Then

cos θ=qm2

1−m2

2/m1=p1−(m2/m1)2.

(b) We need to change to the center of mass system. Since both particles have the same mass,

the conservation of momentum problem is eﬀectively the same as a (vector) conservation of velocity

problem. Since one of the particles is originally at rest, the center of mass moves with speed

vcm =v1i/2. In the ﬁgure below the center of mass velocities are primed; the transformation

velocity is vt.

v’

Note that since vt=v01i =v02i =v01f =v02f the entire problem can be inscribed in a rhombus.

The diagonals of the rhombus are the directions of v1f and v2f ; note that the diagonals of a rhombus

are necessarily at right angles!

P6-19 (a) The speed of the bullet after leaving the ﬁrst block but before entering the second can

be determined by momentum conservation.

Pf=Pi,

pf,bl +pf,bu =pi,bl +pi,bu,

mblvf,bl +mbuvf,bu =mblvi,bl +mbuvi,bu,

(1.78kg)(1.48 m/s)+(3.54×10−3kg)(1.48 m/s) = (1.78kg)(0)+(3.54×10−3kg)vi,bu,

which has solution vi,bl = 746 m/s.

(b) We do the same steps again, except applied to the ﬁrst block,

Pf=Pi,

pf,bl +pf,bu =pi,bl +pi,bu,

mblvf,bl +mbuvf,bu =mblvi,bl +mbuvi,bu,

(1.22kg)(0.63 m/s)+(3.54×10−3kg)(746 m/s) = (1.22kg)(0)+(3.54×10−3kg)vi,bu,

which has solution vi,bl = 963 m/s.

P6-20 The acceleration of the block down the ramp is a1=gsin(22◦). The ramp has a length of

d=h/ sin(22◦), so it takes a time t1=p2d/a1=p2h/g/ sin(22◦) to reach the bottom. The speed

when it reaches the bottom is v1=a1t1=√2gh. Notice that it is independent of the angle!

The collision is inelastic, so the two stick together and move with an initial speed of v2=

m1v1/(m1+m2). They slide a distance xbefore stopping; the average speed while decelerating is

vav =v2/2, so the stopping time is t2= 2x/v2and the deceleration is a2=v2/t2=v2

2/(2x). If the

retarding force is f= (m1+m2)a2, then f=µk(m1+m2)g. Glue it all together and

µk=m2

(m1+m2)2

x=(2.0 kg)2

(2.0 kg + 3.5 kg)2

(0.65 m)

(0.57 m) = 0.15.

P6-21 (a) For an object with initial speed vand deceleration −awhich travels a distance xbefore

stopping, the time tto stop is t=v/a, the average speed while stopping is v/2, and d=at2/2.

Combining, v=√2ax. The deceleration in this case is given by a=µkg.

Then just after the collision

vA=p2(0.130)(9.81 m/s2)(8.20 m) = 4.57 m/s,

while

vB=p2(0.130)(9.81 m/s2)(6.10 m) = 3.94 m/s,

(b) v0= [(1100 kg)(4.57 m/s) + (1400kg)(3.94 m/s)]/(1400 kg) = 7.53 m/s.

E7-1 xcm = (7.36×1022kg)(3.82×108m)/(7.36×1022kg + 5.98×1034kg) = 4.64×106m. This is less

than the radius of the Earth.

E7-2 If the particles are lapart then

x1=m1l(m1+m2)

is the distance from particle 1 to the center of mass and

x2=m2l(m1+m2)

is the distance from particle 2 to the center of mass. Divide the top equation by the bottom and

x1/x2=m1/m2.

E7-3 The center of mass velocity is given by Eq. 7-1,

vcm =m1~

v1+m2~

m1+m2

=(2210 kg)(105 km/h) + (2080 kg)(43.5 km/h)

(2210 kg) + (2080 kg) = 75.2 km/h.

E7-4 They will meet at the center of mass, so

xcm = (65 kg)(9.7 m)/(65 kg + 42 kg) = 5.9 m.

E7-5 (a) No external forces, center of mass doesn’t move.

(b) The collide at the center of mass,

xcm = (4.29 kg)(1.64 m)/(4.29 kg + 1.43 kg) = 1.23 m.

E7-6 The range of the center of mass is

R=v2

0sin 2θ /g = (466 m/s)2sin(2 ×57.4◦)/(9.81 m/s2) = 2.01×104m.

Half lands directly underneath the highest point, or 1.00×104m. The other piece must land at x,

such that

2.01×104m = (1.00×104m + x)/2;

then x= 3.02×104m.

E7-7 The center of mass of the boat + dog doesn’t move because there are no external forces on

the system. Deﬁne the coordinate system so that distances are measured from the shore, so toward

the shore is in the negative xdirection. The change in position of the center of mass is given by

∆xcm =md∆xd+mb∆xb

md+mb

= 0,

Both ∆xdand ∆xbare measured with respect to the shore; we are given ∆xdb =−8.50 ft, the

displacement of the dog with respect to the boat. But

∆xd= ∆xdb + ∆xb.

Since we want to ﬁnd out about the dog, we’ll substitute for the boat’s displacement,

0 = md∆xd+mb(∆xd−∆xdb)

md+mb

Rearrange and solve for ∆xd. Use W=mg and multiply the top and bottom of the expression by

g. Then

∆xd=mb∆xdb

md+mb

g=(46.4 lb)(−8.50 ft)

(10.8 lb) + (46.4 lb) =−6.90 ft.

The dog is now 21.4−6.9 = 14.5 feet from shore.

E7-8 Richard has too much time on his hands.

The center of mass of the system is xcm away from the center of the boat. Switching seats is

eﬀectively the same thing as rotating the canoe through 180◦, so the center of mass of the system

has moved through a distance of 2xcm = 0.412 m. Then xcm = 0.206 m. Then

xcm = (Ml −ml)/(M+m+mc) = 0.206 m,

where l= 1.47 m, M= 78.4 kg, mc= 31.6 kg, and mis Judy’s mass. Rearrange,

m=Ml −(M+mc)xcm

l+xcm

=(78.4 kg)(1.47 m) −(78.4 kg + 31.6 kg)(0.206 m)

(1.47 m) + (0.206 m) = 55.2 kg.

E7-9 It takes the man t= (18.2 m)/(2.08 m/s) = 8.75 s to walk to the front of the boat. During

this time the center of mass of the system has moved forward x= (4.16 m/s)(8.75 s) = 36.4 m. But

in walking forward to the front of the boat the man shifted the center of mass by a distance of

(84.4 kg)(18.2 m)/(84.4 kg + 425 kg) = 3.02 m, so the boat only traveled 36.4 m −3.02 m = 33.4 m.

E7-10 Do each coordinate separately.

xcm =(3 kg)(0) + (8 kg)(1 m) + (4 kg)(2 m)

(3 kg) + (8 kg) + (4 kg) = 1.07 m

and

ycm =(3 kg)(0) + (8 kg)(2 m) + (4 kg)(1 m)

(3 kg) + (8 kg) + (4 kg) = 1.33 m

E7-11 The center of mass of the three hydrogen atoms will be at the center of the pyramid

base. The problem is then reduced to ﬁnding the center of mass of the nitrogen atom and the three

hydrogen atom triangle. This molecular center of mass must lie on the dotted line in Fig. 7-27.

The location of the plane of the hydrogen atoms can be found from Pythagoras theorem

yh=p(10.14×10−11m)2−(9.40×10−11m)2= 3.8×10−11m.

This distance can be used to ﬁnd the center of mass of the molecule. From Eq. 7-2,

ycm =mnyn+mhyh

mn+mh

=(13.9mh)(0) + (3mh)(3.8×10−11m)

(13.9mh) + (3mh)= 6.75×10−12m.

E7-12 The velocity components of the center of mass at t= 1.42 s are vcm,x = 7.3 m/s and

vcm,y = (10.0 m/s) −(9.81 m/s)(1.42 s) = −3.93 m/s. Then the velocity components of the “other”

piece are

v2,x = [(9.6 kg)(7.3 m/s) −(6.5 kg)(11.4 m/s)]/(3.1 kg) = −1.30 m/s.

and

v2,y = [(9.6 kg)(−3.9 m/s) −(6.5 kg)(−4.6 m/s)]/(3.1 kg) = −2.4 m/s.

E7-13 The center of mass should lie on the perpendicular bisector of the rod of mass 3M. We

can view the system as having two parts: the heavy rod of mass 3Mand the two light rods each of

mass M. The two light rods have a center of mass at the center of the square.

Both of these center of masses are located along the vertical line of symmetry for the object.

The center of mass of the heavy bar is at yh,cm = 0, while the combined center of mass of the two

light bars is at yl,cm =L/2, where down is positive. The center of mass of the system is then at

ycm =2Myl,cm + 3Myh,cm

2M+ 3M=2(L/2)

5=L/5.

E7-14 The two slabs have the same volume and have mass mi=ρiV. The center of mass is

located at

xcm =m1l−m2l

m1+m2

=lρ1−ρ2

ρ1+ρ2

= (5.5 cm)(7.85 g/cm3)−(2.70 g/cm3)

(7.85 g/cm3) + (2.70 g/cm3)= 2.68 cm

from the boundary inside the iron; it is centered in the yand zdirections.

E7-15 Treat the four sides of the box as one thing of mass 4mwith a mass located l/2 above the

base. Then the center of mass is

zcm = (l/2)(4m)/(4m+m) = 2l/5 = 2(0.4 m)/5 = 0.16 m,

xcm =ycm = 0.2 m.

E7-16 One piece moves oﬀ with momentum m(31.4 m/s)ˆ

i, another moves oﬀ with momentum

2m(31.4 m/s)ˆ

j. The third piece must then have momentum −m(31.4 m/s)ˆ

i−2m(31.4 m/s)ˆ

jand

velocity −(1/3)(31.4 m/s)ˆ

i−2/3(31.4 m/s)ˆ

j=−10.5 m/sˆ

i−20.9 m/sˆ

j. The magnitude of v3is

23.4 m/s and direction 63.3◦away from the lighter piece.

E7-17 It will take an impulse of (84.7 kg)(3.87 m/s) = 328 kg ·m/s to stop the animal. This would

come from ﬁring nbullets where n= (328 kg ·m/s)/[(0.0126 kg)(975 m/s) = 27.

E7-18 Conservation of momentum for ﬁring one cannon ball of mass mwith muzzle speed vc

forward out of a cannon on a trolley of original total mass Mmoving forward with original speed

v0is

Mv0= (M−m)v1+m(vc+v1) = Mv1+mvc,

where v1is the speed of the trolley after the cannonball is ﬁred. Then to stop the trolley we require

ncannonballs be ﬁred so that

n= (Mv0)/(mvc) = [(3500 kg)(45 m/s)]/[(65 kg)(625 m/s)] = 3.88,

so n= 4.

E7-19 Label the velocities of the various containers as ~

vkwhere kis an integer between one and

twelve. The mass of each container is m. The subscript “g” refers to the goo; the subscript krefers

to the kth container.

The total momentum before the collision is given by

P=X

vk,i+mg~

vg,i= 12m~

vcont.,cm +mg~

vg,i.

We are told, however, that the initial velocity of the center of mass of the containers is at rest, so

the initial momentum simpliﬁes to ~

P=mg~

vg,i, and has a magnitude of 4000 kg·m/s.

(a) Then

vcm =P

12m+mg

=(4000 kg·m/s)

12(100.0 kg) + (50 kg) = 3.2 m/s.

(b) It doesn’t matter if the cord breaks, we’ll get the same answer for the motion of the center

of mass.

E7-20 (a) F= (3270 m/s)(480 kg/s) = 1.57×106N.

(b) m= 2.55×105kg −(480 kg/s)(250 s) = 1.35×105kg.

vf= (−3270 m/s) ln(1.35×105kg/2.55×105kg) = 2080 m/s.

E7-21 Use Eq. 7-32. The initial velocity of the rocket is 0. The mass ratio can then be found

from a minor rearrangement; Mi

=e|vf/vrel|

The “ﬂipping” of the left hand side of this expression is possible because the exhaust velocity is

negative with respect to the rocket. For part (a) Mi/M f=e= 2.72. For part (b) Mi/Mf=e2=

7.39.

E7-22 Eq. 7-32 rearranged:

=e−|∆v/vrel |=e−(22.6m/s)/(1230m/s) = 0.982.

The fraction of the initial mass which is discarded is 0.0182.

E7-23 The loaded rocket has a weight of (1.11×105kg)(9.81 m/s2) = 1.09×106N; the thrust must be

at least this large to get the rocket oﬀ the ground. Then v≥(1.09×106N)/(820 kg/s) = 1.33×103m/s

is the minimum exhaust speed.

E7-24 The acceleration down the incline is (9.8 m/s2) sin(26◦)=4.3 m/s2. It will take t=

p2(93 m)/(4.3 m/s2) = 6.6 s. The sand doesn’t aﬀect the problem, so long as it only “leaks” out.

E7-25 We’ll use Eq. 7-4 to solve this problem, but since we are given weights instead of mass

we’ll multiply the top and bottom by glike we did in Exercise 7-7. Then

vcm =m1~

v1+m2~

m1+m2

g=W1~

v1+W2~

W1+W2

Now for the numbers

vcm =(9.75 T)(1.36 m/s) + (0.50 T)(0)

(9.75 T) + (0.50 T) = 1.29 m/s.

P7-1 (a) The balloon moves down so that the center of mass is stationary;

0 = Mvb+mvm=Mvb+m(v+vb),

or vb=−mv/(m+M).

(b) When the man stops so does the balloon.

P7-2 (a) The center of mass is midway between them.

(b) Measure from the heavier mass.

xcm = (0.0560 m)(0.816 kg)/(1.700 kg) = 0.0269 m,

which is 1.12 mm closer to the heavier mass than in part (a).

a= 2∆m g/(m1+m2) = 2(0.034 kg)g/(1.700 kg) = 0.0400g,

the heavier going down while the lighter moves up. The acceleration of the center of mass is

acm = (am1−am2)/(m1+m2) = (0.0400g)2(0.034 kg)g/(1.700 kg) = 0.00160g.

P7-3 This is a gloriﬁed Atwood’s machine problem. The total mass on the right side is the mass

per unit length times the length, mr=λx; similarly the mass on the left is given by ml=λ(L−x).

Then

a=m2−m1

m2+m1

g=λx −λ(L−x)

λx +λ(L−x)g=2x−L

which solves the problem. The acceleration is in the direction of the side of length xif x > L/2.

P7-4 (a) Assume the car is massless. Then moving the cannonballs is moving the center of mass,

unless the cannonballs don’t move but instead the car does. How far? L.

(b) Once the cannonballs stop moving so does the rail car.

P7-5 By symmetry, the center of mass of the empty storage tank should be in the very center,

along the axis at a height yt,cm =H/2. We can pretend that the entire mass of the tank, mt=M,

is located at this point.

The center of mass of the gasoline is also, by symmetry, located along the axis at half the height of

the gasoline, yg,cm =x/2. The mass, if the tank were ﬁlled to a height H, is m; assuming a uniform

density for the gasoline, the mass present when the level of gas reaches a height xis mg=mx/H.

(a) The center of mass of the entire system is at the center of the cylinder when the tank is full

and when the tank is empty. When the tank is half full the center of mass is below the center. So as

the tank changes from full to empty the center of mass drops, reaches some lowest point, and then

rises back to the center of the tank.

(b) The center of mass of the entire system is found from

ycm =mgyg,cm +mtyt,cm

mg+mt

=(mx/H)(x/2) + (M)(H/2)

(mx/H)+(M)=mx2+MH2

2mx + 2MH .

Take the derivative:

dycm

dx =mmx2+ 2xMH −MH2

(mx +MH)2

Set this equal to zero to ﬁnd the minimum; this means we want the numerator to vanish, or mx2+

2xMH −MH2= 0. Then

x=−M+√M2+mM

mH.

P7-6 The center of mass will be located along symmetry axis. Call this the xaxis. Then

xcm =1

MZxdm,

πR2ZR

0Z√R2−x2

x dy dx,

πR2ZR

xpR2−x2dx,

πR2R3/3 = 4R

3π.

P7-7 (a) The components of the shell velocity with respect to the cannon are

x= (556 m/s) cos(39.0◦) = 432 m/s and v0

y= (556 m/s) sin(39.0◦) = 350 m/s.

The vertical component with respect to the ground is the same, vy=v0

y, but the horizontal compo-

nent is found from conservation of momentum:

M(vx−v0

x) + m(vx) = 0,

so vx= (1400 kg)(432 m/s)(70.0 kg + 1400 kg) = 411 m/s. The resulting speed is v= 540 m/s.

(b) The direction is θ= arctan(350/411) = 40.4◦.

P7-8 v= (2870 kg)(252 m/s)/(2870 kg + 917 kg) = 191 m/s.

P7-9 It takes (1.5 m/s)(20 kg) = 30 N to accelerate the luggage to the speed of the belt. The

people when taking the luggage oﬀ will (on average) also need to exert a 30 N force to remove it;

this force (because of friction) will be exerted on the belt. So the belt requires 60 N of additional

force.

P7-10 (a) The thrust must be at least equal to the weight, so

dm/dt = (5860 kg)(9.81 m/s2)/(1170 m/s) = 49.1 kg/s.

(b) The net force on the rocket will need to be F= (5860 kg)(18.3 m/s2) = 107000 N. Add this

to the weight to ﬁnd the thrust, so

dm/dt = [107000 N + (5860 kg)(9.81 m/s2)]/(1170 m/s) = 141 kg/s

P7-11 Consider Eq. 7-31. We want the barges to continue at constant speed, so the left hand

side of that equation vanishes. Then

Fext =−~

vrel

dt .

We are told that the frictional force is independent of the weight, since the speed doesn’t change the

frictional force should be constant and equal in magnitude to the force exerted by the engine before

the shoveling happens. Then P~

Fext is equal to the additional force required from the engines. We’ll

call it ~

The relative speed of the coal to the faster moving cart has magnitude: 21.2−9.65 = 11.6

km/h= 3.22 m/s. The mass ﬂux is 15.4 kg/s, so P= (3.22 m/s)(15.4kg/s) = 49.6 N. The faster

moving cart will need to increase the engine force by 49.6 N. The slower cart won’t need to do

anything, because the coal left the slower barge with a relative speed of zero according to our

approximation.

P7-12 (a) Nothing is ejected from the string, so vrel = 0. Then Eq. 7-31 reduces to m dv/dt =Fext.

(b) Since Fext is from the weight of the hanging string, and the fraction that is hanging is y/L,

Fext =mgy/L. The equation of motion is then d2y/dt2=gy/L.

dt =y0

2(pg/L)e√g/Lt −e−√g/Lt,

and then second derivative,

d2y

dt2=y0

2(pg/L)2e√g/Lt +e−√g/Lt.

Substitute into equation of motion. It works! Note that when t= 0 we have y=y0.

E8-1 An n-dimensional object can be oriented by stating the position of ndiﬀerent carefully

chosen points Piinside the body. Since each point has ncoordinates, one might think there are n2

coordinates required to completely specify the position of the body. But if the body is rigid then

the distances between the points are ﬁxed. There is a distance dij for every pair of points Piand

Pj. For each distance dij we need one fewer coordinate to specify the position of the body. There

are n(n−1)/2 ways to connect nobjects in pairs, so n2−n(n−1)/2 = n(n+ 1)/2 is the number

of coordinates required.

E8-2 (1 rev/min)(2πrad/rev)/(60 s/min) = 0.105 rad/s.

E8-3 (a) ω=a+ 3bt2−4ct3.

(b) α= 6bt −12t2.

E8-4 (a) The radius is r= (2.3×104ly)(3.0×108m/s) = 6.9×1012m·y/s. The time to make one

revolution is t= (2π6.9×1012m·y/s)/(250×103m/s) = 1.7×108y.

(b) The Sun has made 4.5×109y/1.7×108y = 26 revolutions.

E8-5 (a) Integrate.

ωz=ω0+Zt

04at3−3bt2dt =ω0+at4−bt3

(b) Integrate, again.

∆θ=Zt

ωzdt =Zt

0ω0+at4−bt3dt =ω0t+1

5at5−1

4bt4

E8-6 (a) (1 rev/min)(2πrad/rev)/(60 s/min) = 0.105 rad/s.

(b) (1 rev/h)(2πrad/rev)/(3600 s/h) = 1.75×10−3rad/s.

E8-7 85 mi/h = 125 ft/s. The ball takes t= (60 ft)/(125 ft/s) = 0.48 s to reach the plate. It

makes (30 rev/s)(0.48 s) = 14 revolutions in that time.

E8-8 It takes t=p2(10 m)/(9.81 m/s2)=1.43 s to fall 10 m. The average angular velocity is

then ω= (2.5)(2πrad)/(1.43 s) = 11 rad/s.

E8-9 (a) Since there are eight spokes, this means the wheel can make no more than 1/8 of a

revolution while the arrow traverses the plane of the wheel. The wheel rotates at 2.5 rev/s; it

makes one revolution every 1/2.5=0.4 s; so the arrow must pass through the wheel in less than

0.4/8 = 0.05 s.

The arrow is 0.24 m long, and it must move at least one arrow length in 0.05 s. The corresponding

minimum speed is (0.24 m)/(0.05 s) = 4.8 m/s.

(b) It does not matter where you aim, because the wheel is rigid. It is the angle through which

the spokes have turned, not the distance, which matters here.

E8-10 We look for the times when the Sun, the Earth, and the other planet are collinear in some

speciﬁed order.

Since the outer planets revolve around the Sun more slowly than Earth, after one year the Earth

has returned to the original position, but the outer planet has completed less than one revolution.

The Earth will then “catch up” with the outer planet before the planet has completed a revolution.

If θEis the angle through which Earth moved and θPis the angle through which the planet moved,

then θE=θP+ 2π, since the Earth completed one more revolution than the planet.

If ωPis the angular velocity of the planet, then the angle through which it moves during the

time TS(the time for the planet to line up with the Earth). Then

θE=θP+ 2π,

ωETS=ωPTS+ 2π,

ωE=ωP+ 2π/TS

The angular velocity of a planet is ω= 2π/T , where Tis the period of revolution. Substituting this

into the last equation above yields

1/TE= 1/TP+ 1/TS.

E8-11 We look for the times when the Sun, the Earth, and the other planet are collinear in some

speciﬁed order.

Since the inner planets revolve around the Sun more quickly than Earth, after one year the Earth

has returned to the original position, but the inner planet has completed more than one revolution.

The inner planet must then have “caught-up” with the Earth before the Earth has completed a

revolution. If θEis the angle through which Earth moved and θPis the angle through which the

planet moved, then θP=θE+ 2π, since the inner planet completed one more revolution than the

Earth.

If ωPis the angular velocity of the planet, then the angle through which it moves during the

time TS(the time for the planet to line up with the Earth). Then

θP=θE+ 2π,

ωPTS=ωETS+ 2π,

ωP=ωE+ 2π/TS

The angular velocity of a planet is ω= 2π/T , where Tis the period of revolution. Substituting this

into the last equation above yields

1/TP= 1/TE+ 1/TS.

E8-12 (a) α= (−78 rev/min)/(0.533 min) = −150 rev/min2.

(b) Average angular speed while slowing down is 39 rev/min, so (39 rev/min)(0.533 min) =

21 rev.

E8-13 (a) α= (2880 rev/min −1170 rev/min)/(0.210 min) = 8140 rev/min2.

(b) Average angular speed while accelerating is 2030 rev/min, so (2030 rev/min)(0.210 min) =

425 rev.

E8-14 Find area under curve.

2(5 min + 2.5 min)(3000 rev/min) = 1.13×104rev.

E8-15 (a) ω0z= 25.2 rad/s; ωz= 0; t= 19.7 s; and αzand φare unknown. From Eq. 8-6,

ωz=ω0z+αzt,

(0) = (25.2 rad/s) + αz(19.7 s),

αz=−1.28 rad/s2

(b) We use Eq. 8-7 to ﬁnd the angle through which the wheel rotates.

φ=φ0+ω0zt+1

2αzt2= (0)+(25.2 rad/s)(19.7 s)+ 1

2(−1.28 rad/s2)(19.7 s)2= 248 rad.

2πrad = 39.5 rev.

E8-16 (a) α= (225 rev/min −315 rev/min)/(1.00 min) = −90.0 rev/min2.

(b) t= (0 −225 rev/min)/(−90.0 rev/min2) = 2.50 min.

E8-17 (a) The average angular speed was (90 rev)/(15 s) = 6.0 rev/s. The angular speed at the

beginning of the interval was then 2(6.0 rev/s) −(10 rev/s) = 2.0 rev/s.

(b) The angular acceleration was (10 rev/s−2.0 rev/s)/(15 s) = 0.533 rev/s2. The time required

to get the wheel to 2.0 rev/s was t= (2.0 rev/s)/(0.533 rev/s2) = 3.8 s.

E8-18 (a) The wheel will rotate through an angle φwhere

φ= (563 cm)/(8.14 cm/2) = 138 rad.

(b) t=q2(138 rad)/(1.47 rad/s2) = 13.7 s.

E8-19 (a) We are given φ= 42.3 rev= 266 rad, ω0z= 1.44 rad/s, and ωz= 0. Assuming a

uniform deceleration, the average angular velocity during the interval is

ωav,z =1

2(ω0z+ωz) = 0.72 rad/s.

Then the time taken for deceleration is given by φ=ωav,zt, so t= 369 s.

(b) The angular acceleration can be found from Eq. 8-6,

ωz=ω0z+αzt,

(0) = (1.44 rad/s) + αz(369 s),

αz=−3.9×10−3rad/s2.

φ=φ0+ω0zt+1

2αzt2,

(133 rad) = (0) + (1.44 rad/s)t+1

2(−3.9×10−3rad/s2)t2,

0 = −133 + (1.44 s−1)t−(−1.95 ×10−3s−2)t2.

Solving this quadratic expression yields two answers: t= 108 s and t= 630 s.

E8-20 The angular acceleration is α= (4.96 rad/s)/(2.33 s) = 2.13 rad/s2.The angle through

which the wheel turned while accelerating is φ= (2.13 rad/s2)(23.0 s)2/2 = 563 rad.The angular

speed at this time is ω= (2.13 rad/s2)(23.0 s) = 49.0 rad/s.The wheel spins through an additional

angle of (49.0 rad/s)(46 s −23 s) = 1130 rad, for a total angle of 1690 rad.

E8-21 ω= (14.6 m/s)/(110 m) = 0.133 rad/s.

E8-22 The linear acceleration is (25 m/s−12 m/s)/(6.2 s) = 2.1 m/s2. The angular acceleration is

α= (2.1 m/s2)/(0.75 m/2) = 5.6 rad/s.

E8-23 (a) The angular speed is given by vT=ωr. So ω=vT/r = (28,700 km/hr)/(3220

km) = 8.91 rad/hr. That’s the same thing as 2.48×10−3rad/s.

(b) aR=ω2r= (8.91 rad/h)2(3220 km) = 256000 km/h2, or

aR= 256000 km/h2(1/3600 h/s)2(1000 m/km) = 19.8 m/s2.

trajectory!

E8-24 The bar needs to make

(1.50 cm)(12.0 turns/cm) = 18 turns.

This will happen is (18 rev)/(237 rev/min) = 4.56 s.

E8-25 (a) The angular speed is ω= (2πrad)/(86400 s) = 7.27×10−5rad/s.

(b) The distance from the polar axis is r= (6.37×106m) cos(40◦) = 4.88×106m. The linear speed

is then v= (7.27×10−5rad/s)(4.88×106m) = 355 m/s.

106m) cos(0◦) = 6.37×106m. The linear speed is then v= (7.27×10−5rad/s)(6.37×106m) = 463 m/s.

E8-26 (a) aT= (14.2 rad/s2)(0.0283 m) = 0.402 m/s2.

(b) Full speed is ω= 289 rad/s. aR= (289 rad/s)2(0.0283 m) = 2360 m/s2.

t= (289 rad/s)/(14.2 rad/s2) = 20.4 s

to get up to full speed. Then x= (0.402 m/s2)(20.4 s)2/2 = 83.6 m is the distance through which a

point on the rim moves.

E8-27 (a) The pilot sees the propeller rotate, no more. So the tip of the propeller is moving with

a tangential velocity of vT=ωr = (2000 rev/min)(2πrad/rev)(1.5 m) = 18900 m/min. This is the

same thing as 315 m/s.

(b) The observer on the ground sees this tangential motion and sees the forward motion of

the plane. These two velocity components are perpendicular, so the magnitude of the sum is

p(315 m/s)2+ (133 m/s)2= 342 m/s.

E8-28 aT=aRwhen rα =rω2=r(αt)2, or t=q1/(0.236 rad/s2) = 2.06 s.

E8-29 (a) aR=rω2=rα2t2.

(b) aT=rα.

φ=1

2αt2=1

2tan(57.0◦) = 0.77 rad = 44.1◦.

E8-30 (a) The tangential speed of the edge of the wheel relative axle is v= 27 m/s. ω=

(27 m/s)/(0.38 m) = 71 rad/s.

(b) The average angular speed while slowing is 71 rad/s/2, the time required to stop is then

t= (30 ×2πrad)/(71 rad/s/2) = 5.3 s. The angular acceleration is then α= (−71 rad/s)/(5.3 s) =

−13 rad/s.

E8-31 Yes, the speed would be wrong. The angular velocity of the small wheel would be ω=vt/rs,

but the reported velocity would be v=ωrl=vtrl/rs. This would be in error by a fraction

∆v

=(72 cm)

(62 cm) −1 = 0.16.

E8-32 (a) Square both equations and then add them:

x2+y2= (Rcos ωt)2+ (Rsin ωt)2=R2,

which is the equation for a circle of radius R.

(b) vx=−Rω sin ωt =−ωy;vy=Rω cos ωt =ωx. Square and add, v=ωR. The direction is

tangent to the circle.

(b) ax=−Rω2cos ωt =−ω2x;ay=−Rω2sin ωt =−ω2y. Square and add, a=ω2R. The

direction is toward the center.

E8-33 (a) The object is “slowing down”, so ~α = (−2.66 rad/s2)ˆ

k. We know the direction

because it is rotating about the zaxis and we are given the direction of ~ω. Then from Eq. 8-19,

v=~ω ×~

R= (14.3 rad/s)ˆ

k×[(1.83 m)ˆ

j+ (1.26 m)ˆ

k]. But only the cross term ˆ

k×ˆ

jsurvives, so

v= (−26.2 m/s)ˆ

(b) We ﬁnd the acceleration from Eq. 8-21,

a=~α ×~

R+~ω ×~

= (−2.66 rad/s2)ˆ

k×[(1.83 m)ˆ

j+ (1.26 m)ˆ

k] + (14.3 rad/s)ˆ

k×(−26.2 m/s)ˆ

= (4.87 m/s2)ˆ

i+ (−375 m/s2)ˆ

E8-34 (a) ~

F=−2m~ω ×~

v=−2mωv cos θ, where θis the latitude. Then

F= 2(12 kg)(2πrad/86400 s)(35 m/s) cos(45◦) = 0.043 N,

and is directed west.

(b) Reversing the velocity will reverse the direction, so east.

on the way down the Coriolis force slows down the westerly motion, but does not push it back east.

The object lands to the west of the starting point.

P8-1 (a) ω= (4.0 rad/s) −(6.0 rad/s2)t+ (3.0 rad/s)t2. Then ω(2.0 s) = 4.0 rad/s and ω(4.0 s) =

28.0 rad/s.

(b) αav = (28.0 rad/s −4.0 rad/s)/(4.0 s −2.0 s) = 12 rad/s2.

P8-2 If the wheel really does move counterclockwise at 4.0 rev/min, then it turns through

(4.0 rev/min)/[(60 s/min)(24 frames/s)] = 2.78×10−3rev/frame.

This means that a spoke has moved 2.78×10−3rev.There are 16 spokes each located 1/16 of a

revolution around the wheel. If instead of moving counterclockwise the wheel was instead moving

clockwise so that a diﬀerent spoke had moved 1/16 rev −2.78 ×10−3rev = 0.0597 rev, then the

same eﬀect would be present. The wheel then would be turning clockwise with a speed of ω=

(0.0597 rev)(60 s/min)(24 frames/s) = 86 rev/min.

100

P8-3 (a) In the diagram below the Earth is shown at two locations a day apart. The Earth rotates

clockwise in this ﬁgure.

Note that the Earth rotates through 2πrad in order to be correctly oriented for a complete

sidereal day, but because the Earth has moved in the orbit it needs to go farther through an angle

θin order to complete a solar day. By the time the Earth has gone all of the way around the sun

the total angle θwill be 2πrad, which means that there was one more sidereal day than solar day.

(b) There are (365.25 d)(24.000 h/d) = 8.7660×103hours in a year with 265.25 solar days. But

there are 366.25 sidereal days, so each one has a length of 8.7660×103/366.25 = 23.934 hours, or 23

hours and 56 minutes and 4 seconds.

P8-4 (a) The period is time per complete rotation, so ω= 2π/T .

(b) α= ∆ω/∆t, so

α=2π

T0+ ∆T−2π

T0/(∆t),

=2π

∆t−∆T

T0(T0+ ∆T),

≈2π

∆t−∆T

=2π

(3.16×107s) −(1.26×10−5s)

(0.033 s)2=−2.30×10−9rad/s2.

(d) 2π/T0= 2π/T −αt, or

T0=1/(0.033 s) −(−2.3×10−9rad/s2)(3.0×1010 s)/(2π)−1= 0.024 s.

P8-5 The ﬁnal angular velocity during the acceleration phase is ωz=αzt= (3.0 rad/s)(4.0

s) = 12.0 rad/s. Since both the acceleration and deceleration phases are uniform with endpoints

ωz= 0, the average angular velocity for both phases is the same, and given by half of the maximum:

ωav,z = 6.0 rad/s.

101

The angle through which the wheel turns is then

φ=ωav,zt= (6.0 rad/s)(4.1 s) = 24.6 rad.

The time is the total for both phases.

(a) The ﬁrst student sees the wheel rotate through the smallest angle less than one revolution;

this student would have no idea that the disk had rotated more than once. Since the disk moved

through 3.92 revolutions, the ﬁrst student will either assume the disk moved forward through 0.92

revolutions or backward through 0.08 revolutions.

(b) According to whom? We’ve already answered from the perspective of the second student.

P8-6 ω= (0.652 rad/s2)tand α= (0.652 rad/s2).

(a) ω= (0.652 rad/s2)(5.60 s) = 3.65 rad/s

(b) vT=ωr = (3.65 rad/s)(10.4 m) = 38 m/s.

(d) aR=ω2r= (3.65 rad/s)2(10.4 m) = 139 m/s2.

P8-7 (a) ω= (2πrad)/(3.16×107s) = 1.99×10−7rad/s.

(b) vT=ωR = (1.99×10−7rad/s)(1.50×1011m) = 2.99×104m/s.

P8-8 (a) α= (−156 rev/min)/(2.2×60 min) = −1.18 rev/min2.

(b) The average angular speed while slowing down is 78 rev/min, so the wheel turns through

(78 rev/min)(2.2×60 min) = 10300 revolutions.

10−3m/s2.

(d) aR= (2πrad/rev)(72.5 rev/min)2(0.524 m) = 1.73 ×104m/min2. That’s the same as

4.81m/s2. This is so much larger than the aTterm that the magnitude of the total linear ac-

celeration is simply 4.81m/s2.

P8-9 (a) There are 500 teeth (and 500 spaces between these teeth); so disk rotates 2π/500 rad

between the outgoing light pulse and the incoming light pulse. The light traveled 1000 m, so the

elapsed time is t= (1000 m)/(3×108m/s) = 3.33×10−6s.

Then the angular speed of the disk is ωz=φ/t = 1.26×10−2rad)/(3.33×10−6s) = 3800 rad/s.

(b) The linear speed of a point on the edge of the would be

vT=ωR = (3800 rad/s)(0.05 m) = 190 m/s.

P8-10 The linear acceleration of the belt is a=αArA. The angular acceleration of Cis αC=

a/rC=αA(rA/rC). The time required for Cto get up to speed is

t=(2πrad/rev)(100 rev/min)(1/60 min/s)

(1.60 rad/s2)(10.0/25.0) = 16.4 s.

P8-11 (a) The ﬁnal angular speed is ωo= (130 cm/s)/(5.80 cm) = 22.4 rad/s.

(b) The recording area is π(Ro2−Ri2), the recorded track has a length land width w, so

l=π[(5.80 cm)2−(2.50 cm2)]

(1.60×10−4cm) = 5.38×105cm.

102

P8-12 The angular position is given by φ= arctan(vt/b). The derivative (Maple!) is

ω=vb

b2+v2t2,

and is directed up. Take the derivative again,

α=2bv3t

(b2+v2t2)2,

but is directed down.

P8-13 (a) Let the rocket sled move along the line x=b. The observer is at the origin and

sees the rocket move with a constant angular speed, so the angle made with the xaxis increases

according to θ=ωt. The observer, rocket, and starting point form a right triangle; the position y

of the rocket is the opposite side of this triangle, so

tan θ=y/b implies y=b/ tan ωt.

We want to take the derivative of this with respect to time and get

v(t) = ωb/ cos2(ωt).

(b) The speed becomes inﬁnite (which is clearly unphysical) when t=π/2ω.

103

E9-1 (a) First, ~

F= (5.0 N)ˆ

~τ = [yFz−zFy]ˆ

i+ [zFx−xFz]ˆ

j+ [xFy−yFx]ˆ

= [y(0) −(0)(0)]ˆ

i+ [(0)Fx−x(0)]ˆ

j+ [x(0) −yFx]ˆ

= [−yFx]ˆ

k=−(3.0 m)(5.0 N)ˆ

k=−(15.0 N ·m)ˆ

(b) Now ~

F= (5.0 N)ˆ

j. Ignoring all zero terms,

~τ = [xFy]ˆ

k= (2.0 m)(5.0 N)ˆ

k= (10 N ·m)ˆ

F= (−5.0 N)ˆ

~τ = [−yFx]ˆ

k=−(3.0 m)(−5.0 N)ˆ

k= (15.0 N ·m)ˆ

E9-2 (a) Everything is in the plane of the page, so the net torque will either be directed normal

to the page. Let out be positive, then the net torque is τ=r1F1sin θ1−r2F2sin θ2.

(b) τ= (1.30 m)(4.20 N) sin(75.0◦)−(2.15 m)(4.90 N) sin(58.0◦) = −3.66N ·m.

E9-4 Everything is in the plane of the page, so the net torque will either be directed normal to

the page. Let out be positive, then the net torque is

τ= (8.0 m)(10 N) sin(45◦)−(4.0 m)(16 N) sin(90◦) + (3.0 m)(19 N) sin(20◦) = 12N ·m.

E9-5 Since ~

rand ~

slie in the xy plane, then ~

t=~

r×~

smust be perpendicular to that plane, and

can only point along the zaxis.

The angle between ~

rand ~

sis 320◦−85◦= 235◦. So |~

t|=rs|sin θ|= (4.5)(7.3)|sin(235◦)|= 27.

Now for the direction of ~

t. The smaller rotation to bring ~

rinto ~

sis through a counterclockwise

rotation; the right hand rule would then show that the cross product points along the positive z

direction.

E9-6 ~

a= (3.20)[cos(63.0◦)ˆ

j+ sin(63.0◦)ˆ

k] and ~

b= (1.40)[cos(48.0◦)ˆ

i+ sin(48.0◦)ˆ

k]. Then

a×~

b= (3.20) cos(63.0◦)(1.40) sin(48.0◦)ˆ

+(3.20) sin(63.0◦)(1.40) cos(48.0◦)ˆ

−(3.20) cos(63.0◦)(1.40) cos(48.0◦)ˆ

= 1.51ˆ

i+ 2.67ˆ

j−1.36ˆ

E9-7 ~

b×~

ahas magnitude ab sin φand points in the negative zdirection. It is then perpendicular

to ~

a, so ~

chas magnitude a2bsin φ. The direction of ~

cis perpendicular to ~

abut lies in the plane

containing vectors ~

aand ~

b. Then it makes an angle π/2−φwith ~

E9-8 (a) In unit vector notation,

c= [(−3)(−3) −(−2)(1)]ˆ

i+ [(1)(4) −(2)(−3)]ˆ

j+ [(2)(−2) −(−3)(4)]ˆ

= 11ˆ

i+ 10ˆ

j+ 8ˆ

(b) Evaluate arcsin[|~

a×b|/(ab)], ﬁnding magnitudes with the Pythagoras relationship:

φ= arcsin (16.8)/[(3.74)(5.39)] = 56◦.

104

E9-9 This exercise is a three dimensional generalization of Ex. 9-1, except nothing is zero.

~τ = [yFz−zFy]ˆ

i+ [zFx−xFz]ˆ

j+ [xFy−yFx]ˆ

= [(−2.0 m)(4.3 N) −(1.6 m)(−2.4 N)]ˆ

i+ [(1.6 m)(3.5 N) −(1.5 m)(4.3 N)]ˆ

+[(1.5 m)(−2.4 N) −(−2.0 m)(3.5 N)]ˆ

= [−4.8 N·m]ˆ

i+ [−0.85 N·m]ˆ

j+ [3.4 N·m]ˆ

E9-10 (a) ~

F= (2.6 N)ˆ

i, then ~τ = (0.85 m)(2.6 N)ˆ

j−(−0.36 m)(2.6 N)ˆ

k= 2.2 N ·mˆ

j+ 0.94 N ·mˆ

(b) ~

F= (−2.6 N)ˆ

k, then ~τ = (−0.36 m)(−2.6 N)ˆ

i−(0.54 m)(−2.6 N)ˆ

j= 0.93 N ·mˆ

i+ 1.4 N ·mˆ

E9-11 (a) The rotational inertia about an axis through the origin is

I=mr2= (0.025 kg)(0.74 m)2= 1.4×10−2kg ·m2.

(b) α= (0.74 m)(22 N) sin(50◦)/(1.4×10−2kg ·m2) = 890 rad/s.

E9-12 (a) I0= (0.052 kg)(0.27 m)2+ (0.035 kg)(0.45 m)2+ (0.024 kg)(0.65 m)2= 2.1×10−2kg ·m2.

(b) The center of mass is located at

xcm =(0.052 kg)(0.27 m) + (0.035 kg)(0.45 m) + (0.024 kg)(0.65 m)

(0.052 kg) + (0.035 kg) + (0.024 kg) = 0.41 m.

Applying the parallel axis theorem yields Icm = 2.1×10−2kg ·m2−(0.11 kg)(0.41 m)2= 2.5×10−3kg ·

m2.

E9-13 (a) Rotational inertia is additive so long as we consider the inertia about the same axis.

We can use Eq. 9-10:

I=Xmnr2

n= (0.075 kg)(0.42 m)2+ (0.030 kg)(0.65 m)2= 0.026 kg·m2.

(b) No change.

E9-14 ~τ = [(0.42 m)(2.5 N) −(0.65 m)(3.6 N)]ˆ

k=−1.29 N ·mˆ

k. Using the result from E9-13,

~α = (−1.29 N ·mˆ

k)/(0.026 kg·m2) = 50 rad/s2ˆ

k.That’s clockwise if viewed from above.

E9-15 (a) F=mω2r= (110 kg)(33.5 rad/s)2(3.90 m) = 4.81×105N.

(b) The angular acceleration is α= (33.5 rad/s)/(6.70 s) = 5.00 rad/s2. The rotational inertia

about the axis of rotation is I= (110 kg)(7.80 m)2/3=2.23×103kg ·m2. τ =Iα = (2.23×103kg ·

m2)(5.00 rad/s2) = 1.12×104N·m.

E9-16 We can add the inertias for the three rods together,

I= 3 1

3ML2= (240 kg)(5.20 m)2= 6.49×103kg ·m2.

E9-17 The diagonal distance from the axis through the center of mass and the axis through the

edge is h=p(a/2)2+ (b/2)2, so

I=Icm +Mh2=1

12Ma2+b2+M(a/2)2+ (b/2)2=1

12 +1

4Ma2+b2.

Simplifying, I=1

3Ma2+b2.

105

E9-18 I=Icm +Mh2= (0.56 kg)(1.0 m)2/12 + (0.56)(0.30 m)2= 9.7×10−2kg ·m2.

E9-19 For particle one I1=mr2=mL2; for particle two I2=mr2=m(2L)2= 4mL2. The

rotational inertia of the rod is Irod =1

3(2M)(2L)2=8

3ML2. Add the three inertias:

I=5m+8

3ML2.

E9-20 (a) I=MR2/2 = M(R/√2)2.

(b) Let Ibe the rotational inertia. Assuming that kis the radius of a hoop with an equivalent

rotational inertia, then I=Mk2, or k=pI/M.

E9-21 Note the mistakes in the equation in the part (b) of the exercise text.

(a) mn=M/N.

(b) Each piece has a thickness t=L/N, the distance from the end to the nth piece is xn=

(n−1/2)t= (n−1/2)L/N. The axis of rotation is the center, so the distance from the center is

rn=xn−L/2 = nL/N −(1 + 1/2N)L.

n=1

mnr2

=ML2

n=1

(n−1/2−N)2,

=ML2

n=1 n2−(2N+ 1)n+ (N+ 1/2)2,

=ML2

N3N(N+ 1)(2N+ 1)

6−(2N+ 1)N(N+ 1)

2+ (N+ 1/2)2N,

≈ML2

N32N3

6−2N3

2+N3,

=ML2/3.

E9-22 F= (46 N)(2.6 cm)/(13 cm) = 9.2 N.

E9-23 Tower topples when center of gravity is no longer above base. Assuming center of gravity

is located at the very center of the tower, then when the tower leans 7.0 m then the tower falls. This

is 2.5 m farther than the present.

(b) θ= arcsin(7.0 m/55 m) = 7.3◦.

E9-24 If the torque from the force is suﬃcient to lift edge the cube then the cube will tip. The

net torque about the edge which stays in contact with the ground will be τ=F d −mgd/2 if Fis

suﬃciently large. Then F≥mg/2 is the minimum force which will cause the cube to tip.

The minimum force to get the cube to slide is F≥µsmg = (0.46)mg. The cube will slide ﬁrst.

106

E9-25 The ladder slips if the force of static friction required to keep the ladder up exceeds µsN.

Equations 9-31 give us the normal force in terms of the masses of the ladder and the ﬁreﬁghter,

N= (m+M)g, and is independent of the location of the ﬁreﬁghter on the ladder. Also from Eq.

9-31 is the relationship between the force from the wall and the force of friction; the condition at

which slipping occurs is Fw≥µs(m+M)g.

Now go straight to Eq. 9-32. The a/2 in the second term is the location of the ﬁreﬁghter, who in

the example was halfway between the base of the ladder and the top of the ladder. In the exercise

we don’t know where the ﬁreﬁghter is, so we’ll replace a/2 with x. Then

−Fwh+Mgx +mga

3= 0

is an expression for rotational equilibrium. Substitute in the condition of Fwwhen slipping just

starts, and we get

−(µs(m+M)g)h+Mgx +mga

3= 0.

Solve this for x,

x=µsm

M+ 1h−ma

3M= (0.54) 45 kg

72 kg + 1(9.3 m) −(45 kg)(7.6 m)

3(72 kg) = 6.6 m

This is the horizontal distance; the fraction of the total length along the ladder is then given by

x/a = (6.6 m)/(7.6 m) = 0.87. The ﬁreﬁghter can climb (0.87)(12 m) = 10.4 m up the ladder.

E9-26 (a) The net torque about the rear axle is (1360 kg)(9.8 m/s2)(3.05 m−1.78 m)−Ff(3.05 m) =

0, which has solution Ff= 5.55×103N. Each of the front tires support half of this, or 2.77×103N.

(b) The net torque about the front axle is (1360 kg)(9.8 m/s2)(1.78 m) −Ff(3.05 m) = 0, which

has solution Ff= 7.78×103N. Each of the front tires support half of this, or 3.89×103N.

E9-27 The net torque on the bridge about the end closest to the person is

(160 lb)L/4 + (600 lb)L/2−FfL= 0,

which has a solution for the supporting force on the far end of Ff= 340 lb.

The net force on the bridge is (160 lb)L/4 + (600 lb)L/2−(340 lb) −Fc= 0, so the force on the

close end of the bridge is Fc= 420 lb.

E9-28 The net torque on the board about the left end is

Fr(1.55 m) −(142 N)(2.24 m) −(582 N)(4.48 m) = 0,

which has a solution for the supporting force for the right pedestal of Fr= 1890 N. The force on the

board from the pedestal is up, so the force on the pedestal from the board is down (compression).

The net force on the board is Fl+(1890 N)−(142 N)−(582 N) = 0, so the force from the pedestal

on the left is Fl=−1170 N. The negative sign means up, so the pedestal is under tension.

E9-29 We can assume that both the force ~

Fand the force of gravity ~

Wact on the center of the

wheel. Then the wheel will just start to lift when

W×~

r+~

F×~

r= 0,

Wsin θ=Fcos θ,

107

where θis the angle between the vertical (pointing down) and the line between the center of the

wheel and the point of contact with the step. The use of the sine on the left is a straightforward

application of Eq. 9-2. Why the cosine on the right? Because

sin(90◦−θ) = cos θ.

Then F=Wtan θ. We can express the angle θin terms of trig functions, h, and r.rcos θis the

vertical distance from the center of the wheel to the top of the step, or r−h. Then

cos θ= 1 −h

rand sin θ=s1−1−h

r2

Finally by combining the above we get

F=Wq2h

r−h2

1−h

=W√2hr −h2

r−h.

E9-30 (a) Assume that each of the two support points for the square sign experience the same

tension, equal to half of the weight of the sign. The net torque on the rod about an axis through

the hinge is

(52.3 kg/2)(9.81 m/s2)(0.95 m) + (52.3 kg/2)(9.81 m/s2)(2.88 m) −(2.88 m)Tsin θ= 0,

where Tis the tension in the cable and θis the angle between the cable and the rod. The angle can

be found from θ= arctan(4.12 m/2.88 m) = 55.0◦, so T= 416 N.

(b) There are two components to the tension, one which is vertical, (416 N) sin(55.0◦) = 341 N,

and another which is horizontal, (416 N) cos(55.0◦) = 239 N. The horizontal force exerted by the wall

must then be 239 N. The net vertical force on the rod is F+ (341 N) −(52.3 kg/2)(9.81 m/s2) = 0,

which has solution F= 172 N as the vertical upward force of the wall on the rod.

E9-31 (a) The net torque on the rod about an axis through the hinge is

τ=W(L/2) cos(54.0◦)−T L sin(153.0◦) = 0.

or T= (52.7 lb/2)(sin 54.0◦/sin 153.0◦) = 47.0 lb.

(b) The vertical upward force of the wire on the rod is Ty=Tcos(27.0◦). The vertical upward

force of the wall on the rod is Py=W−Tcos(27.0◦), where Wis the weight of the rod. Then

Py= (52.7 lb) −(47.0 lb) cos(27.0◦) = 10.8 lb

The horizontal force from the wall is balanced by the horizontal force from the wire. Then Px=

(47.0 lb) sin(27.0◦) = 21.3 lb.

E9-32 If the ladder is not slipping then the torque about an axis through the point of contact with

the ground is

τ= (W L/2) cos θ−Nh/ sin θ= 0,

where Nis the normal force of the edge on the ladder. Then N=W L cos θsin θ /(2h).

Nhas two components; one which is vertically up, Ny=Ncos θ, and another which is horizontal,

Nx=Nsin θ. The horizontal force must be oﬀset by the static friction.

The normal force on the ladder from the ground is given by

Ng=W−Ncos θ=W[1 −Lcos2θsin θ /(2h)].

108

The force of static friction can be as large as f=µsNg, so

µs=W L cos θsin2θ /(2h)

W[1 −Lcos2θsin θ /(2h)] =Lcos θsin2θ

2h−Lcos2θsin θ.

Put in the numbers and θ= 68.0◦. Then µs= 0.407.

E9-33 Let out be positive. The net torque about the axis is then

τ= (0.118 m)(5.88 N) −(0.118 m)(4.13 m) −(0.0493 m)(2.12 N) = 0.102 N ·m.

The rotational inertia of the disk is I= (1.92 kg)(0.118 m)2/2 = 1.34 ×10−2kg ·m2. Then α=

(0.102 N ·m)/(1.34×10−2kg ·m2) = 7.61 rad/s2.

E9-34 (a) I=τ/α = (960 N ·m)/(6.23 rad/s2) = 154 kg ·m2.

(b) m= (3/2)I/r2= (1.5)(154 kg ·m2)/(1.88 m)2= 65.4 kg.

E9-35 (a) The angular acceleration is

α=∆ω

∆t=6.20 rad/s

0.22 s = 28.2 rad/s2

(b) From Eq. 9-11, τ=Iα = (12.0 kg·m2)(28.2 rad/s2) = 338 N·m.

E9-36 The angular acceleration is α= 2(π/2 rad)/(30 s)2= 3.5×10−3rad/s2. The required force

is then

F=τ/r =Iα/r = (8.7×104kg ·m2)(3.5×10−3rad/s2)/(2.4 m) = 127 N.

Don’t let the door slam...

E9-37 The torque is τ=rF , the angular acceleration is α=τ/I =rF/I. The angular velocity is

ω=Zt

α dt =rAt2

2I+rBt3

3I,

so when t= 3.60 s,

ω=(9.88×10−2m)(0.496 N/s)(3.60 s)2

2(1.14×10−3kg ·m2)+(9.88×10−2m)(0.305 N/s2)(3.60 s)3

3(1.14×10−3kg ·m2)= 690 rad/s.

E9-38 (a) α= 2θ/t2.

(b) a=αR = 2θR/t2.

Then

T1=Mg −2MRθ/t2,

and

T2=Mg −2MRθ/t2−2(I/R)θ/t2.

109

E9-39 Apply a kinematic equation from chapter 2 to ﬁnd the acceleration:

y=v0yt+1

2ayt2,

ay=2y

t2=2(0.765 m)

(5.11 s)2= 0.0586 m/s2

Closely follow the approach in Sample Problem 9-10. For the heavier block, m1= 0.512 kg, and

Newton’s second law gives

m1g−T1=m1ay,

where ayis positive and down. For the lighter block, m2= 0.463 kg, and Newton’s second law gives

T2−m2g=m2ay,

where ayis positive and up. We do know that T1> T2; the net force on the pulley creates a torque

which results in the pulley rotating toward the heavier mass. That net force is T1−T2; so the

rotational form of Newton’s second law gives

(T1−T2)R=Iαz=IaT/R,

where R= 0.049 m is the radius of the pulley and aTis the tangential acceleration. But this

acceleration is equal to ay, because everything— both blocks and the pulley— are moving together.

We then have three equations and three unknowns. We’ll add the ﬁrst two together,

m1g−T1+T2−m2g=m1ay+m2ay,

T1−T2= (g−ay)m1−(g+ay)m2,

and then combine this with the third equation by substituting for T1−T2,

(g−ay)m1−(g+ay)m2=Iay/R2,

g

ay−1m1−g

+ 1m2R2=I.

Now for the numbers:

(9.81 m/s2)

(0.0586 m/s2)−1(0.512 kg) −(9.81 m/s2)

(0.0586 m/s2)+ 1(0.463 kg) = 7.23 kg,

(7.23 kg)(0.049 m)2= 0.0174 kg·m2.

E9-40 The wheel turns with an initial angular speed of ω0= 88.0 rad/s. The average speed while

decelerating is ωav =ω0/2. The wheel stops turning in a time t=φ/ωav = 2φ/ω0. The deceleration

is then α=−ω0/t =−ω2

0/(2φ).

The rotational inertia is I=MR2/2, so the torque required to stop the disk is τ=Iα =

−MR2ω2

0/(4φ).The force of friction on the disk is f=µN, so τ=Rf. Then

µ=MRω2

4Nφ =(1.40 kg)(0.23 m)(88.0 rad/s)2

4(130 N)(17.6 rad) = 0.272.

E9-41 (a) The automobile has an initial speed of v0= 21.8 m/s. The angular speed is then

ω0= (21.8 m/s)/(0.385 m) = 56.6 rad/s.

(b) The average speed while decelerating is ωav =ω0/2. The wheel stops turning in a time

t=φ/ωav = 2φ/ω0. The deceleration is then

α=−ω0/t =−ω2

0/(2φ) = −(56.6 rad/s)2/[2(180 rad)] = −8.90 rad/s.

110

E9-42 (a) The angular acceleration is derived in Sample Problem 9-13,

α=g

1 + I/(MR2

0)=(981 cm/s2)

(0.320 cm)

1 + (0.950 kg ·cm2)/[(0.120 kg)(0.320 cm)2]= 39.1 rad/s2.

The acceleration is a=αR0= (39.1 rad/s2)(0.320 cm) = 12.5 cm/s2.

(b) Starting from rest, t=p2x/a =q2(134 cm)/(12.5 cm/s2) = 4.63 s.

(d) The yo-yo accelerates toward the ground according to y=at2+v0t, where down is positive.

The time required to move to the end of the string is found from

t=−v0+pv2

0+ 4ay

2a=−(1.30 m/s) + p(1.30 m/s)2+ 4(0.125 m/s2)(1.34 m)

2(0.125 m/s2)= 0.945 s

The initial rotational speed was ω0= (1.30 m/s)/(3.2×10−3m) = 406 rad/s. Then

ω=ω0+αt = (406 rad/s) + (39.1 rad/s2)(0.945 s) = 443 rad/s,

which is the same as 70.5 rev/s.

E9-43 (a) Assuming a perfect hinge at B, the only two vertical forces on the tire will be the

normal force from the belt and the force of gravity. Then N=W=mg, or N= (15.0 kg)(9.8

m/s2) = 147 N.

While the tire skids we have kinetic friction, so f=µkN= (0.600)(147 N) = 88.2 N. The force

of gravity and and the pull from the holding rod AB both act at the axis of rotation, so can’t

contribute to the net torque. The normal force acts at a point which is parallel to the displacement

from the axis of rotation, so it doesn’t contribute to the torque either (because the cross product

would vanish); so the only contribution to the torque is from the frictional force.

The frictional force is perpendicular to the radial vector, so the magnitude of the torque is just

τ=rf = (0.300 m)(88.2 N) = 26.5 N·m. This means the angular acceleration will be α=τ/I =

(26.5 N·m)/(0.750 kg·m2) = 35.3 rad/s2.

When ωR =vT= 12.0 m/s the tire is no longer slipping. We solve for ωand get ω= 40 rad/s.

Now we solve ω=ω0+αt for the time. The wheel started from rest, so t= 1.13 s.

(b) The length of the skid is x=vt = (12.0 m/s)(1.13 s) = 13.6 m long.

P9-1 The problem of sliding down the ramp has been solved (see Sample Problem 5-8); the

critical angle θsis given by tan θs=µs.

The problem of tipping is actually not that much harder: an object tips when the center of

gravity is no longer over the base. The important angle for tipping is shown in the ﬁgure below; we

can ﬁnd that by trigonometry to be

tan θt=O

A=(0.56 m)

(0.56 m) + (0.28 m) = 0.67,

so θt= 34◦.

111

(a) If µs= 0.60 then θs= 31◦and the crate slides.

(b) If µs= 0.70 then θs= 35◦and the crate tips before sliding; it tips at 34◦.

P9-2 (a) The total force up on the chain needs to be equal to the total force down; the force

down is W. Assuming the tension at the end points is Tthen Tsin θis the upward component, so

T=W/(2 sin θ).

(b) There is a horizontal component to the tension Tcos θat the wall; this must be the tension

at the horizontal point at the bottom of the cable. Then Tbottom =W/(2 tan θ).

P9-3 (a) The rope exerts a force on the sphere which has horizontal Tsin θand vertical Tcos θ

components, where θ= arctan(r/L). The weight of the sphere is balanced by the upward force from

the rope, so Tcos θ=W. But cos θ=L/√r2+L2, so T=Wp1 + r2/L2.

(b) The wall pushes outward against the sphere equal to the inward push on the sphere from the

rope, or P=Tsin θ=Wtan θ=W r/L.

P9-4 Treat the problem as having two forces: the man at one end lifting with force F=W/3

and the two men acting together a distance xaway from the ﬁrst man and lifting with a force

2F= 2W/3. Then the torque about an axis through the end of the beam where the ﬁrst man is

lifting is τ= 2xW/3−W L/2, where Lis the length of the beam. This expression equal zero when

x= 3L/4.

P9-5 (a) We can solve this problem with Eq. 9-32 after a few modiﬁcations. We’ll assume the

center of mass of the ladder is at the center, then the third term of Eq. 9-32 is mga/2. The cleaner

didn’t climb half-way, he climbed 3.10/5.12 = 60.5% of the way, so the second term of Eq. 9-32

becomes Mga(0.605). h,L, and aare related by L2=a2+h2, so h=p(5.12 m)2−(2.45 m)2= 4.5

m. Then, putting the correction into Eq. 9-32,

Fw=1

hhMga(0.605) + mga

2i,

(4.5 m) h(74.6 kg)(9.81 m/s2)(2.45 m)(0.605) ,

+ (10.3 kg)(9.81 m/s2)(2.45 m)/2i,

= 269 N

(b) The vertical component of the force of the ground on the ground is the sum of the weight of

the window cleaner and the weight of the ladder, or 833 N.

112

The horizontal component is equal in magnitude to the force of the ladder on the window. Then

the net force of the ground on the ladder has magnitude

p(269 N)2+ (833 N)2= 875 N

and direction

θ= arctan(833/269) = 72◦above the horizontal.

P9-6 (a) There are no upward forces other than the normal force on the bottom ball, so the force

exerted on the bottom ball by the container is 2W.

weight of the top ball. Then W=Nsin θor the force of contact between the balls is N=W/ sin θ.

(b) The force of contact between the balls has a horizontal component P=Ncos θ=W/ tan θ,

this must also be the force of the walls on the balls.

P9-7 (a) There are three forces on the ball: weight ~

W, the normal force from the lower plane ~

N1,

and the normal force from the upper plane ~

N2. The force from the lower plane has components

N1,x =−N1sin θ1and N1,y =N1cos θ1. The force from the upper plane has components N2,x =

N2sin θ2and N2,y =−N2cos θ2. Then N1sin θ1=N2sin θ2and N1cos θ1=W+N2cos θ2.

Solving for N2by dividing one expression by the other,

cos θ1

sin θ1

N2sin θ2

+cos θ2

sin θ2

N2=W

sin θ2cos θ1

sin θ1−cos θ2

sin θ2−1

sin θ2

cos θ1sin θ2−cos θ2sin θ1

sin θ1sin θ2

=Wsin θ1

sin(θ2−θ1).

Then solve for N1,

N1=Wsin θ2

sin(θ2−θ1).

(b) Friction changes everything.

P9-8 (a) The net torque about a line through Ais

τ=W x −T L sin θ= 0,

so T=W x/(Lsin θ).

(b) The horizontal force on the pin is equal in magnitude to the horizontal component of the

tension: Tcos θ=W x/(Ltan θ). The vertical component balances the weight: W−W x/L.

P9-9 (a) As long as the center of gravity of an object (even if combined) is above the base, then

the object will not tip.

Stack the bricks from the top down. The center of gravity of the top brick is L/2 from the edge

of the top brick. This top brick can be oﬀset nor more than L/2 from the one beneath. The center

of gravity of the top two bricks is located at

xcm = [(L/2) + (L)]/2 = 3L/4.

113

These top two bricks can be oﬀset no more than L/4 from the brick beneath. The center of gravity

of the top three bricks is located at

xcm = [(L/2) + 2(L)]/3 = 5L/6.

These top three bricks can be oﬀset no more than L/6 from the brick beneath. The total oﬀset is

then L/2 + L/4 + L/6 = 11L/12.

(b) Actually, we never need to know the location of the center of gravity; we now realize that

each brick is located with an oﬀset L/(2n) with the brick beneath, where nis the number of the

brick counting from the top. The series is then of the form

(L/2)[(1/1) + (1/2) + (1/3) + (1/4) + ···],

a series (harmonic, for those of you who care) which does not converge.

would be at NL/n; the pile will topple when this value exceeds L, or when N=n.

P9-10 (a) For a planar object which lies in the x−yplane, Ix=Rx2dm and Iy=Ry2dm. Then

Ix+Iy=R(x2+y2)dm =Rr2dm. But this is the rotational inertia about the zaxis, sent ris the

distance from the zaxis.

(b) Since the rotational inertia about one diameter (Ix) should be the same as the rotational

inertia about any other (Iy) then Ix=Iyand Ix=Iz/2 = MR2/4.

P9-11 Problem 9-10 says that Ix+Iy=Izfor any thin, ﬂat object which lies only in the

x−yplane.It doesn’t matter in which direction the xand yaxes are chosen, so long as they are

perpendicular. We can then orient our square as in either of the pictures below:

By symmetry Ix=Iyin either picture. Consequently, Ix=Iy=Iz/2 for either picture. It is

the same square, so Izis the same for both picture. Then Ixis also the same for both orientations.

P9-12 Let M0be the mass of the plate before the holes are cut out. Then M1=M0(a/L)2is the

mass of the part cut out of each hole and M=M0−9M1is the mass of the plate. The rotational

inertia (about an axis perpendicular to the plane through the center of the square) for the large

uncut square is M0L2/6 and for each smaller cut out is M1a2/6.

From the large uncut square’s inertia we need to remove M1a2/6 for the center cut-out, M1a2/6+

M1(L/3)2for each of the four edge cut-outs, and M1a2/6 + M1(√2L/3)2for each of the corner

sections.

114

Then

I=M0L2

6−9M1a2

6−4M1L2

9−42M1L2

=M0L2

6−3M0a4

2L2−4M0a2

P9-13 (a) From Eq. 9-15, I=Rr2dm about some axis of rotation when ris measured from that

axis. If we consider the xaxis as our axis of rotation, then r=py2+z2, since the distance to the

xaxis depends only on the yand zcoordinates. We have similar equations for the yand zaxes, so

Ix=Zy2+z2dm,

Iy=Zx2+z2dm,

Iz=Zx2+y2dm.

These three equations can be added together to give

Ix+Iy+Iz= 2 Zx2+y2+z2dm,

so if we now deﬁne rto be measured from the origin (which is not the deﬁnition used above), then

we end up with the answer in the text.

(b) The right hand side of the equation is integrated over the entire body, regardless of how

the axes are deﬁned. So the integral should be the same, no matter how the coordinate system is

rotated.

P9-14 (a) Since the shell is spherically symmetric Ix=Iy=Iz, so Ix= (2/3) Rr2dm =

(2R2/3) Rdm = 2MR2/3.

(b) Since the solid ball is spherically symmetric Ix=Iy=Iz, so

Ix=2

3Zr23Mr2dr

R3=2

5MR2.

P9-15 (a) A simple ratio will suﬃce:

2πr dr =M

πR2or dm =2Mr

R2dr.

(b) dI =r2dm = (2Mr3/R2)dr.

0(2Mr3/R2)dr =MR2/2.

P9-16 (a) Another simple ratio will suﬃce:

πr2dz =M

(4/3)πR3or dm =3M(R2−z2)

4R3dz.

(b) dI =r2dm/2 = [3M(R2−z2)2/8R3]dz.

115

I=ZR

−R

3M(R2−z2)2

8R3dz,

=3M

4R3ZR

(R4−2R2z2+z4)dz,

=3M

4R3(R5−2R5/3 + R5/5) = 2

5MR2.

P9-17 The rotational acceleration will be given by αz=Pτ /I.

The torque about the pivot comes from the force of gravity on each block. This forces will both

originally be at right angles to the pivot arm, so the net torque will be Pτ=mgL2−mgL1, where

clockwise as seen on the page is positive.

The rotational inertia about the pivot is given by I=Pmnr2

n=m(L2

2+L2

1). So we can now

ﬁnd the rotational acceleration,

α=Pτ

I=mgL2−mgL1

m(L2

2+L2

1)=gL2−L1

2+L2

= 8.66 rad/s2.

The linear acceleration is the tangential acceleration, aT=αR. For the left block, aT= 1.73 m/s2;

for the right block aT= 6.93 m/s2.

P9-18 (a) The force of friction on the hub is µkMg. The torque is τ=µkM ga. The angular

acceleration has magnitude α=τ/I =µkga/k2. The time it takes to stop the wheel will be

t=ω0/α =ω0k2/(µkga).

(b) The average rotational speed while slowing is ω0/2. The angle through which it turns while

slowing is ω0t/2 radians, or ω0t/(4π) = ω2

0k2/(4πµkga)

P9-19 (a) Consider a diﬀerential ring of the disk. The torque on the ring because of friction is

dτ =r dF =rµkMg

πR22πr dr =2µkM gr2

R2dr.

The net torque is then

τ=Zdτ =ZR

2µkMgr2

R2dr =2

3µkMgR.

(b) The rotational acceleration has magnitude α=τ/I =4

3µkg/R. Then it will take a time

t=ω0/α =3Rω0

4µkg

to stop.

P9-20 We need only show that the two objects have the same acceleration.

Consider ﬁrst the hoop. There is a force W|| =Wsin θ=mg sin θpulling it down the ramp

and a frictional force fpulling it up the ramp. The frictional force is just large enough to cause

a torque that will allow the hoop to roll without slipping. This means a=αR; consequently,

fR =αI =aI/R. In this case I=mR2.

The acceleration down the plane is

ma =mg sin θ−f=mg sin θ−maI/R2=mg sin θ−ma.

116

Then a=gsin θ /2. The mass and radius are irrelevant!

For a block sliding with friction there are also two forces: W|| =Wsin θ=mg sin θand f=

µkmg cos θ. Then the acceleration down the plane will be given by

a=gsin θ−µkgcos θ,

which will be equal to that of the hoop if

µk=sin θ−sin θ/2

cos θ=1

2tan θ.

P9-21 This problem is equivalent to Sample Problem 9-11, except that we have a sphere instead

of a cylinder. We’ll have the same two equations for Newton’s second law,

Mg sin θ−f=Macm and N−Mg cos θ= 0.

Newton’s second law for rotation will look like

−fR =Icmα.

The conditions for accelerating without slipping are acm =αR, rearrange the rotational equation to

get

f=−Icmα

R=−Icm(−acm)

R2,

and then

Mg sin θ−Icm(acm)

R2=Macm,

and solve for acm. For fun, let’s write the rotational inertia as I=βM R2, where β= 2/5 for the

sphere. Then, upon some mild rearranging, we get

acm =gsin θ

1 + β

For the sphere, acm = 5/7gsin θ.

(a) If acm = 0.133g, then sin θ= 7/5(0.133) = 0.186, and θ= 10.7◦.

(b) A frictionless block has no rotational properties; in this case β= 0! Then acm =gsin θ=

0.186g.

P9-22 (a) There are three forces on the cylinder: gravity Wand the tension from each cable T.

The downward acceleration of the cylinder is then given by ma =W−2T.

The ropes unwind according to α=a/R, but α=τ/I and I=mR2/2. Then

a=τR/I = (2T R)R/(mR2/2) = 4T/m.

Combining the above, 4T=W−2T, or T=W/6.

(b) a= 4(mg/6)/m = 2g/3.

P9-23 The force of friction required to keep the cylinder rolling is given by

f=1

3Mg sin θ;

the normal force is given to be N=Mg cos θ; so the coeﬃcient of static friction is given by

µs≥f

N=1

3tan θ.

117

P9-24 a=F/M, since Fis the net force on the disk. The torque about the center of mass is F R,

so the disk has an angular acceleration of

α=F R

I=F R

MR2/2=2F

M R .

P9-25 This problem is equivalent to Sample Problem 9-11, except that we have an unknown rolling

object. We’ll have the same two equations for Newton’s second law,

Mg sin θ−f=Macm and N−Mg cos θ= 0.

Newton’s second law for rotation will look like

−fR =Icmα.

The conditions for accelerating without slipping are acm =αR, rearrange the rotational equation to

get

f=−Icmα

R=−Icm(−acm)

R2,

and then

Mg sin θ−Icm(acm)

R2=Macm,

and solve for acm. Write the rotational inertia as I=βMR2, where β= 2/5 for a sphere, β= 1/2

for a cylinder, and β= 1 for a hoop. Then, upon some mild rearranging, we get

acm =gsin θ

1 + β

Note that ais largest when βis smallest; consequently the cylinder wins. Neither Mnor Rentered

into the ﬁnal equation.

118

E10-1 l=rp =mvr = (13.7×10−3kg)(380 m/s)(0.12 m) = 0.62 kg ·m2/s.

E10-2 (a) ~

l=m~

r×~

v, or

l=m(yvz−zvy)ˆ

i+m(zvx−xvz)ˆ

j+m(xvy−yvx)ˆ

(b) If ~

vand ~

rexist only in the xy plane then z=vz= 0, so only the uk term survives.

E10-3 If the angular momentum ~

lis constant in time, then d

l/dt = 0. Trying this on Eq. 10-1,

dt =d

dt (~

r×~

p),

dt (~

r×m~

v),

=md~

dt ×~

v+m~

r×d~

dt ,

=m~

v×~

v+m~

r×~

Now the cross product of a vector with itself is zero, so the ﬁrst term vanishes. But in the exercise

we are told the particle has constant velocity, so ~

a= 0, and consequently the second term vanishes.

Hence, ~

lis constant for a single particle if ~

vis constant.

E10-4 (a) L=Pli;li=rimivi. Putting the numbers in for each planet and then summing (I

won’t bore you with the arithmetic details) yields L= 3.15×1043kg ·m2/s.

(b) Jupiter has l= 1.94×1043kg ·m2/s,which is 61.6% of the total.

E10-5 l=mvr =m(2πr/T )r= 2π(84.3 kg)(6.37×106m)2/(86400 s) = 2.49×1011kg ·m2/s.

E10-6 (a) Substitute and expand:

L=X(~

rcm +~

i)×(mi~

vcm +~

i),

=X(mi~

rcm ×~

vcm +~

rcm ×~

i+mi~

i×~

vcm +~

i×~

i),

=M~

rcm ×~

vcm +~

rcm ×(X~

i)+(Xmi~

i)×~

vcm +X~

i×~

(b) But P~

i= 0 and Pmi~

i= 0, because these two quantities are in the center of momentum

and center of mass. Then

L=M~

rcm ×~

vcm +X~

i×~

i=~

L0+M~

rcm ×~

vcm.

E10-7 (a) Substitute and expand:

i=mi

dt =mi

dt −mi

rcm

dt =~

pi−mi~

vcm.

(b) Substitute and expand:

dt =Xd~

dt ×~

i+X~

i×d~

dt =X~

i×d~

dt .

The ﬁrst term vanished because ~

iis parallel to ~

119

dt =X~

i×d(~

pi−mi~

vcm)

dt ,

=X~

i×(mi~

ai−mi~

acm),

=X~

i×mi~

ai+Xmi~

i×~

acm)

The second term vanishes because of the deﬁnition of the center of mass. Then

dt =X~

i×~

Fi,

where ~

Fiis the net force on the ith particle. The force ~

Fimay include both internal and external

components. If there is an internal component, say between the ith and jth particles, then the

torques from these two third law components will cancel out. Consequently,

dt =X~τi=~τext.

E10-8 (a) Integrate.

Z~τ dt =Zd~

dt dt =Zd~

L= ∆~

(b) If Iis ﬁxed, ∆L=I∆ω. Not only that,

Zτdt =ZF r dt =rZF dt =rF av∆t,

where we use the deﬁnition of average that depends on time.

E10-9 (a) ~τ∆t= ∆

l.The disk starts from rest, so ∆

l=~

l−~

l0=~

l. We need only concern

ourselves with the magnitudes, so

l= ∆l=τ∆t= (15.8 N·m)(0.033 s) = 0.521 kg·m2/s.

(b) ω=l/I = (0.521 kg·m2/s)/(1.22×10−3kg·m2) = 427 rad/s.

E10-10 (a) Let v0be the initial speed; the average speed while slowing to a stop is v0/2; the time

required to stop is t= 2x/v0; the acceleration is a=−v0/t =−v2

0/(2x). Then

a=−(43.3 m/s)2/[2(225 m/s)] = −4.17 m/s2.

(b) α=a/r = (−4.17 m/s2)/(0.247 m) = −16.9 rad/s2.

E10-11 Let ~

ri=~

z+~

i.From the ﬁgure, ~

p1=−~

p2and ~

1=−~

2. Then

L=~

l1+~

l2=~

r1×~

p1+~

r2×~

p2,

= (~

r1−~

r2)×~

p1,

= (~

1−~

2)×~

p1,

= 2~

1×~

p1.

Since ~

1and ~

p1both lie in the xy plane then ~

Lmust be along the zaxis.

120

E10-12 Expand:

L=X~

li=X~

ri×~

pi,

=Xmi~

ri×~

vi=Xmi~

ri×(~ω ×~

ri)

=Xmi[(~

ri·~

ri)~ω −(~

ri·~ω)~

r)i],

=Xmi[r2

i~ω −(z2

iω)ˆ

k−(zixiω)ˆ

i−(ziyiω)ˆ

j],

but if the body is symmetric about the zaxis then the last two terms vanish, leaving

L=Xmi[r2

i~ω −(z2

iω)ˆ

k] = Xmi(x2

i+y2

i)~ω =I~ω.

E10-13 An impulse of 12.8 N·s will change the linear momentum by 12.8 N·s; the stick starts

from rest, so the ﬁnal momentum must be 12.8 N·s. Since p=mv, we then can ﬁnd v=p/m = (12.8

N·s)/(4.42 kg) = 2.90 m/s.

Impulse is a vector, given by R~

Fdt. We can take the cross product of the impulse with the

displacement vector ~

r(measured from the axis of rotation to the point where the force is applied)

and get

r×Z~

Fdt ≈Z~

r×~

Fdt,

The two sides of the above expression are only equal if ~

rhas a constant magnitude and direction. This

won’t be true, but if the force is of suﬃciently short duration then it hopefully won’t change much.

The right hand side is an integral over a torque, and will equal the change in angular momentum of

the stick.

The exercise states that the force is perpendicular to the stick, then |~

r×~

F|=rF , and the “torque

impulse” is then (0.464 m)(12.8 N·s) = 5.94 kg·m/s. This “torque impulse” is equal to the change

in the angular momentum, but the stick started from rest, so the ﬁnal angular momentum of the

stick is 5.94 kg·m/s.

But how fast is it rotating? We can use Fig. 9-15 to ﬁnd the rotational inertia about the center

of the stick: I=1

12 ML2=1

12 (4.42 kg)(1.23 m)2= 0.557 kg·m2. The angular velocity of the stick is

ω=l/I = (5.94 kg·m/s)/(0.557 kg·m2) = 10.7 rad/s.

E10-14 The point of rotation is the point of contact with the plane; the torque about that point

is τ=rmg sin θ. The angular momentum is Iω, so τ=Iα. In this case I=mr2/2 + mr2, the

second term from the parallel axis theorem. Then

a=rα =rτ /I =mr2gsin θ/(3mr2/2) = 2

3gsin θ.

E10-15 From Exercise 8 we can immediately write

I1(ω1−ω0)/r1=I2(ω2−0)/r2,

but we also have r1ω1=−r2ω2. Then

ω2=−r1r2I1ω0

1I2−r2

2I1

121

E10-16 (a) ∆ω/ω = (1/T1−1/T2)/(1/T1) = −(T2−T1)/T2=−∆T/T , which in this case is

−(6.0×10−3s)(8.64×104s) = −6.9×10−8.

(b) Assuming conservation of angular momentum, ∆I/I =−∆ω/ω. Then the fractional change

would be 6.9×10−8.

E10-17 The rotational inertia of a solid sphere is I=2

5MR2; so as the sun collapses

Li=~

Lf,

Ii~ωi=If~ωf,

5MRi2~ωi=2

5MRf2~ωf,

Ri2~ωi=Rf2~ωf.

The angular frequency is inversely proportional to the period of rotation, so

Tf=Ti

Rf2

Ri2= (3.6×104min) (6.37×106m)

(6.96×108m) 2

= 3.0 min.

E10-18 The ﬁnal angular velocity of the train with respect to the tracks is ωtt =Rv. The

conservation of angular momentum implies

0 = MR2ω+mR2(ωtt +ω),

ω=−mv

(m+M)R.

E10-19 This is much like a center of mass problem.

0 = Ipφp+Im(φmp +φp),

φmp =−(Ip+Im)φp

Im≈ − (12.6 kg ·m2)(25◦)

(2.47×10−3kg ·m2)= 1.28×105◦.

That’s 354 rotations!

E10-20 ωf= (Ii/If)ωi= [(6.13 kg ·m2)/(1.97 kg ·m2)](1.22 rev/s) = 3.80 rev/s.

E10-21 We have two disks which are originally not in contact which then come into contact;

there are no external torques. We can write

l1,i+~

l2,i=~

l1,f+~

l2,f,

I1~ω1,i+I2~ω2,i=I1~ω1,f+I2~ω2,f.

The ﬁnal angular velocities of the two disks will be equal, so the above equation can be simpliﬁed

and rearranged to yield

ωf=I1

I1+I2

ω1,i=(1.27 kg ·m2)

(1.27 kg ·m2) + (4.85 kg ·m2)(824 rev/min) = 171 rev/min

E10-22 l⊥=lcos θ=mvr cos θ=mvh.

122

E10-23 (a) ωf= (I1/I2)ωi,I1= (3.66 kg)(0.363 m)2= 0.482 kg ·m2. Then

ωf= [(0.482 kg ·m2)/(2.88 kg ·m2)](57.7 rad/s) = 9.66 rad/s,

with the same rotational sense as the original wheel.

(b) Same answer, since friction is an internal force internal here.

E10-24 (a) Assume the merry-go-round is a disk. Then conservation of angular momentum yields

2mmR2+mgR2)ω+ (mrR2)(v/R) = 0,

ω=−(1.13 kg)(7.82 m/s)/(3.72 m)

(827 kg)/2 + (50.6 kg) =−5.12×10−3rad/s.

(b) v=ωR = (−5.12×10−3rad/s)(3.72 m) = −1.90×10−2m/s.

E10-25 Conservation of angular momentum:

(mmk2+mgR2)ω=mgR2(v/R),

ω=(44.3 kg)(2.92 m/s)/(1.22 m)

(176 kg)(0.916 m)2+ (44.3 kg)(1.22 m)2= 0.496 rad/s.

E10-26 Use Eq. 10-22:

ωP=Mgr

Iω =(0.492 kg)(9.81 m/s2)(3.88×10−2m)

(5.12×10−4kg ·m2)(2π28.6 rad/s) = 2.04 rad/s = 0.324 rev/s.

E10-27 The relevant precession expression is Eq. 10-22.

The rotational inertia will be a sum of the contributions from both the disk and the axle, but

the radius of the axle is probably very small compared to the disk, probably as small as 0.5 cm.

Since Iis proportional to the radius squared, we expect contributions from the axle to be less than

(1/100)2of the value for the disk. For the disk only we use

I=1

2MR2=1

2(1.14 kg)(0.487 m)2= 0.135 kg ·m2.

Now for ω,

ω= 975 rev/min 2πrad

1 rev 1 min

60 s = 102 rad/s.

Then L=Iω = 13.8 kg·m2/s.

Back to Eq. 10-22,

ωp=Mgr

L=(1.27 kg)(9.81 m/s2)(0.0610 m)

13.8 kg ·m2/s= 0.0551 rad/s.

The time for one precession is

t=1rev

ωp

=2πrad

(0.0551 rad/s) = 114 s.

123

P10-1 Positive zis out of the page.

(a) ~

l=rmv sin θˆ

k= (2.91 m)(2.13 kg)(4.18 m) sin(147◦)ˆ

k= 14.1 kg ·m2/sˆ

(b) ~τ =rF sin θˆ

k= (2.91 m)(1.88 N) sin(26◦)ˆ

k= 2.40 N ·mˆ

P10-2 Regardless of where the origin is located one can orient the coordinate system so that the

two paths lie in the xy plane and are both parallel to the yaxis. The one of the particles travels

along the path x=vt,y=a,z=b; the momentum of this particle is ~

p1=mvˆ

i. The other

particle will then travel along a path x=c−vt,y=a+d,z=b; the momentum of this particle is

p2=−mvˆ

i. The angular momentum of the ﬁrst particle is

l1=mvbˆ

j−mvaˆ

while that of the second is ~

l2=−mvbˆ

j+mv(a+d)ˆ

so the total is ~

l1+~

l2=mvdˆ

P10-3 Assume that the cue stick strikes the ball horizontally with a force of constant magnitude

Ffor a time ∆t. Then the magnitude of the change in linear momentum of the ball is given by

F∆t= ∆p=p, since the initial momentum is zero.

If the force is applied a distance xabove the center of the ball, then the magnitude of the torque

about a horizontal axis through the center of the ball is τ=xF . The change in angular momentum

of the ball is given by τ∆t= ∆l=l, since initially the ball is not rotating.

For the ball to roll without slipping we need v=ωR. We can start with this:

v=ωR,

m=lR

F∆t

m=τ∆tR

m=xF R

Then x=I/mR is the condition for rolling without sliding from the start. For a solid sphere,

I=2

5mR2, so x=2

5R.

P10-4 The change in momentum of the block is M(v2−v1), this is equal to the magnitude the

impulse delivered to the cylinder. According to E10-8 we can write M(v2−v1)R=Iωf. But in the

end the box isn’t slipping, so ωf=v2/R. Then

Mv2−Mv1= (I/R2)v2,

v2=v1/(1 + I/M R2).

P10-5 Assume that the cue stick strikes the ball horizontally with a force of constant magnitude

Ffor a time ∆t. Then the magnitude of the change in linear momentum of the ball is given by

F∆t= ∆p=p, since the initial momentum is zero. Consequently, F∆t=mv0.

If the force is applied a distance habove the center of the ball, then the magnitude of the torque

about a horizontal axis through the center of the ball is τ=hF . The change in angular momentum

of the ball is given by τ∆t= ∆l=l0,since initially the ball is not rotating. Consequently, the initial

angular momentum of the ball is l0=hmv0=Iω0.

124

The ball originally slips while moving, but eventually it rolls. When it has begun to roll without

slipping we have v=Rω. Applying the results from E10-8,

m(v−v0)R+I(ω−ω0) = 0,

m(v−v0)R+2

5mR2v

R−hmv0= 0,

then, if v= 9v0/7,

h=9

7−1R+2

5R9

7=4

5R.

P10-6 (a) Refer to the previous answer. We now want v=ω= 0, so

m(v−v0)R+2

5mR2v

R−hmv0= 0,

becomes

−v0R−hv0= 0,

or h=−R. That’ll scratch the felt.

(b) Assuming only a horizontal force then

v=(h+R)v0

R(1 + 2/5),

which can only be negative if h < −R, which means hitting below the ball. Can’t happen. If instead

we allow for a downward component, then we can increase the “reverse English” as much as we want

without increasing the initial forward velocity, and as such it would be possible to get the ball to

move backwards.

P10-7 We assume the bowling ball is solid, so the rotational inertia will be I= (2/5)MR2(see

Figure 9-15).

The normal force on the bowling ball will be N=Mg, where Mis the mass of the bowling ball.

The kinetic friction on the bowling ball is Ff=µkN=µkMg. The magnitude of the net torque on

the bowling ball while skidding is then τ=µkMgR.

Originally the angular momentum of the ball is zero; the ﬁnal angular momentum will have

magnitude l=Iω =Iv/R, where vis the ﬁnal translational speed of the ball.

(a) The time requires for the ball to stop skidding is the time required to change the angular

momentum to l, so

∆t=∆l

τ=(2/5)MR2v/R

µkMgR =2v

5µkg.

Since we don’t know v, we can’t solve this for ∆t. But the same time through which the angular

momentum of the ball is increasing the linear momentum of the ball is decreasing, so we also have

∆t=∆p

−Ff

=Mv −Mv0

−µkMg =v0−v

µkg.

Combining,

∆t=v0−v

µkg,

=v0−5µkg∆t/2

µkg,

125

2µkg∆t= 2v0−5µkg∆t,

∆t=2v0

7µkg,

=2(8.50 m/s)

7(0.210)(9.81 m/s2)= 1.18 s.

(d) Use the expression for angular momentum and torque,

v= 5µkg∆t/2 = 5(0.210)(9.81 m/s2)(1.18 s)/2 = 6.08 m/s.

(b) The acceleration of the ball is F/M =−µg. The distance traveled is then given by

x=1

2at2+v0t,

=−1

2(0.210)(9.81 m/s2)(1.18 s)2+ (8.50 m/s)(1.18 s) = 8.6 m,

θ=1

2αt2+ω0t,

=5(0.210)(9.81 m/s2)

4(0.11 m) (1.18 s)2= 32.6 rad = 5.19 revolutions.

P10-8 (a) l=Iω0= (1/2)MR2ω0.

(b) The initial speed is v0=Rω0. The chip decelerates in a time t=v0/g, and during this time

the chip travels with an average speed of v0/2 through a distance of

y=vavt=v0

g=R2ω2

2g.

P10-9 Since L=Iω = 2πI/T and Lis constant, then I∝T. But I∝R2, so R2∝Tand

∆T

T=2R∆R

R2=2∆R

Then

∆T= (86400 s) 2(30 m)

(6.37×106m) ≈0.8 s.

P10-10 Originally the rotational inertia was

Ii=2

5MR2=8π

15 ρ0R5.

The average density can be found from Appendix C. Now the rotational inertia is

If=8π

15 (ρ1−ρ2)R5

1+8π

15 ρ2R5,

where ρ1is the density of the core, R1is the radius of the core, and ρ2is the density of the mantle.

Since the angular momentum is constant we have ∆T /T = ∆I/I. Then

∆T

T=ρ1−ρ2

ρ0

R5+ρ2

ρ0−1 = 10.3−4.50

5.52

35705

63705+4.50

5.52 −1 = −0.127,

so the day is getting longer.

126

P10-11 The cockroach initially has an angular speed of ωc,i=−v/r. The rotational inertia of

the cockroach about the axis of the turntable is Ic=mR2. Then conservation of angular momentum

gives

lc,i+ls,i=lc,f+ls,f,

Icωc,i+Isωs,i=Icωc,f+Isωs,f,

−mR2v/r +Iω = (mR2+I)ωf,

ωf=Iω −mvR

I+mR2.

P10-12 (a) The skaters move in a circle of radius R= (2.92 m)/2=1.46 m centered midway

between the skaters. The angular velocity of the system will be ωi=v/R = (1.38 m/s)/(1.46 m) =

0.945 rad/s.

(b) Moving closer will decrease the rotational inertia, so

ωf=2MRi2

2MRf2ωi=(1.46 m)2

(0.470 m)2(0.945 rad/s) = 9.12 rad/s.

127

E11-1 (a) Apply Eq. 11-2, W=F s cos φ= (190 N)(3.3 m) cos(22◦) = 580 J.

(b) The force of gravity is perpendicular to the displacement of the crate, so there is no work

done by the force of gravity.

by the normal force.

E11-2 (a) The force required is F=ma = (106 kg)(1.97 m/s2) = 209 N. The object moves with

an average velocity vav =v0/2 in a time t=v0/a through a distance x=vavt=v2

0/(2a). So

x= (51.3 m/s)2/[2(1.97 m/s2)] = 668 m.

The work done is W=F x = (−209 N)(668 m) = 1.40×105J.

(b) The force required is

F=ma = (106 kg)(4.82 m/s2) = 511 N.

x= (51.3 m/s)2/[2(4.82 m/s2)] = 273 m.The work done is W=F x = (−511 N)(273 m) = 1.40×105J.

E11-3 (a) W=F x = (120 N)(3.6 m) = 430 J.

(b) W=F x cos θ=mgx cos θ= (25 kg)(9.8 m/s2)(3.6 m) cos(117◦) = 400 N.

E11-4 The worker pushes with a force ~

P; this force has components Px=Pcos θand Py=Psin θ,

where θ=−32.0◦. The normal force of the ground on the crate is N=mg −Py, so the force of

friction is f=µkN=µk(mg −Py). The crate moves at constant speed, so Px=f. Then

Pcos θ=µk(mg −Psin θ),

P=µkmg

cos θ+µksin θ.

The work done on the crate is

W=~

P·~

x=P x cos θ=µkxmg

1 + µktan θ,

=(0.21)(31.3 ft)(58.7 lb)

1 + (0.21) tan(−32.0◦)= 444 ft ·lb.

E11-5 The components of the weight are W|| =mg sin θand W⊥=mg cos θ. The push ~

Phas

components P|| =Pcos θand P⊥=Psin θ.

The normal force on the trunk is N=W⊥+P⊥so the force of friction is f=µk(mg cos θ+

Psin θ). The push required to move the trunk up at constant speed is then found by noting that

P|| =W|| +f.

Then

P=mg(tan θ+µk)

1−µktan θ.

(a) The work done by the applied force is

W=P x cos θ=(52.3 kg)(9.81 m/s2)[sin(28.0◦) + (0.19) cos(28.0◦)](5.95 m)

1−(0.19) tan(28.0◦)= 2160 J.

(b) The work done by the force of gravity is

W=mgx cos(θ+ 90◦) = (52.3 kg)(9.81 m/s2)(5.95 m) cos(118◦) = −1430 J.

128

E11-6 θ= arcsin(0.902 m/1.62 m) = 33.8◦.

The components of the weight are W|| =mg sin θand W⊥=mg cos θ.

The normal force on the ice is N=W⊥so the force of friction is f=µkmg cos θ. The push

required to allow the ice to slide down at constant speed is then found by noting that P=W|| −f.

Then P=mg(sin θ−µkcos θ).

(a) P= (47.2 kg)(9.81 m/s2)[sin(33.8◦)−(0.110) cos(33.8◦)] = 215 N.

(b) The work done by the applied force is W=P x = (215 N)(−1.62 m) = −348 J.

W=mgx cos(90◦−θ) = (47.2 kg)(9.81 m/s2)(1.62 m) cos(56.2◦) = 417 J.

E11-7 Equation 11-5 describes how to ﬁnd the dot product of two vectors from components,

a·~

b=axbx+ayby+azbz,

= (3)(2) + (3)(1) + (3)(3) = 18.

Equation 11-3 can be used to ﬁnd the angle between the vectors,

a=p(3)2+ (3)2+ (3)2= 5.19,

b=p(2)2+ (1)2+ (3)2= 3.74.

Now use Eq. 11-3,

cos φ=~

a·~

ab =(18)

(5.19)(3.74) = 0.927,

and then φ= 22.0◦.

E11-8 ~

a·~

b= (12)(5.8) cos(55◦) = 40.

E11-9 ~

r·~

s= (4.5)(7.3) cos(320◦−85◦) = −19.

E11-10 (a) Add the components individually:

r= (5 + 2 + 4)ˆ

i+ (4 −2 + 3)ˆ

j+ (−6−3 + 2)ˆ

k= 11ˆ

i+ 5ˆ

j−7ˆ

(b) θ= arccos(−7/√112+ 52+ 72) = 120◦.

a·~

b/√ab), or

θ=(5)(−2) + (4)(2) + (−6)(3)

p(52+ 42+ 62)(22+ 22+ 32)= 124◦.

E11-11 There are two forces on the woman, the force of gravity directed down and the normal

force of the ﬂoor directed up. These will be eﬀectively equal, so N=W=mg. Consequently, the

57 kg woman must exert a force of F= (57kg)(9.8m/s2) = 560N to propel herself up the stairs.

From the reference frame of the woman the stairs are moving down, and she is exerting a force

down, so the work done by the woman is given by

W=F s = (560 N)(4.5 m) = 2500 J,

this work is positive because the force is in the same direction as the displacement.

The average power supplied by the woman is given by Eq. 11-7,

P=W/t = (2500 J)/(3.5 s) = 710 W.

129

E11-12 P=W/t =mgy/t = (100 ×667 N)(152 m)/(55.0 s) = 1.84×105W.

E11-13 P=F v = (110 N)(0.22 m/s) = 24 W.

E11-14 F=P/v, but the units are awkward.

F=(4800 hp)

(77 knots)

1 knot

1.688 ft/s

1 ft/s

0.3048 m/s

745.7 W

1 hp = 9.0×104N.

E11-15 P=F v = (720 N)(26 m/s) = 19000 W; in horsepower, P= 19000 W(1/745.7 hp/W) =

25 hp.

E11-16 Change to metric units! Then P= 4920 W, and the ﬂow rate is Q= 13.9 L/s. The

density of water is approximately 1.00 kg/L , so the mass ﬂow rate is R= 13.9 kg/s.

y=P

gR =(4920 kg)

(9.81 m/s2)(13.9 kg/s) = 36.1 m,

which is the same as approximately 120 feet.

E11-17 (a) Start by converting kilowatt-hours to Joules:

1 kW ·h = (1000 W)(3600 s) = 3.6×106J.

The car gets 30 mi/gal, and one gallon of gas produces 140 MJ of energy. The gas required to

produce 3.6×106J is

3.6×106J1 gal

140×106J= 0.026 gal.

The distance traveled on this much gasoline is

0.026 gal 30 mi

1 gal = 0.78 mi.

(b) At 55 mi/h, it will take

0.78 mi 1 hr

55 mi= 0.014 h = 51 s.

The rate of energy expenditure is then (3.6×106J)/(51 s) = 71000 W.

E11-18 The linear speed is v= 2π(0.207 m)(2.53 rev/s) = 3.29 m/s. The frictional force is f=

µkN= (0.32)(180 N) = 57.6 N. The power developed is P=F v = (57.6 N)(3.29 m) = 190 W.

E11-19 The net force required will be (1380 kg −1220 kg)(9.81 m/s2) = 1570 N. The work is

W=F y, the power output is P=W/t = (1570 N)(54.5 m)/(43.0 s) = 1990 W, or P= 2.67 hp.

E11-20 (a) The momentum change of the ejected material in one second is

∆p= (70.2 kg)(497 m/s−184 m/s) + (2.92 kg)(497 m/s) = 2.34×104kg ·m/s.

The thrust is then F= ∆p/∆t= 2.34×104N.

(b) The power is P=F v = (2.34×104N)(184 m/s) = 4.31×106W. That’s 5780 hp.

130

E11-21 The acceleration on the object as a function of position is given by

a=20 m/s2

8 m x,

The work done on the object is given by Eq. 11-14,

W=Z8

Fxdx =Z8

(10 kg) 20 m/s2

8 m x dx = 800 J.

E11-22 Work is area between the curve and the line F= 0. Then

W= (10 N)(2 s) + 1

2(10 N)(2 s) + 1

2(−5 N)(2 s) = 25 J.

E11-23 (a) For a spring, F=−kx, and ∆F=−k∆x.

k=−∆F

∆x=−(−240 N) −(−110 N)

(0.060 m) −(0.040 m) = 6500 N/m.

With no force on the spring,

∆x=−∆F

k=−(0) −(−110 N)

(6500 N/m) =−0.017 m.

This is the amount less than the 40 mm mark, so the position of the spring with no force on it is 23

mm.

(b) ∆x=−10 mm compared to the 100 N picture, so

∆F=−k∆x=−(6500 N/m)(−0.010 m) = 65 N.

The weight of the last object is 110 N −65 N = 45 N.

E11-24 (a) W=1

2k(xf2−xi2) = 1

2(1500 N/m)(7.60×10−3m)2= 4.33×10−2J.

(b) W=1

2(1500 N/m)[(1.52×10−2m)2−(7.60×10−3m)2= 1.30×10−1J.

E11-25 Start with Eq. 11-20, and let Fx= 0 while Fy=−mg:

W=Zf

(Fxdx +Fydy) = −mg Zf

dy =−mgh.

E11-26 (a) F0=mv2

0/r0= (0.675 kg)(10.0 m/s)2/(0.500 m) = 135 N.

(b) Angular momentum is conserved, so v=v0(r0/r). The force then varies as F=mv2/r =

mv2

0r2

0/r3=F0(r0/r)3.The work done is

W=Z~

F·~

dr =(−135 N)(0.500 m)3

−2(0.300 m)−2−(0.500 m)−2= 60.0 J.

E11-27 The kinetic energy of the electron is

4.2 eV 1.60 ×10−19 J

1 eV = 6.7×10−19 J.

Then

v=r2K

m=s2(6.7×10−19J)

(9.1×10−31kg) = 1.2×106m/s.

131

E11-28 (a) K=1

2(110 kg)(8.1 m/s)2= 3600 J.

(b) K=1

2(4.2×10−3kg)(950 m/s)2= 1900 J.

v= 32.0 knots(1.688 ft/s/knot)(0.3048 m/ft) = 16.5 m/s.

K=1

2(8.29×107kg)(16.5 m/s)2= 1.13×1010J.

E11-29 (b) ∆K=W=F x = (1.67×10−27kg)(3.60×1015m/s2)(0.0350 m) = 2.10×10−13J. That’s

2.10×10−13J/(1.60×10−19J/eV) = 1.31×106eV.

(a) Kf= 2.10×10−13J + 1

2(1.67×10−27kg)(2.40×107m/s2) = 6.91×10−13J. Then

vf=p2K/m =p2(6.91×10−13J)/(1.67×10−27kg) = 2.88×107m/s.

E11-30 Work is negative if kinetic energy is decreasing. This happens only in region CD. The

work is zero in region BC. Otherwise it is positive.

E11-31 (a) Find the velocity of the particle by taking the time derivative of the position:

v=dx

dt = (3.0 m/s) −(8.0 m/s2)t+ (3.0 m/s3)t2.

Find vat two times: t= 0 and t= 4 s.

v(0) = (3.0 m/s) −(8.0 m/s2)(0) + (3.0 m/s3)(0)2= 3.0 m/s,

v(4) = (3.0 m/s) −(8.0 m/s2)(4.0 s) + (3.0 m/s3)(4.0 s)2= 19.0 m/s

The initial kinetic energy is Ki=1

2(2.80 kg)(3.0 m/s)2= 13 J, while the ﬁnal kinetic energy is

Kf=1

2(2.80 kg)(19.0 m/s)2= 505 J.

The work done by the force is given by Eq. 11-24,

W=Kf−Ki= 505 J −13 J = 492 J.

(b) This question is asking for the instantaneous power when t= 3.0 s. P=F v, so ﬁrst ﬁnd a;

a=dv

dt =−(8.0 m/s2) + (6.0 m/s3)t.

Then the power is given by P=mav, and when t= 3 s this gives

P=mav = (2.80 kg)(10 m/s2)(6 m/s) = 168 W.

E11-32 W= ∆K=−Ki. Then

W=−1

2(5.98×1024kg)(29.8×103m/s)2= 2.66×1033J.

E11-33 (a) K=1

2(1600 kg)(20 m/s)2= 3.2×105J.

(b) P=W/t = (3.2×105J)/(33 s) = 9.7×103W.

E11-34 (a) I= 1.40×104u·pm2(1.66×10−27kg/mboxu) = 2.32×10−47kg ·m2.

(b) K=1

2Iω2=1

2(2.32×10−47kg ·m2)(4.30×1012 rad/s)2= 2.14×10−22J.That’s 1.34 meV.

132

E11-35 The translational kinetic energy is Kt=1

2mv2, the rotational kinetic energy is Kr=

2Iω2=2

3Kt. Then

ω=r2m

3Iv=s2(5.30×10−26kg)

3(1.94×10−46kg ·m2)(500 m/s) = 6.75×1012 rad/s.

E11-36 Kr=1

2Iω2=1

4(512 kg)(0.976 m)2(624 rad/s)2= 4.75×107J.

(b) t=W/P = (4.75×107J)/(8130 W) = 5840 s, or 97.4 minutes.

E11-37 From Eq. 11-29, Ki=1

2Iωi2. The object is a hoop, so I=MR2. Then

Ki=1

2MR2ω2=1

2(31.4 kg)(1.21 m)2(29.6 rad/s)2= 2.01 ×104J.

Finally, the average power required to stop the wheel is

P=W

t=Kf−Ki

t=(0) −(2.01 ×104J)

(14.8 s) =−1360 W.

E11-38 The wheels are connected by a belt, so rAωA=rBωB, or ωA= 3ωB.

(a) If lA=lBthen

=lA/ωA

lB/ωB

=ωB

ωA

(b) If instead KA=KBthen

=2KA/ωA2

2KB/ωB2=ωB2

ωA2=1

E11-39 (a) ω= 2π/T , so

K=1

2Iω2=4π2

(5.98×1024kg)(6.37×106m)2

(86,400 s)2= 2.57×1029J

(b) t= (2.57×1029J)/(6.17×1012W) = 4.17×1016s, or 1.3 billion years.

E11-40 (a) The velocities relative to the center of mass are m1v1=m2v2; combine with v1+v2=

910.0 m/s and get

(290.0 kg)v1= (150.0 kg)(910 m/s−v1),

v1= (150 kg)(910 m/s)/(290 kg + 150 kg) = 310 m/s

and v2= 600 m/s. The rocket case was sent back, so vc= 7600 m/s−310 m/s = 7290 m/s. The

payload capsule was sent forward, so vp= 7600 m/s + 600 m/s = 8200 m/s.

(b) Before,

Ki=1

2(290 kg + 150 kg)(7600 m/s)2= 1.271×1010J.

After,

Kf=1

2(290 kg)(7290 m/s)2+1

2(150 kg)(8200 m/s)2= 1.275×1010J.

The “extra” energy came from the spring.

133

E11-41 Let the mass of the freight car be Mand the initial speed be vi. Let the mass of the

caboose be mand the ﬁnal speed of the coupled cars be vf. The caboose is originally at rest, so the

expression of momentum conservation is

Mvi=Mvf+mvf= (M+m)vf

The decrease in kinetic energy is given by

Ki−Kf=1

2Mvi2−1

2Mvf2+1

2mvf2,

2Mvi2−(M+m)vf2

What we really want is (Ki−Kf)/Ki, so

Ki−Kf

=Mvi2−(M+m)vf2

Mvi2,

= 1 −M+m

Mvf

vi2

= 1 −M+m

MM

M+m2

where in the last line we substituted from the momentum conservation expression.

Then Ki−Kf

= 1 −M

M+m= 1 −Mg

Mg +mg .

The left hand side is 27%. We want to solve this for mg, the weight of the caboose. Upon rearranging,

mg =Mg

1−0.27 −Mg =(35.0 ton)

(0.73) −(35.0 ton) = 12.9 ton.

E11-42 Since the body splits into two parts with equal mass then the velocity gained by one is

identical to the velocity “lost” by the other. The initial kinetic energy is

Ki=1

2(8.0 kg)(2.0 m/s)2= 16 J.

The ﬁnal kinetic energy is 16 J greater than this, so

Kf= 32 J = 1

2(4.0 kg)(2.0 m/s + v)2+1

2(4.0 kg)(2.0 m/s−v)2,

2(8.0 kg)[(2.0 m/s)2+v2],

so 16.0 J = (4.0 kg)v2. Then v= 2.0 m/s; one chunk comes to a rest while the other moves oﬀ at a

speed of 4.0 m/s.

E11-43 The initial velocity of the neutron is v0ˆ

i, the ﬁnal velocity is v1ˆ

j. By momentum conser-

vation the ﬁnal momentum of the deuteron is mn(v0ˆ

i−v1ˆ

j). Then mdv2=mnpv2

0+v2

There is also conservation of kinetic energy:

2mnv2

0=1

2mnv2

1+1

2mdv2

Rounding the numbers slightly we have md= 2mn, then 4v2

2=v2

0+v2

1is the momentum expression

and v2

0=v2

1+ 2v2

2is the energy expression. Combining,

2v2

0= 2v2

1+ (v2

0+v2

1),

or v2

1=v2

0/3. So the neutron is left with 1/3 of its original kinetic energy.

134

E11-44 (a) The third particle must have a momentum

p3=−(16.7×10−27kg)(6.22×106m/s)ˆ

i+ (8.35×10−27kg)(7.85×106m/s)ˆ

= (−1.04ˆ

i+ 0.655ˆ

j)×10−19kg ·m/s.

(b) The kinetic energy can also be written as K=1

2mv2=1

2m(p/m)2=p2/2m. Then the

kinetic energy appearing in this process is

K=1

2(16.7×10−27kg)(6.22×106m/s)2+1

2(8.35×10−27kg)(7.85×106m/s)2

2(11.7×10−27kg) (1.23×10−19kg ·m/s)2= 1.23×10−12J.

This is the same as 7.66 MeV.

P11-1 Change your units! Then

F=W

s=(4.5 eV)(1.6×10−19 J/eV)

(3.4×10−9m) = 2.1×10−10 N.

P11-2 (a) If the acceleration is −g/4 the the net force on the block is −Mg/4, so the tension in

the cord must be T= 3Mg/4.

(a) The work done by the cord is W=~

F·~

s= (3Mg/4)(−d) = −(3/4)Mgd.

(b) The work done by gravity is W=~

F·~

s= (−Mg)(−d) = Mgd.

P11-3 (a) There are four cords which are attached to the bottom load L. Each supports a tension

F, so to lift the load 4F= (840 lb) + (20.0 lb), or F= 215 lb.

(b) The work done against gravity is W=~

F·~

s= (840 lb)(12.0 ft) = 10100 ft ·lb.

segments, so the end of the cord must be pulled through a distance of 48.0 ft.

(d) The work done by the applied force is W=~

F·~

s= (215 lb)(48.0 ft) = 10300 ft ·lb.

P11-4 The incline has a height hwhere h=W/mg = (680 J)/[(75 kg)(9.81 m/s2)]. The work

required to lift the block is the same regardless of the path, so the length of the incline lis l=

W/F = (680 J)/(320 N).The angle of the incline is then

θ= arcsin h

l= arcsin F

mg = arcsin (320 N)

(75 kg)(9.81 m/s2)= 25.8◦.

P11-5 (a) In 12 minutes the horse moves x= (5280 ft/mi)(6.20 mi/h)(0.200 h) = 6550 ft. The

work done by the horse in that time is W=~

F·~

s= (42.0 lb)(6550 ft) cos(27.0◦) = 2.45×105ft ·lb.

(b) The power output of the horse is

P=(2.45×105ft ·lb)

(720 s) = 340 ft ·lb/s 1 hp

550 ft ·lb/s = 0.618 hp.

P11-6 In this problem θ= arctan(28.2/39.4) = 35.5◦.

The weight of the block has components W|| =mg sin θand W⊥=mg cos θ. The force of friction

on the block is f=µkN=µkmg cos θ. The tension in the rope must then be

T=mg(sin θ+µkcos θ)

in order to move the block up the ramp. The power supplied by the winch will be P=T v, so

P= (1380 kg)(9.81 m/s2)[sin(35.5◦) + (0.41) cos(35.5◦)](1.34 m/s) = 1.66×104W.

135

P11-7 If the power is constant then the force on the car is given by F=P/v. But the force is

related to the acceleration by F=ma and to the speed by F=mdv

dt for motion in one dimension.

Then

F=P

mdv

dt =P

mdx

dx =P

mv dv

dx =P

mv2dv =Zx

P dx,

3mv3=P x.

We can rearrange this ﬁnal expression to get vas a function of x,v= (3xP/m)1/3.

P11-8 (a) If the drag is D=bv2, then the force required to move the plane forward at constant

speed is F=D=bv2, so the power required is P=F v =bv3.

(b) P∝v3, so if the speed increases to 125% then Pincreases by a factor of 1.253= 1.953, or

increases by 95.3%.

P11-9 (a) P=mgh/t, but m/t is the persons per minute times the average mass, so

P= (100 people/min)(75.0kg)(9.81 m/s2)(8.20 m) = 1.01×104W.

(b) In 9.50 s the Escalator has moved (0.620 m/s)(9.50 s) = 5.89 m; so the Escalator has “lifted”

the man through a distance of (5.89 m)(8.20 m/13.3 m) = 3.63 m. The man did the rest himself.

The work done by the Escalator is then W= (83.5 kg)(9.81 m/s2)(3.63 m) = 2970 J.

ponent of the force. The power is

P= (83.5 m/s2)(9.81 m/s2)(0.620 m/s)(8.20 m/13.3 m) = 313 W.

(d) If there is a force of contact between the man and the Escalator then the Escalator is doing

work on the man.

P11-10 (a) dP/dv =ab −3av2, so Pmax occurs when 3v2=b, or v=pb/3.

(b) F=P/v, so dF/dv =−2v, which means Fis a maximum when v= 0.

P11-11 (b) Integrate,

W=Z3x0

F·d~

s=F0

x0Z3x0

(x−x0)dx =F0x09

2−3,

or W= 3F0x0/2.

136

P11-12 (a) Simpson’s rule gives

W=1

3[(10 N) + 4(2.4 N) + (0.8 N)] (1.0 m) = 6.8 J.

(b) W=RF ds =R(A/x2)dx =−A/x, evaluating this between the limits of integration gives

W= (9 N ·m2)(1/1 m −1/3 m) = 6 J.

P11-13 The work required to stretch the spring from xito xfis given by

W=Zxf

kx3dx =k

4xf4−k

4xi4.

The problem gives

W0=k

4(l)4−k

4(0)4=k

4l4.

We then want to ﬁnd the work required to stretch from x=lto x= 2l, so

Wl→2l=k

4(2l)4−k

4(l)4,

= 16k

4l4−k

4l4,

= 15k

4l4= 15W0.

P11-14 (a) The spring extension is δl =pl2

0+x2−l0. The force from one spring has magnitude

kδl, but only the xcomponent contributes to the problem, so

F= 2kql2

0+x2−l0x

pl2

0+x2

is the force required to move the point.

The work required is the integral, W=Rx

0F dx, which is

W=kx2−2kl0ql2

0+x2+ 2kl2

Note that it does reduce to the expected behavior for xl0.

(b) Binomial expansion of square root gives

ql2

0+x2=l01 + 1

0−1

0···,

so the ﬁrst term in the above expansion cancels with the last term in W; the second term cancels

with the ﬁrst term in W, leaving

W=1

4kx4

P11-15 Number the springs clockwise from the top of the picture. Then the four forces on each

spring are

F1=k(l0−px2+ (l0−y)2),

F2=k(l0−p(l0−x)2+y2),

F3=k(l0−px2+ (l0+y)2),

F4=k(l0−p(l0+x)2+y2).

137

The directions are much harder to work out, but for small xand ywe can assume that

F1=k(l0−px2+ (l0−y)2)ˆ

F2=k(l0−p(l0−x)2+y2)ˆ

F3=k(l0−px2+ (l0+y)2)ˆ

F4=k(l0−p(l0+x)2+y2)ˆ

Then

W=Z~

F·d~

s=Z(F1+F3)dy +Z(F2+F4)dx,

Since xand yare small, expand the force(s) in a binomial expansion:

F1(x, y)≈F1(0,0) + ∂F1

∂x x,y=0

x+∂F1

∂y x,y=0

y=ky;

there will be similar expression for the other four forces. Then

W=Z2ky dy +Z2kx dx =k(x2+y2) = kd2.

P11-16 (a) Ki=1

2(1100 kg)(12.8 m/s)2= 9.0×104J. Removing 51 kJ leaves 39 kJ behind, so

vf=p2Kf/m =p2(3.9×104J)/(1100 kg) = 8.4 m/s,

or 30 km/h.

(b) 39 kJ, as was found above.

P11-17 Let Mbe the mass of the man and mbe the mass of the boy. Let vMbe the original

speed of the man and vmbe the original speed of the boy. Then

2Mv2

M=1

21

2mv2

m

and 1

2M(vM+ 1.0 m/s)2=1

2mv2

Combine these two expressions and solve for vM,

2Mv2

M=1

21

2M(vM+ 1.0 m/s)2,

M=1

2(vM+ 1.0 m/s)2,

0 = −v2

M+ (2.0 m/s)vM+ (1.0 m/s)2.

The last line can be solved as a quadratic, and vM= (1.0 m/s) ±(1.41 m/s). Now we use the very

ﬁrst equation to ﬁnd the speed of the boy,

2Mv2

M=1

21

2mv2

m,

M=1

4v2

2vM=vm.

138

P11-18 (a) The work done by gravity on the projectile as it is raised up to 140 m is W=

−mgy =−(0.550 kg)(9.81 m/s2)(140 m) = −755 J. Then the kinetic energy at the highest point is

1550 J −755 J = 795 J. Since the projectile must be moving horizontally at the highest point, the

horizontal velocity is vx=p2(795 J)/(0.550 kg) = 53.8 m/s.

(b) The magnitude of the velocity at launch is v=p2(1550 J)/(0.550 kg) = 75.1 m/s. Then

vy=p(75.1 m/s)2−(53.8 m/s)2= 52.4 m/s.

2(0.550 kg)[(65.0 m/s)2+ (53.8 m/s)2] = 1960 J. Since it

has extra kinetic energy, it must be below the launch point, and gravity has done 410 J of work on

it. That means it is y= (410 J)/[(0.550 kg)(9.81 m/s2)] = 76.0 m below the launch point.

P11-19 (a) K=1

2mv2=1

2(8.38 ×1011kg)(3.0×104m/s)2= 3.77 ×1020J.In terms of TNT,

K= 9.0×104megatons.

(b) The diameter will be 3

√8.98×104= 45 km.

P11-20 (a) Wg=−(0.263 kg)(9.81 m/s2)(−0.118 m) = 0.304 J.

(b) Ws=−1

2(252 N/m)(−0.118 m)2=−1.75 J.

is then v=p2(1.45 J)/(0.263 kg) = 3.32 m/s.

(d) Doubling the speed quadruples the initial kinetic energy to 5.78 J. The compression will then

be given by

−5.78 J = −1

2(252 N/m)y2−(0.263 kg)(9.81 m/s2)y,

with solution y= 0.225 m.

P11-21 (a) We can solve this with a trick of integration.

W=Zx

F dx,

=Zx

max

dt dx =maxZt

dt dt

=maxZt

vxdt =maxZt

at dt,

2ma2

xt2.

Basically, we changed the variable of integration from xto t, and then used the fact the the accel-

eration was constant so vx=v0x+axt. The object started at rest so v0x= 0, and we are given in

the problem that vf=atf. Combining,

W=1

2ma2

xt2=1

2mvf

tf2

t2.

(b) Instantaneous power will be the derivative of this, so

P=dW

dt =mvf

tf2

139

P11-22 (a) α= (−39.0 rev/s)(2πrad/rev)/(32.0 s) = −7.66 rad/s2.

(b) The total rotational inertia of the system about the axis of rotation is

I= (6.40 kg)(1.20 m)2/12 + 2(1.06 kg)(1.20 m/2)2= 1.53 kg ·m2.

The torque is then τ= (1.53 kg ·m2)(7.66 rad/s2) = 11.7 N ·m.

2(1.53 kg ·m2)(245 rad/s)2= 4.59×104J.

(d) θ=ωavt= (39.0 rev/s/2)(32.0 s) = 624 rev.

(e) Only the loss in kinetic energy is independent of the behavior of the frictional torque.

P11-23 The wheel turn with angular speed ω=v/r, where ris the radius of the wheel and vthe

speed of the car. The total rotational kinetic energy in the four wheels is then

Kr = 41

2Iω2= 2 1

2(11.3 kg)r2hv

ri2= (11.3 kg)v2.

The translational kinetic energy is Kt=1

2(1040 kg)v2, so the fraction of the total which is due to

the rotation of the wheels is 11.3

520 + 11.3= 0.0213 or 2.13%.

P11-24 (a) Conservation of angular momentum: ωf= (6.13 kg ·m2/1.97 kg ·m2)(1.22 rev/s) =

3.80 rev/s.

(b) Kr∝Iω2∝l2/I, so

Kf/Ki=Ii/If= (6.13 kg ·m2)/(1.97 kg ·m2) = 3.11.

P11-25 We did the ﬁrst part of the solution in Ex. 10-21. The initial kinetic energy is (again,

ignoring the shaft),

Ki=1

2I1~ω1,i2,

since the second wheel was originally at rest. The ﬁnal kinetic energy is

Kf=1

2(I1+I2)~ωf2,

since the two wheels moved as one. Then

Ki−Kf

2I1~ω1,i2−1

2(I1+I2)~ωf2

2I1~ω1,i2,

= 1 −(I1+I2)~ωf2

I1~ω1,i2,

= 1 −I1

I1+I2

where in the last line we substituted from the results of Ex. 10-21.

Using the numbers from Ex. 10-21,

Ki−Kf

= 1 −(1.27 kg·m2)

(1.27 kg·m2) + (4.85 kg·m2)= 79.2%.

140

P11-26 See the solution to P10-11.

Ki=I

2ω2+m

2v2

while

Kf=1

2(I+mR2)ωf2

according to P10-11,

ωf=Iω −mvR

I+mR2.

Then

Kf=1

(Iω −mvR)2

I+mR2.

Finally,

∆K=1

(Iω −mvR)2−(I+mR2)(Iω2+mv2)

I+mR2,

=−1

ImR2ω2+ 2mvRIω +Imv2

I+mR2,

=−Im

(Rω +v)2

I+mR2.

P11-27 See the solution to P10-12.

(a) Ki=1

2Iiωi2, so

Ki=1

22(51.2 kg)(1.46 m)2(0.945 rad/s)2= 97.5 J.

(b) Kf=1

22(51.2 kg)(0.470 m)2(9.12 rad/s)2= 941 J.The energy comes from the work they

do while pulling themselves closer together.

P11-28 K=1

2mv2=1

2mp2=1

2m~

p·~

p. Then

Kf=1

2m(~

pi+ ∆~

p)·(~

pi+ ∆~

p),

2mpi2+ 2~

pi·∆~

p+ (∆p)2,

∆K=1

2m2~

pi·∆~

p+ (∆p)2.

In all three cases ∆p= (3000 N)(65.0 s) = 1.95×105N·s and pi= (2500 kg)(300 m/s) = 7.50×105kg ·

m/s.

(a) If the thrust is backward (pushing rocket forward),

∆K=+2(7.50×105kg ·m/s)(1.95×105N·s) + (1.95×105N·s)2

2(2500 kg) = +6.61×107J.

(b) If the thrust is forward,

∆K=−2(7.50×105kg ·m/s)(1.95×105N·s) + (1.95×105N·s)2

2(2500 kg) =−5.09×107J.

∆K=+(1.95×105N·s)2

2(2500 kg) = 7.61×106J.

141

P11-29 There’s nothing to integrate here! Start with the work-energy theorem

W=Kf−Ki=1

2mvf2−1

2mvi2,

2mvf2−vi2,

2m(vf−vi) (vf+vi),

where in the last line we factored the diﬀerence of two squares. Continuing,

W=1

2(mvf−mvi) (vf+vi),

2(∆p) (vf+vi),

but ∆p=J, the impulse. That ﬁnishes this problem.

P11-30 Let Mbe the mass of the helicopter. It will take a force Mg to keep the helicopter

airborne. This force comes from pushing the air down at a rate ∆m/∆twith a speed of v; so

Mg =v∆m/∆t. The blades sweep out a cylinder of cross sectional area A, so ∆m/∆t=ρAv.

The force is then Mg =ρAv2; the speed that the air must be pushed down is v=pMg/ρA. The

minimum power is then

P=F v =MgsMg

ρA =s(1820 kg)3(9.81 m/s2)3

(1.23 kg/m3)π(4.88 m)2= 2.49×105W.

P11-31 (a) Inelastic collision, so vf=mvi/(m+M).

(b) K=1

2mv2=p2/2m, so

∆K

=1/m −1/(m+M)

1/m =M

m+M.

P11-32 Inelastic collision, so

vf=(1.88 kg)(10.3 m/s) + (4.92 kg)(3.27 m/s)

(1.88 kg) + (4.92 kg) = 5.21 m/s.

The loss in kinetic energy is

∆K=(1.88 kg)(10.3 m/s)2

2+(4.92 kg)(3.27 m/s)2

2−(1.88 kg + 4.92 kg)(5.21 m/s)2

2= 33.7 J.

This change is because of work done on the spring, so

x=p2(33.7 J)/(1120 N/m) = 0.245 m

P11-33 ~

pf,B =~

pi,A +~

pi,B −~

pf,A, so

pf,B = [(2.0 kg)(15 m/s) + (3.0 kg)(−10 m/s) −(2.0 kg)(−6.0 m/s)]ˆ

+[(2.0 kg)(30 m/s) + (3.0 kg)(5.0 m/s) −(2.0 kg)(30 m/s)]ˆ

= (12 kg ·m/s)ˆ

i+ (15 kg ·m/s)ˆ

142

Then ~

vf,B = (4.0 m/s)ˆ

i+ (5.0 m/s)ˆ

j.Since K=m

2(v2

x+v2

y), the change in kinetic energy is

∆K=(2.0 kg)[(−6.0 m/s)2+ (30 m/s)2−(15 m/s)2−(30 m/s)2]

+(3.0 kg)[(4.0 m/s)2+ (5 m/s)2−(−10 m/s)2−(5.0 m/s)2]

=−315 J.

P11-34 For the observer on the train the acceleration of the particle is a, the distance traveled is

xt=1

2at2, so the work done as measured by the train is Wt=maxt=1

2a2t2.The ﬁnal speed of

the particle as measured by the train is vt=at, so the kinetic energy as measured by the train is

K=1

2mv2=1

2m(at)2.The particle started from rest, so ∆Kt=Wt.

For the observer on the ground the acceleration of the particle is a, the distance traveled is

xg=1

2at2+ut, so the work done as measured by the ground is Wg=maxg=1

2a2t2+maut.

The ﬁnal speed of the particle as measured by the ground is vg=at +u, so the kinetic energy as

measured by the ground is

Kg=1

2mv2=1

2m(at +u)2=1

2a2t2+maut +1

2mu2.

But the initial kinetic energy as measured by the ground is 1

2mu2, so Wg= ∆Kg.

P11-35 (a) Ki=1

2m1v1,i2.

(b) After collision vf=m1v1,i/(m1+m2), so

Kf=1

2(m1+m2)m1v1,i

m1+m22

2m1v1,i2m1

m1+m2.

1−m1

m1+m2

=m2

m1+m2

(d) Note that vcm =vf. The initial kinetic energy of the system is

Ki=1

2m1v01,i2+1

2m2v02,i2.

The ﬁnal kinetic energy is zero (they stick together!), so the fraction lost is 1. The amount lost,

however, is the same.

P11-36 Only consider the ﬁrst two collisions, the one between mand m0, and then the one between

m0and M.

Momentum conservation applied to the ﬁrst collision means the speed of m0will be between

v0=mv0/(m+m0) (completely inelastic)and v0= 2mv0/(m+m0) (completely elastic). Momentum

conservation applied to the second collision means the speed of Mwill be between V=m0v0/(m0+M)

and V= 2m0v0/(m0+M). The largest kinetic energy for Mwill occur when it is moving the fastest,

v0=2mv0

m+m0and V=2m0v0

m0+M=4m0mv0

(m+m0)(m0+M).

We want to maximize Vas a function of m0, so take the derivative:

dm0=4mv0(mM −m02)

(m0+M)2(m+m0)2.

This vanishes when m0=√mM .

143

E12-1 (a) Integrate.

U(x) = −Zx

∞

Gm1m2

x2dx =−Gm1m2

(b) W=U(x)−U(x+d), so

W=Gm1m21

x−1

x+d=Gm1m2

x(x+d).

E12-2 If d << x then x(x+d)≈x2, so

W≈Gm1m2

x2d.

E12-3 Start with Eq. 12-6.

U(x)−U(x0) = −Zx

Fx(x)dx,

=−Zx

x0−αxe−βx2dx,

=−α

2βe−βx2

Finishing the integration,

U(x) = U(x0) + α

2βe−βx2

0−e−βx2.

If we choose x0=∞and U(x0) = 0 we would be left with

U(x) = −α

2βe−βx2.

E12-4 ∆K=−∆Uso ∆K=mg∆y. The power output is then

P= (58%)(1000 kg/m3)(73,800 m3)

(60 s) (9.81 m/s2)(96.3 m) = 6.74×108W.

E12-5 ∆U=−∆K, so 1

2kx2=1

2mv2. Then

k=mv2

x2=(2.38 kg)(10.0×103/s)

(1.47 m)2= 1.10×108N/m.

Wow.

E12-6 ∆Ug+ ∆Us= 0, since K= 0 when the man jumps and when the man stops. Then

∆Us=−mg∆y= (220 lb)(40.4 ft) = 8900 ft ·lb.

E12-7 Apply Eq. 12-15,

Kf+Uf=Ki+Ui,

2mvf2+mgyf=1

2mvi2+mgyi,

2vf2+g(−r) = 1

2(0)2+g(0).

Rearranging,

vf=p−2g(−r) = p−2(9.81 m/s2)(−0.236 m) = 2.15 m/s.

144

E12-8 (a) K=1

2mv2=1

2(2.40 kg)(150 m/s)2= 2.70×104J.

(b) Assuming that the ground is zero, U=mgy = (2.40 kg)(9.81 m/s2)(125 m) = 2.94×103J.

vf=s2(2.70×104J) + (2.94×103J)

(2.40 kg) = 158 m/s.

Only (a) and (b) depend on the mass.

E12-9 (a) Since ∆y= 0, then ∆U= 0 and ∆K= 0. Consequently, at B,v=v0.

(b) At C KC=KA+UA−UC, or

2mvC2=1

2mv02+mgh −mg h

vB=rv02+ 2gh

2=pv02+gh.

2mvD2=1

2mv02+mgh −mg(0),

vB=pv02+ 2gh.

E12-10 From the slope of the graph, k= (0.4 N)/(0.04 m) = 10 N/m.

(a) ∆K=−∆U, so 1

2mvf2=1

2kxi2, or

vf=s(10 N/m)

(0.00380 kg) (0.0550 m) = 2.82 m/s.

(b) ∆K=−∆U, so 1

2mvf2=1

2k(xi2−xf2), or

vf=s(10 N/m)

(0.00380 kg) [(0.0550 m)2−(0.0150 m)2] = 2.71 m/s.

E12-11 (a) The force constant of the spring is

k=F/x =mg/x = (7.94 kg)(9.81 m/s2)/(0.102 m) = 764 N/m.

(b) The potential energy stored in the spring is given by Eq. 12-8,

U=1

2kx2=1

2(764 N/m)(0.286 m + 0.102 m)2= 57.5 J.

Kf+Uf=Ki+Ui,

2mvf2+mgyf+1

2kxf2=1

2mvi2+mgyi+1

2kxi2,

2(0)2+mgh +1

2k(0)2=1

2(0)2+mg(0) + 1

2kxi2.

Rearranging,

h=k

2mg xi2=(764 N/m)

2(7.94 kg)(9.81 m/s2)(0.388 m)2= 0.738 m.

145

E12-12 The annual mass of water is m= (1000 kg/m3)(8 ×1012m2)(0.75 m). The change in

potential energy each year is then ∆U=−mgy, where y=−500 m. The power available is then

P=1

3(1000 kg/m3)(8×1012m2)(0.75 m)

(3.15×107m) (500 m) = 3.2×107W.

E12-13 (a) From kinematics, v=−gt, so K=1

2mg2t2and U=U0−K=mgh −1

2mg2t2.

(b) U=mgy so K=U0−U=mg(h−y).

E12-14 The potential energy is the same in both cases. Consequently, mgE∆yE=mgM∆yM, and

then

yM= (2.05 m −1.10 m)(9.81 m/s2)/(1.67 m/s2)+1.10 m = 6.68 m.

E12-15 The working is identical to Ex. 12-11,

Kf+Uf=Ki+Ui,

2mvf2+mgyf+1

2kxf2=1

2mvi2+mgyi+1

2kxi2,

2(0)2+mgh +1

2k(0)2=1

2(0)2+mg(0) + 1

2kxi2,

h=k

2mg xi2=(2080 N/m)

2(1.93 kg)(9.81 m/s2)(0.187 m)2= 1.92 m.

The distance up the incline is given by a trig relation,

d=h/ sin θ= (1.92 m)/sin(27◦) = 4.23 m.

E12-16 The vertical position of the pendulum is y=−lcos θ, where θis measured from the

downward vertical and lis the length of the string. The total mechanical energy of the pendulum is

E=1

2mvb2

if we set U= 0 at the bottom of the path and vbis the speed at the bottom. In this case

U=mg(l+y).

(a) K=E−U=1

2mvb2−mgl(1 −cos θ). Then

v=p(8.12 m/s)2−2(9.81 m/s2)(3.82 kg)(1 −cos 58.0◦) = 5.54 m/s.

(b) U=E−K, but at highest point K= 0. Then

θ= arccos 1−1

(8.12 m/s)2

(9.81 m/s2)(3.82 kg) = 83.1◦.

2(1.33 kg)(8.12 m/s)2= 43.8 J.

E12-17 The equilibrium position is when F=ky =mg. Then ∆Ug=−mgy and ∆Us=

2(ky)y=1

2mgy. So 2∆Us=−∆Ug.

146

E12-18 Let the spring get compressed a distance x. If the object fell from a height h= 0.436 m,

then conservation of energy gives 1

2kx2=mg(x+h). Solving for x,

x=mg

k±rmg

k2+ 2mg

only the positive answer is of interest, so

x=(2.14 kg)(9.81 m/s2)

(1860 N/m) ±s(2.14 kg)(9.81 m/s2)

(1860 N/m) 2

+ 2(2.14 kg)(9.81 m/s2)

(1860 N/m) (0.436 m) = 0.111 m.

E12-19 The horizontal distance traveled by the marble is R=vtf, where tfis the time of ﬂight

and vis the speed of the marble when it leaves the gun. We ﬁnd that speed using energy conservation

principles applied to the spring just before it is released and just after the marble leaves the gun.

Ki+Ui=Kf+Uf,

0 + 1

2kx2=1

2mv2+ 0.

Ki= 0 because the marble isn’t moving originally, and Uf= 0 because the spring is no longer

compressed. Substituting Rinto this,

2kx2=1

2mR

tf2

We have two values for the compression, x1and x2, and two ranges, R1and R2. We can put both

pairs into the above equation and get two expressions; if we divide one expression by the other we

get

x2

x12

=R2

R12

We can easily take the square root of both sides, then

=R2

R1was Bobby’s try, and was equal to 2.20 −0.27 = 1.93 m. x1= 1.1 cm was his compression. If

Rhoda wants to score, she wants R2= 2.2 m, then

x2=2.2 m

1.93 m1.1 cm = 1.25 cm.

E12-20 Conservation of energy— U1+K1=U2+K2— but U1=mgh,K1= 0, and U2= 0, so

K2=1

2mv2=mgh at the bottom of the swing.

The net force on Tarzan at the bottom of the swing is F=mv2/r, but this net force is equal to

the tension Tminus the weight W=mg. Then 2mgh/r =T−mg. Rearranging,

T= (180 lb) 2(8.5 ft)

(50 ft) + 1= 241 lb.

This isn’t enough to break the vine, but it is close.

147

E12-21 Let point 1 be the start position of the ﬁrst mass, point 2 be the collision point, and point

3 be the highest point in the swing after the collision. Then U1=K2, or 1

2m1v12=m1gd, where v1

is the speed of m1just before it collides with m2. Then v1=√2gd.

After the collision the speed of both objects is, by momentum conservation, v2=m1v1/(m1+m2).

Then, by energy conservation, U3=K0

2, or 1

2(m1+m2)v22= (m1+m2)gy, where yis the height

to which the combined masses rise.

Combining,

y=v22

2g=m12v12

2(m1+m2)2g=m1

m1+m22

E12-22 ∆K=−∆U, so 1

2mv2+1

2Iω2=mgh,

where I=1

2MR2and ω=v/R. Combining,

2mv2+1

4Mv2=mgh,

v=r4mgh

2m+M=s4(0.0487 kg)(9.81 m/s2)(0.540 m)

2(0.0487 kg) + (0.396 kg) = 1.45 m/s.

E12-23 There are three contributions to the kinetic energy: rotational kinetic energy of the shell

(Ks), rotational kinetic energy of the pulley (Kp), and translational kinetic energy of the block

(Kb). The conservation of energy statement is then

Ks,i+Kp,i+Kb,i+Ui=Ks,f+Kp,f+Kb,f+Uf,

(0) + (0) + (0) + (0) = 1

2Isωs2+1

2Ipωp2+1

2mvb2+mgy.

Finally, y=−hand

ωsR=ωpr=vb.

Combine all of this together, and our energy conservation statement will look like this:

0 = 1

22

3MR2vb

R2+1

2Ipvb

r2+1

2mvb2−mgh

which can be fairly easily rearranged into

vb2=2mgh

2M/3 + IP/r2+m.

E12-24 The angular speed of the ﬂywheel and the speed of the car are related by

k=ω

v=(1490 rad/s)

(24.0 m/s) = 62.1 rad/m.

The height of the slope is h= (1500 m) sin(5.00◦) = 131 m. The rotational inertia of the ﬂywheel is

I=1

(194 N)

(9.81 m/s2)(0.54 m)2= 2.88 kg ·m2.

148

(a) Energy is conserved as the car moves down the slope: Ui=Kf, or

mgh =1

2mv2+1

2Iω2=1

2mv2+1

2Ik2v2,

v=r2mgh

m+Ik2=s2(822 kg)(9.81 m/s2)(131 m)

(822 kg) + (2.88 kg ·m2)(62.1 rad/m)2= 13.3 m/s,

or 47.9 m/s.

(b) The average speed down the slope is 13.3 m/s/2=6.65 m/s. The time to get to the bottom

is t= (1500 m)/(6.65 m/s) = 226 s. The angular acceleration of the disk is

α=ω

t=(13.3 m/s)(62.1 rad/m)

(226 s) = 3.65 rad/s2.

P= (2.88 kg ·m2)(3.65 rad/s2)(13.3 m/s)(62.1 rad/m) = 8680 W.

E12-25 (a) For the solid sphere I=2

5mr2; if it rolls without slipping ω=v/r; conservation of

energy means Ki=Uf. Then

2mv2+1

22

5mr2v

r2=mgh.

h=(5.18 m/s)2

2(9.81 m/s2)+(5.18 m/s)2

5(9.81 m/s2)= 1.91 m.

The distance up the incline is (1.91 m)/sin(34.0◦) = 3.42 m.

(b) The sphere will travel a distance of 3.42 m with an average speed of 5.18 m/2, so t=

(3.42 m)/(2.59 m/s) = 1.32 s. But wait, it goes up then comes back down, so double this time to get

2.64 s.

E12-26 Conservation of energy means 1

2mv2+1

2Iω2=mgh. But ω=v/r and we are told

h= 3v2/4g, so

2mv2+1

2Iv2

r2=mg 3v2

4g,

I= 2r23

4m−1

2m=1

2mr2,

which could be a solid disk or cylinder.

E12-27 We assume the cannon ball is solid, so the rotational inertia will be I= (2/5)MR2

The normal force on the cannon ball will be N=Mg, where Mis the mass of the bowling ball.

The kinetic friction on the cannon ball is Ff=µkN=µkMg. The magnitude of the net torque on

the bowling ball while skidding is then τ=µkMgR.

Originally the angular momentum of the cannon ball is zero; the ﬁnal angular momentum will

have magnitude l=Iω =Iv/R, where vis the ﬁnal translational speed of the ball.

The time requires for the cannon ball to stop skidding is the time required to change the angular

momentum to l, so

∆t=∆l

τ=(2/5)MR2v/R

µkMgR =2v

5µkg.

149

Since we don’t know ∆t, we can’t solve this for v. But the same time through which the angular

momentum of the ball is increasing the linear momentum of the ball is decreasing, so we also have

∆t=∆p

−Ff

=Mv −Mv0

−µkMg =v0−v

µkg.

Combining,

5µkg=v0−v

µkg,

2v= 5(v0−v),

v= 5v0/7

E12-28

0123456

-2

-1

F(x) (N)

K(x) (J)

x (m)x(m)

E12-29 (a) F=−∆U/∆x=−[(−17 J) −(−3 J)]/[(4 m) −(1 m)] = 4.7 N.

(b) The total energy is 1

2(2.0 kg)(−2.0 m/s)2+ (−7 J), or −3 J. The particle is constrained to

move between x= 1 m and x= 14 m.

E12-30 Energy is conserved, so 1

2mv2

0=1

2mv2+mgy,

v=qv2

0−2gy,

which depends only on y.

E12-31 (a) We can ﬁnd Fxand Fyfrom the appropriate derivatives of the potential,

Fx=−∂U

∂x =−kx,

Fy=−∂U

∂y =−ky.

The force at point (x, y) is then

F=Fxˆ

i+Fyˆ

j=−kxˆ

i−kyˆ

(b) Since the force vector points directly toward the origin there is no angular component, and

Fθ= 0. Then Fr=−kr where ris the distance from the origin.

150

E12-32 (a) By symmetry we expect Fx,Fy, and Fzto all have the same form.

Fx=−∂U

∂x =−kx

(x2+y2+z2)3/2,

with similar expressions for Fyand Fz. Then

F=−k

(x2+y2+z2)3/2(xˆ

i+yˆ

j+zˆ

k).

(b) In spherical polar coordinates r2=x2+y2+z2. Then U=−k/r and

Fr=−∂U

∂r =−k

r2.

E12-33 We’ll just do the paths, showing only non-zero terms.

Path 1: W=Rb

0(−k2a)dy) = −k2ab.

Path 2: W=Ra

0(−k1b)dx) = −k1ab.

Path 3: W= (cos φsin φ)Rd

0(−k1−k2)r dr =−(k1+k2)ab/2.

These three are only equal if k1=k2.

P12-1 (a) We need to integrate an expression like

−Zz

∞

(z+l)2dz =k

z+l.

The second half is dealt with in a similar manner, yielding

U(z) = k

z+l−k

z−l.

(b) If zlthen we can expand the denominators, then

U(z) = k

z+l−k

z−l,

≈k

z−kl

z2−k

z+kl

z2,

=−2kl

z2.

P12-2 The ball just reaches the top, so K2= 0. Then K1=U2−U1=mgL, so v1=

p2(mgL)/m =√2gL.

P12-3 Measure distances along the incline by x, where x= 0 is measured from the maximally

compressed spring. The vertical position of the mass is given by xsin θ. For the spring k=

(268 N)/(0.0233 m) = 1.15×104N/m. The total energy of the system is

2(1.15×104N/m)(0.0548 m)2= 17.3 J.

(a) The block needs to have moved a vertical distance xsin(32.0◦), where

17.3 J = (3.18 kg)(9.81 m/s2)xsin(32.0◦),

or x= 1.05 m.

(b) When the block hits the top of the spring the gravitational potential energy has changed by

∆U=−(3.18 kg)(9.81 m/s2)(1.05 m −0.0548 m) sin(32.0◦) = 16.5,J;

hence the speed is v=p2(16.5 J)/(3.18 kg) = 3.22 m/s.

151

P12-4 The potential energy associated with the hanging part is

U=Z0

−L/4

Lgy dy =Mg

2Ly2

−L/4

=−MgL

32 ,

so the work required is W=M gL/32.

P12-5 (a) Considering points Pand Qwe have

KP+UP=KQ+UQ,

(0) + mg(5R) = 1

2mv2+mg(R),

4mgR =1

2mv2,

p8gR =v.

There are two forces on the block, the normal force from the track,

N=mv2

R=m(8gR)

R= 8mg,

and the force of gravity W=mg. They are orthogonal so

Fnet =p(8mg)2+ (mg)2=√65 mg

and the angle from the horizontal by

tan θ=−mg

8mg =−1

or θ= 7.13◦below the horizontal.

(b) If the block barely makes it over the top of the track then the speed at the top of the loop

(point S, perhaps?) is just fast enough so that the centripetal force is equal in magnitude to the

weight,

mvS2/R =mg.

Assume the block was released from point T. The energy conservation problem is then

KT+UT=KS+US,

(0) + mgyT=1

2mv2

S+mgyS,

yT=1

2(R) + m(2R),

= 5R/2.

P12-6 The wedge slides to the left, the block to the right. Conservation of momentum requires

Mvw+mvb,x = 0. The block is constrained to move on the surface of the wedge, so

tan α=vb,y

vb,x −vw

vb,y =vb,x tan α(1 + m/M).

152

Conservation of energy requires

2mvb2+1

2Mvw2=mgh.

Combining,

2mvb,x2+vb,y 2+1

2Mm

Mvb,x2=mgh,

tan2α(1 + m/M )2+1+ m

Mvb,x2= 2gh,

sin2α(M+m)2+M2cos2α+mM cos2αvb,x2= 2M2gh cos2α,

M2+mM +mM sin2α+m2sin2αvb,x2= 2M2gh cos2α,

(M+m)(M+msin2α)vb,x2= 2M2gh cos2α,

vb,x =Mcos αs2gh

(M+m)(M+msin2α).

Then

vw=−mcos αs2gh

(M+m)(M+msin2α).

P12-7 U(x) = −RFxdx =−Ax2/2−Bx3/3.

(a) U=−(−3.00 N/m)(2.26 m)2/2−(−5.00 N/m2)(2.26 m)3/3 = 26.9 J.

(b) There are two points to consider:

U1=−(−3.00 N/m)(4.91 m)2/2−(−5.00 N/m2)(4.91 m)3/3 = 233 J,

U2=−(−3.00 N/m)(1.77 m)2/2−(−5.00 N/m2)(1.77 m)3/3 = 13.9 J,

K1=1

2(1.18kg)(4.13 m/s)2= 10.1 J.

Then

v2=s2(10.1 J + 233 J −13.9 J)

(1.18 kg) = 19.7 m/s.

P12-8 Assume that U0=K0= 0. Then conservation of energy requires K=−U; consequently,

v=p2g(−y).

(a) v=p2(9.81 m/s2)(1.20 m) = 4.85 m/s.

(b) v=p2(9.81 m/s2)(1.20 m −0.45 m −0.45 m) = 2.43 m/s.

P12-9 Assume that U0=K0= 0. Then conservation of energy requires K=−U; consequently,

v=p2g(−y). If the ball barely swings around the top of the peg then the speed at the top of the

loop is just fast enough so that the centripetal force is equal in magnitude to the weight,

mv2/R =mg.

The energy conservation problem is then

mv2= 2mg(L−2(L−d)) = 2mg(2d−L)

mg(L−d)=2mg(2d−L),

d= 3L/5.

153

P12-10 The speed at the top and the speed at the bottom are related by

2mvb2=1

2mvt2+ 2mgR.

The magnitude of the net force is F=mv2/R, the tension at the top is

Tt=mvt2/R −mg,

while tension at the bottom is

Tb=mvb2/R +mg,

The diﬀerence is

∆T= 2mg +m(vb2−vt2)/R = 2mg + 4mg = 6mg.

P12-11 Let the angle θbe measured from the horizontal to the point on the hemisphere where

the boy is located. There are then two components to the force of gravity— a component tangent

to the hemisphere, W|| =mg cos θ, and a component directed radially toward the center of the

hemisphere, W⊥=mg sin θ.

While the boy is in contact with the hemisphere the motion is circular so

mv2/R =W⊥−N.

When the boy leaves the surface we have mv2/R =W⊥, or mv2=mgR sin θ. Now for energy

conservation,

K+U=K0+U0,

2mv2+mgy =1

2m(0)2+mgR,

2gR sin θ+mgy =mgR,

2y+y=R,

y= 2R/3.

P12-12 (a) To be in contact at the top requires mvt2/R =mg. The speed at the bottom would

be given by energy conservation

2mvb2=1

2mvt2+ 2mgR,

so vb=√5gR is the speed at the bottom that will allow the object to make it around the circle

without loosing contact.

(b) The particle will lose contact with the track if mv2/R ≤mg sin θ. Energy conservation gives

2mv2

0=1

2mv2+mgR(1 + sin θ)

for points above the half-way point. Then the condition for “sticking” to the track is

Rv2

0−2g(1 + sin θ)≤gsin θ,

or, if v0= 0.775vm,

5(0.775)2−2≤3 sin θ,

or θ= arcsin(1/3).

154

P12-13 The rotational inertia is

I=1

3ML2+ML2=4

3ML2.

Conservation of energy is 1

2Iω2= 3Mg(L/2),

so ω=p9g/(4L).

P12-14 The rotational speed of the sphere is ω=v/r; the rotational kinetic energy is Kr=

2Iω2=1

5mv2.

(a) For the marble to stay on the track mv2/R =mg at the top of the track. Then the marble

needs to be released from a point

mgh =1

2mv2+1

5mv2+ 2mgR,

or h=R/2 + R/5+2R= 2.7R.

(b) Energy conservation gives

6mgR =1

2mv2+1

5mv2+mgR,

or mv2/R = 50mg/7. This corresponds to the horizontal force acting on the marble.

P12-15 1

2mv2

0+mgy = 0, where yis the distance beneath the rim, or y=−rcos θ0. Then

v0=p−2gy =p2gr cos θ0.

P12-16 (a) For E1the atoms will eventually move apart completely.

(b) For E2the moving atom will bounce back and forth between a closest point and a farthest

point.

(d) K=E1−U≈2.2×10−19J.

(e) Find the slope of the curve, so

F≈ −(−1×10−19J) −(−2×10−19J)

(0.3×10−9m) −(0.2×10−9m) =−1×10−9N,

which would point toward the larger mass.

P12-17 The function needs to fall oﬀ at inﬁnity in both directions; an exponential envelope

would work, but it will need to have an −x2term to force the potential to zero on both sides. So we

propose something of the form

U(x) = P(x)e−βx2

where P(x) is a polynomial in xand βis a positive constant.

We proposed the polynomial because we need a symmetric function which has two zeroes. A

quadratic of the form αx2−U0would work, it has two zeroes, a minimum at x= 0, and is symmetric.

So our trial function is

U(x) = αx2−U0e−βx2.

155

This function should have three extrema. Take the derivative, and then we’ll set it equal to zero,

dx = 2αxe−βx2−2αx2−U0βxe−βx2.

Setting this equal to zero leaves two possibilities,

x= 0,

2α−2αx2−U0β= 0.

The ﬁrst equation is trivial, the second is easily rearranged to give

x=±sα+βU0

βα

These are the points ±x1. We can, if we wanted, try to ﬁnd αand βfrom the picture, but you

might notice we have one equation, U(x1) = U1and two unknowns. It really isn’t very illuminating

to take this problem much farther, but we could.

(b) The force is the derivative of the potential; this expression was found above.

in the well.

P12-18 (a) F=−∂U/∂r, or

F=−U0r0

r2+1

re−r/r0.

(b) Evaluate the force at the four points:

F(r0) = −2(U0/r0)e−1,

F(2r0) = −(3/4)(U0/r0)e−2,

F(4r0) = −(5/16)(U0/r0)e−4,

F(10r0) = −(11/100)(U0/r0)e−10.

The ratios are then

F(2r0)/F (r0) = (3/8)e−1= 0.14,

F(4r0)/F (r0) = (5/32)e−3= 7.8×10−3,

F(10r0)/F (r0) = (11/200)e−9= 6.8×10−6.

156

E13-1 If the projectile had not experienced air drag it would have risen to a height y2, but

because of air drag 68 kJ of mechanical energy was dissipated so it only rose to a height y1. In

either case the initial velocity, and hence initial kinetic energy, was the same; and the velocity at

the highest point was zero. Then W= ∆U, so the potential energy would have been 68 kJ greater,

and

∆y= ∆U/mg = (68×103J)/(9.4 kg)(9.81 m/s2) = 740 m

is how much higher it would have gone without air friction.

E13-2 (a) The road incline is θ= arctan(0.08) = 4.57◦. The frictional forces are the same; the car

is now moving with a vertical upward speed of (15 m/s) sin(4.57◦) = 1.20 m/s. The additional power

required to drive up the hill is then ∆P=mgvy= (1700 kg)(9.81 m/s2)(1.20 m/s) = 20000 W. The

total power required is 36000 W.

(b) The car will “coast” if the power generated by rolling downhill is equal to 16000 W, or

vy= (16000 W)/[(1700 kg)(9.81 m/s2)] = 0.959 m/s,

down. Then the incline is

θ= arcsin(0.959 m/s/15 m/s) = 3.67◦.

This corresponds to a downward grade of tan(3.67◦) = 6.4 %.

E13-3 Apply energy conservation:

2mv2+mgy +1

2ky2= 0,

v=p−2(9.81 m/s2)(−0.084 m) −(262 N/m)(−0.084 m)2/(1.25 kg) = 0.41 m/s.

E13-4 The car climbs a vertical distance of (225 m) sin(10◦) = 39.1 m in coming to a stop. The

change in energy of the car is then

∆E=−1

(16400 N)

(9.81 m/s2)(31.4 m/s)2+ (16400 N)(39.1 m) = −1.83×105J.

E13-5 (a) Applying conservation of energy to the points where the ball was dropped and where

it entered the oil,

2mvf2+mgyf=1

2mvi2+mgyi,

2vf2+g(0) = 1

2(0)2+gyi,

vf=p2gyi,

=p2(9.81 m/s2)(0.76 m) = 3.9 m/s.

(b) The change in internal energy of the ball + oil can be found by considering the points where

the ball was released and where the ball reached the bottom of the container.

∆E=Kf+Uf−Ki−Ui,

2mvf2+mgyf−1

2m(0)2−mgyi,

2(12.2×10−3kg)(1.48m/s)2−(12.2×10−3kg)(9.81m/s2)(−0.55m −0.76m),

=−0.143 J

157

E13-6 (a) Ui= (25.3 kg)(9.81 m/s2)(12.2 m) = 3030 J.

(b) Kf=1

2(25.3 kg)(5.56 m/s)2= 391 J.

E13-7 (a) At atmospheric entry the kinetic energy is

K=1

2(7.9×104kg)(8.0×103m/s)2= 2.5×1012J.

The gravitational potential energy is

U= (7.9×104kg)(9.8 m/s2)(1.6×105m) = 1.2×1011J.

The total energy is 2.6×1012J.

(b) At touch down the kinetic energy is

K=1

2(7.9×104kg)(9.8×101m/s)2= 3.8×108J.

E13-8 ∆E/∆t= (68 kg)(9.8 m/s2)(59 m/s) = 39000 J/s.

E13-9 Let mbe the mass of the water under consideration. Then the percentage of the potential

energy “lost” which appears as kinetic energy is

Kf−Ki

Ui−Uf

Then

Kf−Ki

Ui−Uf

2mvf2−vi2/(mgyi−mgyf),

=vf2−vi2

−2g∆y,

=(13 m/s)2−(3.2 m/s)2

−2(9.81 m/s2)(−15 m),

= 54 %.

The rest of the energy would have been converted to sound and thermal energy.

E13-10 The change in energy is

∆E=1

2(524 kg)(62.6 m/s)2−(524 kg)(9.81 m/s2)(292 m) = 4.74×105J.

E13-11 Uf=Ki−(34.6 J). Then

h=1

(7.81 m/s)2

(9.81 m/s2)−(34.6 J)

(4.26 kg)(9.81 m/s2)= 2.28 m;

which means the distance along the incline is (2.28 m)/sin(33.0◦) = 4.19 m.

158

E13-12 (a) Kf=Ui−Uf, so

vf=p2(9.81 m/s2)[(862 m) −(741 m)] = 48.7 m/s.

That’s a quick 175 km/h; but the speed at the bottom of the valley is 40% of the speed of sound!

(b) ∆E=Uf−Ui, so

∆E= (54.4 kg)(9.81 m/s2)[(862 m) −(741 m)] = −6.46×104J;

which means the internal energy of the snow and skis increased by 6.46×104J.

E13-13 The ﬁnal potential energy is 15% less than the initial kinetic plus potential energy of the

ball, so

0.85(Ki+Ui) = Uf.

But Ui=Uf, so Ki= 0.15Uf/0.85,and then

vi=r0.15

0.852gh =p2(0.176)(9.81 m/s2)(12.4 m) = 6.54 m/s.

E13-14 Focus on the potential energy. After the nth bounce the ball will have a potential energy

at the top of the bounce of Un= 0.9Un−1. Since U∝h, one can write hn= (0.9)nh0. Solving for n,

n= log(hn/h0)/log(0.9) = log(3 ft/6 ft)/log(0.9) = 6.58,

which must be rounded up to 7.

E13-15 Let mbe the mass of the ball and Mbe the mass of the block.

The kinetic energy of the ball just before colliding with the block is given by K1=U0, so

v1=p2(9.81 m/s2)(0.687 m) = 3.67 m/s.

Momentum is conserved, so if v2and v3are velocities of the ball and block after the collision

then mv1=mv2+Mv3. Kinetic energy is not conserved, instead

21

2mv2

1=1

2mv2

2+1

2Mv2

Combine the energy and momentum expressions to eliminate v3:

mv2

1= 2mv2

2+ 2Mm

M(v1−v2)2,

Mv2

1= 2Mv2

2+ 2mv2

1−4mv1v2+ 2mv2

which can be formed into a quadratic. The solution for v2is

v2=2m±p2(M2−mM)

2(M+m)v1= (0.600 ±1.95) m/s.

The corresponding solutions for v3are then found from the momentum expression to be v3=

0.981 m/s and v3= 0.219. Since it is unlikely that the ball passed through the block we can toss

out the second set of answers.

E13-16 Ef=Kf+Uf= 3mgh, or

vf=p2(9.81 m/s2)2(0.18 m) = 2.66 m/s.

159

E13-17 We can ﬁnd the kinetic energy of the center of mass of the woman when her feet leave

the ground by considering energy conservation and her highest point. Then

2mvi2+mgyi=1

2mvf2+mgyf,

2mvi=mg∆y,

= (55.0 kg)(9.81 m/s2)(1.20 m −0.90 m) = 162 J.

(a) During the jumping phase her potential energy changed by

∆U=mg∆y= (55.0 kg)(9.81 m/s2)(0.50 m) = 270 J

while she was moving up. Then

Fext =∆K+ ∆U

∆s=(162 J) + (270 J)

(0.5 m) = 864 N.

(b) Her fastest speed was when her feet left the ground,

v=2K

m=2(162 J)

(55.0 kg) = 2.42 m/s.

E13-18 (b) The ice skater needs to lose 1

2(116 kg)(3.24 m/s)2= 609 J of internal energy.

(a) The average force exerted on the rail is F= (609 J)/(0.340 m) = 1790 N.

E13-19 12.6 km/h is equal to 3.50 m/s; the initial kinetic energy of the car is

2(2340 kg)(3.50 m/s)2= 1.43×104J.

(a) The force exerted on the car is F= (1.43×104J)/(0.64 m) = 2.24×104N.

(b) The change increase in internal energy of the car is

∆Eint = (2.24×104N)(0.640 m −0.083 m) = 1.25×104J.

E13-20 Note that v2

n=v0

n2−2~

n·~

vcm +vcm2.Then

K=X

2mnv0

n2−2mn~

n·~

vcm +mnvcm2,

2mnv0

n2− X

mn~

n!·~

vcm + X

2mn!vcm2,

=Kint − X

mn~

n!·~

vcm +Kcm.

The middle term vanishes because of the deﬁnition of velocities relative to the center of mass.

160

E13-21 Momentum conservation requires mv0=mv+MV, where the sign indicates the direction.

We are assuming one dimensional collisions. Energy conservation requires

2mv2

0=1

2mv2+1

2MV 2+E.

Combining,

2mv2

0=1

2mv2+1

2Mm

Mv0−m

Mv2+E,

Mv2

0=Mv2+m(v0−v)2+ 2(M/m)E.

Arrange this as a quadratic in v,

(M+m)v2−(2mv0)v+2(M/m)E+mv2

0−Mv2

0= 0.

This will only have real solutions if the discriminant (b2−4ac) is greater than or equal to zero. Then

(2mv0)2≥4 (M+m)2(M/m)E+mv2

0−Mv2

0

is the condition for the minimum v0. Solving the equality condition,

4m2v2

0= 4(M+m)2(M/m)E+ (m−M)v2

0,

or M2v2

0= 2(M+m)(M/m)E. One last rearrangement, and v0=p2(M+m)E/(mM).

P13-1 (a) The initial kinetic energy will equal the potential energy at the highest point plus the

amount of energy which is dissipated because of air drag.

mgh +fh =1

2mv2

h=v2

2(g+f/m)=v2

2g(1 + f/w).

(b) The ﬁnal kinetic energy when the stone lands will be equal to the initial kinetic energy minus

twice the energy dissipated on the way up, so

2mv2=1

2mv2

0−2fh,

2mv2

0−2fv2

2g(1 + f/w),

=m

2−f

g(1 + f/w)v2

v2=1−2f

w+fv2

v=w−f

w+f1/2

v0.

P13-2 The object starts with U= (0.234 kg)(9.81 m/s2)(1.05 m) = 2.41 J. It will move back and

forth across the ﬂat portion (2.41 J)/(0.688 J) = 3.50 times, which means it will come to a rest at

the center of the ﬂat part while attempting one last right to left journey.

161

P13-3 (a) The work done on the block block because of friction is

(0.210)(2.41 kg)(9.81 m/s2)(1.83 m) = 9.09 J.

The energy dissipated because of friction is (9.09 J)/0.83 = 11.0 J.

The speed of the block just after the bullet comes to a rest is

v=p2K/m =p2(1.10 J)/(2.41 kg) = 3.02 m/s.

(b) The initial speed of the bullet is

v0=M+m

mv=(2.41 kg) + (0.00454 kg)

(0.00454 kg) (3.02 m/s) = 1.60×103m/s.

P13-4 The energy stored in the spring after compression is 1

2(193 N/m)(0.0416 m)2= 0.167 J.

Since 117 mJ was dissipated by friction, the kinetic energy of the block before colliding with the

spring was 0.284 J. The speed of the block was then

v=p2(0.284 J)/(1.34 kg) = 0.651 m/s.

P13-5 (a) Using Newton’s second law, F=ma, so for circular motion around the proton

mv2

r=F=ke2

r2.

The kinetic energy of the electron in an orbit is then

K=1

2mv2=1

2ke2

The change in kinetic energy is

∆K=1

2ke21

r2−1

r1.

(b) The potential energy diﬀerence is

∆U=−Zr2

ke2

r2dr =−ke21

r2−1

r1.

∆E= ∆K+ ∆U=−1

2ke21

r2−1

r1.

P13-6 (a) The initial energy of the system is (4000 lb)(12ft) = 48,000 ft ·lb.The safety device

removes (1000 lb)(12ft) = 12,000 ft ·lb before the elevator hits the spring, so the elevator has a

kinetic energy of 36,000 ft ·lb when it hits the spring. The speed of the elevator when it hits the

spring is

v=s2(36,000 ft ·lb)(32.0 ft/s2)

(4000 lb) = 24.0 ft/s.

(b) Assuming the safety clamp remains in eﬀect while the elevator is in contact with the spring

then the distance compressed will be found from

36,000 ft ·lb = 1

2(10,000 lb/ft)y2−(4000 lb)y+ (1000 lb)y.

162

This is a quadratic expression in ywhich can be simpliﬁed to look like

5y2−3y−36 = 0,

which has solutions y= (0.3±2.7) ft. Only y= 3.00 ft makes sense here.

33,000 ft = (4000 lb)y−(1000 lb)y,

where yis measured from the most compressed point of the spring. Then y= 11 ft, or the elevator

bounces back up 8 feet.

(d) The elevator will bounce until it has traveled a total distance so that the safety device

dissipates all of the original energy, or 48 ft.

P13-7 The net force on the top block while it is being pulled is

11.0 N −Ff= 11.0 N −(0.35)(2.5 kg)(9.81 m/s2) = 2.42 N.

This means it is accelerating at (2.42 N)/(2.5 kg) = 0.968 m/s2. That acceleration will last a time

t=p2(0.30 m)/(0.968 m/s2)=0.787 s. The speed of the top block after the force stops pulling is

then (0.968 m/s2)(0.787 s) = 0.762 m/s. The force on the bottom block is Ff, so the acceleration of

the bottom block is

(0.35)(2.5 kg)(9.81 m/s2)/(10.0 kg) = 0.858 m/s2,

and the speed after the force stops pulling on the top block is (0.858 m/s2)(0.787 s) = 0.675 m/s.

(a) W=F s = (11.0 N)(0.30 m) = 3.3 J of energy were delivered to the system, but after the

force stops pulling only

2(2.5 kg)(0.762 m/s)2+1

2(10.0 kg)(0.675 m/s)2= 3.004 J

were present as kinetic energy. So 0.296 J is “missing” and would be now present as internal energy.

(b) The impulse received by the two block system is then J= (11.0 N)(0.787 s) = 8.66 N·s. This

impulse causes a change in momentum, so the speed of the two block system after the external

force stops pulling and both blocks move as one is (8.66 N·s)(12.5 kg) = 0.693 m/s. The ﬁnal kinetic

energy is 1

2(12.5 kg)(0.693 m/s)2= 3.002 J;

this means that 0.002 J are dissipated.

P13-8 Hmm.

163

E14-1 FS/F E=MSrE2/M ErS2, since everything else cancels out in the expression. Then

=(1.99×1030kg)(3.84×108m)2

(5.98×1024)(1.50×1011m)2= 2.18

E14-2 Consider the force from the Sun and the force from the Earth. FS/F E=MSrE2/MErS2,

since everything else cancels out in the expression. We want the ratio to be one; we are also

constrained because rE+rS=Ris the distance from the Sun to the Earth. Then

ME(R−rE)2=MSrE2,

R−rE=rMS

rE,

rE= (1.50×1011m)/ 1 + s(1.99×1030kg)

(5.98×1024)!= 2.6×108m.

E14-3 The masses of each object are m1= 20.0 kg and m2= 7.0 kg; the distance between the

centers of the two objects is 15 + 3 = 18 m.

The magnitude of the force from Newton’s law of gravitation is then

F=Gm1m2

r2=(6.67×10−11N·m2/kg2)(20.0 kg)(7.0 kg)

(18 m)2= 2.9×10−11N.

E14-4 (a) The magnitude of the force from Newton’s law of gravitation is

F=Gm1m2

r2=(6.67×10−11N·m2/kg2)(12.7 kg)(9.85×10−3kg)

(0.108 m)2= 7.15×10−10N.

(b) The torque is τ= 2(0.262 m)(7.15×10−10N) = 3.75×10−10N·m.

E14-5 The force of gravity on an object near the surface of the earth is given by

F=GMm

(re+y)2,

where Mis the mass of the Earth, mis the mass of the object, reis the radius of the Earth, and

yis the height above the surface of the Earth. Expand the expression since yre. We’ll use a

Taylor expansion, where F(re+y)≈F(re) + y∂F/∂re;

F≈GMm

e−2yGMm

Since we are interested in the diﬀerence between the force at the top and the bottom, we really want

∆F= 2yGMm

= 2 y

GMm

= 2 y

where in the last part we substituted for the weight, which is the same as the force of gravity,

W=GMm

Finally,

∆F= 2(411 m)/(6.37×106m)(120 lb) = 0.015 lb.

164

E14-6 g∝1/r2, so g1/g2=r2

2/r2

1. Then

r2=p(9.81 m/s2)/(7.35 m/s2)(6.37×106m) = 7.36×106m.

That’s 990 kilometers above the surface of the Earth.

E14-7 (a) a=GM/r2, or

a=(6.67×10−11N·m2/kg2)(1.99×1030kg)

(10.0×103m)2= 1.33×1012m/s2.

(b) v=√2ax =p2(1.33×1012m/s2)(1.2 m/s) = 1.79×106m/s.

E14-8 (a) g0=GM/r2, or

g0=(6.67×10−11N·m2/kg2)(7.36×1022kg)

(1.74×106m)2= 1.62 m/s2.

(b) Wm=We(gm/ge) so

Wm= (100 N)(1.62 m/s2/9.81 m/s2) = 16.5 N.

r=pGM/g =q(6.67×10−11N·m2/kg2)(5.98×1024kg)/(1.62 m/s2) = 1.57×107m.

That’s 2.46 Earth radii, or 1.46 Earth radii above the surface of the Earth.

E14-9 The object fell through y=−10.0 m; the time required to fall would then be

t=p−2y/g =p−2(−10.0 m)/(9.81 m/s2) = 1.43 s.

We are interested in the error, that means taking the total derivative of y=−1

2gt2.and getting

δy =−1

2δg t2−gt δt.

δy = 0 so −1

2δg t =gδ t, which can be rearranged as

δt =−δg t

The percentage error in tneeds to be δt/t = 0.1 %/2=0.05 %. The absolute error is then δt =

(0.05 %)(1.43 s) = 0.7 ms.

E14-10 Treat mass which is inside a spherical shell as being located at the center of that shell.

Ignore any contributions from shells farther away from the center than the point in question.

(a) F=G(M1+M2)m/a2.

(b) F=G(M1)m/b2.

165

E14-11 For a sphere of uniform density and radius R > r,

M(r)

3πr3=M

3πR3,

where Mis the total mass.

The force of gravity on the object of mass mis then

F=GMm

R3=GMmr

R3.

gis the free-fall acceleration of the object, and is the gravitational force divided by the mass, so

g=GMr

R3=GM

R=GM

R−D

Since Ris the distance from the center to the surface, and Dis the distance of the object beneath

the surface, then r=R−Dis the distance from the center to the object. The ﬁrst fraction is the

free-fall acceleration on the surface, so

g=GM

R−D

R=gs

R−D

R=gs1−D

R

E14-12 The work required to move the object is GM Sm/r, where ris the gravitational radius.

But if this equals mc2we can write

mc2=GMSm/r,

r=GMS/c2.

For the sun, r= (6.67×10−11N·m2/kg2)(1.99×1030kg)/(3.00×108m/s)2= 1.47×103m. That’s

2.1×10−6RS.

E14-13 The distance from the center is

r= (80000)(3.00×108m/s)(3.16×107s) = 7.6×1020m.

The mass of the galaxy is

M= (1.4×1011)(1.99×1030kg) = 2.8×1041kg.

The escape velocity is

v=p2GM/r =q2(6.67×10−11N·m2/kg2)(2.8×1041kg)/(7.6×1020m) = 2.2×105m/s.

E14-14 Staying in a circular orbit requires the centripetal force be equal to the gravitational force,

mvorb2/r =GMm/r2,

or mvorb2=GMm/r. But −GMm/r is the gravitational potential energy; to escape one requires a

kinetic energy

mvesc2/2 = GMm/r =mvorb2,

which has solution vesc =√2vorb.

166

E14-15 (a) Near the surface of the Earth the total energy is

E=K+U=1

2m2pgRE2−GMEm

but

g=GM

RE2,

so the total energy is

E= 2mgRE−GMEm

= 2mGM

RE2RE−GMEm

=GMEm

This is a positive number, so the rocket will escape.

(b) Far from earth there is no gravitational potential energy, so

2mv2=GMEm

=GME

RE2mRE=gmRE,

with solution v=√2gRE.

E14-16 The rotational acceleration of the sun is related to the galactic acceleration of free fall by

4π2mr/T 2=GNm2/r2,

where Nis the number of “sun” sized stars of mass m,ris the size of the galaxy, Tis period of

revolution of the sun. Then

N=4π2r3

GmT 2=4π2(2.2×1020m)3

(6.67×10−11N·m2/kg2)(2.0×1030kg)(7.9×1015s)2= 5.1×1010.

E14-17 Energy conservation is Ki+Ui=Kf+Uf, but at the highest point Kf= 0, so

Uf=Ki+Ui,

−GMEm

R=1

2mv2

0−GMEm

R=1

RE−1

2GME

R=1

(6.37×106m) −(9.42×103m/s)2)

2(6.67×10−11N·m2/kg2)(5.98×1024kg) ,

R= 2.19×107m.

The distance above the Earth’s surface is 2.19×107m−6.37×106m=1.55×106m.

167

E14-18 (a) Free-fall acceleration is g=GM/r2. Escape speed is v=p2GM/r. Then v=√2gr =

p2(1.30 m/s2)(1.569×106m) = 2.02×103m/s.

(b) Uf=Ki+Ui. But U/m =−g0r2

0/r, so

(1.569×106m) −(1.01×103m/s)2

2(1.30 m/s2)(1.569×106m)2=1

2.09×106m.

That’s 523 km above the surface.

0/r, so

v=p2(1.30 m/s2)(1.569×106m)2[1/(1.569×106m) −1/(2.569×106m)] = 1260 m/s.

(d) M=gr2/G, or

M= (1.30 m/s2)(1.569×106m)2/(6.67×10−11N·m2/kg2) = 4.8×1022kg.

E14-19 (a) Apply ∆K=−∆U. Then mv2=Gm2(1/r2−1/r1), so

v=s(6.67×10−11N·m2/kg2)(1.56×1030kg) 1

(4.67×104m) −1

(9.34×104m) = 3.34×107m/s.

(b) Apply ∆K=−∆U. Then mv2=Gm2(1/r2−1/r1), so

v=s(6.67×10−11N·m2/kg2)(1.56×1030kg) 1

(1.26×104m) −1

(9.34×104m) = 5.49×107m/s.

E14-20 Call the particles 1 and 2. Then conservation of momentum requires the particle to have

the same momentum of the same magnitude, p=mv1=M v2. The momentum of the particles is

given by

2mp2+1

2Mp2=GMm

m+M

mM p2= 2GMm/d,

p=mMp2G/d(m+M).

Then vrel =|v1|+|v2|is equal to

vrel =mMp2G/d(m+M)1

m+1

M,

=mMp2G/d(m+M)m+M

mM ,

=p2G(m+M)/d

E14-21 The maximum speed is mv2=Gm2/d, or v=pGm/d.

E14-22 T2

1/r3

1=T2

2/r3

2, or

T2=T1(r2/r1)3/2= (1.00 y)(1.52)3/2= 1.87 y.

168

E14-23 We can use Eq. 14-23 to ﬁnd the mass of Mars; all we need to do is rearrange to solve

for M—

M=4π2r3

GT 2=4π2(9.4×106m)3

(6.67×10−11N·m2/kg2)(2.75×104s)2= 6.5×1023kg.

E14-24 Use GM/r2= 4π2r/T 2, so M= 4π2r3/GT 2, and

M=4π2(3.82×108m)3

(6.67×10−11N·m2/kg2)(27.3×86400 s)2= 5.93×1024kg.

E14-25 T2

1/r3

1=T2

2/r3

2, or

T2=T1(r2/r1)3/2= (1.00 month)(1/2)3/2= 0.354 month.

E14-26 Geosynchronous orbit was found in Sample Problem 14-8 to be 4.22×107m. The latitude

is given by

θ= arccos(6.37×106m/4.22×107m) = 81.3◦.

E14-27 (b) Make the assumption that the altitude of the satellite is so low that the radius of

the orbit is eﬀectively the radius of the moon. Then

T2=4π2

GM r3,

=4π2

(6.67×10−11N·m2/kg2)(7.36×1022kg) (1.74×106m)3= 4.24×107s2.

So T= 6.5×103s.

(a) The speed of the satellite is the circumference divided by the period, or

v=2πr

T=2π(1.74×106m)

(6.5×103s) = 1.68×103m/s.

E14-28 The total energy is −GMm/2a. Then

2mv2−GMm

r=−GMm

2a,

v2=GM 2

r−1

a.

E14-29 ra=a(1 + e), so from Ex. 14-28,

va=sGM 2

a(1 + e)−1

a;

rp=a(1 −e), so from Ex. 14-28,

vp=sGM 2

a(1 −e)−1

a;

Dividing one expression by the other,

vp=vas2/(1 −e)−1

2/(1 + e)−1= (3.72 km/s)s2/0.12 −1

2/1.88 −1= 58.3 km/s.

169

E14-30 (a) Convert.

G=6.67×10−11 m3

kg ·s21.99×1030kg

MS3.156×107s

y2AU

1.496×1011m3

which is G= 39.49 AU3/MS2·y2.

(b) Here is a hint: 4π2= 39.48. Kepler’s law then looks like

T2=MS2·y2

AU3r3

E14-31 Kepler’s third law states T2∝r3, where ris the mean distance from the Sun and Tis

the period of revolution. Newton was in a position to ﬁnd the acceleration of the Moon toward the

Earth by assuming the Moon moved in a circular orbit, since ac=v2/r = 4π2r/T 2. But this means

that, because of Kepler’s law, ac∝r/T 2∝1/r2.

E14-32 (a) The force of attraction between the two bodies is

F=GMm

(r+R)2.

The centripetal acceleration for the body of mass mis

rω2=GM

(r+R)2,

ω2=GM

r3(1 + R/r)2,

T2=4π2

GM r3(1 + R/r)2.

(b) Note that r=Md/(m+M) and R=md/(m+M). Then R/r =m/M, so the correction is

(1 + 5.94×1024/1.99×1030)2= 1.000006 for the Earth/Sun system and 1.025 for the Earth/Moon

system.

E14-33 (a) Use the results of Exercise 14-32. The center of mass is located a distance r=

2md/(m+ 2m)=2d/3 from the star of mass mand a distance R=d/3 from the star of mass 2m.

The period of revolution is then given by

T2=4π2

G(2m)2

3d31 + d/3

2d/32

=4π2

3Gmd3.

(b) Use Lm=mr2ω, then

=mr2

MR2=m(2d/3)2

(2m)(d/3)2= 2.

=mr2

MR2=m(2d/3)2

(2m)(d/3)2= 2.

170

E14-34 Since we don’t know which direction the orbit will be, we will assume that the satellite

on the surface of the Earth starts with zero kinetic energy. Then Ei=Ui.

∆U=Uf−Uito get the satellite up to the speciﬁed altitude. ∆K=Kf=−Uf/2. We want

to know if ∆U−∆Kis positive (more energy to get it up) or negative (more energy to put it in

orbit). Then we are interested in

∆U−∆K= 3Uf/2−Ui=GMm 1

ri−3

2rf.

The “break-even” point is when rf= 3ri/2 = 3(6400 km)/2 = 9600 km, which is 3200 km above the

Earth.

(a) More energy to put it in orbit.

(b) Same energy for both.

E14-35 (a) The approximate force of gravity on a 2000 kg pickup truck on Eros will be

F=GMm

r2=(6.67×10−11N·m2/kg2)(5.0×1015 kg)(2000 kg)

(7000 m)2= 13.6 N.

(b) Use

v=rGM

r=s(6.67×10−11N·m2/kg2)(5.0×1015 kg)

(7000 m) = 6.9 m/s.

E14-36 (a) U=−GMm/r. The variation is then

∆U= (6.67×10−11N·m2/kg2)(1.99×1030kg)(5.98×1024kg) 1

(1.47×1011m) −1

(1.52×1011m) 

= 1.78×1032J.

(b) ∆K+ ∆U= ∆E= 0, so |∆K|= 1.78×1032J.

(d) Since ∆l= 0 and l=mvr, we have

vp−va=vp1−rp

ra=vp1−(1.47×1011m)

(1.52×1011m) = 3.29×10−2vp.

But vp≈vav = 2π(1.5×1011m)/(3.16×107s) = 2.98×104m/s. Then ∆v= 981 m/s.

E14-37 Draw a triangle. The angle made by Chicago, Earth center, satellite is 47.5◦. The distance

from Earth center to satellite is 4.22×107m. The distance from Earth center to Chicago is 6.37×106m.

Applying the cosine law we ﬁnd the distance from Chicago to the satellite is

p(4.22×107m)2+ (6.37×106m)2−2(4.22×107m)(6.37×106m) cos(47.5◦) = 3.82×107m.

Applying the sine law we ﬁnd the angle made by Earth center, Chicago, satellite to be

arcsin (4.22×107m)

(3.82×107m) sin(47.5◦)= 126◦.

That’s 36◦above the horizontal.

171

E14-38 (a) The new orbit is an ellipse with eccentricity given by r=a0(1 + e). Then

e=r/a0−1 = (6.64×106m)/(6.52×106m) −1 = 0.0184.

The distance at P0is given by rP0=a0(1 −e). The potential energy at P0is

UP0=UP

1 + e

1−e= 2(−9.76×1010J) 1+0.0184

1−0.0184 =−2.03×1011J.

The kinetic energy at P0is then

KP0= (−9.94×1010J) −(−2.03×1011J) = 1.04×1011J.

That would mean v=p2(1.04×1011J)/(3250kg) = 8000 m/s.

(b) The average speed is

v=2π(6.52×106m)

(5240 s) = 7820 m/s.

E14-39 (a) The Starshine satellite was approximately 275 km above the surface of the Earth on

1 January 2000. We can ﬁnd the orbital period from Eq. 14-23,

T2=4π2

GM r3,

=4π2

(6.67×10−11N·m2/kg2)(5.98×1024kg) (6.65×106m)3= 2.91×107s2,

so T= 5.39×103s.

(b) Equation 14-25 gives the total energy of the system of a satellite of mass min a circular orbit

of radius raround a stationary body of mass Mm,

E=−GMm

2r.

We want the rate of change of this with respect to time, so

dt =GMm

2r2

We can estimate the value of dr/dt from the diagram. I’ll choose February 1 and December 1 as my

two reference points.

dt t=t0≈∆r

∆t=(240 km) −(300 km)

(62 days) ≈ −1 km/day

The rate of energy loss is then

dt =(6.67×10−11N·m2/kg2)(5.98×1024kg)(39 kg)

2(6.65×106m)2−1000 m

8.64×104s=−2.0 J/s.

P14-1 The object on the top experiences a force down from gravity W1and a force down from

the tension in the rope T. The object on the bottom experiences a force down from gravity W2and

a force up from the tension in the rope.

In either case, the magnitude of Wiis

Wi=GMm

172

where riis the distance of the ith object from the center of the Earth. While the objects fall they

have the same acceleration, and since they have the same mass we can quickly write

GMm

+T=GMm

2−T,

T=GMm

2r2

2−GMm

2r2

=GMm

21

1−1

2,

=GMm

2−r2

1r2

Now r1≈r2≈Rin the denominator, but r2=r1+l, so r2

2−r2

1≈2Rl in the numerator. Then

T≈GMml

R3.

P14-2 For a planet of uniform density, g=GM/r2=G(4πρr3/3)/r2= 4πGρr/3. Then if ρis

doubled while ris halved we ﬁnd that gwill be unchanged.

P14-3 (a) F=GMm/r2,a=F/m =GM/r.

(b) The acceleration of the Earth toward the center of mass is aE=F/M =Gm/r2. The

relative acceleration is then GM/r +Gm/r =G(m+M)/r. Only if Mmcan we assume that a

is independent of mrelative to the Earth.

P14-4 (a) g=GM/r2,δg =−(2GM/r3)δr. In this case δr =hand M= 4πρr3/3. Then

δW =m δg = 8πGρmh/3.

(b) ∆W/W = ∆g/g = 2h/r. Then an error of one part in a million will occur when his one

part in two million of r, or 3.2 meters.

P14-5 (a) The magnitude of the gravitational force from the Moon on a particle at Ais

FA=GMm

(r−R)2,

where the denominator is the distance from the center of the moon to point A.

(b) At the center of the Earth the gravitational force of the moon on a particle of mass mis

FC=GMm/r2.

FA−FC=GMm

(r−R)2−GMm

r2,

=GMm r2

r2(r−R)2−(r−R)2

r2(r−R)2,

=GMm r2−(r−R)2

r2(r−R)2,

=GMm R(2r−R)

r2(r−R)2.

173

To simplify assume Rrand then substitute (r−R)≈r. The force diﬀerence simpliﬁes to

FT=GMm R(2r)

r2(r)2=2GMmR

(d) Repeat part (c) except we want r+Rinstead of r−R. Then

FA−FC=GMm

(r+R)2−GMm

r2,

=GMm r2

r2(r+R)2−(r+R)2

r2(r+R)2,

=GMm r2−(r+R)2

r2(r+R)2,

=GMm −R(2r+R)

r2(r+R)2.

To simplify assume Rrand then substitute (r+R)≈r. The force diﬀerence simpliﬁes to

FT=GMm−R(2r)

r2(r)2=−2GMmR

The negative sign indicates that this “apparent” force points away from the moon, not toward it.

(e) Consider the directions: the water is eﬀectively attracted to the moon when closer, but

repelled when farther.

P14-6 Fnet =mrωs2, where ωsis the rotational speed of the ship. But since the ship is moving

relative to the earth with a speed v, we can write ωs=ω±v/r, where the sign is positive if the ship

is sailing east. Then Fnet =mr(ω±v/r)2.

The scale measures a force Wwhich is given by mg −Fnet, or

W=mg −mr(ω±v/r)2.

Note that W0=m(g−rω2). Then

W=W0

g−r(ω±v/r)2

g−rω2,

≈W01±2ωv

1−rω2,

≈W0(1 ±2vω/g).

P14-7 (a) a=GM/r2−rω2.ωis the rotational speed of the Earth. Since Frank observes a=g/2

we have

g/2 = GM/r2−rω2,

r2= (2GM −2r3ω2)/g,

r=p2(GM −r3ω2)/g

Note that

GM = (6.67×10−11N·m2/kg2)(5.98×1024kg) = 3.99×1014m3/s2

while

r3ω2= (6.37×106m)3(2π/86400 s)2= 1.37×1012m3/s2.

174

Consequently, r3ω2can be treated as a perturbation of GM bear the Earth. Solving iteratively,

r0=p2[(3.99×1014m3/s2)−(6.37×106m)3(2π/86400 s)2]/(9.81 m/s2) = 9.00×106m,

r1=p2[(3.99×1014m3/s2)−(9.00×106m)3(2π/86400 s)2]/(9.81 m/s2) = 8.98×106m,

which is close enough for me. Then h= 8.98×106m−6.37×106m = 2610 km.

(b) ∆E=Ef−Ei=Uf/2−Ui. Then

∆E= (6.67×10−11N·m2/kg2)(5.98×1024kg)(100 kg) 1

(6.37×106m) −1

2(8.98×106m) = 4.0×109J.

P14-8 (a) Equate centripetal force with the force of gravity.

4π2mr

T2=GMm

r2,

4π2

T2=G(4/3)πr3ρ

r3,

T=r3π

Gρ

(b) T=q3π/(6.7×10−11N·m2/kg2)(3.0×103kg/m3) = 6800 s.

P14-9 (a) One can ﬁnd δg by pretending the Earth is not there, but the material in the hole is.

Concentrate on the vertical component of the resulting force of attraction. Then

δg =GM

where ris the straight line distance from the prospector to the center of the hole and Mis the mass

of material that would ﬁll the hole. A few substitutions later,

δg =4πGρR3d

3(√d2+x2)3.

(b) Directly above the hole x= 0, so a ratio of the two readings gives

(10.0 milligals)

(14.0 milligals) =d2

d2+ (150 m)23/2

(0.800)(d2+ 2.25×104m2) = d2,

which has solution d= 300 m. Then

R3=3(14.0×10−5m/s2)(300 m)2

4π(6.7×10−11N·m2/kg2)(2800 kg/m3),

so R= 250 m. The top of the cave is then 300 m −250 m = 50 m beneath the surface.

(b) All of the formulae stay the same except replace ρwith the diﬀerence between rock and water.

ddoesn’t change, but Rwill now be given by

R3=3(14.0×10−5m/s2)(300 m)2

4π(6.7×10−11N·m2/kg2)(1800 kg/m3),

so R= 292 m, and then the cave is located 300 m −292 m = 7 m beneath the surface.

175

P14-10 g=GM/r2, where Mis the mass enclosed in within the sphere of radius r. Then

dg = (G/r2)dM −2(GM/r3)dr, so that gis locally constant if dM/dr = 2M/r. Expanding,

4πr2ρl= 8πr2ρ/3,

ρl= 2ρ/3.

P14-11 The force of gravity on the small sphere of mass mis equal to the force of gravity from

a solid lead sphere minus the force which would have been contributed by the smaller lead sphere

which would have ﬁlled the hole. So we need to know about the size and mass of the lead which was

removed to make the hole.

The density of the lead is given by

ρ=M

3πR3

The hole has a radius of R/2, so if the density is constant the mass of the hole will be

Mh=ρV =M

3πR34

3πR

23

The “hole” is closer to the small sphere; the center of the hole is d−R/2 away. The force of the

whole lead sphere minus the force of the “hole” lead sphere is

GMm

d2−G(M/8)m

(d−R/2)2

P14-12 (a) Use v=ω√R2−r2, where ω=pGME/R3. Then

T=ZT

dt =Z0

dr/dt =Z0

=Z0

ω√R2−r2,

=π

2ω

Knowing that

ω=s(6.67×10−11N·m2/kg2)(5.98×1024kg)

(6.37×106m)3= 1.24×10−3/s,

we can ﬁnd T= 1260 s = 21 min.

(b) Same time, 21 minutes. To do a complete journey would require four times this, or 2π/ω.

That’s 84 minutes!

P14-13 (a) g=GM/r2and M= 1.93×1024kg + 4.01×1024kg + 3.94×1022kg = 5.98×1024kg so

g= (6.67×10−11N·m2/kg2)(5.98×1024kg)/(6.37×106m)2= 9.83 m/s2.

(b) Now M= 1.93×1024kg + 4.01×1024kg = 5.94×1024kg so

g= (6.67×10−11N·m2/kg2)(5.94×1024kg)/(6.345×106m)2= 9.84 m/s2.

g= (6.67×10−11N·m2/kg2)(5.98×1024kg)(6.345×106m)/(6.37×106m)3= 9.79 m/s2.

176

P14-14 (a) Use g=GM/r2, then

g= (6.67×10−11N·m2/kg2)(1.93×1024kg)/(3.490×106m)2= 106 m/s2.

The variation with depth is linear if core has uniform density.

(b) In the mantle we have g=G(Mc+M)/r2, where Mis the amount of the mass of the mantle

which is enclosed in the sphere of radius r. The density of the core is

ρc=3(1.93×1024kg)

4π(3.490×106m)3= 1.084×104kg/m3.

The density of the mantle is harder to ﬁnd,

ρc=3(4.01×1024kg)

4π[(6.345×106m)3−(3.490×106m)3= 4.496×103kg/m3.

We can pretend that the core is made up of a point mass at the center and the rest has a density

equal to that of the mantle. That point mass would be

Mp=4π(3.490×106m)3(1.084×104kg/m3−4.496×103kg/m3)

3= 1.130×1024kg.

Then

g=GMp/r2+ 4πGρmr/3.

Find dg/dr, and set equal to zero. This happens when

2Mp/r3= 4πρm/3,

or r= 4.93×106m. Then g= 9.29 m/s2. Since this is less than the value at the end points it must

be a minimum.

P14-15 (a) We will use part of the hint, but we will integrate instead of assuming the bit about

gav; doing it this way will become important for later chapters. Consider a small horizontal slice of

the column of thickness dr. The weight of the material above the slice exerts a force F(r) on the

top of the slice; there is a force of gravity on the slice given by

dF =GM(r)dm

r2,

where M(r) is the mass contained in the sphere of radius r,

M(r) = 4

3πr3ρ.

Lastly, the mass of the slice dm is related to the thickness and cross sectional area by dm =ρA dr.

Then

dF =4πGAρ2

3r dr.

Integrate both sides of this expression. On the left the limits are 0 to Fcenter, on the right the limits

are Rto 0; we need to throw in an extra negative sign because the force increases as rdecreases.

Then

F=2

3πGAρ2R2.

Divide both sides by Ato get the compressive stress.

177

(b) Put in the numbers!

S=2

3π(6.67×10−11N·m2/kg2)(4000 kg/m3)2(3.0×105m)2= 2.0×108N/m2.

R=s3(4.0×107N/m2)

2π(6.67×10−11N·m2/kg2)(3000 kg/m3)2= 1.8×105m.

P14-16 The two mass increments each exert a vertical and a horizontal force on the particle, but

the horizontal components will cancel. The vertical component is proportional to the sine of the

angle, so that

dF =2Gm dm

r=2Gmλ dx

where r2=x2+y2. We will eventually integrate from 0 to ∞, so

F=Z∞

2Gmλ dx

= 2Gmλy Z∞

(x2+y2)3/2,

=2Gmλ

P14-17 For any arbitrary point Pthe cross sectional area which is perpendicular to the axis

dA0=r2dΩ is not equal to the projection dA onto the surface of the sphere. It depends on the angle

that the axis makes with the normal, according to dA0= cos θdA. Fortunately, the angle made at

point 1 is identical to the angle made at point 2, so we can write

dΩ1=dΩ2,

dA1/r2

1=dA2/r2

But the mass of the shell contained in dA is proportional to dA, so

1dm1=r2

2dm2,

Gm dm1/r2

1=Gm dm2/r2

Consequently, the force on an object at point Pis balanced by both cones.

(b) Evaluate RdΩ for the top and bottom halves of the sphere. Since every dΩ on the top is

balanced by one on the bottom, the net force is zero.

P14-18

P14-19 Assume that the small sphere is always between the two spheres. Then

W= ∆U1+ ∆U2,

= (6.67×10−11N·m2/kg2)(0.212 kg) [(7.16 kg) −(2.53 kg)] 1

(0.420 m) −1

(1.14 m),

= 9.85×10−11J.

178

P14-20 Note that 1

2mvesc2=−U0, where U0is the potential energy at the burn-out height.

Energy conservation gives

K=K0+U0,

2mv2=1

2mv2

0−1

2mvesc2,

v=qv2

0−vesc2.

P14-21 (a) The force of one star on the other is given by F=Gm2/d2, where dis the distance

between the stars. The stars revolve around the center of mass, which is halfway between the stars

so r=d/2 is the radius of the orbit of the stars. If ais the centripetal acceleration of the stars, the

period of revolution is then

T=r4π2r

a=r4mπ2r

F=r16π2r3

Gm .

The numerical value is

T=s16π2(1.12×1011m)3

(6.67×10−11N·m2/kg2)(3.22×1030kg) = 3.21×107s=1.02 y.

(b) The gravitational potential energy per kilogram midway between the stars is

−2Gm

r=−2(6.67×10−11N·m2/kg2)(3.22×1030kg)

(1.12×1011m) =−3.84×109J/kg.

An object of mass Mat the center between the stars would need (3.84×109J/kg)Mkinetic energy

to escape, this corresponds to a speed of

v=p2K/M =p2(3.84×109J/kg) = 8.76×104m/s.

P14-22 (a) Each diﬀerential mass segment on the ring contributes the same amount to the force

on the particle,

dF =Gm dm

where r2=x2+R2. Since the diﬀerential mass segments are all equal distance, the integration is

trivial, and the net force is

F=GMmx

(x2+R2)3/2.

(b) The potential energy can be found by integrating with respect to x,

∆U=Z∞

F dx =Z∞

GMmx

(x2+R2)3/2dx =GMm

Then the particle of mass mwill pass through the center of the ring with a speed v=p2∆U/m =

p2GM/R.

179

P14-23 (a) Consider the following diagram.

The distance ris given by the cosine law to be

r2=R2+R2−2R2cos θ= 2R2(1 −cos θ).

The force between two particles is then F=Gm2/r2.Each particle has a symmetric partner, so

only the force component directed toward the center contributes. If we call this the Rcomponent

we have

FR=Fcos α=Fcos(90◦−θ/2) = Fsin(θ/2).

Combining,

FR=Gm2

2R2

sin(θ/2)

1−cos θ.

But each of the other particles contributes to this force, so

Fnet =Gm2

2R2X

sin(θi/2)

1−cos θi

When there are only 9 particles the angles are in steps of 40◦; the θiare then 40◦, 80◦, 120◦, 160◦,

200◦, 240◦, 280◦, and 320◦. With a little patience you will ﬁnd

sin(θi/2)

1−cos θi

= 6.649655,

using these angles. Then Fnet = 3.32Gm2/R2.

(b) The rotational period of the ring would need to be

T=r4π2R

a=r4mπ2R

F=r16π2R3

3.32Gm.

P14-24 The potential energy of the system is U=−Gm2/r. The kinetic energy is mv2. The total

energy is E=−Gm2/d. Then

dt = 2pGm(1/r −1/d),

180

so the time to come together is

T=Z0

2pGm(1/r −1/d)=rd3

4Gm Z1

0rx

1−xdx =π

4rd3

Gm.

P14-25 (a) E=U/2 for each satellite, so the total mechanical energy is −GMm/r.

(b) Now there is no K, so the total mechanical energy is simply U=−2GMm/r. The factor of

2 is because there are two satellites.

P14-26 Let ra=a(1 + e) and rp=a(1 −e). Then ra+re= 2aand ra−rp= 2ae. So the answer

2(0.0167)(1.50×1011m) = 5.01×109m,

or 7.20 solar radii.

P14-27

P14-28 The net force on an orbiting star is

F=Gm M

r2+m4r2.

This is the centripetal force, and is equal to 4π2mr/T 2. Combining,

4π2

T2=G

4r3(4M+m),

so T= 4πpr3/[G(4M+m)].

P14-29 (a) v=pGM/r, so

v=q(6.67×10−11N·m2/kg2)(5.98×1024kg)/(7.01×106m) = 7.54×103m/s.

(b) T= 2π(7.01×106m)/(7.54×103m/s) = 5.84×103s.

E=−(6.67×10−11N·m2/kg2)(5.98×1024kg)(220kg)

2(7.01×106m) =−6.25×109J.

After 1500 orbits the energy is now −6.25×109J−(1500)(1.40×105J) = −6.46×109J. The new

distance from the Earth is then

r=−(6.67×10−11N·m2/kg2)(5.98×1024kg)(220kg)

2(−6.46×109J) = 6.79×106m.

The altitude is now 6790 −6370 = 420 km.

(d) F= (1.40×105J)/(2π7.01×106m) = 3.2×10−3N.

(e) No.

181

P14-30 Let the satellite Sbe directly overhead at some time. The magnitude of the speed is

equal to that of a geosynchronous satellite Twhose orbit is not inclined, but since there are both

parallel and perpendicular components to the motion of Sit will appear to move north while “losing

ground” compared to T. Eventually, though, it must pass overhead again in 12 hours. When Sis as

far north as it will go (6 hours) it has a velocity which is parallel to T, but it is located in a region

where the required speed to appear ﬁxed is slower. Hence, it will appear to be “gaining ground”

against the background stars. Consequently, the motion against the background stars appears to be

a ﬁgure 8.

P14-31 The net force of gravity on one star because of the other two is

F=2GM2

L2cos(30◦).

The stars orbit about a point r=L/2 cos(30◦) from any star. The orbital speed is then found from

Mv2

r=Mv2

L/2 cos(30◦)=2GM2

L2cos(30◦),

or v=pGM/L.

P14-32 A parabolic path will eventually escape; this means that the speed of the comet at any

distance is the escape speed for that distance, or v=p2GM/r. The angular momentum is constant,

and is equal to

l=mvArA=mp2GMrA.

For a parabolic path, r= 2rA/(1 + cos θ). Combining with Eq. 14-21 and the equation before that

one we get

dθ

dt =√2GMrA

4rA2(1 + cos θ)2.

The time required is the integral

T=r8rA3

GM Zπ/2

dθ

(1 + cos θ)2=r8rA3

GM 2

3.

Note that prA3/GM is equal to 1/2πyears. Then the time for the comet to move is

T=1

2π√82

3y = 0.300 y.

P14-33 There are three forces on loose matter (of mass m0) sitting on the moon: the force of

gravity toward the moon, Fm=Gmm0/a2,the force of gravity toward the planet, FM=GM m0/(r−

a)2,and the normal force Nof the moon pushing the loose matter away from the center of the moon.

The net force on this loose matter is FM+N−Fm, this value is exactly equal to the centripetal

force necessary to keep the loose matter moving in a uniform circle. The period of revolution of the

loose matter is identical to that of the moon,

T= 2πpr3/GM,

but since the loose matter is actually revolving at a radial distance r−athe centripetal force is

Fc=4π2m0(r−a)

T2=GMm0(r−a)

r3.

182

Only if the normal force is zero can the loose matter can lift oﬀ, and this will happen when Fc=

FM−Fm, or

M(r−a)

r3=M

(r−a)2−m

a2,

=Ma2−m(r−a)2

a2(r−a)2,

Ma2(r−a)3=Mr3a2−mr3(r−a)2,

−3r2a3+ 3ra4−a4=m

M−r5+ 2r4a−r3a2

Let r=ax, then xis dimensionless; let β=m/M , then βis dimensionless. The expression then

simpliﬁes to

−3x2+ 3x−1 = β(−x5+ 2x4−x3).

If we assume than xis very large (ra) then only the largest term on each side survives. This means

3x2≈βx5,or x= (3/β)1/3.In that case, r=a(3M/m)1/3. For the Earth’s moon rc= 1.1×107m,

which is only 4,500 km away from the surface of the Earth. It is somewhat interesting to note that

the radius ris actually independent of both aand mif the moon has a uniform density!

183

E15-1 The pressure in the syringe is

p=(42.3 N)

π(1.12×10−2m/s)2= 4.29×105Pa.

E15-2 The total mass of ﬂuid is

m= (0.5×10−3m3)(2600 kg/m3)+(0.25×10−3m3)(1000 kg/m3)+(0.4×10−3m3)(800 kg/m3) = 1.87 kg.

The weight is (18.7 kg)(9.8 m/s2) = 18 N.

E15-3 F=A∆p, so

F= (3.43 m)(2.08 m)(1.00 atm −0.962 atm)(1.01×105Pa/atm) = 2.74×104N.

E15-4 B∆V/V =−∆p;V=L3; ∆V≈L2∆L/3. Then

∆p= (140×109Pa)(5×10−3m)

3(0.85 m) = 2.74×109Pa.

E15-5 There is an inward force F1pushing the lid closed from the pressure of the air outside the

box; there is an outward force F2pushing the lid open from the pressure of the air inside the box.

To lift the lid we need to exert an additional outward force F3to get a net force of zero.

The magnitude of the inward force is F1=PoutA, where Ais the area of the lid and Pout is

the pressure outside the box. The magnitude of the outward force F2is F2=PinA. We are told

F3= 108 lb. Combining,

F2=F1−F3,

PinA=PoutA−F3,

Pin =Pout −F3/A,

so Pin = (15 lb/in2−(108 lb)/(12 in2) = 6.0 lb/in2.

E15-6 h= ∆p/ρg, so

h=(0.05 atm)(1.01×105Pa/atm)

(1000 kg/m3)(9.8 m/s2)= 0.52 m.

E15-7 ∆p= (1060 kg/m3)(9.81 m/s2)(1.83 m) = 1.90×104Pa.

E15-8 ∆p= (1024 kg/m3)(9.81 m/s2)(118 m) = 1.19×106Pa.Add this to p0; the total pressure is

then 1.29×106Pa.

E15-9 The pressure diﬀerential assuming we don’t have a sewage pump:

p2−p1=−ρg (y2−y1) = (926 kg/m3)(9.81 m/s2)(8.16 m −2.08 m) = 5.52×104Pa.

We need to overcome this pressure diﬀerence with the pump.

E15-10 (a) p= (1.00 atm)e−5.00/8.55 = 0.557 atm.

(b) h= (8.55 km) ln(1.00/0.500) = 5.93 km.

184

E15-11 The mercury will rise a distance aon one side and fall a distance aon the other so that

the diﬀerence in mercury height will be 2a. Since the masses of the “excess” mercury and the water

will be proportional, we have 2aρm=dρw, so

a=(0.112m)(1000 kg/m3)

2(13600 kg/m3)= 4.12×10−3m.

E15-12 (a) The pressure (due to the water alone) at the bottom of the pool is

P= (62.45 lb/ft3)(8.0 ft) = 500 lb/ft2.

The force on the bottom is

F= (500 lb/ft2)(80 ft)(30 ft) = 1.2×106lb.

The average pressure on the side is half the pressure on the bottom, so

F= (250 lb/ft2)(80 ft)(8.0 ft) = 1.6×105lb.

The average pressure on the end is half the pressure on the bottom, so

F= (250 lb/ft2)(30 ft)(8.0 ft) = 6.0×104lb.

(b) No, since that additional pressure acts on both sides.

E15-13 (a) Equation 15-8 can be used to ﬁnd the height y2of the atmosphere if the density is

constant. The pressure at the top of the atmosphere would be p2= 0, and the height of the bottom

y1would be zero. Then

y2= (1.01×105Pa)/(1.21 kg/m3)(9.81 m/s2)= 8.51×103m.

(b) We have to go back to Eq. 15-7 for an atmosphere which has a density which varies linearly

with altitude. Linear variation of density means

ρ=ρ01−y

ymax 

Substitute this into Eq. 15-7,

p2−p1=−Zymax

ρg dy,

=−Zymax

ρ0g1−y

ymax dy,

=−ρ0gy−y2

2ymax 

ymax

=−ρgymax/2.

In this case we have ymax = 2p1/(ρg), so the answer is twice that in part (a), or 17 km.

E15-14 ∆P= (1000 kg/m3)(9.8 m/s2)(112 m) = 1.1×106Pa.The force required is then F=

(1.1×106Pa)(1.22 m)(0.590 m) = 7.9×105N.

185

E15-15 (a) Choose any inﬁnitesimally small spherical region where equal volumes of the two ﬂuids

are in contact. The denser ﬂuid will have the larger mass. We can treat the system as being a sphere

of uniform mass with a hemisphere of additional mass being superimposed in the region of higher

density. The orientation of this hemisphere is the only variable when calculating the potential energy.

The center of mass of this hemisphere will be a low as possible only when the surface is horizontal.

So all two-ﬂuid interfaces will be horizontal.

(b) If there exists a region where the interface is not horizontal then there will be two diﬀerent

values for ∆p=ρgh, depending on the path taken. This means that there will be a horizontal

pressure gradient, and the ﬂuid will ﬂow along that gradient until the horizontal pressure gradient

is equalized.

E15-16 The mass of liquid originally in the ﬁrst vessel is m1=ρAh1; the center of gravity is

at h1/2, so the potential energy of the liquid in the ﬁrst vessel is originally U1=ρgAh2

1/2. A

similar expression exists for the liquid in the second vessel. Since the two vessels have the same

cross sectional area the ﬁnal height in both containers will be hf= (h1+h2)/2. The ﬁnal potential

energy of the liquid in each container will be Uf=ρgA(h1+h2)2/8. The work done by gravity is

then

W=U1+U2−2Uf,

=ρgA

42h2

1+ 2h2

2−(h2

1+ 2h1h2+h2

2),

=ρgA

4(h1−h2)2.

E15-17 There are three force on the block: gravity (W=mg), a buoyant force B0=mwg, and a

tension T0. When the container is at rest all three forces balance, so B0−W−T0= 0. The tension

in this case is T0= (mw−m)g.

When the container accelerates upward we now have B−W−T=ma. Note that neither the

tension nor the buoyant force stay the same; the buoyant force increases according to B=mw(g+a).

The new tension is then

T=mw(g+a)−mg −ma = (mw−m)(g+a) = T0(1 + a/g).

E15-18 (a) F1/d2

1=F2/d2

2, so

F2= (18.6 kN)(3.72 cm)2/(51.3 cm)2= 97.8 N.

(b) F2h2=F1h1, so

h2= (1.65 m)(18.6 kN)/(97.8 N) = 314 m.

E15-19 (a) 35.6 kN; the boat doesn’t get heavier or lighter just because it is in diﬀerent water!

(b) Yes.

∆V=(35.6×103N)

(9.81 m/s2)1

(1024 kg/m3)−1

(1000 kg/m3)=−8.51×10−2m3.

E15-20 (a) ρ2=ρ1(V1/V2) = (1000 kg/m3)(0.646) = 646 kg/m3.

(b) ρ2=ρ1(V1/V2) = (646 kg/m3)(0.918)−1= 704 kg/m3.

186

E15-21 The can has a volume of 1200 cm3, so it can displace that much water. This would

provide a buoyant force of

B=ρV g = (998kg/m3)(1200×10−6m3)(9.81 m/s2) = 11.7 N.

This force can then support a total mass of (11.7 N)/(9.81 m/s2) = 1.20 kg. If 130 g belong to the

can, then the can will be able to carry 1.07 kg of lead.

E15-22 ρ2=ρ1(V1/V2) = (0.98 g/cm3)(2/3)−1= 1.47 g/cm3.

E15-23 Let the object have a mass m. The buoyant force of the air on the object is then

Bo=ρa

ρo

mg.

There is also a buoyant force on the brass, equal to

Bb=ρa

ρb

mg.

The fractional error in the weighing is then

Bo−Bb

mg =(0.0012 g/cm3)

(3.4 g/cm3)−(0.0012 g/cm3)

(8.0 g/cm3)= 2.0×10−4

E15-24 The volume of iron is

Vi= (6130 N)/(9.81 m/s2)(7870 kg/m3) = 7.94×10−2m3.

The buoyant force of water is 6130 N −3970 N = 2160 N. This corresponds to a volume of

Vw= (2160 N)/(9.81 m/s2)(1000 kg/m3) = 2.20×10−1m3.

The volume of air is then 2.20×10−1m3−7.94×10−2m3= 1.41×10−1m3.

E15-25 (a) The pressure on the top surface is p=p0+ρgL/2. The downward force is

Ft= (p0+ρgL/2)L2,

=(1.01×105Pa) + (944 kg/m3)(9.81 m/s2)(0.608 m)/2(0.608 m)2= 3.84×104N.

(b) The pressure on the bottom surface is p=p0+ 3ρgL/2. The upward force is

Fb= (p0+ 3ρgL/2)L2,

=(1.01×105Pa) + 3(944 kg/m3)(9.81 m/s2)(0.608 m)/2(0.608 m)2= 4.05×104N.

T= (4450 N) + (3.84×104N) −(4.05×104N) = 2350 N.

(d) The buoyant force is

B=L3ρg = (0.6083)(944 kg/m3)(9.81 m/s2) = 2080 N.

187

E15-26 The ﬁsh has the (average) density of water if

ρw=mf

Vc+Va

Va=mf

ρw−Vc.

We want the fraction Va/(Vc+Va), so

Vc+Va

= 1 −ρw

= 1 −ρw/ρc= 1 −(1.024 g/cm3)/(1.08 g/cm3) = 5.19×10−2.

E15-27 There are three force on the dirigible: gravity (W=mgg), a buoyant force B=mag,

and a tension T. Since these forces must balance we have T=B−W. The masses are related to

the densities, so we can write

T= (ρa−ρg)V g = (1.21 kg/m3−0.796 kg/m3)(1.17×106m3)(9.81m/s2) = 4.75×106N.

E15-28 ∆m= ∆ρV , so

∆m= [(0.160 kg/m3)−(0.0810 kg/m3)](5000 m3) = 395 kg.

E15-29 The volume of one log is π(1.05/2 ft)2(5.80 ft) = 5.02 ft3. The weight of the log is

(47.3 lb/ft3)(5.02 ft3) = 237 lb. Each log if completely submerged will displace a weight of wa-

ter (62.4 lb/ft3)(5.02 ft3) = 313 lb. So each log can support at most 313 lb −237 lb = 76 lb. The

three children have a total weight of 247 lb, so that will require 3.25 logs. Round up to four.

E15-30 (a) The ice will hold up the automobile if

ρw>ma+mi

=ma

At +ρi.

Then

A=(1120 kg)

(0.305 m)[(1000 kg/m3)−(917 kg/m3)] = 44.2 m2.

E15-31 If there were no water vapor pressure above the barometer then the height of the water

would be y1=p/(ρg), where p=p0is the atmospheric pressure. If there is water vapor where there

should be a vacuum, then pis the diﬀerence, and we would have y2= (p0−pv)/(ρg). The relative

error is

(y1−y2)/y1= [p0/(ρg)−(p0−pv)/(ρg)] /[p0/(ρg)] ,

=pv/p0= (3169 Pa)/(1.01×105Pa) = 3.14 %.

E15-32 ρ= (1.01×105Pa)/(9.81 m/s2)(14 m) = 740 kg/m3.

E15-33 h= (90)(1.01×105Pa)/(8.60 m/s2)(1.36×104kg/m3) = 78 m.

E15-34 ∆U= 2(4.5×10−2N/m)4π(2.1×10−2m)2= 5.0×10−4J.

188

E15-35 The force required is just the surface tension times the circumference of the circular

patch. Then

F= (0./072 N/m)2π(0.12 m) = 5.43×10−2N.

E15-36 ∆U= 2(2.5×10−2N/m)4π(1.4×10−2m)2= 1.23×10−4J.

P15-1 (a) One can replace the two hemispheres with an open ﬂat end with two hemispheres with

a closed ﬂat end. Then the area of relevance is the area of the ﬂat end, or πR2. The net force from

the pressure diﬀerence is ∆pA = ∆pπR2; this much force must be applied to pull the hemispheres

apart.

(b) F=π(0.9)(1.01×105Pa)(0.305 m)2= 2.6×104N.

P15-2 The pressure required is 4×109Pa. This will happen at a depth

h=(4×109Pa)

(9.8 m/s2)(3100 kg/m3)= 1.3×105m.

P15-3 (a) The resultant force on the wall will be

F=Z Z P dx dy,

=Z(−ρgy)W dy,

=ρgD2W/2.

(b) The torque will is given by τ=F(D−y) (the distance is from the bottom) so if we generalize,

τ=Z Z P y dx dy,

=Z(−ρg(D−y)) yW dy,

=ρgD3W/6.

d=τ/F = (ρgD3W/6)/(ρgD2W/2) = D/3,

this distance being measured from the bottom.

P15-4 p=ρgy =ρg(3.6 m); the force on the bottom is F=pA =ρg(3.6 m)π(0.60 m)2= 1.296πρg.

The volume of liquid is

V= (1.8 m) π(0.60 m) + 4.6×10−4m2= 2.037 m3

The weight is W=ρg(2.037 m3). The ratio is 2.000.

P15-5 The pressure at bis ρc(3.2×104m)+ρmy. The pressure at ais ρc(3.8×104m+d)+ρm(y−d).

Set these quantities equal to each other:

ρc(3.8×104m + d) + ρm(y−d) = ρc(3.2×104m) + ρmy,

ρc(6×103m + d) = ρmd,

d=ρc(6×103m)/(ρm−ρc),

= (2900 kg/m3)(6×103m)/(400 kg/m3) = 4.35×104m.

189

P15-6 (a) The pressure (diﬀerence) at a depth yis ∆p=ρgy. Since ρ=m/V , then

∆ρ≈ −m

∆V

V=ρs

∆p

Then

ρ≈ρs+ ∆ρ=ρs+ρs2gy

(b) ∆ρ/ρs=ρsgy/B, so

∆ρ/ρ ≈(1000 kg/m3)(9.8 m/s2)(4200 m)/(2.2×109Pa) = 1.9 %.

P15-7 (a) Use Eq. 15-10, p= (p0/ρ0)ρ, then Eq. 15-13 will look like

(p0/ρ0)ρ= (p0/ρ0)ρ0e−h/a.

(b) The upward velocity of the rocket as a function of time is given by v=art. The height of

the rocket above the ground is given by y=1

2art2. Combining,

v=arr2y

=p2yar.

Put this into the expression for drag, along with the equation for density variation with altitude;

D=CAρv2=CAρ0e−y/a2yar.

Now take the derivative with respect to y,

dD/dy = (−1/a)CAρ0e−y/a(2yar) + CAρ0e−y/a(2ar).

This will vanish when y=a, regardless of the acceleration ar.

P15-8 (a) Consider a slice of cross section Aa depth hbeneath the surface. The net force on the

ﬂuid above the slice will be

Fnet =ma =ρhAg,

Since the weight of the ﬂuid above the slice is

W=mg =ρhAg,

then the upward force on the bottom of the ﬂuid at the slice must be

W+Fnet =ρhA(g+a),

so the pressure is p=F/A =ρh(g+a).

(b) Change ato −a.

P15-9 (a) Consider a portion of the liquid at the surface. The net force on this portion is ~

a=maˆ

i. The force of gravity on this portion is ~

W=−mgˆ

j. There must then be a buoyant

force on the portion with direction ~

B=~

F−~

W=m(aˆ

i+gˆ

j). The buoyant force makes an angle

θ= arctan(a/g) with the vertical. The buoyant force must be perpendicular to the surface of the

ﬂuid; there are no pressure-related forces which are parallel to the surface. Consequently, the surface

must make an angle θ= arctan(a/g) with the horizontal.

(b) It will still vary as ρgh; the derivation on page 334 is still valid for vertical displacements.

190

P15-10 dp/dr =−ρg, but now g= 4πGρr/3. Then

dp =−4

3πGρ2Zr

r dr,

p=2

3πGρ2R2−r2.

P15-11 We can start with Eq. 15-11, except that we’ll write our distance in terms of rinstead

if y. Into this we can substitute our expression for g,

g=g0

r2.

Substituting, then integrating,

p=−gρ0

dr,

p=−g0ρ0R2

r2,

p=−Zr

g0ρ0R2

r2,

ln p

=g0ρ0R2

p01

r−1

R

If k=g0ρ0R2/p0, then

p=p0ek(1/r−1/R).

P15-12 (a) The net force on a small volume of the ﬂuid is dF =rω2dm directed toward the

center. For radial displacements, then, dF/dr =−rω2dm/dr or dp/dr =−rω2ρ.

(b) Integrating outward,

p=pc+Zr

ρω2r dr =pc+1

2ρr2ω2.

(d) It will still vary as ρgh; the derivation on page 334 is still valid for vertical displacements.

p=p0+1

2ρr2ω2−ρgy,

where p0is the pressure on the surface, yis measured from the bottom of the paraboloid, and ris

measured from the center. The surface is deﬁned by p=p0, so

2ρr2ω2−ρgy = 0,

or y=r2ω2/2g.

P15-13 The total mass of the shell is m=ρwπdo3/3, or it wouldn’t barely ﬂoat. The mass of iron

in the shell is m=ρiπ(do3−di3)/3, so

di3=ρi−ρw

ρi

do3,

di=3

s(7870 kg/m3)−(1000 kg/m3)

(7870 kg/m3)(0.587 m) = 0.561 m.

191

P15-14 The wood will displace a volume of water equal to (3.67 kg)/(594 kg/m3)(0.883) = 5.45×

10−3m3in either case. That corresponds to a mass of (1000 kg/m3)(5.45×10−3m3)=5.45 kg that

can be supported.

(a) The mass of lead is 5.45 kg −3.67 kg = 1.78 kg.

(b) When the lead is submerged beneath the water it displaces water, which aﬀects the “apparent”

mass of the lead. The true weight of the lead is mg, the buoyant force is (ρw/ρl)mg, so the apparent

weight is (1 −ρw/ρl)mg. This means the apparent mass of the submerged lead is (1 −ρw/ρl)m.

This apparent mass is 1.78 kg, so the true mass is

m=(11400 kg/m3)

(11400 kg/m3)−(1000 kg)(1.78 kg) = 1.95 kg.

P15-15 We initially have

4=ρo

ρmercury

When water is poured over the object the simple relation no longer works.

Once the water is over the object there are two buoyant forces: one from mercury, F1, and one

from the water, F2. Following a derivation which is similar to Sample Problem 15-3, we have

F1=ρ1V1gand F2=ρ2V2g

where ρ1is the density of mercury, V1the volume of the object which is in the mercury, ρ2is the

density of water, and V2is the volume of the object which is in the water. We also have

F1+F2=ρoVogand V1+V2=Vo

as expressions for the net force on the object (zero) and the total volume of the object. Combining

these four expressions,

ρ1V1+ρ2V2=ρoVo,

ρ1V1+ρ2(Vo−V1) = ρoVo,

(ρ1−ρ2)V1= (ρo−ρ2)Vo,

=ρo−ρ2

ρ1−ρ2

The left hand side is the fraction that is submerged in the mercury, so we just need to substitute

our result for the density of the material from the beginning to solve the problem. The fraction

submerged after adding water is then

=ρo−ρ2

ρ1−ρ2

=ρ1/4−ρ2

ρ1−ρ2

=(13600 kg/m3)/4−(998 kg/m3)

(13600 kg/m3)−(998 kg/m3)= 0.191.

P15-16 (a) The car ﬂoats if it displaces a mass of water equal to the mass of the car. Then

V= (1820 kg)/(1000 kg/m3) = 1.82 m3.

(b) The car has a total volume of 4.87 m3+0.750 m3+0.810 m3= 6.43 m3. It will sink if the total

mass inside the car (car + water) is then (6.43 m3)(1000 kg/m3) = 6430 kg. So the mass of the water

in the car is 6430 kg−1820 kg = 4610 kg when it sinks. That’s a volume of (4610 kg)/(1000 kg/m3) =

4.16 m3.

192

P15-17 When the beaker is half ﬁlled with water it has a total mass exactly equal to the maximum

amount of water it can displace. The total mass of the beaker is the mass of the beaker plus the

mass of the water inside the beaker. Then

ρw(mg/ρg+Vb) = mg+ρwVb/2,

where mg/ρgis the volume of the glass which makes up the beaker. Rearrange,

ρg=mg

mg/ρw−Vb/2=(0.390 kg)

(0.390 kg)/(1000 kg/m3)−(5.00×10−4m3)/2= 2790 kg/m3.

P15-18 (a) If each atom is a cube then the cube has a side of length

l=3

p(6.64×10−27 kg)/(145 kg/m3) = 3.58×10−10m.

Then the atomic surface density is l−2= (3.58×10−10m)−2= 7.8×1018/m2.

(b) The bond surface density is twice the atomic surface density. Show this by drawing a square

array of atoms and then joining each adjacent pair with a bond. You will need twice as many bonds

as there are atoms. Then the energy per bond is

(3.5×10−4N/m)

2(7.8×1018/m2)(1.6×10−19 J/eV) = 1.4×10−4eV.

P15-19 Pretend the bubble consists of two hemispheres. The force from surface tension holding

the hemispheres together is F= 2γL = 4πrγ. The “extra” factor of two occurs because each

hemisphere has a circumference which “touches” the boundary that is held together by the surface

tension of the liquid. The pressure diﬀerence between the inside and outside is ∆p=F/A, where A

is the area of the ﬂat side of one of the hemispheres, so ∆p= (4πrγ)/(πr2) = 4γ/r.

P15-20 Use the results of Problem 15-19. To get a numerical answer you need to know the surface

tension; try γ= 2.5×10−2N/m. The initial pressure inside the bubble is pi=p0+ 4γ/ri. The ﬁnal

pressure inside the bell jar is p=pf−4γ/rf. The initial and ﬁnal pressure inside the bubble are

related by piri3=pfrf3. Now for numbers:

pi= (1.00×105Pa) + 4(2.5×10−2N/m)/(1.0×10−3m) = 1.001×105Pa.

pf= (1.0×10−3m/1.0×10−2m)3(1.001×105Pa) = 1.001×102Pa.

p= (1.001×102Pa) −4(2.5×10−2N/m)/(1.0×10−2m) = 90.1Pa.

P15-21 The force on the liquid in the space between the rod and the cylinder is F=γL =

2πγ(R+r). This force can support a mass of water m=F/g. This mass has a volume V=m/ρ.

The cross sectional area is π(R2−r2), so the height hto which the water rises is

h=2πγ(R+r)

ρgπ(R2−r2)=2γ

ρg(R−r),

=2(72.8×10−3N/m)

(1000 kg/m3)(9.81 m/s2)(4.0×10−3m) = 3.71×10−3m.

193

P15-22 (a) Refer to Problem 15-19. The initial pressure diﬀerence is

4(2.6×10−2N/m)/(3.20×10−2m) = 3.25Pa.

(b) The ﬁnal pressure diﬀerence is

4(2.6×10−2N/m)/(5.80×10−2m) = 1.79Pa.

(1.01×105Pa)4π

3[(5.80×10−2m)3−(3.20×10−2m)3] = 68.7 J.

(d) The work done in stretching the bubble surface is γ∆A, or

(2.60×10−2N/m)4π[(5.80×10−2m)2−(3.20×10−2m)2] = 7.65×10−4J.

194

E16-1 R=Av =πd2v/4 and V=Rt, so

t=4(1600 m3)

π(0.345 m)2(2.62 m) = 6530 s

E16-2 A1v1=A2v2,A1=πd2

1/4 for the hose, and A2=Nπd2

2for the sprinkler, where N= 24.

Then

v2=(0.75 in)2

(24)(0.050 in)2(3.5 ft/s) = 33 ft/s.

E16-3 We’ll assume that each river has a rectangular cross section, despite what the picture

implies. The cross section area of the two streams is then

A1= (8.2 m)(3.4 m) = 28 m2and A2= (6.8 m)(3.2 m) = 22 m2.

The volume ﬂow rate in the ﬁrst stream is

R1=A1v1= (28 m2)(2.3 m/s) = 64 m3/s,

while the volume ﬂow rate in the second stream is

R2=A2v2= (22 m2)(2.6 m/s) = 57 m3/s.

The amount of ﬂuid in the stream/river system is conserved, so

R3=R1+R2= (64 m3/s) + (57 m3/s) = 121 m3/s.

where R3is the volume ﬂow rate in the river. Then

D3=R3/(v3W3) = (121 m3/s)/[(10.7 m)(2.9 m/s)] = 3.9 m.

E16-4 The speed of the water is originally zero so both the kinetic and potential energy is zero.

When it leaves the pipe at the top it has a kinetic energy of 1

2(5.30 m/s)2= 14.0 J/kg and a

potential energy of (9.81 m/s2)(2.90 m) = 28.4 J/kg. The water is ﬂowing out at a volume rate of

R= (5.30 m/s)π(9.70×10−3m)2= 1.57×10−3m3/s. The mass rate is ρR = (1000 kg/m3)(1.57×

10−3m3/s) = 1.57 kg/s.

The power supplied by the pump is (42.8 J/kg)(1.57 kg/s) = 67.2W.

E16-5 There are 8500 km2which collects an average of (0.75)(0.48 m/y), where the 0.75 reﬂects

the fact that 1/4 of the water evaporates, so

R=8500(103m)2(0.75)(0.48 m/y) 1 y

365 ×24 ×60 ×60 s= 97 m3/s.

Then the speed of the water in the river is

v=R/A = (97 m3/s)/[(21 m)(4.3 m)] = 1.1 m/s.

E16-7 (a) ∆p=ρg(y1−y2) + ρ(v2

1−v2

2)/2. Then

∆p= (62.4 lb/ft3)(572 ft)+ [(62.4 lb/ft3)/(32 ft/s2)][(1.33 ft/s)2−(31.0 ft/s)2]/2 = 3.48×104lb/ft2.

(b) A2v2=A1v1, so

A2= (7.60 ft2)(1.33 ft/s)/(31.0 ft/s) = 0.326 ft2.

195

E16-8 (a) A2v2=A1v1, so

v2= (2.76 m/s)[(0.255 m)2−(0.0480 m)2]/(0.255 m)2= 2.66 m/s.

(b) ∆p=ρ(v2

1−v2

2)/2,

∆p= (1000 kg/m3)[(2.66 m/s)2−(2.76 m/s)2]/2 = −271 Pa

E16-9 (b) We will do part (b) ﬁrst.

R= (100 m2)(1.6 m/y) 1 y

365 ×24 ×60 ×60 s= 5.1×10−6m3/s.

(b) The speed of the ﬂow Rthrough a hole of cross sectional area awill be v=R/a.p=p0+ρgh,

where h= 2.0 m is the depth of the hole. Bernoulli’s equation can be applied to ﬁnd the speed of

the water as it travels a horizontal stream line out the hole,

p0+1

2ρv2=p,

where we drop any terms which are either zero or the same on both sides. Then

v=p2(p−p0)/ρ =p2gh =p2(9.81 m/s2)(2.0 m) = 6.3 m/s.

Finally, a= (5.1×10−6m3/s)/(6.3 m/s) = 8.1×10−7m2, or about 0.81 mm2.

E16-10 (a) v2= (A1/A2)v1= (4.20 cm2)(5.18 m/s)/(7.60 cm2) = 2.86 m/s.

(b) Use Bernoulli’s equation:

p2+ρgy2+1

2ρv2

2=p1+ρgy1+1

2ρv2

Then

p2= (1.52×105Pa) + (1000 kg/m3)(9.81 m/s2)(9.66 m) + 1

2(5.18 m/s)2−1

2(2.86 m/s)2,

= 2.56×105Pa.

E16-11 (a) The wind speed is (110 km/h)(1000 m/km)/(3600 s/h) = 30.6 m/s. The pressure

diﬀerence is then

∆p=1

2(1.2 kg/m3)(30.6 m/s)2= 562 Pa.

(b) The lifting force would be F= (562 Pa)(93 m2) = 52000 N.

E16-12 The pressure diﬀerence is

∆p=1

2(1.23 kg/m3)(28.0 m/s)2= 482 N.

The net force is then F= (482 N)(4.26 m)(5.26 m) = 10800 N.

196

E16-13 The lower pipe has a radius r1= 2.52 cm, and a cross sectional area of A1=πr2

1. The

speed of the ﬂuid ﬂow at this point is v1. The higher pipe has a radius of r2= 6.14 cm, a cross

sectional area of A2=πr2

2, and a ﬂuid speed of v2. Then

A1v1=A2v2or r2

1v1=r2

2v2.

Set y1= 0 for the lower pipe. The problem speciﬁes that the pressures in the two pipes are the

same, so

p0+1

2ρv2

1+ρgy1=p0+1

2ρv2

2+ρgy2,

2v2

1=1

2v2

2+gy2,

We can combine the results of the equation of continuity with this and get

1=v2

2+ 2gy2,

1=v1r2

1/r2

22+ 2gy2,

11−r4

1/r4

2= 2gy2,

1= 2gy2/1−r4

1/r4

2.

Then

1= 2(9.81 m/s2)(11.5 m)/1−(0.0252 m)4/(0.0614 m)4= 232 m2/s2

The volume ﬂow rate in the bottom (and top) pipe is

R=πr2

1v1=π(0.0252 m)2(15.2 m/s) = 0.0303 m3/s.

E16-14 (a) As instructed,

p0+1

2ρv2

1+ρgy1=p0+1

2ρv2

3+ρgy3,

0 = 1

2v2

3+g(y3−y1),

But y3−y1=−h, so v3=√2gh.

(b) habove the hole. Just reverse your streamline!

E16-15 Sea level will be deﬁned as y= 0, and at that point the ﬂuid is assumed to be at rest.

Then

p0+1

2ρv2

1+ρgy1=p0+1

2ρv2

2+ρgy2,

0 = 1

2v2

2+gy2,

where y2=−200 m. Then

v2=p−2gy2=p−2(9.81 m/s2)(−200 m) = 63 m/s.

197

E16-16 Assume streamlined ﬂow, then

p1+1

2ρv2

1+ρgy1=p2+1

2ρv2

2+ρgy2,

(p1−p2)/ρ +g(y1−y2) = 1

2v2

Then upon rearranging

v2=p2 [(2.1)(1.01×105Pa)/(1000 kg/m3) + (9.81 m/s2)(53.0 m)] = 38.3 m/s.

E16-17 (a) Points 1 and 3 are both at atmospheric pressure, and both will move at the same

speed. But since they are at diﬀerent heights, Bernoulli’s equation will be violated.

(b) The ﬂow isn’t steady.

E16-18 The atmospheric pressure diﬀerence between the two sides will be ∆p=1

2ρav2.The height

diﬀerence in the U-tube is given by ∆p=ρwgh. Then

h=(1.20 kg/m3)(15.0 m/s)2

2(1000 kg/m3)(9.81 m/s2)= 1.38×10−2m.

E16-19 (a) There are three forces on the plug. The force from the pressure of the water, F1=

P1A, the force from the pressure of the air, F2=P2A, and the force of friction, F3. These three

forces must balance, so F3=F1−F2,or F3=P1A−P2A. But P1−P2is the pressure diﬀerence

between the surface and the water 6.15 m below the surface, so

F3= ∆P A =−ρgyA,

=−(998 kg/m3)(9.81 m/s2)(−6.15 m)π(0.0215 m)2,

= 87.4 N

(b) To ﬁnd the volume of water which ﬂows out in three hours we need to know the volume

ﬂow rate, and for that we need both the cross section area of the hole and the speed of the ﬂow.

The speed of the ﬂow can be found by an application of Bernoulli’s equation. We’ll consider the

horizontal motion only— a point just inside the hole, and a point just outside the hole. These points

are at the same level, so

p1+1

2ρv2

1+ρgy1=p2+1

2ρv2

2+ρgy2,

p1=p2+1

2ρv2

Combine this with the results of Pascal’s principle above, and

v2=p2(p1−p2)/ρ =p−2gy =p−2(9.81 m/s2)(−6.15 m) = 11.0 m/s.

The volume of water which ﬂows out in three hours is

V=Rt = (11.0 m/s)π(0.0215 m)2(3 ×3600 s) = 173 m3.

E16-20 Apply Eq. 16-12:

v1=p2(9.81 m/s2)(0.262 m)(810 kg/m3)/(1.03 kg/m3) = 63.6 m/s.

198

E16-21 We’ll assume that the central column of air down the pipe exerts minimal force on the

card when it is deﬂected to the sides. Then

p1+1

2ρv2

1+ρgy1=p2+1

2ρv2

2+ρgy2,

p1=p2+1

2ρv2

The resultant upward force on the card is the area of the card times the pressure diﬀerence, or

F= (p1−p2)A=1

2ρAv2.

E16-22 If the air blows uniformly over the surface of the plate then there can be no torque about

any axis through the center of mass of the plate. Since the weight also doesn’t introduce a torque,

then the hinge can’t exert a force on the plate, because any such force would produce an unbalanced

torque. Consequently mg = ∆pA. ∆p=ρv2/2, so

v=r2mg

ρA =s2(0.488 kg)(9.81 m/s2)

(1.21 kg/m3)(9.10×10−2m)2= 30.9 m/s.

E16-23 Consider a streamline which passes above the wing and a streamline which passes beneath

the wing. Far from the wing the two streamlines are close together, move with zero relative velocity,

and are eﬀectively at the same pressure. So we can pretend they are actually one streamline. Then,

since the altitude diﬀerence between the two points above and below the wing (on this new, single

streamline) is so small we can write

∆p=1

2ρ(vt2−vu2)

The lift force is then

L= ∆p A =1

2ρA(vt2−vu2)

E16-24 (a) From Exercise 16-23,

L=1

2(1.17 kg/m3)(2)(12.5 m2)(49.8 m/s)2−(38.2 m/s)2= 1.49×104N.

The mass of the plane must be m=L/g = (1.49×104N)/(9.81 m/s2) = 1520 kg.

(b) The lift is directed straight up.

(d) The lift is directed 15◦oﬀ the vertical toward the front of the plane.

E16-25 The larger pipe has a radius r1= 12.7 cm, and a cross sectional area of A1=πr2

1. The

speed of the ﬂuid ﬂow at this point is v1. The smaller pipe has a radius of r2= 5.65 cm, a cross

sectional area of A2=πr2

2, and a ﬂuid speed of v2. Then

A1v1=A2v2or r2

1v1=r2

2v2.

Now Bernoulli’s equation. The two pipes are at the same level, so y1=y2. Then

p1+1

2ρv2

1+ρgy1=p2+1

2ρv2

2+ρgy2,

p1+1

2ρv2

1=p2+1

2ρv2

199

Combining this with the results from the equation of continuity,

p1+1

2ρv2

1=p2+1

2ρv2

1=v2

2+2

ρ(p2−p1),

1=v1

22

ρ(p2−p1),

11−r4

2=2

ρ(p2−p1),

1=2 (p2−p1)

ρ(1 −r4

1/r4

2).

It may look a mess, but we can solve it to ﬁnd v1,

v1=s2(32.6×103Pa −57.1×103Pa)

(998 kg/m3)(1 −(0.127 m)4/(0.0565 m)4)= 1.41 m/s.

The volume ﬂow rate is then

R=Av =π(0.127 m)2(1.41 m/s) = 7.14×10−3m3/s.

That’s about 71 liters/second.

E16-26 The lines are parallel and equally spaced, so the velocity is everywhere the same. We can

transform to a reference frame where the liquid appears to be at rest, so the Pascal’s equation would

apply, and p+ρgy would be a constant. Hence,

p0=p+ρgy +1

2ρv2

is the same for all streamlines.

E16-27 (a) The “particles” of ﬂuid in a whirlpool would obey conservation of angular momentum,

meaning a particle oﬀ mass mwould have l=mvr be constant, so the speed of the ﬂuid as a function

of radial distance from the center would be given by k=vr, where kis some constant representing

the angular momentum per mass. Then v=k/r.

(b) Since v=k/r and v= 2πr/T , the period would be T∝r2.

E16-28 Rc= 2000. Then

v < Rcη

ρD =(2000)(4.0×10−3N·s/m2)

(1060 kg/m3)(3.8×10−3m) = 2.0 m/s.

E16-29 (a) The volume ﬂux is given; from that we can ﬁnd the average speed of the ﬂuid in the

pipe.

v=5.35 ×10−2L/min

π(1.88 cm)2= 4.81×10−3L/cm2·min.

But 1 L is the same as 1000 cm3and 1 min is equal to 60 seconds, so v= 8.03×10−4m/s.

200

Reynold’s number from Eq. 16-22 is then

R=ρDv

η=(13600 kg/m3)(0.0376 m)(8.03×10−4m/s)

(1.55 ×10−3N·s/m2)= 265.

This is well below the critical value of 2000.

(b) Poiseuille’s Law, Eq. 16-20, can be used to ﬁnd the pressure diﬀerence between the ends of

the pipe. But ﬁrst, note that the mass ﬂux dm/dt is equal to the volume rate times the density

when the density is constant. Then ρ dV /dt =dm/dt, and Poiseuille’s Law can be written as

δp =8ηL

πR4

dt =8(1.55 ×10−3N·s/m2)(1.26 m)

π(1.88×10−2m)4(8.92×10−7m3/s) = 0.0355 Pa.

P16-1 The volume of water which needs to ﬂow out of the bay is

V= (6100 m)(5200 m)(3 m) = 9.5×107m3

during a 6.25 hour (22500 s) period. The average speed through the channel must be

v=(9.5×107m3)

(22500 s)(190 m)(6.5 m) = 3.4 m/s.

P16-2 (a) The speed of the ﬂuid through either hole is v=√2gh. The mass ﬂux through a hole

is Q=ρAv, so ρ1A1=ρ2A2. Then ρ1/ρ2=A2/A1= 2.

(b) R=Av, so R1/R2=A1/A2= 1/2.

h2/h1= (A1/A2)2= (1/2)2= 1/4.

So h2=h1/4.

P16-3 (a) Apply Torricelli’s law (Exercise 16-14): v=√2gh. The speed vis a horizontal velocity,

and serves as the initial horizontal velocity of the ﬂuid “projectile” after it leaves the tank. There

is no initial vertical velocity.

This ﬂuid “projectile” falls through a vertical distance H−hbefore splashing against the ground.

The equation governing the time tfor it to fall is

−(H−h) = −1

2gt2,

Solve this for the time, and t=p2(H−h)/g. The equation which governs the horizontal distance

traveled during the fall is x=vxt, but vx=vand we just found t, so

x=vxt=p2gh p2(H−h)/g = 2ph(H−h).

(b) How many values of hwill lead to a distance of x? We need to invert the expression, and

we’ll start by squaring both sides

x2= 4h(H−h) = 4hH −4h2,

and then solving the resulting quadratic expression for h,

h=4H±√16H2−16x2

8=1

2H±pH2−x2.

201

For values of xbetween 0 and Hthere are two real solutions, if x=Hthere is one real solution,

and if x > H there are no real solutions.

If h1is a solution, then we can write h1= (H+ ∆)/2, where ∆ = 2h1−Hcould be positive or

negative. Then h2= (H+ ∆)/2 is also a solution, and

h2= (H+ 2h1−2H)/2 = h1−H

is also a solution.

previous section.

P16-4 (a) Apply Torricelli’s law (Exercise 16-14): v=p2g(d+h2), assuming that the liquid

remains in contact with the walls of the tube until it exits at the bottom.

(b) The speed of the ﬂuid in the tube is everywhere the same. Then the pressure diﬀerence at

various points are only functions of height. The ﬂuid exits at C, and assuming that it remains in

contact with the walls of the tube the pressure diﬀerence is given by ∆p=ρ(h1+d+h2), so the

pressure at Bis

p=p0−ρ(h1+d+h2).

principle applies. Then the maximum height is given by 0 = p0+ρgh1, or

h1= (1.01×105Pa)/[(9.81 m/s2)(1000 kg/m3)] = 10.3 m.

P16-5 (a) The momentum per kilogram of the ﬂuid in the smaller pipe is v1. The momentum per

kilogram of the ﬂuid in the larger pipe is v2. The change in momentum per kilogram is v2−v1.

There are ρa2v2kilograms per second ﬂowing past any point, so the change in momentum per

second is ρa2v2(v2−v1). The change in momentum per second is related to the net force according

to F= ∆p/∆t, so F=ρa2v2(v2−v1). But F≈∆p/a2, so p1−p2≈ρv2(v2−v1).

(b) Applying the streamline equation,

p1+ρgy1+1

2ρv2

1=p2+ρgy2+1

2ρv2

2ρ(v2

1−v2

2) = p2−p1

widened pipe. Then we want

∆p=1

2ρ(v2

1−v2

2)−ρv2(v1−v2),

2ρ(v2

1−v2

2)−ρv2v1+ρv2

2ρ(v2

1−2v1v2+v2

2) = 1

2ρ(v1−v2)2.

P16-6 The juice leaves the jug with a speed v=√2gy, where yis the height of the juice in the

jug. If Ais the cross sectional area of the base of the jug and athe cross sectional area of the hole,

then the juice ﬂows out the hole with a rate dV /dt =va =a√2gy, which means the level of jug

varies as dy/dt =−(a/A)√2gy. Rearrange and integrate,

dy/√y=Zt

0p2g(a/A)dt,

202

2(√h−√y) = p2gat/A.

as2h

g!(√h−√y) = t

When y= 14h/15 we have t= 12.0 s. Then the part in the parenthesis on the left is 3.539×102s.

The time to empty completely is then 354 seconds, or 5 minutes and 54 seconds. But we want the

remaining time, which is 12 seconds less than this.

P16-7 The greatest possible value for vwill be the value over the wing which results in an air

pressure of zero. If the air at the leading edge is stagnant (not moving) and has a pressure of p0,

then Bernoulli’s equation gives

p0=1

2ρv2,

or v=p2p0/ρ =p2(1.01×105Pa)/(1.2 kg/m3) = 410 m/s. This value is only slightly larger than

the speed of sound; they are related because sound waves involve the movement of air particles which

“shove” other air particles out of the way.

P16-8 Bernoulli’s equation together with continuity gives

p1+1

2ρv2

1=p2+1

2ρv2

p1−p2=1

2ρv2

2−v2

1,

2ρA2

1−v2

1,

=v2

2A2

ρA2

1−A2

2.

But p1−p2= (ρ0−ρ)gh. Note that we are not assuming ρis negligible compared to ρ0. Combining,

v1=A2s2(ρ0−ρ)gh

ρ(A2

1−A2

2).

P16-9 (a) Bernoulli’s equation together with continuity gives

p1+1

2ρv2

1=p2+1

2ρv2

p1=1

2ρv2

2−v2

1,

2ρA2

1−v2

1,

=v2

1ρ(4.75)2−1/2.

Then

v1=s2(2.12)(1.01×105Pa)

(1000 kg/m3)(21.6) = 4.45 m/s,

and then v2= (4.75)(4.45 m/s) = 21.2 m/s.

(b) R=π(2.60×10−2m)2(4.45 m/s) = 9.45×10−3m3/s.

203

P16-10 (a) For Fig. 16-13 the velocity is constant, or ~

v=vˆ

i.d~

s=ˆ

idx +ˆ

jdy. Then

v·d~

s=vIdx = 0,

because Hdx = 0.

(b) For Fig. 16-16 the velocity is ~

v= (k/r)ˆ

r.d~

s=ˆ

rdr +ˆ

θr dφ. Then

v·d~

s=vIdr = 0,

because Hdr = 0.

P16-11 (a) For an element of the ﬂuid of mass dm the net force as it moves around the circle is

dF = (v2/r)dm.dm/dV =ρand dV =A dr and dF/A =dp. Then dp/dr =ρv2/r.

(b) From Bernoulli’s equation p+ρv2/2 is a constant. Then

dr +ρv dv

dr = 0,

or v/r +dv/dr = 0, or d(vr) = 0. Consequently vr is a constant.

v= (k/r)ˆ

r.d~

s=ˆ

rdr +ˆ

θr dφ. Then

v·d~

s=vIdr = 0,

because Hdr = 0. This means the ﬂow is irrotational.

P16-12 F/A =ηv/D, so

F/A = (4.0×1019N·s/m2)(0.048 m/3.16×107s)/(1.9×105m) = 3.2×105Pa.

P16-13 A ﬂow will be irrotational if and only if H~

v·d~

s= 0 for all possible paths. It is fairly

easy to construct a rectangular path which is parallel to the ﬂow on the top and bottom sides, but

perpendicular on the left and right sides. Then only the top and bottom paths contribute to the

integral. ~

vis constant for either path (but not the same), so the magnitude vwill come out of the

integral sign. Since the lengths of the two paths are the same but vis diﬀerent the two terms don’t

cancel, so the ﬂow is not irrotational.

P16-14 (a) The area of a cylinder is A= 2πrL. The velocity gradient if dv/dr. Then the retarding

force on the cylinder is F=−η(2πrL)dv/dr.

(b) The force pushing a cylinder through is F0=A∆p=πr2∆p.

πr2∆p=−η(2πrL)dv

dr ,

∆pZR

r dr = 2ηL Zv

dv,

∆p1

2(R2−r2)=2ηLv.

Then

v=∆p

4ηL(R2−r2).

204

P16-15 The volume ﬂux (called Rfto distinguish it from the radius R) through an annular ring

of radius rand width δr is

δRf=δA v = 2πr δr v,

where vis a function of rgiven by Eq. 16-18. The mass ﬂux is the volume ﬂux times the density,

so the total mass ﬂux is

dt =ρZR

δRf

δr dr,

=ρZR

2πr ∆p

4ηL(R2−r2)dr,

=πρ∆p

2ηL ZR

(rR2−r3)dr,

=πρ∆p

2ηL (R4/2−R4/4),

=πρ∆pR4

8ηL .

P16-16 The pressure diﬀerence in the tube is ∆p= 4γ/r, where ris the (changing) radius of the

bubble. The mass ﬂux through the tube is

dt =4ρπR4γ

8ηLr ,

Ris the radius of the tube. dm =ρdV , and dV = 4πr2dr. Then

Zr2

r3dr =Zt

R4γ

8ηL dt,

1−r4

2=ρR4γ

2ηL t,

Then

t=2(1.80×10−5N·s/m2)(0.112 m)

(0.54×10−3m)4(2.50×10−2N/m) [(38.2×10−3m)4−(21.6×10−3m)4] = 3630 s.

205

E17-1 For a perfect spring |F|=k|x|.x= 0.157 m when 3.94 kg is suspended from it. There

would be two forces on the object— the force of gravity, W=mg, and the force of the spring, F.

These two force must balance, so mg =kx or

k=mg

x=(3.94 kg)(9.81 m/s2)

(0.157 m) = 0.246 N/m.

Now that we know k, the spring constant, we can ﬁnd the period of oscillations from Eq. 17-8,

T= 2πrm

k= 2πs(0.520 kg)

(0.246 N/m) = 0.289 s.

E17-2 (a) T= 0.484 s.

(b) f= 1/T = 1/(0.484 s) = 2.07 s−1.

(d) k=mω2= (0.512 kg)(13.0 rad/s)2= 86.5 N/m.

(e) vm=ωxm= (13.0 rad/s)(0.347 m) = 4.51 m/s.

(f) Fm=mam= (0.512 kg)(13.0 rad/s)2(0.347 m) = 30.0 N.

E17-3 am= (2πf)2xm. Then

f=p(9.81 m/s2)/(1.20×10−6m)/(2π) = 455 Hz.

E17-4 (a) ω= (2π)/(0.645 s) = 9.74 rad/s. k=mω2= (5.22 kg)(9.74 rad/s)2= 495 N/m.

(b) xm=vm/ω = (0.153 m/s)/(9.74 rad/s) = 1.57×10−2m.

E17-5 (a) The amplitude is half of the distance between the extremes of the motion, so A= (2.00

mm)/2 = 1.00 mm.

(b) The maximum blade speed is given by vm=ωxm. The blade oscillates with a frequency of

120 Hz, so ω= 2πf = 2π(120 s−1) = 754 rad/s,and then vm= (754 rad/s)(0.001 m) = 0.754 m/s.

E17-6 (a) k=mω2= (1460 kg/4)(2π2.95/s)2= 1.25×105N/m

(b) f=pk/m/2π=p(1.25×105N/m)/(1830 kg/4)/2π= 2.63/s.

E17-7 (a) x= (6.12 m) cos[(8.38 rad/s)(1.90 s) + 1.92 rad] = 3.27 m.

(b) v=−(6.12 m)(8.38/s) sin[(8.38 rad/s)(1.90 s) + 1.92 rad] = 43.4 m/s.

(d) f= (8.38 rad/s)/2π= 1.33/s.

(e) T= 1/f = 0.750 s.

E17-8 k= (50.0 lb)/(4.00 in) = 12.5 lb/in.

mg =(32 ft/s2)(12 in/ft)(12.5 lb/in)

[2π(2.00/s)]2= 30.4 lb.

E17-9 If the drive wheel rotates at 193 rev/min then

ω= (193 rev/min)(2πrad/rev)(1/60 s/min) = 20.2 rad/s,

then vm=ωxm= (20.2 rad/s)(0.3825 m) = 7.73 m/s.

206

E17-10 k= (0.325 kg)(9.81 m/s2)/(1.80×10−2m) = 177 N/m..

T= 2πrm

k= 2πs(2.14 kg)

(177 N/m) = 0.691 s.

E17-11 For the tides ω= 2π/(12.5 h). Half the maximum occurs when cos ωt = 1/2, or ωt =π/3.

Then t= (12.5 h)/6 = 2.08 h.

E17-12 The two will separate if the (maximum) acceleration exceeds g.

(a) Since ω= 2π/T = 2π/(1.18 s) = 5.32 rad/s the maximum amplitude is

xm= (9.81 m/s2)/(5.32 rad/s)2= 0.347 m.

(b) In this case ω=p(9.81 m/s2)/(0.0512 m) = 13.8 rad/s.Then f= (13.8 rad/s)/2π= 2.20/s.

E17-13 (a) ax/x =−ω2. Then

ω=p−(−123 m/s)/(0.112 m) = 33.1 rad/s,

so f= (33.1 rad/s)/2π= 5.27/s.

(b) m=k/ω2= (456 N/m)/(33.1 rad/s)2= 0.416 kg.

x2+ (v/ω)2=xm2cos2ωt +xm2sin2ωt =xm2.

Consequently,

xm=p(0.112 m)2+ (−13.6 m/s)2/(33.1 rad/s)2= 0.426 m.

E17-14 x1=xmcos ωt,x2=xmcos(ωt +φ). The crossing happens when x1=xm/2, or when

ωt =π/3 (and other values!). The same constraint happens for x2, except that it is moving in the

other direction. The closest value is ωt +φ= 2π/3, or φ=π/3.

E17-15 (a) The net force on the three cars is zero before the cable breaks. There are three forces

on the cars: the weight, W, a normal force, N, and the upward force from the cable, F. Then

F=Wsin θ= 3mg sin θ.

This force is from the elastic properties of the cable, so

k=F

x=3mg sin θ

The frequency of oscillation of the remaining two cars after the bottom car is released is

f=1

2πrk

2m=1

2πr3mg sin θ

2mx =1

2πr3gsin θ

2x.

Numerically, the frequency is

f=1

2πr3gsin θ

2x=1

2πs3(9.81 m/s2) sin(26◦)

2(0.142 m) = 1.07 Hz.

(b) Each car contributes equally to the stretching of the cable, so one car causes the cable to

stretch 14.2/3 = 4.73 cm. The amplitude is then 4.73 cm.

207

E17-16 Let the height of one side over the equilibrium position be x. The net restoring force

on the liquid is 2ρAxg, where Ais the cross sectional area of the tube and gis the acceleration of

free-fall. This corresponds to a spring constant of k= 2ρAg. The mass of the ﬂuid is m=ρAL.

The period of oscillation is

T= 2πrm

k=πs2L

E17-17 (a) There are two forces on the log. The weight, W=mg, and the buoyant force B.

We’ll assume the log is cylindrical. If xis the length of the log beneath the surface and Athe

cross sectional area of the log, then V=Ax is the volume of the displaced water. Furthermore,

mw=ρwVis the mass of the displaced water and B=mwgis then the buoyant force on the log.

Combining,

B=ρwAgx,

where ρwis the density of water. This certainly looks similar to an elastic spring force law, with

k=ρwAg. We would then expect the motion to be simple harmonic.

(b) The period of the oscillation would be

T= 2πrm

k= 2πrm

ρwAg ,

where mis the total mass of the log and lead. We are told the log is in equilibrium when x=L= 2.56

m. This would give us the weight of the log, since W=Bis the condition for the log to ﬂoat. Then

m=B

g=ρwAgL

g=ρAL.

From this we can write the period of the motion as

T= 2πsρAL

ρwAg = 2πpL/g = 2πs(2.56 m)

(9.81 m/s2)= 3.21 s.

E17-18 (a) k= 2(1.18 J)/(0.0984 m)2= 244 N/m.

(b) m= 2(1.18 J)/(1.22 m/s)2= 1.59 kg.

E17-19 (a) Equate the kinetic energy of the object just after it leaves the slingshot with the

potential energy of the stretched slingshot.

k=mv2

x2=(0.130 kg)(11.2×103m/s)2

(1.53 m)2= 6.97×106N/m.

(b) N= (6.97×106N/m)(1.53 m)/(220 N) = 4.85×104people.

E17-20 (a) E=kxm2/2, U=kx2/2 = k(xm/2)2/2 = E/4. K=E−U= 3E/4. The the energy

is 25% potential and 75% kinetic.

(b) If U=E/2 then kx2/2 = kxm2/4, or x=xm/√2.

208

E17-21 (a) am=ω2xmso

ω=ram

=s(7.93 ×103m/s2)

(1.86 ×10−3m) = 2.06 ×103rad/s

The period of the motion is then

T=2π

ω= 3.05×10−3s.

(b) The maximum speed of the particle is found by

vm=ωxm= (2.06 ×103rad/s)(1.86 ×10−3m) = 3.83 m/s.

because then x= 0 and

E=1

2mvm2=1

2(12.3 kg)(3.83 m/s)2= 90.2 J.

E17-22 (a) f=pk/m/2π=p(988 N/m)/(5.13 kg)/2π= 2.21/s.

(b) Ui= (988 N/m)(0.535 m)2/2 = 141 J.

(d) xm=p2E/k =p2(322 J + 141 J)/(988 N/m) = 0.968 m.

E17-23 (a) ω=p(538 N/m)/(1.26 kg) = 20.7 rad/s.

xm=p(0.263 m)2+ (3.72 m/s)2/(20.7 rad/s)2= 0.319 m.

(b) φ= arctan {−(−3.72 m/s)/[(20.7 rad/s)(0.263 m)]}= 34.3◦.

E17-24 Before doing anything else apply conservation of momentum. If v0is the speed of the

bullet just before hitting the block and v1is the speed of the bullet/block system just after the two

begin moving as one, then v1=mv0/(m+M), where mis the mass of the bullet and Mis the mass

of the block.

For this system ω=pk/(m+M).

(a) The total energy of the oscillation is 1

2(m+M)v2

1, so the amplitude is

xm=rm+M

kv1=rm+M

mv0

m+M=mv0s1

k(m+M).

The numerical value is

xm= (0.050 kg)(150 m/s)s1

(500 N/m)(0.050 kg + 4.00 kg) = 0.167 m.

(b) The fraction of the energy is

(m+M)v2

mv2

=m+M

mm

m+M2

m+M=(0.050 kg)

(0.050 kg + 4.00 kg) = 1.23×10−2.

E17-25 L= (9.82 m/s2)(1.00 s/2π)2= 0.249 m.

209

E17-26 T= (180 s)/(72.0). Then

g=2π(72.0)

(180 s) 2

(1.53 m) = 9.66 m/s.

E17-27 We are interested in the value of θmwhich will make the second term 2% of the ﬁrst

term. We want to solve

0.02 = 1

22sin2θm

which has solution

sin θm

2=√0.08

or θm= 33◦.

(b) How large is the third term at this angle?

2242sin4θm

2=32

221

22sin2θm

22

4(0.02)2

or 0.0009, which is very small.

E17-28 Since T∝p1/g we have

Tp=Teqge/gp= (1.00 s)s(9.78 m/s2)

(9.834 m/s2)= 0.997 s.

E17-29 Let the period of the clock in Paris be T1. In a day of length D1= 24 hours it will

undergo n=D/T1oscillations. In Cayenne the period is T2.noscillations should occur in 24 hours,

but since the clock runs slow, D2is 24 hours + 2.5 minutes elapse. So

T2=D2/n = (D2/D1)T1= [(1442.5 min)/(1440.0 min)]T1= 1.0017T1.

Since the ratio of the periods is (T2/T1) = p(g1/g2), the g2in Cayenne is

g2=g1(T1/T2)2= (9.81 m/s2)/(1.0017)2= 9.78 m/s2.

E17-30 (a) Take the diﬀerential of

g=2π(100)

T2

(10 m) = 4π2×105m

T2,

so δg = (−8π2×105m/T 3)δT . Note that Tis not the period here, it is the time for 100 oscillations!

The relative error is then δg

g=−2δT

If δg/g = 0.1% then δT/T = 0.05%.

(b) For g≈10 m/s2we have

T≈2π(100)p(10 m)/(10 m/s2) = 628 s.

Then δT ≈(0.0005)(987 s) ≈300 ms.

210

E17-31 T= 2πp(17.3 m)/(9.81 m/s2) = 8.34 s.

E17-32 The spring will extend until the force from the spring balances the weight, or when Mg =

kh. The frequency of this system is then

f=1

2πrk

M=1

2πrMg/h

M=1

2πrg

which is the frequency of a pendulum of length h. The mass of the bob is irrelevant.

E17-33 The frequency of oscillation is

f=1

2πrMgd

where dis the distance from the pivot about which the hoop oscillates and the center of mass of the

hoop.

The rotational inertia Iis about an axis through the pivot, so we apply the parallel axis theorem.

Then

I=Md2+Icm =Md2+Mr2.

But dis r, since the pivot point is on the rim of the hoop. So I= 2Md2, and the frequency is

f=1

2πrMgd

2Md2=1

2πrg

2d=1

2πs(9.81 m/s2)

2(0.653 m) = 0.436 Hz.

(b) Note the above expression looks like the simple pendulum equation if we replace 2dwith l.

Then the equivalent length of the simple pendulum is 2(0.653 m) = 1.31 m.

E17-34 Apply Eq. 17-21:

I=T2κ

4π2=(48.7 s/20.0)2(0.513 N ·m)

4π2= 7.70 ×10−2kg ·m2.

E17-35 κ= (0.192 N ·m)/(0.850 rad) = 0.226 N ·m. I=2

5(95.2 kg)(0.148 m)2= 0.834 kg ·m2.

Then

T= 2πpI/κ = 2πp(0.834 kg ·m2)/(0.226 N ·m) = 12.1 s.

E17-36 xis din Eq. 17-29. Since the hole is drilled oﬀ center we apply the principle axis theorem

to ﬁnd the rotational inertia:

I=1

12ML2+Mx2.

Then

12ML2+Mx2=T2Mgx

4π2,

12(1.00 m)2+x2=(2.50 s)2(9.81 m/s2)

4π2x,

(8.33×10−2m2)−(1.55 m)x+x2= 0.

This has solutions x= 1.49 m and x= 0.0557 m. Use the latter.

211

E17-37 For a stick of length Lwhich can pivot about the end, I=1

3ML2. The center of mass

of such a stick is located d=L/2 away from the end.

The frequency of oscillation of such a stick is

f=1

2πrMgd

f=1

2πsMg (L/2)

3ML2,

f=1

2πr3g

2L.

This means that fis proportional to p1/L, regardless of the mass or density of the stick. The ratio

of the frequency of two such sticks is then f2/f1=pL1/L2, which in our case gives

f2=f1pL2/L1=f1p(L1)/(2L1/3) = 1.22f1.

E17-38 The rotational inertia of the pipe section about the cylindrical axis is

Icm =M

2r2

1+r2

2=M

2(0.102 m)2+ (0.1084 m)2= (1.11×10−2m2)M

(a) The total rotational inertia about the pivot axis is

I= 2Icm +M(0.102 m)2+M(0.3188 m)2= (0.134 m2)M.

The period of oscillation is

T= 2πs(0.134 m2)M

M(9.81 m/s2)(0.2104 m) = 1.60 s

(b) The rotational inertia of the pipe section about a diameter is

Icm =M

4r2

1+r2

2=M

4(0.102 m)2+ (0.1084 m)2= (5.54×10−3m2)M

The total rotational inertia about the pivot axis is now

I=M(1.11×10−2m2) + M(0.102 m)2+M(5.54×10−3m2) + M(0.3188 m)2= (0.129 m2)M

The period of oscillation is

T= 2πs(0.129 m2)M

M(9.81 m/s2)(0.2104 m) = 1.57 s.

The percentage diﬀerence with part (a) is (0.03 s)/(1.60 s) = 1.9%.

E17-39

E17-40

E17-41 (a) Since eﬀectively x=y, the path is a diagonal line.

(b) The path will be an ellipse which is symmetric about the line x=y.

212

E17-42 (a)

(b) Take two time derivatives and multiply by m,

F=−mAω2ˆ

icos ωt + 9ˆ

jcos 3ωt.

F·d~

r, so

U=1

2mA2ω2cos2ωt + 9 cos23ωt.

(d) K=1

2mv2, so

K=1

2mA2ω2sin2ωt + 9 sin23ωt;

And then E=K+U= 5mA2ω2.

(e) Yes; the period is 2π/ω.

E17-43 The ωwhich describes the angular velocity in uniform circular motion is eﬀectively the

same ωwhich describes the angular frequency of the corresponding simple harmonic motion. Since

ω=pk/m, we can ﬁnd the eﬀective force constant kfrom knowledge of the Moon’s mass and the

period of revolution.

The moon orbits with a period of T, so

ω=2π

T=2π

(27.3×24 ×3600 s) = 2.66×10−6rad/s.

This can be used to ﬁnd the value of the eﬀective force constant kfrom

k=mω2= (7.36×1022kg)(2.66×10−6rad/s)2= 5.21×1011N/m.

E17-44 (a) We want to know when e−bt/2m= 1/3, or

t=2m

bln 3 = 2(1.52 kg)

(0.227 kg/s) ln 3 = 14.7 s

(b) The (angular) frequency is

ω0=s(8.13 N/m)

(1.52 kg) −(0.227 kg/s)

2(1.52 kg) 2

= 2.31 rad/s.

The number of oscillations is then

(14.7 s)(2.31 rad/s)/2π= 5.40

E17-45 The ﬁrst derivative of Eq. 17-39 is

dt =xm(−b/2m)e−bt/2mcos(ω0t+φ) + xme−bt/2m(−ω0) sin(ω0t+φ),

=−xme−bt/2m((b/2m) cos(ω0t+φ) + ω0sin(ω0t+φ))

The second derivative is quite a bit messier;

dx2=−xm(−b/2m)e−bt/2m((b/2m) cos(ω0t+φ) + ω0sin(ω0t+φ))

−xme−bt/2m(b/2m)(−ω0) sin(ω0t+φ)+(ω0)2cos(ω0t+φ),

=xme−bt/2m(ω0b/m) sin(ω0t+φ)+(b2/4m2−ω02) cos(ω0t+φ).

213

Substitute these three expressions into Eq. 17-38. There are, however, some fairly obvious simpli-

ﬁcations. Every one of the terms above has a factor of xm, and every term above has a factor of

e−bt/2m, so simultaneously with the substitution we will cancel out those factors. Then Eq. 17-38

becomes

m(ω0b/m) sin(ω0t+φ)+(b2/4m2−ω02) cos(ω0t+φ)

−b[(b/2m) cos(ω0t+φ) + ω0sin(ω0t+φ)] + kcos(ω0t+φ) = 0

Now we collect terms with cosine and terms with sine,

(ω0b−ω0b) sin(ω0t+φ) + mb2/4m2−ω02−b2/2m+kcos(ω0t+φ) = 0.

The coeﬃcient for the sine term is identically zero; furthermore, because the cosine term must then

vanish regardless of the value of t, the coeﬃcient for the sine term must also vanish. Then

mb2/4m2−mω02−b2/2m+k= 0,

ω02=k

m−b2

4m2.

If this condition is met, then Eq. 17-39 is indeed a solution of Eq. 17-38.

E17-46 (a) Four complete cycles requires a time t4= 8π/ω0. The amplitude decays to 3/4 the

original value in this time, so 0.75 = e−bt4/2m, or

ln(4/3) = 8πb

2mω0.

It is probably reasonable at this time to assume that b/2mis small compared to ωso that ω0≈ω.

We’ll do it the hard way anyway. Then

ω02=8π

ln(4/3)2b

2m2

m−b

2m2

=8π

ln(4/3)2b

2m2

m= (7630) b

2m2

Numerically, then,

b=s4(1.91 kg)(12.6 N/m)

(7630) = 0.112 kg/s.

(b)

E17-47 (a) Use Eqs. 17-43 and 17-44. At resonance ω00 =ω, so

G=√b2ω2=bω,

and then xm=Fm/bω.

(b) vm=ωxm=Fm/b.

214

E17-48 We need the ﬁrst two derivatives of

x=Fm

Gcos(ω00t−β)

The derivatives are easy enough to ﬁnd,

dt =Fm

G(−ω00) sin(ω00t−β),

and d2x

dt2=−Fm

G(ω00)2cos(ω00t−β),

We’ll substitute this into Eq. 17-42,

m−Fm

G(ω00)2cos(ω00t−β)

+bFm

G(−ω00) sin(ω00t−β)+kFm

Gcos(ω00t−β) = Fmcos ω00t.

Then we’ll cancel out as much as we can and collect the sine and cosine terms,

k−m(ω00)2cos(ω00t−β)−(bω00) sin(ω00t−β) = Gcos ω00t.

We can write the left hand side of this equation in the form

Acos α1cos α2−Asin α1sin α2,

if we let α2=ω00t−βand choose Aand α1correctly. The best choice is

Acos α1=k−m(ω00)2,

Asin α1=bω00,

and then taking advantage of the fact that sin2+ cos2= 1,

A2=k−m(ω00)22+ (bω00)2,

which looks like Eq. 17-44! But then we can apply the cosine angle addition formula, and

Acos(α1+ω00t−β) = Gcos ω00t.

This expression needs to be true for all time. This means that A=Gand α1=β.

E17-49 The derivatives are easy enough to ﬁnd,

dt =Fm

G(−ω000) sin(ω000t−β),

and d2x

dt2=−Fm

G(ω000)2cos(ω000t−β),

We’ll substitute this into Eq. 17-42,

m−Fm

G(ω000)2cos(ω000t−β)

+bFm

G(−ω000) sin(ω000t−β)+kFm

Gcos(ω000t−β) = Fmcos ω00t.

215

Then we’ll cancel out as much as we can and collect the sine and cosine terms,

k−m(ω000)2cos(ω000t−β)−(bω000) sin(ω000t−β) = Gcos ω00t.

We can write the left hand side of this equation in the form

Acos α1cos α2−Asin α1sin α2,

if we let α2=ω000t−βand choose Aand α1correctly. The best choice is

Acos α1=k−m(ω000)2,

Asin α1=bω000,

and then taking advantage of the fact that sin2+ cos2= 1,

A2=k−m(ω000)22+ (bω000)2,

which looks like Eq. 17-44! But then we can apply the cosine angle addition formula, and

Acos(α1+ω000t−β) = Gcos ω00t.

This expression needs to be true for all time. This means that A=Gand α1+ω000t−β=ω00tand

α1=βand ω000 =ω00.

E17-50 Actually, Eq. 17-39 is not a solution to Eq. 17-42 by itself, this is a wording mistake in

the exercise. Instead, Eq. 17-39 can be added to any solution of Eq. 17-42 and the result will still

be a solution.

Let xnbe any solution to Eq. 17-42 (such as Eq. 17-43.) Let xhbe given by Eq. 17-39. Then

x=xn+xh.

Take the ﬁrst two time derivatives of this expression.

dt =dxn

dt +dxh

dt ,

d2x

dt2=d2xn

dt2+d2xh

dt2

Substitute these three expressions into Eq. 17-42.

md2xn

dt2+d2xh

dt2+bdxn

dt +dxh

dt +k(xn+xh) = Fmcos ω00t.

Rearrange and regroup.

md2xn

dt2+bdxn

dt +kxn+md2xh

dt2+bdxh

dt +kxh=Fmcos ω00t.

Consider the second term on the left. The parenthetical expression is just Eq. 17-38, the damped

harmonic oscillator equation. It is given in the text (and proved in Ex. 17-45) the xhis a solution,

so this term is identically zero. What remains is Eq. 17-42; and we took as a given that xnwas a

solution.

(b) The “add-on” solution of xhrepresents the transient motion that will die away with time.

216

E17-51 The time between “bumps” is the solution to

vt =x,

t=(13 ft)

(10 mi/hr) 1 mi

5280 ft3600 s

1 hr = 0.886 s

The angular frequency is

ω=2π

T= 7.09 rad/s

This is the driving frequency, and the problem states that at this frequency the up-down bounce

oscillation is at a maximum. This occurs when the driving frequency is approximately equal to the

natural frequency of oscillation. The force constant for the car is k, and this is related to the natural

angular frequency by

k=mω2=W

gω2,

where W= (2200 + 4 ×180) lb= 2920 lb is the weight of the car and occupants. Then

k=(2920 lb)

(32 ft/s2)(7.09 rad/s)2= 4590 lb/ft

When the four people get out of the car there is less downward force on the car springs. The

important relationship is

∆F=k∆x.

In this case ∆F= 720 lb, the weight of the four people who got out of the car. ∆xis the distance

the car will rise when the people get out. So

∆x=∆F

k=(720 lb)

4590 lb/ft = 0.157 ft ≈2 in.

E17-52 The derivative is easy enough to ﬁnd,

dt =Fm

G(−ω00) sin(ω00t−β),

The velocity amplitude is

vm=Fm

Gω00,

=Fm

ω00 pm2(ω002−ω2)2+b2ω002,

=Fm

p(mω00 −k/ω00)2+b2.

Note that this is exactly a maximum when ω00 =ω.

E17-53 The reduced mass is

m= (1.13 kg)(3.24 kg)/(1.12 kg + 3.24 kg) = 0.840 kg.

The period of oscillation is

T= 2πp(0.840 kg)/(252 N/m) = 0.363 s

217

E17-54

E17-55 Start by multiplying the kinetic energy expression by (m1+m2)/(m1+m2).

K=(m1+m2)

2(m1+m2)m1v2

1+m2v2

2,

2(m1+m2)m2

1v2

1+m1m2(v2

1+v2

2) + m2

2v2

2,

and then add 2m1m2v1v2−2m1m2v1v2,

K=1

2(m1+m2)m2

1v2

1+ 2m1m2v1v2+m2

2v2

2+m1m2(v2

1+v2

2−2v1v2),

2(m1+m2)(m1v1+m2v2)2+m1m2(v1−v2)2.

But m1v2+m2v2= 0 by conservation of momentum, so

K=(m1m2)

2(m1+m2)(v1−v2)2,

2(v1−v2)2.

P17-1 The mass of one silver atom is (0.108 kg)/(6.02×1023) = 1.79×10−25kg.The eﬀective spring

constant is

k= (1.79×10−25kg)4π2(10.0×1012/s)2= 7.07×102N/m.

P17-2 (a) Rearrange Eq. 17-8 except replace mwith the total mass, or m+M. Then (M+m)/k =

T2/(4π2),or

M= (k/4π2)T2−m.

(b) When M= 0 we have

m= [(605.6 N/m)/(4π2)](0.90149 s)2= 12.467 kg.

P17-3 The maximum static friction is Ff≤µsN. Then

Ff=µsN=µsW=µsmg

is the maximum available force to accelerate the upper block. So the maximum acceleration is

am=Ff

m=µsg

The maximum possible amplitude of the oscillation is then given by

xm=am

ω2=µsg

k/(m+M),

where in the last part we substituted the total mass of the two blocks because both blocks are

oscillating. Now we put in numbers, and ﬁnd

xm=(0.42)(1.22 kg + 8.73 kg)(9.81 m/s2)

(344 N/m) = 0.119 m.

218

P17-4 (a) Equilibrium occurs when F= 0, or b/r3=a/r2. This happens when r=b/a.

(b) dF/dr = 2a/r3−3b/r4. At r=b/a this becomes

dF/dr = 2a4/b3−3a4/b3=−a4/b3,

which corresponds to a force constant of a4/b3.

P17-5 Each spring helps to restore the block. The net force on the block is then of magnitude

F1+F2=k1x+k2x= (k1+k2)x=kx. We can then write the frequency as

f=1

2πrk

m=1

2πrk1+k2

With a little algebra,

f=1

2πrk1+k2

=r1

4π2

m+1

4π2

=qf2

1+f2

P17-6 The tension in the two spring is the same, so k1x1=k2x2, where xiis the extension of the

ith spring. The total extension is x1+x2, so the eﬀective spring constant of the combination is

x=F

x1+x2

x1/F +x2/F =1

1/k1+ 1/k2

=k1k2

k1+k2

The period is then

T=1

2πrk

m=1

2πsk1k2

(k1+k2)m

With a little algebra,

f=1

2πsk1k2

(k1+k2)m,

2πs1

m/k1+m/k2

2πs1

1/ω2

1+ 1/ω2

2πsω2

1ω2

ω2

1+ω2

=f1f2

pf2

1+f2

219

P17-7 (a) When a spring is stretched the tension is the same everywhere in the spring. The

stretching, however, is distributed over the entire length of the spring, so that the relative amount

of stretch is proportional to the length of the spring under consideration. Half a spring, half the

extension. But k=−F/x, so half the extension means twice the spring constant.

In short, cutting the spring in half will create two stiﬀer springs with twice the spring constant,

so k= 7.20 N/cm for each spring.

(b) The two spring halves now support a mass M. We can view this as each spring is holding

one-half of the total mass, so in eﬀect

f=1

2πsk

M/2

or, solving for M,

M=2k

4π2f2=2(720 N/m)

4π2(2.87 s−1)2= 4.43 kg.

P17-8 Treat the spring a being composed of Nmassless springlets each with a point mass ms/N

at the end. The spring constant for each springlet will be kN . An expression for the conservation

of energy is then

2v2+ms

vn2+Nk

xn2=E.

Since the spring stretches proportionally along the length then we conclude that each springlet

compresses the same amount, and then xn=A/N sin ωt could describe the change in length of each

springlet. The energy conservation expression becomes

2v2+ms

vn2+k

2A2sin2ωt =E.

v=Aω cos ωt. The hard part to sort out is the vn, since the displacements for all springlets to one

side of the nth must be added to get the net displacement. Then

vn=nA

Nωcos ωt,

and the energy expression becomes

2+ms

2N3

n2!A2ω2cos2ωt +k

2A2sin2ωt =E.

Replace the sum with an integral, then

N3ZN

n2dn =1

and the energy expression becomes

2m+ms

3A2ω2cos2ωt +k

2A2sin2ωt =E.

This will only be constant if

ω2=m+ms

3/k,

or T= 2πp(m+ms/3)/k.

220

P17-9 (a) Apply conservation of energy. When x=xmv= 0, so

2kxm2=1

2m[v(0)]2+1

2k[x(0)]2,

xm2=m

k[v(0)]2+ [x(0)]2,

xm=p[v(0)/ω]2+ [x(0)]2.

(b) When t= 0 x(0) = xmcos φand v(0) = −ωxmsin φ, so

v(0)

ωx(0) =−sin φ

cos φ= tan φ.

P17-10

P17-11 Conservation of momentum for the bullet block collision gives mv = (m+M)vfor

vf=m

m+Mv.

This vfwill be equal to the maximum oscillation speed vm. The angular frequency for the oscillation

is given by

ω=rk

m+M.

Then the amplitude for the oscillation is

xm=vm

ω=vm

m+Mrm+M

k=mv

pk(m+M).

P17-12 (a) W=Fs, or mg =kx, so x=mg/k.

(b) F=ma, but F=W−Fs=mg −kx, and since ma =m d2x/dt2,

md2x

dt2+kx =mg.

The solution can be veriﬁed by direct substitution.

(d) dE/dt is

mv dv

dt +kxdx

dt −mg dx

dt = 0,

mdv

dt +kx =mg.

P17-13 The initial energy stored in the spring is kxm2/2. When the cylinder passes through the

equilibrium point it has a translational velocity vmand a rotational velocity ωr=vm/R, where R

is the radius of the cylinder. The total kinetic energy at the equilibrium point is

2mvm2+1

2Iωr2=1

2m+1

2mvm2.

Then the kinetic energy is 2/3 translational and 1/3 rotational. The total energy of the system is

E=1

2(294 N/m)(0.239 m)2= 8.40 J.

221

(a) Kt= (2/3)(8.40 J) = 5.60 J.

(b) Kr= (1/3)(8.40 J) = 2.80 J.

23m

2v2+1

2kx2=E,

which leads to a standard expression for the period with 3M/2 replacing m. Then T= 2πp3M/2k.

P17-14 (a) Integrate the potential energy expression over one complete period and then divide by

the time for one period:

2πZ2π/ω

2kx2dt =kω

4πZ2π/ω

xm2cos2ωt dt,

=kω

4πxm2π

ω,

4kxm2.

This is half the total energy; since the average total energy is E, then the average kinetic energy

must be the other half of the average total energy, or (1/4)kxm2.

(b) Integrate over half a cycle and divide by twice the amplitude.

2xmZxm

−xm

2kx2dx =1

2xm

3kxm3,

6kxm2.

This is one-third the total energy. The average kinetic energy must be two-thirds the total energy,

or (1/3)kxm2.

P17-15 The rotational inertia is

I=1

2MR2+Md2=M1

2(0.144 m)2+ (0.102 m)2= (2.08×10−2m2)M.

The period of oscillation is

T= 2πsI

Mgd = 2πs(2.08×10−2m2)M

M(9.81 m/s2)(0.102 m) = 0.906 s.

P17-16 (a) The rotational inertia of the pendulum about the pivot is

(0.488 kg) 1

2(0.103 m)2+ (0.103 m + 0.524 m)2+1

3(0.272 kg)(0.524 m)2= 0.219 kg ·m2.

(b) The center of mass location is

d=(0.524 m)(0.272 kg)/2 + (0.103 m + 0.524 m)(0.488 kg)

(0.272 kg) + (0.488 kg) = 0.496 m.

T= 2πp(0.291 kg ·m2)/(0.272 kg + 0.488 kg)(9.81 m/s2)(0.496 m) = 1.76 s.

222

P17-17 (a) The rotational inertia of a stick about an axis through a point which is a distance d

from the center of mass is given by the parallel axis theorem,

I=Icm +md2=1

12mL2+md2.

The period of oscillation is given by Eq. 17-28,

T= 2πsI

mgd = 2πsL2+ 12d2

12gd

(b) We want to ﬁnd the minimum period, so we need to take the derivative of Twith respect to

d. It’ll look weird, but

dd =π12d2−L2

p12gd3(L2+ 12d2).

This will vanish when 12d2=L2, or when d=L/√12.

P17-18 The energy stored in the spring is given by kx2/2, the kinetic energy of the rotating wheel

is 1

2(MR2)v

r2,

where vis the tangential velocity of the point of attachment of the spring to the wheel. If x=

xmsin ωt, then v=xmωcos ωt, and the energy will only be constant if

ω2=k

R2.

P17-19 The method of solution is identical to the approach for the simple pendulum on page 381

except replace the tension with the normal force of the bowl on the particle. The eﬀective pendulum

with have a length R.

P17-20 Let xbe the distance from the center of mass to the ﬁrst pivot point. Then the period is

given by

T= 2πsI+Mx2

Mgx .

Solve this for xby expressing the above equation as a quadratic:

MgT 2

4π2x=I+Mx2,

Mx2−MgT 2

4π2x+I= 0

There are two solutions. One corresponds to the ﬁrst location, the other the second location. Adding

the two solutions together will yield L; in this case the discriminant of the quadratic will drop out,

leaving

L=x1+x2=MgT 2

M4π2=gT 2

4π2.

Then g= 4π2L/T 2.

223

P17-21 In this problem

I= (2.50 kg) (0.210 m)2

2+ (0.760 m + 0.210 m)2= 2.41 kg/·m2.

The center of mass is at the center of the disk.

(a) T= 2πp(2.41 kg/·m2)/(2.50 kg)(9.81 m/s2)(0.760 m + 0.210 m) = 2.00 s.

(b) Replace Mgd with Mgd +κand 2.00 s with 1.50 s. Then

κ=4π2(2.41 kg/·m2)

(1.50 s)2−(2.50 kg)(9.81 m/s2)(0.760 m + 0.210 m) = 18.5 N ·m/rad.

P17-22 The net force on the bob is toward the center of the circle, and has magnitude Fnet =

mv2/R. This net force comes from the horizontal component of the tension. There is also a vertical

component of the tension of magnitude mg. The tension then has magnitude

T=p(mg)2+ (mv2/R)2=mpg2+v4/R2.

It is this tension which is important in ﬁnding the restoring force in Eq. 17-22; in eﬀect we want to

replace gwith pg2+v4/R2in Eq. 17-24. The frequency will then be

f=1

2πsL

pg2+v4/R2.

P17-23 (a) Consider an object of mass mat a point Pon the axis of the ring. It experiences a

gravitational force of attraction to all points on the ring; by symmetry, however, the net force is not

directed toward the circumference of the ring, but instead along the axis of the ring. There is then

a factor of cos θwhich will be thrown in to the mix.

The distance from Pto any point on the ring is r=√R2+z2, and θis the angle between the

axis on the line which connects Pand any point on the circumference. Consequently,

cos θ=z/r,

and then the net force on the star of mass mat Pis

F=GMm

r2cos θ=GMmz

r3=GMmz

(R2+z2)3/2.

(b) If zRwe can apply the binomial expansion to the denominator, and

(R2+z2)−3/2=R−31 + z

R2−3/2

≈R−31−3

2z

R2.

Keeping terms only linear in zwe have

F=GMm

R3z,

which corresponds to a spring constant k=GM m/R3. The frequency of oscillation is then

f=pk/m/(2π) = pGM/R3/(2π).

f=q(7×10−11N·m2/kg2)(2×1043kg)/(6×1019m)3/(2π) = 1×10−14 Hz.

224

P17-24 (a) The acceleration of the center of mass (point C) is a=F/M. The torque about an

axis through the center of mass is tau =F R/2, since Ois R/2 away from the center of mass. The

angular acceleration of the disk is then

α=τ/I = (F R/2)/(MR2/2) = F/(MR).

Note that the angular acceleration will tend to rotate the disk anti-clockwise. The tangential com-

ponent to the angular acceleration at Pis aT=−αR =−F/M; this is exactly the opposite of the

linear acceleration, so Pwill not (initially) accelerate.

(b) There is no net force at P.

P17-25 The value for kis closest to

k≈(2000 kg/4)(9.81 m/s2)/(0.10 m) = 4.9×104N/m.

One complete oscillation requires a time t1= 2π/ω0. The amplitude decays to 1/2 the original

value in this time, so 0.5 = e−bt1/2m, or

ln(2) = 2πb

2mω0.

It is not reasonable at this time to assume that b/2mis small compared to ωso that ω0≈ω. Then

ω02=2π

ln(2)2b

2m2

m−b

2m2

=2π

ln(2)2b

2m2

m= (81.2) b

2m2

Then the value for bis

b=s4(2000 kg/4)(4.9×104N/m)

(81.2) = 1100 kg/s.

P17-26 a=d2x/dt2=−Aω2cos ωt. Substituting into the non-linear equation,

−mAω2cos ωt +kA3cos3ωt =Fcos ωdt.

Now let ωd= 3ω. Then

kA3cos3ωt −mAω2cos ωt =Fcos 3ωt

Expand the right hand side as cos 3ωt = 4 cos3ωt −3 cos ωt, then

kA3cos3ωt −mAω2cos ωt =F(4 cos3ωt −3 cos ωt)

This will only work if 4F=kA3and 3F=mAω2. Dividing one condition by the other means

4mAω2=kA3, so A∝ωand then F∝ω3∝ωd3.

225

P17-27 (a) Divide the top and the bottom by m2. Then

m1m2

m1+m2

=m1

(m1/m2)+1,

and in the limit as m2→ ∞ the value of (m1/m2)→0, so

lim

m2→∞

m1m2

m1+m2

= lim

m2→∞

(m1/m2)+1 =m1.

(b) mis called the reduced mass because it is always less than either m1or m2. Think about it

in terms of

m=m1

(m1/m2)+1 =m2

(m2/m1)+1.

Since mass is always positive, the denominator is always greater than or equal to 1. Equality only

occurs if one of the masses is inﬁnite. Now ω=pk/m, and since mis always less than m1, so the

existence of a ﬁnite wall will cause ωto be larger, and the period to be smaller.

In eﬀect, the spring constant is doubled, which is what happens if a spring is cut in half.

226

E18-1 (a) f=v/λ = (243 m/s)/(0.0327 m) = 7.43×103Hz.

(b) T= 1/f = 1.35×10−4s.

E18-2 (a) f= (12)/(30 s) = 0.40 Hz.

(b) v= (15 m)/(5.0 s) = 3.0 m/s.

E18-3 (a) The time for a particular point to move from maximum displacement to zero dis-

placement is one-quarter of a period; the point must then go to maximum negative displacement,

zero displacement, and ﬁnally maximum positive displacement to complete a cycle. So the period is

4(178 ms) = 712 ms.

(b) The frequency is f= 1/T = 1/(712×10−3s) = 1.40 Hz.

E18-4 Use Eq. 18-9, except let f= 1/T :

y= (0.0213 m) sin 2πx

(0.114 m) −(385 Hz)t= (0.0213 m) sin [(55.1 rad/m)x−(2420 rad/s)t].

E18-5 The dimensions for tension are [F] = [M][L]/[T]2where M stands for mass, L for length, T

for time, and F stands for force. The dimensions for linear mass density are [M]/[L].The dimensions

for velocity are [L]/[T].

Inserting this into the expression v=Fa/µb,

[L]

[T] =[M][L]

[T]2a

/[M]

[L] b

[L]

[T] =[M]a[L]a

[T]2a

[L]b

[M]b,

[L]

[T] =[M]a−b[L]a+b

[T]2a

There are three equations here. One for time, −1 = −2a; one for length, 1 = a+b; and one for

mass, 0 = a−b. We need to satisfy all three equations. The ﬁrst is fairly quick; a= 1/2. Either of

the other equations can be used to show that b= 1/2.

E18-6 (a) ym= 2.30 mm.

(b) f= (588 rad/s)/(2πrad) = 93.6 Hz.

(d) λ= (2πrad)/(1822 rad/m) = 3.45 mm.

(e) uy=ymω= (2.30 mm)(588 rad/s) = 1.35 m/s.

E18-7 (a) ym= 0.060 m.

(b) λ= (2πrad)/(2.0πrad/m) = 1.0 m.

(d) v= (4.0πrad/s)/(2.0πrad/m) = 2.0 m/s.

(e) Since the second term is positive the wave is moving in the −xdirection.

(f) uy=ymω= (0.060 m)(4.0πrad/s) = 0.75 m/s.

E18-8 v=pF/µ =p(487 N)/[(0.0625 kg)/(2.15 m)] = 129 m/s.

227

E18-9 We’ll ﬁrst ﬁnd the linear mass density by rearranging Eq. 18-19,

µ=F

Since this is the same string, we expect that changing the tension will not signiﬁcantly change the

linear mass density. Then for the two diﬀerent instances,

=F2

We want to know the new tension, so

F2=F1

= (123 N)(180 m/s)2

(172 m/s)2= 135 N

E18-10 First v= (317 rad/s)/(23.8 rad/m) = 13.32 m/s. Then

µ=F/v2= (16.3 N)/(13.32 m/s)2= 0.0919 kg/m.

E18-11 (a) ym= 0.05 m.

(b) λ= (0.55 m) −(0.15 m) = 0.40 m.

(d) T= 1/f =λ/v = (0.40 m)/(12 m/s) = 3.33×10−2s.

(e) uy=ymω= 2πym/T = 2π(0.05 m)/(3.33×10−2s) = 9.4 m/s.

(f) k= (2πrad)/(0.40 m) = 5.0πrad/m; ω=kv = (5.0πrad/m)(12 m/s) = 60πrad/s. The

phase angle can be found from

(0.04 m) = (0.05 m) sin(φ),

or φ= 0.93 rad. Then

y= (0.05 m) sin[(5.0πrad/m)x+ (60πrad/s)t+ (0.93 rad)].

E18-12 (a) The tensions in the two strings are equal, so F= (0.511 kg)(9.81 m/s2)/2=2.506 N.

The wave speed in string 1 is

v=pF/µ =p(2.506 N)/(3.31×10−3kg/m) = 27.5 m/s,

while the wave speed in string 2 is

v=pF/µ =p(2.506 N)/(4.87×10−3kg/m) = 22.7 m/s.

(b) We have pF1/µ1=pF2/µ2, or F1/µ1=F2/µ2. But Fi=Mig, so M1/µ1=M2/µ2. Using

M=M1+M2,

µ1

=M−M1

µ2

µ1

+M1

µ2

M1=M

µ2 1

µ1

µ2,

=(0.511 kg)

(4.87×10−3kg/m)  1

(3.31×10−3kg/m) +1

(4.87×10−3kg/m) ,

= 0.207 kg

and M2= (0.511 kg) −(0.207 kg) = 0.304 kg.

228

E18-13 We need to know the wave speed before we do anything else. This is found from Eq.

18-19,

v=sF

µ=sF

m/L =s(248 N)

(0.0978 kg)/(10.3 m) = 162 m/s.

The two pulses travel in opposite directions on the wire; one travels as distance x1in a time t, the

other travels a distance x2in a time t+ 29.6 ms, and since the pulses meet, we have x1+x2= 10.3

Our equations are then x1=vt = (162 m/s)t, and x2=v(t+29.6 ms) = (162 m/s)(t+29.6 ms) =

(162 m/s)t+ 4.80 m.We can add these two expressions together to solve for the time tat which the

pulses meet,

10.3 m = x1+x2= (162 m/s)t+ (162 m/s)t+ 4.80 m = (324 m/s)t+ 4.80 m.

which has solution t= 0.0170 s. The two pulses meet at x1= (162 m/s)(0.0170 s) = 2.75 m, or

x2= 7.55 m.

E18-14 (a) ∂y/∂r = (A/r)kcos(kr −ωt)−(A/r2) sin(kr −ωt).Multiply this by r2, and then ﬁnd

∂

∂r r2∂y

∂r =Ak cos(kr −ωt)−Ak2rsin(kr −ωt)−Ak cos(kr −ωt).

Simplify, and then divide by r2to get

∂

∂r r2∂y

∂r =−(Ak2/r) sin(kr −ωt).

Now ﬁnd ∂2y/∂t2=−Aω2sin(kr −ωt).But since 1/v2=k2/ω2, the two sides are equal.

(b) [length]2.

E18-15 The liner mass density is µ= (0.263 kg)/(2.72 m) = 9.669×10−2kg/m. The wave speed is

v=p(36.1 N)/(9.669×10−2kg/m) = 19.32 m/s.

Pav =1

2µω2ym2v, so

ω=s2(85.5 W)

(9.669×10−2kg/m)(7.70×10−3m)2(19.32 m/s) = 1243 rad/s.

Then f= (1243 rad/s)/2π= 199 Hz.

E18-16 (a) If the medium absorbs no energy then the power ﬂow through any closed surface

which contains the source must be constant. Since for a cylindrical surface the area grows as r, then

intensity must fall of as 1/r.

(b) Intensity is proportional to the amplitude squared, so the amplitude must fall oﬀ as 1/√r.

E18-17 The intensity is the average power per unit area (Eq. 18-33); as you get farther from the

source the intensity falls oﬀ because the perpendicular area increases. At some distance rfrom the

source the total possible area is the area of a spherical shell of radius r, so intensity as a function of

distance from the source would be

I=Pav

4πr2

229

We are given two intensities: I1= 1.13 W/m2at a distance r1;I2= 2.41 W/m2at a distance

r2=r1−5.30 m. Since the average power of the source is the same in both cases we can equate

these two values as

4πr2

1I1= 4πr2

2I2,

4πr2

1I1= 4π(r1−d)2I2,

where d= 5.30 m, and then solve for r1. Doing this we ﬁnd a quadratic expression which is

1I1= (r2

1−2dr1+d2)I2,

0 = 1−I1

I2r2

1−2dr1+d2,

0 = 1−(1.13 W/m2)

(2.41 W/m2)r2

1−2(5.30 m)r1+ (5.30 m)2,

0 = (0.531)r2

1−(10.6 m)r1+ (28.1 m2).

The solutions to this are r1= 16.8 m and r1= 3.15 m; but since the person walked 5.3 m toward

the lamp we will assume they started at least that far away. Then the power output from the light

P= 4πr2

1I1= 4π(16.8 m)2(1.13 W/m2) = 4.01×103W.

E18-18 Energy density is energy per volume, or u=U/V . A wave front of cross sectional area A

sweeps out a volume of V=Al when it travels a distance l. The wave front travels that distance

lin a time t=l/v. The energy ﬂow per time is the power, or P=U/t. Combine this with the

deﬁnition of intensity, I=P/A, and

I=P

A=U

At =uV

At =uAl

At =uv.

E18-19 Refer to Eq. 18-40, where the amplitude of the combined wave is

2ymcos(∆φ/2),

where ymis the amplitude of the combining waves. Then

cos(∆φ/2) = (1.65ym)/(2ym) = 0.825,

which has solution ∆φ= 68.8◦.

E18-20 Consider only the point x= 0. The displacement yat that point is given by

y=ym1 sin(ωt) + ym2 sin(ωt +π/2) = ym1 sin(ωt) + ym2 cos(ωt).

This can be written as

y=ym(A1sin ωt +A2cos ωt),

where Ai=ymi/ym. But if ymis judiciously chosen, A1= cos βand A2= sin β, so that

y=ymsin(ωt +β).

Since we then require A2

1+A2

2= 1, we must have

ym=p(3.20 cm)2+ (4.19 cm)2= 5.27 cm.

230

E18-21 The easiest approach is to use a phasor representation of the waves.

Write the phasor components as

x1=ym1 cos φ1,

y1=ym1 sin φ1,

x2=ym2 cos φ2,

y2=ym2 sin φ2,

and then use the cosine law to ﬁnd the magnitude of the resultant.

The phase angle can be found from the arcsine of the opposite over the hypotenuse.

E18-22 (a) The diagrams for all times except t= 15 ms should show two distinct pulses, ﬁrst

moving closer together, then moving farther apart. The pulses don not ﬂip over when passing each

other. The t= 15 ms diagram, however, should simply be a ﬂat line.

E18-23 Use a program such as Maple or Mathematica to plot this.

E18-24 Use a program such as Maple or Mathematica to plot this.

E18-25 (a) The wave speed can be found from Eq. 18-19; we need to know the linear mass

density, which is µ=m/L = (0.122 kg)/(8.36 m) = 0.0146 kg/m. The wave speed is then given by

v=sF

µ=s(96.7 N)

(0.0146 kg/m) = 81.4 m/s.

(b) The longest possible standing wave will be twice the length of the string; so λ= 2L= 16.7 m.

f=v

λ=(81.4 m/s)

(16.7 m) = 4.87 Hz

E18-26 (a) v=p(152 N)/(7.16×10−3kg/m) = 146 m/s.

(b) λ= (2/3)(0.894 m) = 0.596 m.

E18-27 (a) y=−3.9 cm.

(b) y= (0.15 m) sin[(0.79 rad/m)x+ (13 rad/s)t].

E18-28 (a) The amplitude is half of 0.520 cm, or 2.60 mm. The speed is

v= (137 rad/s)/(1.14 rad/cm) = 1.20 m/s.

(b) The nodes are (πrad)/(1.14 rad/cm) = 2.76 cm apart.

equation with respect to time, or

uy=−(0.520 cm)(137 rad/s) sin[(1.14 rad/cm)(1.47 cm)] sin[(137 rad/s)(1.36 s)] = −0.582 m/s.

231

E18-29 (a) We are given the wave frequency and the wave-speed, the wavelength is found from

Eq. 18-13,

λ=v

f=(388 m/s)

(622 Hz) = 0.624 m

The standing wave has four loops, so from Eq. 18-45

L=nλ

2= (4)(0.624 m)

2= 1.25 m

is the length of the string.

(b) We can just write it down,

y= (1.90 mm) sin[(2π/0.624 m)x] cos[(2π622 s−1)t].

E18-30 (a) fn=nv/2L= (1)(250 m/s)/2(0.150 m) = 833 Hz.

(b) λ=v/f = (348 m/s)/(833 Hz) = 0.418 m.

E18-31 v=pF/µ =pF L/m. Then fn=nv/2L=npF/4mL, so

f1= (1)p(236 N)/4(0.107 kg)(9.88 m)7.47 Hz,

and f2= 2f1= 14.9 Hz while f3= 3f1= 22.4 Hz.

E18-32 (a) v=pF/µ =pF L/m =p(122 N)(1.48 m)/(8.62×10−3kg) = 145 m/s.

(b) λ1= 2(1.48 m) = 2.96 m; λ2= 1.48 m.

E18-33 Although the tied end of the string forces it to be a node, the fact that the other end

is loose means that it should be an anti-node. The discussion of Section 18-10 indicated that the

spacing between nodes is always λ/2. Since anti-nodes occur between nodes, we can expect that the

distance between a node and the nearest anti-node is λ/4.

The longest possible wavelength will have one node at the tied end, an anti-node at the loose

end, and no other nodes or anti-nodes. In this case λ/4 = 120 cm, or λ= 480 cm.

The next longest wavelength will have a node somewhere in the middle region of the string. But

this means that there must be an anti-node between this new node and the node at the tied end

of the string. Moving from left to right, we then have an anti-node at the loose end, a node, and

anti-node, and ﬁnally a node at the tied end. There are four points, each separated by λ/4, so the

wavelength would be given by 3λ/4 = 120 cm, or λ= 160 cm.

To progress to the next wavelength we will add another node, and another anti-node. This will

add another two lengths of λ/4 that need to be ﬁt onto the string; hence 5λ/4 = 120 cm, or λ= 100

cm.

In the ﬁgure below we have sketched the ﬁrst three standing waves.

232

E18-34 (a) Note that fn=nf1. Then fn+1 −fn=f1. Since there is no resonant frequency

between these two then they must diﬀer by 1, and consequently f1= (420 Hz) −(315 Hz) = 105 Hz.

(b) v=fλ = (105 Hz)[2(0.756 m)] = 159 m/s.

P18-1 (a) λ=v/f and k= 360◦/λ. Then

x= (55◦)λ/(360◦) = 55(353 m/s)/360(493 Hz) = 0.109 m.

(b) ω= 360◦f, so

φ=ωt = (360◦)(493 Hz)(1.12×10−3s) = 199◦.

P18-2 ω= (2πrad)(548 Hz) = 3440 rad/s; λ=v/f and then

k= (2πrad)/[(326 m/s)/(548 Hz)] = 10.6 rad/m.

Finally, y= (1.12×10−2m) sin[(10.6 rad/m)x+ (3440 rad/s)t].

P18-3 (a) This problem really isn’t as bad as it might look. The tensile stress Sis tension per

unit cross sectional area, so

S=F

Aor F=SA.

We already know that linear mass density is µ=m/L, where Lis the length of the wire. Substituting

into Eq. 18-19,

v=sF

µ=sSA

m/LsS

m/(AL).

But AL is the volume of the wire, so the denominator is just the mass density ρ.

(b) The maximum speed of the transverse wave will be

v=sS

ρ=s(720 ×106Pa)

(7800 kg/m3)= 300 m/s.

P18-4 (a) f=ω/2π= (4.08 rad/s)/(2πrad) = 0.649 Hz.

(b) λ=v/f = (0.826 m/s)/(0.649 Hz) = 1.27 m.

y= (5.12 cm) sin[(4.95 rad/mx−(4.08 rad/s)t+φ],

where φis determined by (4.95 rad/m)(9.60×10−2m) + φ= (1.16 rad), or φ= 0.685 rad.

(d) F=µv2= (0.386 kg/m)(0.826 m/s)2= 0.263 m/s.

P18-5 We want to show that dy/dx =uy/v. The easy way, although not mathematically rigorous:

dx =dy

dt =dy

dx =uy

v=uy

P18-6 The maximum value for uyoccurs when the cosine function in Eq. 18-14 returns unity.

Consequently, um/ym=ω.

233

P18-7 (a) The linear mass density changes as the rubber band is stretched! In this case,

µ=m

L+ ∆L.

The tension in the rubber band is given by F=k∆L. Substituting this into Eq. 18-19,

v=sF

µ=rk∆L(L+ ∆L)

(b) We want to know the time it will take to travel the length of the rubber band, so

v=L+ ∆L

tor t=L+ ∆L

Into this we will substitute our expression for wave speed

t= (L+ ∆L)rm

k∆L(L+ ∆L)=rm(L+ ∆L)

k∆L

We have to possibilities to consider: either ∆LLor ∆LL. In either case we are only

interested in the part of the expression with L+ ∆L; whichever term is much larger than the other

will be the only signiﬁcant part.

Then if ∆LLwe get L+ ∆L≈Land

t=rm(L+ ∆L)

k∆L≈rmL

k∆L,

so that tis proportional to 1/√∆L.

But if ∆LLwe get L+ ∆L≈∆Land

t=rm(L+ ∆L)

k∆L≈rm∆L

k∆L=rm

so that tis constant.

P18-8 (a) The tension in the rope at some point is a function of the weight of the cable beneath

it. If the bottom of the rope is y= 0, then the weight beneath some point yis W=y(m/L)g. The

speed of the wave at that point is v=pT/(m/L) = py(M/L)g/(m/L) = √gy.

(b) dy/dt =√gy, so

dt =dy

√gy ,

t=ZL

√gy = 2pL/g.

P18-9 (a) M=Rµ dx, so

M=ZL

kx dx =1

2kL2.

Then k= 2M/L2.

(b) v=pF/µ =pF/kx, then

dt =pkx/F dx,

t=ZL

0p2M/F L2√x dx =2

3p2M/F L2L3/2=p8ML/9F .

234

P18-10 Take a cue from pressure and surface tension. In the rotating non-inertial reference frame

for which the hoop appears to be at rest there is an eﬀective force per unit length acting to push on

each part of the loop directly away from the center. This force per unit length has magnitude

∆F

∆L= (∆m/∆L)v2

r=µv2

There must be a tension Tin the string to hold the loop together. Imagine the loop to be replaced

with two semicircular loops. Each semicircular loop has a diameter part; the force tending to pull

oﬀ the diameter section is (∆F/∆L)2r= 2µv2. There are two connections to the diameter section,

so the tension in the string must be half the force on the diameter section, or T=µv2.

The wave speed is vw=pT/µ =v.

Note that the wave on the string can travel in either direction relative to an inertial observer.

One wave will appear to be ﬁxed in space; the other will move around the string with twice the

speed of the string.

P18-11 If we assume that Handel wanted his violins to play in tune with the other instruments

then all we need to do is ﬁnd an instrument from Handel’s time that will accurately keep pitch over

a period of several hundred years. Most instruments won’t keep pitch for even a few days because of

temperature and humidity changes; some (like the piccolo?) can’t even play in tune for more than

a few notes! But if someone found a tuning fork...

Since the length of the string doesn’t change, and we are using a string with the same mass

density, the only choice is to change the tension. But f∝v∝√T, so the percentage change in the

tension of the string is

Tf−Ti

=ff2−fi2

fi2=(440 Hz)2−(422.5 Hz)2

(422.5 Hz)2= 8.46 %.

P18-12

P18-13 (a) The point sources emit spherical waves; the solution to the appropriate wave equation

is found in Ex. 18-14:

yi=A

sin(kri−ωt).

If riis suﬃciently large compared to A, and r1≈r2, then let r1=r−δr and r2=r−δr;

r2≈2A

with an error of order (δr/r)2. So ignore it.

Then

y1+y2≈A

r[sin(kr1−ωt) + sin(kr2−ωt)] ,

=2A

rsin(kr −ωt) cos k

2(r1−r2),

ym=2A

rcos k

2(r1−r2).

(b) A maximum (minimum) occurs when the operand of the cosine, k(r1−r2)/2 is an integer

multiple of π(a half odd-integer multiple of π)

235

P18-14 The direct wave travels a distance dfrom Sto D. The wave which reﬂects oﬀ the original

layer travels a distance √d2+ 4H2between Sand D. The wave which reﬂects oﬀ the layer after

it has risen a distance htravels a distance pd2+ 4(H+h)2. Waves will interfere constructively if

there is a diﬀerence of an integer number of wavelengths between the two path lengths. In other

words originally we have

pd2+ 4H2−d=nλ,

and later we have destructive interference so

pd2+ 4(H+h)2−d= (n+ 1/2)λ.

We don’t know n, but we can subtract the top equation from the bottom and get

pd2+ 4(H+h)2−pd2+ 4H2=λ/2

P18-15 The wavelength is

λ=v/f = (3.00×108m/s)/(13.0×106Hz) = 23.1 m.

The direct wave travels a distance dfrom Sto D. The wave which reﬂects oﬀ the original layer

travels a distance √d2+ 4H2between Sand D. The wave which reﬂects oﬀ the layer one minute

later travels a distance pd2+ 4(H+h)2. Waves will interfere constructively if there is a diﬀerence

of an integer number of wavelengths between the two path lengths. In other words originally we

have pd2+ 4H2−d=n1λ,

and then one minute later we have

pd2+ 4(H+h)2−d=n2λ.

We don’t know either n1or n2, but we do know the diﬀerence is 6, so we can subtract the top

equation from the bottom and get

pd2+ 4(H+h)2−pd2+ 4H2= 6λ

We could use that expression as written, do some really obnoxious algebra, and then get the

answer. But we don’t want to; we want to take advantage of the fact that his small compared to d

and H. Then the ﬁrst term can be written as

pd2+ 4(H+h)2=pd2+ 4H2+ 8Hh + 4h2,

≈pd2+ 4H2+ 8Hh,

≈pd2+ 4H2r1 + 8H

d2+ 4H2h,

≈pd2+ 4H21 + 1

d2+ 4H2h.

Between the second and the third lines we factored out d2+ 4H2; that last line is from the binomial

expansion theorem. We put this into the previous expression, and

pd2+ 4(H+h)2−pd2+ 4H2= 6λ,

pd2+ 4H21 + 4H

d2+ 4H2h−pd2+ 4H2= 6λ,

√d2+ 4H2h= 6λ.

236

Now what were we doing? We were trying to ﬁnd the speed at which the layer is moving. We know

H,d, and λ; we can then ﬁnd h,

h=6(23.1 m)

4(510×103m) p(230×103m)2+ 4(510×103m)2= 71.0 m.

The layer is then moving at v= (71.0 m)/(60 s) = 1.18 m/s.

P18-16 The equation of the standing wave is

y= 2ymsin kx cos ωt.

The transverse speed of a point on the string is the derivative of this, or

uy=−2ymωsin kx sin ωt,

this has a maximum value when ωt −π/2 is a integer multiple of π. The maximum value is

um= 2ymωsin kx.

Each mass element on the string dm then has a maximum kinetic energy

dKm= (dm/2)um2=ym2ω2sin2kx dm.

Using dm =µdx, and integrating over one loop from kx = 0 to kx =π, we get

Km=ym2ω2µ/2k= 2π2ym2fµv.

P18-17 (a) For 100% reﬂection the amplitudes of the incident and reﬂected wave are equal, or

Ai=Ar, which puts a zero in the denominator of the equation for SWR. If there is no reﬂection,

Ar= 0 leaving the expression for SWR to reduce to Ai/Ai= 1.

(b) Pr/Pi=A2

r/A2

i. Do the algebra:

Ai+Ar

Ai−Ar

= SWR,

Ai+Ar= SWR(Ai−Ar),

Ar(SWR + 1) = Ai(SWR −1),

Ar/Ai= (SWR −1)/(SWR + 1).

Square this, and multiply by 100.

P18-18 Measure with a ruler; I get 2Amax = 1.1 cm and 2Amin = 0.5 cm.

(a) SWR = (1.1/0.5) = 2.2

(b) (2.2−1)2/(2.2 + 1)2= 0.14 %.

P18-19 (a) Call the three waves

yi=Asin k1(x−v1t),

yt=Bsin k2(x−v2t),

yr=Csin k1(x+v1t),

where the subscripts i, t, and r refer to the incident, transmitted, and reﬂected waves respectively.

237

Apply the principle of superposition. Just to the left of the knot the wave has amplitude yi+yr

while just to the right of the knot the wave has amplitude yt. These two amplitudes must line up

at the knot for all times t, or the knot will come undone. Remember the knot is at x= 0, so

yi+yr=yt,

Asin k1(−v1t) + Csin k1(+v1t) = Bsin k2(−v2t),

−Asin k1v1t+Csin k1v1t=−Bsin k2v2t

We know that k1v1=k2v2=ω, so the three sin functions are all equivalent, and can be canceled.

This leaves A=B+C.

(b) We need to match more than the displacement, we need to match the slope just on either

side of the knot. In that case we need to take the derivative of

yi+yr=yt

with respect to x, and then set x= 0. First we take the derivative,

dx (yi+yr) = d

dx (yt),

k1Acos k1(x−v1t) + k1Ccos k1(x+v1t) = k2Bcos k2(x−v2t),

and then we set x= 0 and simplify,

k1Acos k1(−v1t) + k1Ccos k1(+v1t) = k2Bcos k2(−v2t),

k1Acos k1v1t+k1Ccos k1v1t=k2Bcos k2v2t.

This last expression simpliﬁes like the one in part (a) to give

k1(A+C) = k2B

We can combine this with A=B+Cto solve for C,

k1(A+C) = k2(A−C),

C(k1+k2) = A(k2−k1),

C=Ak2−k1

k1+k2

If k2< k1Cwill be negative; this means the reﬂected wave will be inverted.

P18-20

P18-21 Find the wavelength from

λ= 2(0.924 m)/4 = 0.462 m.

Find the wavespeed from

v=fλ = (60.0 Hz)(0.462 m) = 27.7 m/s.

Find the tension from

F=µv2= (0.0442 kg)(27.7 m/s)2/(0.924 m) = 36.7 N.

238

P18-22 (a) The frequency of vibration fis the same for both the aluminum and steel wires; they

don not, however, need to vibrate in the same mode. The speed of waves in the aluminum is v1,

that in the steel is v2. The aluminum vibrates in a mode given by n1= 2L1f/v1, the steel vibrates

in a mode given by n2= 2L2f/v2. Both n1and n2need be integers, so the ratio must be a rational

fraction. Note that the ratio is independent of f, so that L1and L2must be chosen correctly for

this problem to work at all!

This ratio is

=L2

L1rµ2

µ1

=(0.866 m)

(0.600 m)s(7800 kg/m3)

(2600 kg/m3)= 2.50 ≈5

Note that since the wires have the same tension and the same cross sectional area it is acceptable

to use the volume density instead of the linear density in the problem.

The smallest integer solution is then n1= 2 and n2= 5. The frequency of vibration is then

f=n1v

2L1

=n1

2L1sT

ρ1A=(2)

2(0.600 m)s(10.0 kg)(9.81 m/s2)

(2600 kg/m3)(1.00×10−6m2)= 323 Hz.

(b) There are three nodes in the aluminum and six in the steel. But one of those nodes is shared,

and two are on the ends of the wire. The answer is then six.

239

E19-1 (a) v=fλ = (25 Hz)(0.24 m) = 6.0 m/s.

(b) k= (2πrad)/(0.24 m) = 26 rad/m; ω= (2πrad)(25 Hz) = 160 rad/s. The wave equation is

s= (3.0×10−3m) sin[(26 rad/m)x+ (160 rad/s)t]

E19-2 (a) [∆P]m= 1.48 Pa.

(b) f= (334πrad/s)/(2πrad) = 167 Hz.

(d) v= (167 Hz)(1.87 m) = 312 m/s.

E19-3 (a) The wavelength is given by λ=v/f = (343 m/s)/(4.50×106Hz = 7.62×10−5m.

(b) The wavelength is given by λ=v/f = (1500 m/s)/(4.50×106Hz = 3.33×10−4m.

E19-4 Note: There is a typo; the mean free path should have been measured in “µm” instead of

“pm”.

λmin = 1.0×10−6m; fmax = (343 m/s)/(1.0×10−6m) = 3.4×108Hz.

E19-5 (a) λ= (240 m/s)/(4.2×109Hz) = 5.7×10−8m.

E19-6 (a) The speed of sound is

v= (331 m/s)(6.21×10−4mi/m) = 0.206 mi/s.

In ﬁve seconds the sound travels (0.206 mi/s)(5.0 s) = 1.03 mi, which is 3% too large.

(b) Count seconds and divide by 3.

E19-7 Marching at 120 paces per minute means that you move a foot every half a second. The

soldiers in the back are moving the wrong foot, which means they are moving the correct foot half

a second later than they should. If the speed of sound is 343 m/s, then the column of soldiers must

be (343 m/s)(0.5 s) = 172 m long.

E19-8 It takes (300 m)/(343 m/s) = 0.87 s for the concert goer to hear the music after it has passed

the microphone. It takes (5.0×106m)/(3.0×108m/s) = 0.017 s for the radio listener to hear the music

after it has passed the microphone. The radio listener hears the music ﬁrst, 0.85 s before the concert

goer.

E19-9 x/vP=tPand x/vS=tS; subtracting and rearranging,

x= ∆t/[1/vS−1/vP] = (180 s)/[1/(4.5 km/s) −1/(8.2 km/s)] = 1800 km.

E19-10 Use Eq. 19-8, sm= [∆p]m/kB, and Eq. 19-14, v=pB/ρ0. Then

[∆p]m=kBsm=kv2ρ0sm= 2πfvρ0sm.

Insert into Eq. 19-18, and

I= 2π2ρvf2sm2.

E19-11 If the source emits equally in all directions the intensity at a distance ris given by the

average power divided by the surface area of a sphere of radius rcentered on the source.

The power output of the source can then be found from

P=IA =I(4πr2) = (197×10−6W/m2)4π(42.5 m)2= 4.47 W.

240

E19-12 Use the results of Exercise 19-10.

sm=s(1.13×10−6W/m2)

2π2(1.21 kg/m3)(343 m/s)(313 Hz)2= 3.75×10−8m.

E19-13 U=IAt = (1.60×10−2W/m2)(4.70×10−4m2)(3600 s) = 2.71×10−2W.

E19-14 Invert Eq. 19-21:

I1/I2= 10(1.00dB)/10 = 1.26.

E19-15 (a) Relative sound level is given by Eq. 19-21,

SL1−SL2= 10 log I1

or I1

= 10(SL1−SL2)/10,

so if ∆SL = 30 then I1/I2= 1030/10 = 1000.

(b) Intensity is proportional to pressure amplitude squared according to Eq. 19-19; so

∆pm,1/∆pm,2=pI1/I2=√1000 = 32.

E19-16 We know where her ears hurt, so we know the intensity at that point. The power output

is then

P= 4π(1.3 m)2(1.0 W/m2) = 21 W.

This is less than the advertised power.

E19-17 Use the results of Exercise 18-18, I=uv. The intensity is

I= (5200 W)/4π(4820 m)2= 1.78×10−5W/m2,

so the energy density is

u=I/v = (1.78×10−5W/m2)/(343 m/s) = 5.19×10−8J/m3.

E19-18 I2= 2I1, since I∝1/r2then r2

1= 2r2

2. Then

D=√2(D−51.4 m),

D(√2−1) = √2(51.4 m),

D= 176 m.

E19-19 The sound level is given by Eq. 19-20,

SL = 10 log I

where I0is the threshold intensity of 10−12 W/m2. Intensity is given by Eq. 19-19,

I=(∆pm)2

2ρv

If we assume the maximum possible pressure amplitude is equal to one atmosphere, then

I=(∆pm)2

2ρv =(1.01×105Pa)2

2(1.21 kg/m3)(343 m/s) = 1.22×107W/m2.

241

The sound level would then be

SL = 10 log I

= 10 log 1.22×107W/m2

(10−12 W/m2)= 191 dB

E19-20 Let one person speak with an intensity I1.Npeople would have an intensity NI1. The

ratio is N, so by inverting Eq. 19-21,

N= 10(15dB)/10 = 31.6,

so 32 people would be required.

E19-21 Let one leaf rustle with an intensity I1.Nleaves would have an intensity N I1. The ratio

is N, so by Eq. 19-21,

SLN= (8.4 dB) + 10 log(2.71×105) = 63 dB.

E19-22 Ignoring the ﬁnite time means that we can assume the sound waves travels vertically,

which considerably simpliﬁes the algebra.

The intensity ratio can be found by inverting Eq. 19-21,

I1/I2= 10(30dB)/10 = 1000.

But intensity is proportional to the inverse distance squared, so I1/I2= (r2/r1)2, or

r2= (115 m)p(1000) = 3640 m.

E19-23 A minimum will be heard at the detector if the path length diﬀerence between the

straight path and the path through the curved tube is half of a wavelength. Both paths involve a

straight section from the source to the start of the curved tube, and then from the end of the curved

tube to the detector. Since it is the path diﬀerence that matters, we’ll only focus on the part of

the path between the start of the curved tube and the end of the curved tube. The length of the

straight path is one diameter, or 2r. The length of the curved tube is half a circumference, or πr.

The diﬀerence is (π−2)r. This diﬀerence is equal to half a wavelength, so

(π−2)r=λ/2,

r=λ

2π−4=(42.0 cm)

2π−4= 18.4 cm.

E19-24 The path length diﬀerence here is

p(3.75 m)2+ (2.12 m)2−(3.75 m) = 0.5578 m.

(a) A minimum will occur if this is equal to a half integer number of wavelengths, or (n−1/2)λ=

0.5578 m. This will occur when

f= (n−1/2) (343 m/s)

(0.5578 m) = (n−1/2)(615 Hz).

(b) A maximum will occur if this is equal to an integer number of wavelengths, or nλ = 0.5578 m.

This will occur when

f=n(343 m/s)

(0.5578 m) =n(615 Hz).

242

E19-25 The path length diﬀerence here is

p(24.4 m + 6.10 m)2+ (15.2 m)2−p(24.4 m)2+ (15.2 m)2= 5.33 m.

A maximum will occur if this is equal to an integer number of wavelengths, or nλ = 5.33 m.This

will occur when

f=n(343 m/s)/(5.33 m) = n(64.4 Hz)

The two lowest frequencies are then 64.4 Hz and 129 Hz.

E19-26 The wavelength is λ= (343 m/s)/(300 Hz) = 1.143 m. This means that the sound maxima

will be half of this, or 0.572 m apart. Directly in the center the path length diﬀerence is zero, but

since the waves are out of phase, this will be a minimum. The maxima should be located on either

side of this, a distance (0.572 m)/2=0.286 m from the center. There will then be maxima located

each 0.572 m farther along.

E19-27 (a) f1=v/2Land f2=v/2(L−∆L). Then

r=f1

=L−∆L

L= 1 −∆L

or ∆L=L(1 −1/r).

(b) The answers are ∆L= (0.80 m)(1 −5/6) = 0.133 m; ∆L= (0.80 m)(1 −4/5) = 0.160 m;

∆L= (0.80 m)(1 −3/4) = 0.200 m; and ∆L= (0.80 m)(1 −2/3) = 0.267 m.

E19-28 The wavelength is twice the distance between the nodes in this case, so λ= 7.68 cm. The

frequency is

f= (1520 m/s)/(7.68×10−2m) = 1.98×104Hz.

E19-29 The well is a tube open at one end and closed at the other; Eq. 19-28 describes the

allowed frequencies of the resonant modes. The lowest frequency is when n= 1, so f1=v/4L. We

know f1; to ﬁnd the depth of the well, L, we need to know the speed of sound.

We should use the information provided, instead of looking up the speed of sound, because maybe

the well is ﬁlled with some kind of strange gas.

Then, from Eq. 19-14,

v=sB

ρ=s(1.41 ×105Pa)

(1.21 kg/m3)= 341 m/s.

The depth of the well is then

L=v/(4f1) = (341 m/s)/[4(7.20 Hz)] = 11.8 m.

E19-30 (a) The resonant frequencies of the pipe are given by fn=nv/2L, or

fn=n(343 m/s)/2(0.457 m) = n(375 Hz).

The lowest frequency in the speciﬁed range is f3= 1130 Hz; the other allowed frequencies in the

speciﬁed range are f4= 1500 Hz, and f5= 1880 Hz.

243

E19-31 The maximum reﬂected frequencies will be the ones that undergo constructive inter-

ference, which means the path length diﬀerence will be an integer multiple of a wavelength. A

wavefront will strike a terrace wall and part will reﬂect, the other part will travel on to the next

terrace, and then reﬂect. Since part of the wave had to travel to the next terrace and back, the path

length diﬀerence will be 2 ×0.914 m = 1.83 m.

If the speed of sound is v= 343 m/s, the lowest frequency wave which undergoes constructive

interference will be

f=v

λ=(343 m/s)

(1.83 m) = 187 Hz

Any integer multiple of this frequency will also undergo constructive interference, and will also be

heard. The ear and brain, however, will most likely interpret the complex mix of frequencies as a

single tone of frequency 187 Hz.

E19-32 Assume there is no frequency between these two that is ampliﬁed. Then one of these

frequencies is fn=nv/2L, and the other is fn+1 = (n+ 1)v/2L. Subtracting the larger from the

smaller, ∆f=v/2L, or

L=v/2∆f= (343 m/s)/2(138 Hz −135 Hz) = 57.2 m.

E19-33 (a) v= 2Lf = 2(0.22 m)(920 Hz) = 405 m/s.

(b) F=v2µ= (405 m/s)2(820×10−6kg)/(0.220 m) = 611 N.

E19-34 f∝v, and v∝√F, so f∝√F. Doubling frequires Fincrease by a factor of 4.

E19-35 The speed of a wave on the string is the same, regardless of where you put your ﬁnger,

so fλ is a constant. The string will vibrate (mostly) in the lowest harmonic, so that λ= 2L, where

Lis the length of the part of the string that is allowed to vibrate. Then

f2λ2=f1λ1,

2f2L2= 2f1L1,

L2=L1

= (30 cm) (440 Hz)

(528 Hz) = 25 cm.

So you need to place your ﬁnger 5 cm from the end.

E19-36 The open organ pipe has a length

Lo=v/2f1= (343 m/s)/2(291 Hz) = 0.589 m.

The second harmonic of the open pipe has frequency 2f1; this is the ﬁrst overtone of the closed pipe,

so the closed pipe has a length

Lc= (3)v/4(2f1) = (3)(343 m/s)/4(2)(291 Hz) = 0.442 m.

E19-37 The unknown frequency is either 3 Hz higher or lower than the standard fork. A small

piece of wax placed on the fork of this unknown frequency tuning fork will result in a lower frequency

because f∝pk/m. If the beat frequency decreases then the two tuning forks are getting closer

in frequency, so the frequency of the ﬁrst tuning fork must be above the frequency of the standard

fork. Hence, 387 Hz.

244

E19-38 If the string is too taut then the frequency is too high, or f= (440 + 4)Hz. Then

T= 1/f = 1/(444 Hz) = 2.25×10−3s.

E19-39 One of the tuning forks need to have a frequency 1 Hz diﬀerent from another. Assume

then one is at 501 Hz. The next fork can be played against the ﬁrst or the second, so it could have

a frequency of 503 Hz to pick up the 2 and 3 Hz beats. The next one needs to pick up the 5, 7, and

8 Hz beats, and 508 Hz will do the trick. There are other choices.

E19-40 f=v/λ = (5.5 m/s)/(2.3 m) = 2.39 Hz. Then

f0=f(v+vO)/v = (2.39 Hz)(5.5 m/s + 3.3 m/s)/(5.5 m/s) = 3.8 Hz.

E19-41 We’ll use Eq. 19-44, since both the observer and the source are in motion. Then

f0=fv±vO

v∓vS

= (15.8 kHz)(343 m/s) + (246 m/s)

(343 m/s) + (193 m/s) = 17.4 kHzr

E19-42 Solve Eq. 19-44 for vS;

vS= (v+vO)f/f0−v= (343 m/s + 2.63 m/s)(1602 Hz)/(1590 Hz) −(343 m/s) = 5.24 m/s.

E19-43 vS= (14.7 Rad/s)(0.712 m) = 10.5 m/s.

(a) The low frequency heard is

f0= (538 Hz)(343 m/s)/(343 m/s + 10.5 m/s) = 522 Hz.

(a) The high frequency heard is

f0= (538 Hz)(343 m/s)/(343 m/s−10.5 m/s) = 555 Hz.

E19-44 Solve Eq. 19-44 for vS;

vS=v−vf/f0= (343 m/s) −(343 m/s)(440 Hz)/(444 Hz) = 3.1 m/s.

E19-45

E19-46 The approaching car “hears”

f0=fv+vO

v−vS

= (148 Hz) (343 m/s) + (44.7 m/s)

(343 m/s) −(0) = 167 Hz

This sound is reﬂected back at the same frequency, so the police car “hears”

f0=fv+vO

v−vS

= (167 Hz) (343 m/s) + (0)

(343 m/s) −(44.7 m/s) = 192 Hz

E19-47 The departing intruder “hears”

f0=fv−vO

v+vS

= (28.3 kHz)(343 m/s) −(0.95 m/s)

(343 m/s) −(0) = 28.22 kHz

This sound is reﬂected back at the same frequency, so the alarm “hears”

f0=fv−vO

v+vS

= (28.22 kHz) (343 m/s) + (0)

(343 m/s) + (0.95 m/s) = 28.14 kHz

The beat frequency is 28.3 kHz −28.14 kHz = 160 Hz.

245

E19-48 (a) f0= (1000 Hz)(330 m/s)(330 m/s + 10.0 m/s) = 971 Hz.

(b) f0= (1000 Hz)(330 m/s)(330 m/s−10.0 m/s) = 1030 Hz.

E19-49 (a) The frequency “heard” by the wall is

f0=fv+vO

v−vS

= (438 Hz) (343 m/s) + (0)

(343 m/s) −(19.3 m/s) = 464 Hz

(b) The wall then reﬂects a frequency of 464 Hz back to the trumpet player. Sticking with Eq.

19-44, the source is now at rest while the observer moving,

f0=fv+vO

v−vS

= (464 Hz) (343 m/s) + (19.3 m/s)

(343 m/s) −(0) = 490 Hz

E19-50 The body part “hears”

f0=fv+vb

This sound is reﬂected back to the detector which then “hears”

f00 =f0v

v−vb

=fv+vb

v−vb

Rearranging,

vb/v =f00 −f

f00 +f≈1

∆f

so v≈2(1×10−3m/s)/(1.3×10−6)≈1500 m/s.

E19-51 The wall “hears”

f0=fv+vO

v−vS

= (39.2 kHz) (343 m/s) + (0)

(343 m/s) −(8.58 m/s) = 40.21 kHz

This sound is reﬂected back at the same frequency, so the bat “hears”

f0=fv+vO

v−vS

= (40.21 kHz)(343 m/s) + (8.58 m/s)

(343 m/s) −(0) = 41.2 kHz.

P19-1 (a) tair =L/vair and tm=L/v, so the diﬀerence is

∆t=L(1/vair −1/v)

(b) Rearrange the above result, and

L= (0.120 s)/[1/(343 m/s) −1/(6420 m/s)] = 43.5 m.

P19-2 The stone falls for a time t1where y=gt2

1/2 is the depth of the well. Note yis positive in

this equation. The sound travels back in a time t2where v=y/t2is the speed of sound in the well.

t1+t2= 3.00 s, so

2y=g(3.00 s −t2)2=g[(9.00 s2)−(6.00 s)y/v +y2/v2],

or, using g= 9.81 m/s2and v= 343 m/s,

y2−(2.555×105m)y+ (1.039×107m2) = 0,

which has a positive solution y= 40.7 m.

246

P19-3 (a) The intensity at 28.5 m is found from the 1/r2dependence;

I2=I1(r1/r2)2= (962 µW/m2)(6.11 m/28.5 m)2= 44.2µW/m2.

∆pm=p2ρvI =p2(1.21 kg/m3)(343 m/s)(962×10−6W/m2) = 0.894 Pa.

(b) The displacement amplitude is found from Eq. 19-8,

sm= ∆pm/(kB),

where k= 2πf/v is the wave number. From Eq. 19-14 w know that B=ρv2, so

sm=∆pm

2πfρv =(0.894 Pa)

2π(2090 Hz)(1.21 kg/m3)(343 m/s) = 1.64×10−7m.

P19-4 (a) If the intensities are equal, then ∆pm∝√ρv, so

[∆pm]water

[∆pm]air

=s(998 kg/m3)(1482 m/s)

(1.2 kg/m3)(343 m/s) = 59.9.

(b) If the pressure amplitudes are equal, then I∝∝ 1/ρv, so

Iwater

Iair

=(1.2 kg/m3)(343 m/s)

(998 kg/m3)(1482 m/s) = 2.78×10−4.

P19-5 The energy is dissipated on a cylindrical surface which grows in area as r, so the intensity is

proportional to 1/r. The amplitude is proportional to the square root of the intensity, so sm∝1/√r.

P19-6 (a) The ﬁrst position corresponds to maximum destructive interference, so the waves are half

a wavelength out of phase; the second position corresponds to maximum constructive interference, so

the waves are in phase. Shifting the tube has in eﬀect added half a wavelength to the path through

B. But each segment is added, so

λ= (2)(2)(1.65 cm) = 6.60 cm,

and f= (343 m/s)/(6.60 cm) = 5200 Hz.

(b) Imin ∝(s1−s2)2,Imax ∝(s1+s2)2, then dividing one expression by the other and rearranging

we ﬁnd

=√Imax +√Imin

√Imax −√Imin

=√90 + √10

√90 −√10 = 2

P19-7 (a) I=P/4πr2= (31.6 W)/4π(194 m)2= 6.68×10−5W/m2.

(b) P=IA = (6.68×10−5W/m2)(75.2×10−6m2) = 5.02×10−9W.

P19-8 Note that the reverberation time is logarithmically related to the intensity, but linearly

related to the sound level. As such, the reverberation time is the amount of time for the sound level

to decrease by

∆SL = 10 log(10−6) = 60 dB.

Then

t= (87 dB)(2.6s)/(60 dB) = 3.8 s

247

P19-9 What the device is doing is taking all of the energy which strikes a large surface area and

concentrating it into a small surface area. It doesn’t succeed; only 12% of the energy is concentrated.

We can think, however, in terms of power: 12% of the average power which strikes the parabolic

reﬂector is transmitted into the tube.

If the sound intensity on the reﬂector is I1, then the average power is P1=I1A1=I1πr2

1, where

r1is the radius of the reﬂector. The average power in the tube will be P2= 0.12P1, so the intensity

in the tube will be

I2=P2

=0.12I1πr2

πr2

= 0.12I1

Since the lowest audible sound has an intensity of I0= 10−12 W/m2, we can set I2=I0as the

condition for “hearing” the whisperer through the apparatus. The minimum sound intensity at the

parabolic reﬂector is

I1=I0

0.12

Now for the whisperers. Intensity falls oﬀ as 1/d2, where dis the distance from the source. We

are told that when d= 1.0 m the sound level is 20 dB; this sound level has an intensity of

I=I01020/10 = 100I0

Then at a distance dfrom the source the intensity must be

I1= 100I0

(1 m)2

d2.

This would be the intensity “picked-up” by the parabolic reﬂector. Combining this with the condition

for being able to hear the whisperers through the apparatus, we have

0.12

= 100I0

(1 m)2

or, upon some rearranging,

d= (√12 m)r1

= (√12 m) (0.50 m)

(0.005 m) = 346 m.

P19-10 (a) A displacement node; at the center the particles have nowhere to go.

(b) This systems acts like a pipe which is closed at one end.

T= 4(0.009)(6.96×108m)p(1.0×1010kg/m3)/(1.33×1022Pa) = 22 s.

P19-11 The cork ﬁling collect at pressure antinodes when standing waves are present, and the

antinodes are each half a wavelength apart. Then v=fλ =f(2d).

P19-12 (a) f=v/4L= (343 m/s)/4(1.18 m) = 72.7 Hz.

(b) F=µv2=µf2λ2, or

F= (9.57×10−3kg/0.332 m)(72.7 Hz)2[2(0.332 m)]2= 67.1N.

248

P19-13 In this problem the string is observed to resonate at 880 Hz and then again at 1320 Hz,

so the two corresponding values of nmust diﬀer by 1. We can then write two equations

(880 Hz) = nv

2Land (1320 Hz) = (n+ 1)v

and solve these for v. It is somewhat easier to ﬁrst solve for n. Rearranging both equations, we get

(880 Hz)

n=v

2Land (1320 Hz)

n+ 1 =v

2L.

Combining these two equations we get

(880 Hz)

n=(1320 Hz)

n+ 1 ,

(n+ 1)(880 Hz) = n(1320 Hz),

n=(880 Hz)

(1320 Hz) −(880 Hz) = 2.

Now that we know nwe can ﬁnd v,

v= 2(0.300 m)(880 Hz)

2= 264 m/s

And, ﬁnally, we are in a position to ﬁnd the tension, since

F=µv2= (0.652×10−3kg/m)(264 m/s)2= 45.4 N.

P19-14 (a) There are ﬁve choices for the ﬁrst fork, and four for the second. That gives 20 pairs.

But order doesn’t matter, so we need divide that by two to get a maximum of 10 possible beat

frequencies.

(b) If the forks are ordered to have equal diﬀerences (say, 400 Hz, 410 Hz, 420 Hz, 430 Hz, and

440 Hz) then there will actually be only 4 beat frequencies.

P19-15 v= (2.25×108m/s)/sin(58.0◦) = 2.65×108m/s.

P19-16 (a) f1= (442 Hz)(343 m/s)/(343 m/s−31.3 m/s) = 486 Hz, while

f2= (442 Hz)(343 m/s)/(343 m/s + 31.3 m/s) = 405 Hz,

so ∆f= 81 Hz.

(b) f1= (442 Hz)(343 m/s−31.3 m/s)/(343 m/s) = 402 Hz, while

f2= (442 Hz)(343 m/s + 31.3 m/s)/(343 m/s) = 482 Hz,

so ∆f= 80 Hz.

P19-17 The sonic boom that you hear is not from the sound given oﬀ by the plane when it is

overhead, it is from the sound given oﬀ before the plane was overhead. So this problem isn’t as

simple as distance equals velocity ×time. It is very useful to sketch a picture.

249

x2 x1H

We can ﬁnd the angle θfrom the ﬁgure, we’ll get Eq. 19-45, so

sin θ=v

=(330 m/s)

(396 m/s) = 0.833 or θ= 56.4◦

Note that vsis the speed of the source, not the speed of sound!

Unfortunately t= 12 s is not the time between when the sonic boom leaves the plane and when

it arrives at the observer. It is the time between when the plane is overhead and when the sonic

boom arrives at the observer. That’s why there are so many marks and variables on the ﬁgure. x1

is the distance from where the sonic boom which is heard by the observer is emitted to the point

directly overhead; x2is the distance from the point which is directly overhead to the point where

the plane is when the sonic boom is heard by the observer. We do have x2=vs(12.0 s). This length

forms one side of a right triangle HSO, the opposite side of this triangle is the side HO, which is

the height of the plane above the ground, so

h=x2tan θ= (343 m/s)(12.0 s) tan(56.4◦) = 7150 m.

P19-18 (a) The target “hears”

f0=fs

v+V

This sound is reﬂected back to the detector which then “hears”

fr=f0v

v−V=fs

v+V

v−V.

(b) Rearranging,

V/v =fr−fs

fr+fs≈1

fr−fs

where we have assumed that the source frequency and the reﬂected frequency are almost identical,

so that when added fr+fs≈2fs.

P19-19 (a) We apply Eq. 19-44

f0=fv+vO

v−vS

= (1030 Hz) (5470 km/h) + (94.6 km/h)

(5470 km/h) −(20.2 km/h) = 1050 Hz

250

(b) The reﬂected signal has a frequency equal to that of the signal received by the second sub

originally. Applying Eq. 19-44 again,

f0=fv+vO

v−vS

= (1050 Hz) (5470 km/h) + (20.2 km/h)

(5470 km/h) −(94.6 km/h) = 1070 Hz

P19-20 In this case vS= 75.2 km/h −30.5 km/h = 12.4 m/s. Then

f0= (989 Hz)(1482 m/s)(1482 m/s−12.4 m/s) = 997 Hz.

P19-21 There is no relative motion between the source and observer, so there is no frequency shift

regardless of the wind direction.

P19-22 (a) vS= 34.2 m/s and vO= 34.2 m/s, so

f0= (525 Hz)(343 m/s + 34.2 m/s)/(343 m/s−34.2 m/s) = 641 Hz.

(b) vS= 34.2 m/s + 15.3 m/s = 49.5 m/s and vO= 34.2 m/s−15.3 m/s = 18.9 m/s, so

f0= (525 Hz)(343 m/s + 18.9 m/s)/(343 m/s−49.5 m/s) = 647 Hz.

f0= (525 Hz)(343 m/s + 49.5 m/s)/(343 m/s−18.9 m/s) = 636 Hz.

251

E20-1 (a) t=x/v = (0.20 m)/(0.941)(3.00×108m/s) = 7.1×10−10s.

(b) y=−gt2/2 = −(9.81 m/s2)(7.1×10−10s)2/2 = 2.5×10−18m.

E20-2 L=L0p1−u2/c2= (2.86 m)p1−(0.999987)2= 1.46 cm.

E20-3 L=L0p1−u2/c2= (1.68 m)p1−(0.632)2= 1.30 m.

E20-4 Solve ∆t= ∆t0/p1−u2/c2for u:

u=cs1−∆t0

∆t2

= (3.00×108m/s)s1−(2.20 µs)

(16.0µs)2

= 2.97×108m/s.

E20-5 We can apply ∆x=v∆tto ﬁnd the time the particle existed before it decayed. Then

∆t=x

(1.05 ×10−3m)

(0.992)(3.00 ×108m/s) = 3.53 ×10−12 s.

The proper lifetime of the particle is

∆t0= ∆tp1−u2/c2= (3.53 ×10−12 s)p1−(0.992)2= 4.46 ×10−13 s.

E20-6 Apply Eq. 20-12:

v=(0.43c) + (0.587c)

1 + (0.43c)(0.587c)/c2= 0.812c.

E20-7 (a) L=L0p1−u2/c2= (130 m)p1−(0.740)2= 87.4 m

(b) ∆t=L/v = (87.4 m)/(0.740)(3.00×108m/s) = 3.94×10−7s.

E20-8 ∆t= ∆t0/p1−u2/c2= (26 ns)p1−(0.99)2= 184 ns.Then

L=v∆t= (0.99)(3.00×108m/s)(184×10−9s) = 55 m.

E20-9 (a) vg= 2v= (7.91 + 7.91) km/s = 15.82 km/s.

(b) A relativistic treatment yields vr= 2v/(1 + v2/c2). The fractional error is

vr−1 = 1 + v2

c2−1 = v2

c2=(7.91×103m/s)2

(3.00×108m/s)2= 6.95×10−10.

E20-10 Invert Eq. 20-15 to get β=p1−1/γ2.

(a) β=p1−1/(1.01)2= 0.140.

(b) β=p1−1/(10.0)2= 0.995.

(d) β=p1−1/(1000)2= 0.9999995.

252

E20-11 The distance traveled by the particle is (6.0 y)c; the time required for the particle to

travel this distance is 8.0 years. Then the speed of the particle is

v=∆x

∆t=(6.0 y)c

(8.0 y) =3

4c.

The speed parameter βis given by

β=v

c=3

E20-12 γ= 1/p1−(0.950)2= 3.20. Then

x0= (3.20)[(1.00×105m) −(0.950)(3.00×108m/s)(2.00×10−4s)] = 1.38×105m,

t0= (3.20)[(2.00×10−4s) −(1.00×105m)(0.950)/(3.00×108m/s)] = −3.73×10−4s.

E20-13 (a) γ= 1/p1−(0.380)2= 1.081. Then

x0= (1.081)[(3.20×108m) −(0.380)(3.00×108m/s)(2.50 s)] = 3.78×107m,

t0= (1.081)[(2.50 s) −(3.20×108m)(0.380)/(3.00×108m/s)] = 2.26 s.

(b) γ= 1/p1−(0.380)2= 1.081. Then

x0= (1.081)[(3.20×108m) −(−0.380)(3.00×108m/s)(2.50 s)] = 6.54×108m,

t0= (1.081)[(2.50 s) −(3.20×108m)(−0.380)/(3.00×108m/s)] = 3.14 s.

E20-14

E20-15 (a) v0

x= (−u)/(1 −0) and v0

y=cp1−u2/c2.

(b) (v0

x)2+ (v0

y)2=u2+c2−u2=c2.

E20-16 v0= (0.787c+ 0.612c)/[1 + (0.787)(0.612)] = 0.944c.

E20-17 (a) The ﬁrst part is easy; we appear to be moving away from Aat the same speed as A

appears to be moving away from us: 0.347c.

(b) Using the velocity transformation formula, Eq. 20-18,

x=vx−u

1−uvx/c2=(0.347c)−(−0.347c)

1−(−0.347c)(0.347c)/c2= 0.619c.

The negative sign reﬂects the fact that these two velocities are in opposite directions.

E20-18 v0= (0.788c−0.413c)/[1 + (0.788)(−0.413)] = 0.556c.

E20-19 (a) γ= 1/p1−(0.8)2= 5/3.

x=vx

γ(1 −uvy/c2)=3(0.8c)

5[1 −(0)] =12

25c,

y=vy−u

1−uvy/c2=(0) −(0.8c)

1−(0) =−4

5c.

Then v0=cp(−4/5)2+ (12/25)2= 0.933cdirected θ= arctan(−12/20) = 31◦East of South.

253

(b) γ= 1/p1−(0.8)2= 5/3.

x=vx−u

1−uvx/c2=(0) −(−0.8c)

1−(0) = +4

5c,

y=vy

γ(1 −uvx/c2)=3(0.8c)

5[1 −(0)] =12

25c.

Then v0=cp(4/5)2+ (12/25)2= 0.933cdirected θ= arctan(20/12) = 59◦West of North.

E20-20 This exercise should occur in Section 20-9.

(a) v= 2π(6.37×106m)c/(1 s)(3.00×108m/s) = 0.133c.

(b) K= (γ−1)mc2= (1/p1−(0.133)2−1)(511 keV) = 4.58 keV.

(4.52 −4.58)/(4.58) = −1.31%.

E20-21 ∆L=L0−L0so

∆L= 2(6.370×106m)(1 −p1−(29.8×103m/s)2/(3.00×108m/s)2) = 6.29×10−2m.

E20-22 (a) ∆L/L0= 1 −L0/L0so

∆L= (1 −p1−(522 m/s)2/(3.00×108m/s)2) = 1.51×10−12.

(b) We want to solve ∆t−∆t0= 1 µs, or

1µs=∆t(1 −1/p1−(522 m/s)2/(3.00×108m/s)2),

which has solution ∆t= 6.61×105s. That’s 7.64 days.

E20-23 The length of the ship as measured in the “certain” reference frame is

L=L0p1−v2/c2= (358 m)p1−(0.728)2= 245 m.

In a time ∆tthe ship will move a distance x1=v1∆twhile the micrometeorite will move a distance

x2=v2∆t; since they are moving toward each other then the micrometeorite will pass then ship

when x1+x2=L. Then

∆t=L/(v1+v2) = (245 m)/[(0.728 + 0.817)(3.00×108m/s)] = 5.29×10−7s.

This answer is the time measured in the “certain” reference frame. We can use Eq. 20-21 to ﬁnd

the time as measured on the ship,

∆t=∆t0+u∆x0/c2

p1−u2/c2=(5.29×10−7s) + (0.728c)(116 m)/c2

p1−(0.728)2= 1.23×10−6s.

E20-24 (a) γ= 1/p1−(0.622)2= 1.28.

(b) ∆t= (183 m)/(0.622)(3.00×108m/s) = 9.81×10−7s.On the clock, however,

∆t0= ∆t/γ = (9.81×10−7s)/(1.28) = 7.66×10−7s.

254

E20-25 (a) ∆t= (26.0 ly)/(0.988)(1.00 ly/y) = 26.3 y.

(b) The signal takes 26 years to return, so 26 + 26.3 = 52.3 years.

E20-26 (a) γ= (1000 y)(1 y) = 1000;

v=cp1−1/γ2≈c(1 −1/2γ2) = 0.9999995c

(b) No.

E20-27 (5.61×1029 MeV/c2)c/(3.00×108m/s) = 1.87×1021 MeV/c.

E20-28 p2=m2c2=m2v2/(1 −v2/c2), so 2v2/c2= 1, or v=√2c.

E20-29 The magnitude of the momentum of a relativistic particle in terms of the magnitude of

the velocity is given by Eq. 20-23,

p=mv

p1−v2/c2.

The speed parameter, β, is what we are looking for, so we need to rearrange the above expression

for the quantity v/c.

p/c =mv/c

p1−v2/c2,

c=mβ

p1−β2,

p=p1−β2

β,

p=p1/β2−1.

Rearranging,

mc2

pc =p1/β2−1,

mc2

pc 2

β2−1,

smc2

pc 2

+ 1 = 1

β,

pm2c4+p2c2=β

(a) For the electron,

β=(12.5 MeV/c)c

p(0.511 MeV/c2)2c4+ (12.5 MeV/c)2c2= 0.999.

(b) For the proton,

β=(12.5 MeV/c)c

p(938 MeV/c2)2c4+ (12.5 MeV/c)2c2= 0.0133.

255

E20-30 K=mc2(γ−1), so γ= 1 + K/mc2.β=p1−1/γ2.

(a) γ= 1 + (1.0 keV)/(511 keV) = 1.00196. β= 0.0625c.

(b) γ= 1 + (1.0 MeV)/(0.511 MeV) = 2.96. β= 0.941c.

E20-31 The kinetic energy is given by Eq. 20-27,

K=mc2

p1−v2/c2−mc2.

We rearrange this to solve for β=v/c,

β=s1−mc2

K+mc22

It is actually much easier to ﬁnd γ, since

γ=1

p1−v2/c2,

so K=γmc2−mc2implies

γ=K+mc2

mc2

(a) For the electron,

β=s1−(0.511 MeV/c2)c2

(10 MeV) + (0.511 MeV/c2)c22

= 0.9988,

and

γ=(10 MeV) + (0.511 MeV/c2)c2

(0.511 MeV/c2)c2= 20.6.

(b) For the proton,

β=s1−(938 MeV/c2)c2

(10 MeV) + (938 MeV/c2)c22

= 0.0145,

and

γ=(10 MeV) + (938 MeV/c2)c2

(938 MeV/c2)c2= 1.01.

(b) For the alpha particle,

β=s1−4(938 MeV/c2)c2

(10 MeV) + 4(938 MeV/c2)c22

= 0.73,

and

γ=(10 MeV) + 4(938 MeV/c2)c2

4(938 MeV/c2)c2= 1.0027.

E20-32 γ= 1/p1−(0.99)2= 7.089.

(a) E=γmc2= (7.089)(938.3 MeV) = 6650 MeV. K=E−mc2= 5710 MeV. p=mvγ =

(938.3 MeV/c2)(0.99c)(7.089) = 6580 MeV/c.

(b) E=γmc2= (7.089)(0.511 MeV) = 3.62 MeV. K=E−mc2= 3.11 MeV. p=mvγ =

(0.511 MeV/c2)(0.99c)(7.089) = 3.59 MeV/c.

256

E20-33 ∆m/∆t= (1.2×1041W)/(3.0×108m/s)2= 1.33×1024kg/s, which is

∆m

∆t=(1.33×1024kg/s)(3.16×107s/y)

(1.99×1030kg/sun) = 21.1

E20-34 (a) If K=E−mc2= 2mc2, then E= 3mc2, so γ= 3, and

v=cp1−1/γ2=cp1−1/(3)2= 0.943c.

(b) If E= 2mc2, then γ= 2, and

v=cp1−1/γ2=cp1−1/(2)2= 0.866c.

E20-35 (a) The kinetic energy is given by Eq. 20-27,

K=mc2

p1−v2/c2−mc2=mc21−β2−1/2−1.

We want to expand the 1 −β2part for small β,

1−β2−1/2= 1 + 1

2β2+3

8β4+···

Inserting this into the kinetic energy expression,

K=1

2mc2β2+3

8mc2β4+···

But β=v/c, so

K=1

2mv2+3

8mv4

c2+···

(b) We want to know when the error because of neglecting the second (and higher) terms is 1%;

0.01 = (3

8mv4

c2)/(1

2mv2) = 3

4v

c2.

This will happen when v/c =p(0.01)4/3) = 0.115.

E20-36 Kc= (1000 kg)(20 m/s)2/2 = 2.0×105J. The relativistic calculation is slightly harder:

Kr= (1000 kg)(3×108m/s)2(1/p1−(20 m/s)2/(3×108m/s)2−1),

≈(1000 kg) 1

2(20 m/s)2+3

8(20 m/s)4/(3×108m/s)2+...,

= 2.0×105J+6.7×10−10J.

E20-37 Start with Eq. 20-34 in the form

E2= (pc)2+ (mc2)2

The rest energy is mc2, and if the total energy is three times this then E= 3mc2, so

(3mc2)2= (pc)2+ (mc2)2,

8(mc2)2= (pc)2,

√8mc =p.

257

E20-38 The initial kinetic energy is

Ki=1

2m2v

1 + v2/c2=2mv2

(1 + v2/c2)2.

The ﬁnal kinetic energy is

Kf= 21

2mvp2−v2/c22=mv2(2 −v2/c2).

E20-39 This exercise is much more involved than the previous one!

The initial kinetic energy is

Ki=mc2

r1−2v

1+v2/c22/c2−mc2,

=mc2(1 + v2/c2)

p(1 + v2/c2)2−4v2/c2−mc2,

=m(c2+v2)

1−v2/c2−m(c2−v2)

1−v2/c2,

=2mv2

1−v2/c2.

The ﬁnal kinetic energy is

Kf= 2 mc2

r1−vp2−v2/c22/c2−2mc2,

= 2 mc2

p1−(v2/c2)(2 −v2/c2)−2mc2,

= 2 mc2

1−v2/c2−2mc2,

= 2 mc2

1−v2/c2−2m(c2−v2)

1−v2/c2,

=2mv2

1−v2/c2.

E20-40 For a particle with mass, γ=K/mc2+ 1. For the electron, γ= (0.40)/(0.511) + 1 = 1.78.

For the proton, γ= (10)/(938) + 1 = 1.066.

For the photon, pc =E. For a particle with mass, pc =p(K+mc2)2−m2c4. For the electron,

pc =p[(0.40 MeV) + (0.511 MeV)]2−(0.511 MeV)2= 0.754 MeV.

For the proton,

pc =p[(10 MeV) + (938 MeV)]2−(938 MeV)2= 137 MeV.

(a) Only photons move at the speed of light, so it is moving the fastest.

(b) The proton, since it has smallest value for γ.

(d) The photon has the least.

258

E20-41 Work is change in energy, so

W=mc2/p1−(vf/c)2−mc2/p1−(vi/c)2.

(a) Plug in the numbers,

W= (0.511 MeV)(1/p1−(0.19)2−1/p1−(0.18)2) = 0.996 keV.

(b) Plug in the numbers,

W= (0.511 MeV)(1/p1−(0.99)2−1/p1−(0.98)2) = 1.05 MeV.

E20-42 E= 2γm0c2=mc2, so

m= 2γm0= 2(1.30 mg)/p1−(0.580)2= 3.19 mg.

E20-43 (a) Energy conservation requires Ek= 2Eπ, or mkc2= 2γmπc2. Then

γ= (498 MeV)/2(140 MeV) = 1.78

This corresponds to a speed of v=cp1−1/(1.78)2= 0.827c.

(b) γ= (498 MeV + 325 MeV)/(498 MeV) = 1.65, so v=cp1−1/(1.65)2= 0.795c.

1=(0.795) + (−0.827)

1 + (0.795)(−0.827)c=−0.0934c,

and

2=(0.795) + (0.827)

1 + (0.795)(0.827)c= 0.979c,

The corresponding kinetic energies are

K1= (140 MeV)(1/p1−(−0.0934)2−1) = 0.614 MeV

and

K1= (140 MeV)(1/p1−(0.979)2−1) = 548 MeV

E20-44

P20-1 (a) γ= 2, so v=p1−1/(2)2= 0.866c.

(b) γ= 2.

P20-2 (a) Classically, v0= (0.620c) + (0.470c) = 1.09c. Relativistically,

v0=(0.620c) + (0.470c)

1 + (0.620)(0.470) = 0.844c.

(b) Classically, v0= (0.620c)+(−0.470c) = 0.150c. Relativistically,

v0=(0.620c)+(−0.470c)

1 + (0.620)(−0.470) = 0.211c.

259

P20-3 (a) γ= 1/p1−(0.247)2= 1.032.Use the equations from Table 20-2.

∆t= (1.032)[(0) −(0.247)(30.4×103m)/(3.00×108m/s)] = −2.58×10−5s.

(b) The red ﬂash appears to go ﬁrst.

P20-4 Once again, the “pico” should have been a µ.

γ= 1/p1−(0.60)2= 1.25.Use the equations from Table 20-2.

∆t= (1.25)[(4.0×10−6s) −(0.60)(3.0×103m)/(3.00×108m/s)] = −2.5×10−6s.

P20-5 We can choose our coordinate system so that uis directed along the xaxis without any

loss of generality. Then, according to Table 20-2,

∆x0=γ(∆x−u∆t),

∆y0= ∆y,

∆z0= ∆z,

c∆t0=γ(c∆t−u∆x/c).

Square these expressions,

(∆x0)2=γ2(∆x−u∆t)2=γ2(∆x)2−2u(∆x)(∆t) + (∆t)2,

(∆y0)2= (∆y)2,

(∆z0)2= (∆z)2,

c2(∆t0)2=γ2(c∆t−u∆x/c)2=γ2c2(∆t)2−2u(∆t)(∆x) + u2(∆x)2/c2.

We’ll add the ﬁrst three equations and then subtract the fourth. The left hand side is the equal to

(∆x0)2+ (∆y0)2+ (∆z0)2−c2(∆t0)2,

while the right hand side will equal

γ2(∆x)2+u2(∆t)2−c2(∆t)2−u2/c2(∆x)2+ (∆y)2+ (∆z)2,

which can be rearranged as

γ21−u2/c2(∆x)2+γ2u2−c2(∆t)2+ (∆y)2+ (∆z)2,

γ21−u2/c2(∆x)2+ (∆y)2+ (∆z)2−c2γ21−u2/c2(∆t)2.

But

γ2=1

1−u2/c2,

so the previous expression will simplify to

(∆x)2+ (∆y)2+ (∆z)2−c2(∆t)2.

P20-6 (a) vx= [(0.780c) + (0.240c)]/[1 + (0.240)(0.780)] = 0.859c.

(b) vx= [(0) + (0.240c)]/[1 + (0)] = 0.240c, while

vy= (0.780c)p1−(0.240)2/[1 + (0)] = 0.757c.

Then v=p(0.240c)2+ (0.757c)2= 0.794c.

(b) v0

x= [(0) −(0.240c)]/[1 + (0)] = −0.240c, while

y= (0.780c)p1−(0.240)2/[1 + (0)] = 0.757c.

Then v0=p(−0.240c)2+ (0.757c)2= 0.794c.

260

P20-7 If we look back at the boost equation we might notice that it looks very similar to the

rule for the tangent of the sum of two angles. It is exactly the same as the rule for the hyperbolic

tangent,

tanh(α1+α2) = tanh α1+ tanh α2

1 + tanh α1tanh α2

This means that each boost of β= 0.5 is the same as a “hyperbolic” rotation of αrwhere tanh αr=

0.5. We need only add these rotations together until we get to αf, where tanh αf= 0.999.

αf= 3.800, and αR= 0.5493. We can ﬁt (3.800)/(0.5493) = 6.92 boosts, but we need an integral

number, so there are seven boosts required. The ﬁnal speed after these seven boosts will be 0.9991c.

P20-8 (a) If ∆x0= 0, then ∆x=u∆t, or

u= (730 m)/(4.96×10−6s) = 1.472×108m/s=0.491c.

(b) γ= 1/p1−(0.491)2= 1.148,

∆t0= (1.148)[(4.96×10−6s) −(0.491)(730 m)/(3×108)] = 4.32×10−6s.

P20-9 Since the maximum value for uis c, then the minimum ∆tis

∆t≥(730 m)/(3.00×108m/s) = 2.43×10−6s.

P20-10 (a) Yes.

(b) The speed will be very close to the speed of light, consequently γ≈(23,000)/(30) = 766.7.

Then

v=p1−1/γ2≈1−1/2γ2= 1 −1/2(766.7)2= 0.99999915c.

P20-11 (a) ∆t0= (5.00 µs)p1−(0.6)2= 4.00 µs.

(b) Note: it takes time for the reading on the S0clock to be seen by the Sclock. In this case,

∆t1+ ∆t2= 5.00 µs, where ∆t1=x/u and ∆t2=x/c. Solving for ∆t1,

∆t1=(5.00 µs)/(0.6c)

1/(0.6c)+1/c = 3.125 s,

and

∆t0

1= (3.125 µs)p1−(0.6)2= 2.50 µs.

P20-12 The only change in the components of ∆roccur parallel to the boost. Then we can choose

the boost to be parallel to ∆rand then

∆r0=γ[∆r−u(0)] = γ∆r≥∆r,

since γ≥1.

P20-13 (a) Start with Eq. 20-34,

E2= (pc)2+ (mc2)2,

and substitute into this E=K+mc2,

K2+ 2Kmc2+ (mc2)2= (pc)2+ (mc2)2.

261

We can rearrange this, and then

K2+ 2Kmc2= (pc)2,

m=(pc)2−K2

2Kc2

(b) As v/c →0 we have K→1

2mv2and p→mv, the classical limits. Then the above expression

becomes

m=m2v2c2−1

4m2v4

mv2c2,

=mv2c2−1

4v4

v2c2,

=m1−1

c2

But v/c →0, so this expression reduces to m=min the classical limit, which is a good thing.

m=(121 MeV)2−(55.0 MeV)2

2(55.0 MeV)c2= 1.06 MeV/c2,

which is (1.06 MeV/c2)/(0.511 MeV/c2) = 207me. A muon.

P20-14 Since Emc2the particle is ultra-relativistic and v≈c.γ= (135)/(0.1396) = 967.

Then the particle has a lab-life of ∆t0= (967)(35.0×10−9s) = 3.385×10−5s. The distance traveled

x= (3.00×108m/s)(3.385×10−5s) = 1.016×104m,

so the pion decays 110 km above the Earth.

P20-15 (a) A completely inelastic collision means the two particles, each of mass m1, stick

together after the collision, in eﬀect becoming a new particle of mass m2. We’ll use the subscript 1

for moving particle of mass m1, the subscript 0 for the particle which is originally at rest, and the

subscript 2 for the new particle after the collision. We need to conserve momentum,

p1+p0=p2,

γ1m1u1+ (0) = γ2m2u2,

and we need to conserve total energy,

E1+E0=E2,

γ1m1c2+m1c2=γ2m2c2,

Divide the momentum equation by the energy equation and then

γ1u1

γ1+ 1 =u2.

But u1=cp1−1/γ2

1, so

u2=cγ1p1−1/γ2

γ1+ 1 ,

=cpγ2

1−1

γ1+ 1 ,

262

=cp(γ1+ 1)(γ1−1)

γ1+ 1 ,

=crγ1−1

γ1+ 1.

(b) Using the momentum equation,

m2=m1

γ1u1

γ2u2

=m1

cγ1p1−1/γ2

u2/p1−(u2/c)2,

=m1pγ2

1−1

1/p(c/u2)2−1,

=m1pγ2

1−1

1/p(γ1+ 1)/(γ1−1) −1,

=m1p(γ1+ 1)(γ1−1)

p(γ1−1)/2,

=m1p2(γ1+ 1).

P20-16 (a) K=W=RF dx =R(dp/dt)dx =R(dx/dt)dp =Rv dp.

(b) dp =mγ dv +mv(dγ/dv)dv. Now use Maple or Mathematica to save time, and get

dp =m dv

(1 −v2/c2)1/2+mv2dv

c2(1 −v2/c2)3/2.

Now integrate:

K=Zvm

(1 −v2/c2)1/2+mv2

c2(1 −v2/c2)3/2dv,

=mv2

p1−v2/c2.

P20-17 (a) Since E=K+mc2, then

Enew = 2E= 2mc2+ 2K= 2mc2(1 + K/mc2).

(b) Enew = 2(0.938 GeV) + 2(100 GeV) = 202 GeV.

P20-18 (a) Assume only one particle is formed. That particle can later decay, but it sets the

standard on energy and momentum conservation. The momentum of this one particle must equal

that of the incident proton, or

p2c2= [(mc2+K)2−m2c4].

The initial energy was K+ 2mc2, so the mass of the “one” particle is given by

M2c4= [(K+ 2mc2)2−p2c2] = 2Kmc2+ 4m2c4.

This is a measure of the available energy; the remaining energy is required to conserve momentum.

Then

Enew =√M2c4= 2mc2p1 + K/2mc2.

263

P20-19 The initial momentum is mγivi. The ﬁnal momentum is (M−m)γfvf. Manipulating the

momentum conservation equation,

mγivi= (M−m)γfvf,

mγiβi

=p1−βf2

(M−m)βf

M−m

mγiβi

=1

βf2−1,

M−m

mγiβi

+ 1 = 1

βf2,

264

E21-1 (a) We’ll assume that the new temperature scale is related to the Celsius scale by a linear

transformation; then TS=mTC+b, where mand bare constants to be determined, TSis the

temperature measurement in the “new” scale, and TCis the temperature measurement in Celsius

degrees.

One of our known points is absolute zero;

TS=mTC+b,

(0) = m(−273.15◦C) + b.

We have two other points, the melting and boiling points for water,

(TS)bp =m(100◦C) + b,

(TS)mp =m(0◦C) + b;

we can subtract the top equation from the bottom equation to get

(TS)bp −(Ts)Smp = 100 C◦m.

We are told this is 180 S◦, so m= 1.8 S◦/C◦. Put this into the ﬁrst equation and then ﬁnd b,

b= 273.15◦Cm= 491.67◦S.The conversion is then

TS= (1.8 S◦/C◦)TC+ (491.67◦S).

(b) The melting point for water is 491.67◦S; the boiling point for water is 180 S◦above this, or

671.67◦S.

E21-2 TF= 9(−273.15 deg C)/5 + 32◦F = −459.67◦F.

E21-3 (a) We’ll assume that the new temperature scale is related to the Celsius scale by a linear

transformation; then TS=mTC+b, where mand bare constants to be determined, TSis the

temperature measurement in the “new” scale, and TCis the temperature measurement in Celsius

degrees.

One of our known points is absolute zero;

TS=mTC+b,

(0) = m(−273.15◦C) + b.

We have two other points, the melting and boiling points for water,

(TS)bp =m(100◦C) + b,

(TS)mp =m(0◦C) + b;

we can subtract the top equation from the bottom equation to get

(TS)bp −(Ts)Smp = 100 C◦m.

We are told this is 100 Q◦, so m= 1.0 Q◦/C◦. Put this into the ﬁrst equation and then ﬁnd b,

b= 273.15◦C = 273.15◦Q.The conversion is then

TS=TC+ (273.15◦S).

(b) The melting point for water is 273.15◦Q; the boiling point for water is 100 Q◦above this, or

373.15◦Q.

265

E21-4 (a) T= (9/5)(6000 K −273.15) + 32 = 10000◦F.

(b) T= (5/9)(98.6◦F−32) = 37.0◦C.

(d) T= (9/5)(−183◦C) + 32 = −297◦F.

(e) It depends on what you think is hot. My mom thinks 79◦F is too warm; that’s T=

(5/9)(79◦F−32) = 26◦C.

E21-5 T= (9/5)(310 K −273.15) + 32 = 98.3◦F, which is ﬁne.

E21-6 (a) T= 2(5/9)(T−32), so −T/10 = −32, or T= 320◦F.

(b) 2T= (5/9)(T−32), so 13T/5 = −32, or T=−12.3◦F.

E21-7 If the temperature (in Kelvin) is directly proportional to the resistance then T=kR,

where kis a constant of proportionality. We are given one point, T= 273.16 K when R= 90.35 Ω,

but that is okay; we only have one unknown, k. Then (273.16 K) = k(90.35 Ω) or k= 3.023 K/Ω.

If the resistance is measured to be R= 96.28 Ω, we have a temperature of

T=kR = (3.023 K/Ω)(96.28 Ω) = 291.1 K.

E21-8 T= (510◦C)/(0.028 V)V, so T= (1.82×104◦C/V)(0.0102 V) = 186◦C.

E21-9 We must ﬁrst ﬁnd the equation which relates gain to temperature, and then ﬁnd the gain

at the speciﬁed temperature. If we let Gbe the gain we can write this linear relationship as

G=mT +b,

where mand bare constants to be determined. We have two known points:

(30.0) = m(20.0◦C) + b,

(35.2) = m(55.0◦C) + b.

If we subtract the top equation from the bottom we get 5.2 = m(35.0◦C),or m= 1.49 C−1. Put

this into either of the ﬁrst two equations and

(30.0) = (0.149 C−1)(20.0◦C) + b,

which has a solution b= 27.0

Now to ﬁnd the gain when T= 28.0◦C:

G=mT +b= (0.149 C−1)(28.0◦C) + (27.0) = 31.2

E21-10 p/ptr = (373.15 K)/(273.16 K) = 1.366.

E21-11 100 cm Hg is 1000 torr. PHe = (100 cm Hg)(373 K)/(273.16 K) = 136.550 cm Hg. Ni-

trogen records a temperature which is 0.2 K higher, so PN= (100 cm Hg)(373.2 K)/(273.16 K) =

136.623 cm Hg. The diﬀerence is 0.073 cm Hg.

E21-12 ∆L= (23×10−6/C◦)(33 m)(15C◦) = 1.1×10−2m.

E21-13 ∆L= (3.2×10−6/C◦)(200 in)(60C◦) = 3.8×10−2in.

266

E21-14 L0= (2.725cm)[1 + (23×10−6/C◦)(128C◦)] = 2.733 cm.

E21-15 We want to focus on the temperature change, not the absolute temperature. In this

case, ∆T=Tf−Ti= (42◦C) −(−5.0◦C) = 47 C◦.

Then

∆L= (11 ×10−6C−1)(12.0 m)(47 C◦) = 6.2×10−3m.

E21-16 ∆A= 2αA∆T, so

∆A= 2(9×10−6/C◦)(2.0 m)(3.0 m)(30C◦) = 3.2×10−3m2.

E21-17 (a) We’ll apply Eq. 21-10. The surface area of a cube is six times the area of one face,

which is the edge length squared. So A= 6(0.332 m)2= 0.661 m2.The temperature change is

∆T= (75.0◦C) −(20.0◦C) = 55.0 C◦. Then the increase in surface area is

∆A= 2αA∆T= 2(19 ×10−6C−1)(0.661 m2)(55.0 C◦) = 1.38 ×10−3m2

(b) We’ll now apply Eq. 21-11. The volume of the cube is the edge length cubed, so

V= (0.332 m)3= 0.0366 m3.

and then from Eq. 21-11,

∆V= 2αV ∆T= 3(19 ×10−6C−1)(0.0366 m3)(55.0 C◦) = 1.15 ×10−4m3,

is the change in volume of the cube.

E21-18 V0=V(1 + 3α∆T), so

V0= (530 cm3)[1 + 3(29×10−6/C◦)(−172 C◦)] = 522 cm3.

E21-19 (a) The slope is approximately 1.6×10−4/C◦.

(b) The slope is zero.

E21-20 ∆r= (β/3)r∆T, so

∆r= [(3.2×10−5/K)/3](6.37×106m)(2700 K) = 1.8×105m.

E21-21 We’ll assume that the steel ruler measures length correctly at room temperature. Then

the 20.05 cm measurement of the rod is correct. But both the rod and the ruler will expand in the

oven, so the 20.11 cm measurement of the rod is not the actual length of the rod in the oven. What

is the actual length of the rod in the oven? We can only answer that after ﬁguring out how the 20.11

cm mark on the ruler moves when the ruler expands.

Let L= 20.11 cm correspond to the ruler mark at room temperature. Then

∆L=αsteelL∆T= (11 ×10−6C−1)(20.11 cm)(250 C◦) = 5.5×10−2cm

is the shift in position of the mark as the ruler is raised to the higher temperature. Then the change

in length of the rod is not (20.11 cm) −(20.05 cm) = 0.06 cm, because the 20.11 cm mark is shifted

out. We need to add 0.055 cm to this; the rod changed length by 0.115 cm.

The coeﬃcient of thermal expansion for the rod is

α=∆L

L∆T=(0.115 cm)

(20.05 cm)(250 C◦)= 23 ×10−6C−1.

267

E21-22 A=ab,A0= (a+ ∆a)(b+ ∆b) = ab +a∆b+b∆a+ ∆a∆b, so

∆A=a∆b+b∆a+ ∆a∆b,

=A(∆b/b + ∆a/a + ∆a∆b/ab),

≈A(α∆T+α∆T),

= 2αA∆T.

E21-23 Solve this problem by assuming the solid is in the form of a cube.

If the length of one side of a cube is originally L0, then the volume is originally V0=L3

0. After

heating, the volume of the cube will be V=L3, where L=L0+ ∆L.

Then

V=L3,

= (L0+ ∆L)3,

= (L0+αL0∆T)3,

=L3

0(1 + α∆T)3.

As long as the quantity α∆Tis much less than one we can expand the last line in a binomial

expansion as

V≈V0(1 + 3α∆T+···),

so the change in volume is ∆V≈3αV0∆T.

E21-24 (a) ∆A/A = 2(0.18%) = (0.36%).

(b) ∆L/L = 0.18%.

(d) Zero.

(e) α= (0.0018)/(100 C◦) = 1.8×10−5/C◦.

E21-25 ρ0−ρ=m/V 0−m/V =m/(V+ ∆V)−m/V ≈ −m∆V/V 2.Then

∆ρ=−(m/V )(∆V/V ) = −ρβ∆T.

E21-26 Use the results of Exercise 21-25.

(a) ∆V/V = 3∆L/L = 3(0.092%) = 0.276%. The change in density is

∆ρ/ρ =−∆V/V =−(0.276%) = −0.28

(b) α=β/3 = (0.28%)/3(40 C◦) = 2.3×10−5/C◦.Must be aluminum.

E21-27 The diameter of the rod as a function of temperature is

ds=ds,0(1 + αs∆T),

The diameter of the ring as a function of temperature is

db=db,0(1 + αb∆T).

268

We are interested in the temperature when the diameters are equal,

ds,0(1 + αs∆T) = db,0(1 + αb∆T),

αsds,0∆T−αbdb,0∆T=db,0−ds,0,

∆T=db,0−ds,0

αsds,0−αbdb,0

∆T=(2.992 cm) −(3.000 cm)

(11×10−6/C◦)(3.000 cm) −(19×10−6/C◦)(2.992 cm) ,

= 335 C◦.

The ﬁnal temperature is then Tf= (25◦) + 335 C◦= 360◦.

E21-28 (a) ∆L= ∆L1+ ∆L2= (L1α1+L2α2)∆T. The eﬀective value for αis then

α=∆L

L∆T=α1L1+α2L2

(b) Since L2=L−L1we can write

α1L1+α2(L−L1) = αL,

L1=Lα−α2

α1−α2

= (0.524 m)(13×10−6)−(11×10−6)

(19×10−6)−(11×10−6)= 0.131 m.

The brass length is then 13.1 cm and the steel is 39.3 cm.

E21-29 At 100◦C the glass and mercury each have a volume V0. After cooling, the diﬀerence in

volume changes is given by

∆V=V0(3αg−βm)∆T.

Since m=ρV , the mass of mercury that needs to be added can be found by multiplying though by

the density of mercury. Then

∆m= (0.891 kg)[3(9.0×10−6/C◦)−(1.8×10−4/C◦)](−135C◦) = 0.0184 kg.

This is the additional amount required, so the total is now 909 g.

E21-30 (a) The rotational inertia is given by I=Rr2dm; changing the temperature requires

r→r0=r+ ∆r=r(1 + α∆T). Then

I0=Z(1 + α∆T)2r2dm ≈(1 + 2α∆T)Zr2dm,

so ∆I= 2αI∆T.

(b) Since L=Iω, then 0 = ω∆I+I∆ω. Rearranging, ∆ω/ω =−∆I/I =−2α∆T. Then

∆ω=−2(19×10−6/C◦)(230 rev/s)(170 C◦) = −1.5 rev/s.

269

E21-31 This problem is related to objects which expand when heated, but we never actually need

to calculate any temperature changes. We will, however, be interested in the change in rotational

inertia. Rotational inertia is directly proportional to the square of the (appropriate) linear dimension,

If/Ii= (rf/ri)2.

(a) If the bearings are frictionless then there are no external torques, so the angular momentum

is constant.

(b) If the angular momentum is constant, then

Li=Lf,

Iiωi=Ifωf.

We are interested in the percent change in the angular velocity, which is

ωf−ωi

ωi

=ωf

ωi−1 = Ii

If−1 = ri

rf2

−1 = 1

1.00182

−1 = −0.36%.

Kf−Ki

=ωf−ωi

ωi

=−0.36%.

E21-32 (a) The period of a physical pendulum is given by Eq. 17-28. There are two variables

in the equation that depend on length. I, which is proportional to a length squared, and d, which

is proportional to a length. This means that the period have an overall dependence on length

proportional to √r. Taking the derivative,

∆P≈dP =1

rdr ≈1

2P α∆T.

(b) ∆P/P = (0.7×10−6C◦)(10C◦)/2 = 3.5×10−6.After 30 days the clock will be slow by

∆t= (30 ×24 ×60 ×60 s)(3.5×10−6) = 9.07 s.

E21-33 Refer to the Exercise 21-32.

∆P= (3600 s)(19×10−6C◦)(−20C◦)/2 = 0.68 s.

E21-34 At 22◦C the aluminum cup and glycerin each have a volume V0. After heating, the

diﬀerence in volume changes is given by

∆V=V0(3αa−βg)∆T.

The amount that spills out is then

∆V= (110 cm3)[3(23×10−6/C◦)−(5.1×10−4/C◦)](6C◦) = −0.29 cm3.

E21-35 At 20.0◦C the glass tube is ﬁlled with liquid to a volume V0. After heating, the diﬀerence

in volume changes is given by

∆V=V0(3αg−βl)∆T.

The cross sectional area of the tube changes according to

∆A=A02αg∆T.

270

Consequently, the height of the liquid changes according to

∆V= (h0+ ∆h)(A0+ ∆A)−h0A,

≈h0∆A+A0∆h,

∆V/V0= ∆A/A0+ ∆h/h0.

Then

∆h= (1.28 m/2)[(1.1×10−5/C◦)−(4.2×10−5/C◦)](13 C◦) = 2.6×10−4m.

E21-36 (a) β= (dV/dT )/V . If pV =nRT , then p dV =nR dT , so

β= (nR/p)/V =nR/pV = 1/T.

(b) Kelvins.

E21-37 (a) V= (1 mol)(8.31 J/mol ·K)(273 K)/(1.01×105Pa) = 2.25×10−2m3.

(b) (6.02×1023mol−1)/(2.25×104/cm3) = 2.68×1019.

E21-38 n/V =p/kT , so

n/V = (1.01×10−13Pa)/(1.38×10−23J/K)(295 K) = 25 part/cm3.

E21-39 (a) Using Eq. 21-17,

n=pV

RT =(108×103Pa)(2.47 m3)

(8.31 J/mol·K)([12 + 273] K) = 113 mol.

(b) Use the same expression again,

V=nRT

p=(113 mol)(8.31 J/mol·K)([31 + 273] K)

(316×103Pa) = 0.903 m3.

E21-40 (a) n=pV/RT = (1.01×105Pa)(1.13×10−3m3)/(8.31 J/mol·K)(315 K) = 4.36×10−2mol.

(b) Tf=TipfVf/piVi, so

Tf=(315 K)(1.06×105Pa)(1.530×10−3m3)

(1.01×105Pa)(1.130×10−3m3)= 448 K.

E21-41 pi= (14.7 + 24.2) lb/in2= 38.9 lb/in2.pf=piTfVi/T iVf, so

pf=(38.9 lb/in2)(299K)(988 in3)

(270 K)(1020 in3)= 41.7 lb/in2.

The gauge pressure is then (41.7−14.7) lb/in2= 27.0 lb/in2.

E21-42 Since p=F/A and F=mg, a reasonable estimate for the mass of the atmosphere is

m=pA/g = (1.01×105Pa)4π(6.37×106m)2/(9.81 m/s2) = 5.25×1018kg.

271

E21-43 p=p0+ρgh, where his the depth. Then Pf= 1.01×105Pa and

pi= (1.01×105Pa) + (998 kg/m3)(9.81 m/s2)(41.5 m) = 5.07×105Pa.

Vf=VipiTf/pfTi, so

Vf=(19.4 cm3)(5.07×105Pa.)(296 K)

(1.01×105Pa)(277 K) = 104 cm3.

E21-44 The new pressure in the pipe is

pf=piVi/V f= (1.01×105Pa)(2) = 2.02×105Pa.

The water pressure at some depth yis given by p=p0+ρgy, so

y=(2.02×105Pa) −(1.01×105Pa)

(998 kg/m3)(9.81 m/s2)= 10.3 m.

Then the water/air interface inside the tube is at a depth of 10.3 m; so h= (10.3 m) + (25.0 m)/2 =

22.8 m.

P21-1 (a) The dimensions of Amust be [time]−1, as can be seen with a quick inspection of the

equation. We would expect that Awould depend on the surface area at the very least; however,

that means that it must also depend on some other factor to ﬁx the dimensionality of A.

(b) Rearrange and integrate,

∆T0

d∆T

∆T=−Zt

A dt,

ln(∆T/∆T0) = −At,

∆T= ∆T0e−At.

P21-2 First ﬁnd A.

A=ln(∆T0/∆T)

t=ln[(29 C◦)/(25 C◦)]

(45 min) = 3.30×10−3/min.

Then ﬁnd time to new temperature diﬀerence.

t=ln(∆T0/∆T)

t=ln[(29 C◦)/(21 C◦)]

(3.30×10−3/min) = 97.8min

This happens 97.8−45 = 53 minutes later.

P21-3 If we neglect the expansion of the tube then we can assume the cross sectional area of the

tube is constant. Since V=Ah, we can assume that ∆V=A∆h. Then since ∆V=βV0∆T, we

can write ∆h=βh0∆T.

P21-4 For either container we can write piVi=niRTi.We are told that Viand niare constants.

Then ∆p=AT1−BT2,where Aand Bare constants. When T1=T2∆p= 0, so A=B. When

T1=Ttr and T2=Tbwe have

(120 mm Hg) = A(373 K −273.16 K),

so A= 1.202 mm Hg/K. Then

T=(90 mm Hg) + (1.202 mm Hg/K)(273.16 K)

(1.202 mm Hg/K) = 348 K.

Actually, we could have assumed Awas negative, and then the answer would be 198 K.

272

P21-5 Start with a diﬀerential form for Eq. 21-8, dL/dT =αL0, rearrange, and integrate:

dL =ZT

αL0dT,

L−L0=L0ZT

α dT,

L=L0 1 + ZT

α dT !.

P21-6 ∆L=αL∆T, so

∆T

∆t=1

αL

∆L

∆t=(96×10−9m/s)

(23×10−6/C◦)(1.8×10−2m) = 0.23◦C/s.

P21-7 (a) Consider the work that was done for Ex. 21-27. The length of rod ais

La=La,0(1 + αa∆T),

while the length of rod bis

Lb=Lb,0(1 + αb∆T).

The diﬀerence is

La−Lb=La,0(1 + αa∆T)−Lb,0(1 + αb∆T),

=La,0−Lb,0+ (La,0αa−Lb,0αb)∆T,

which will be a constant is La,0αa=Lb,0αbor

Li,0∝1/αi.

(b) We want La,0−Lb,0= 0.30 m so

k/αa−k/αb= 0.30 m,

where kis a constant of proportionality;

k= (0.30 m)/1/(11×10−6/C◦)−1/(19×10−6/C◦)= 7.84×10−6m/C◦.

The two lengths are

La= (7.84×10−6m/C◦)/(11×10−6/C◦) = 0.713 m

for steel and

Lb= (7.84×10−6m/C◦)/(19×10−6/C◦) = 0.413 m

for brass.

P21-8 The fractional increase in length of the bar is ∆L/L0=α∆T. The right triangle on the left

has base L0/2, height x, and hypotenuse (L0+ ∆L)/2. Then

x=1

2q(L0+ ∆L)2−L2

0=L0

2r2∆L

With numbers,

x=(3.77 m)

2p2(25×10−6/C◦)(32 C◦) = 7.54×10−2m.

273

P21-9 We want to evaluate V=V0(1 + Rβ dT ); the integral is the area under the graph; the

graph looks like a triangle, so the result is

V=V0[1 + (16 C◦)(0.0002/C◦)/2] = (1.0016)V0.

The density is then

ρ=ρ0(V0/V ) = (1000 kg/m3)/(1.0016) = 0.9984 kg/m3.

P21-10 At 0.00◦C the glass bulb is ﬁlled with mercury to a volume V0. After heating, the diﬀerence

in volume changes is given by

∆V=V0(β−3α)∆T.

Since T0= 0.0◦C, then ∆T=T, if it is measured in ◦C. The amount of mercury in the capillary is

∆V, and since the cross sectional area is ﬁxed at A, then the length is L= ∆V/A, or

L=V

A(β−3α)∆T.

P21-11 Let a,b, and ccorrespond to aluminum, steel, and invar, respectively. Then

cos C=a2+b2−c2

2ab .

We can replace awith a0(1 + αa∆T), and write similar expressions for band c. Since a0=b0=c0,

this can be simpliﬁed to

cos C=(1 + αa∆T)2+ (1 + αb∆T)2−(1 + αc∆T)2

2(1 + αa∆T)(1 + αb∆T).

Expand this as a Taylor series in terms of ∆T, and we ﬁnd

cos C≈1

2+1

2(αa+αb−2αc) ∆T.

Now solve:

∆T=2 cos(59.95◦)−1

(23×10−6/C◦) + (11×10−6/C◦)−2(0.7×10−6/C◦)= 46.4◦C.

The ﬁnal temperature is then 66.4◦C.

P21-12 The bottom of the iron bar moves downward according to ∆L=αL∆T. The center of

mass of the iron bar is located in the center; it moves downward half the distance. The mercury

expands in the glass upwards; subtracting oﬀ the distance the iron moves we get

∆h=βh∆T−∆L= (βh −αL)∆T.

The center of mass in the mercury is located in the center. If the center of mass of the system is to

remain constant we require

mi∆L/2 = mm(∆h−∆L)/2;

or, since ρ=mV =mAy,

ρiαL =ρm(βh −2αL).

Solving for h,

h=(12×10−6/C◦)(1.00 m)[(7.87×103kg/m3) + 2(13.6×103kg/m3)]

(13.6×103kg/m3)(18×10−5/C◦)= 0.17 m.

274

P21-13 The volume of the block which is beneath the surface of the mercury displaces a mass

of mercury equal to the mass of the block. The mass of the block is independent of the temperature

but the volume of the displaced mercury changes according to

Vm=Vm,0(1 + βm∆T).

This volume is equal to the depth which the block sinks times the cross sectional area of the block

(which does change with temperature). Then

hshb2=hs,0hb,02(1 + βm∆T),

where hsis the depth to which the block sinks and hb,0= 20 cm is the length of the side of the

block. But

hb=hb,0(1 + αb∆T),

hs=hs,0

1 + βm∆T

(1 + αb∆T)2.

Since the changes are small we can expand the right hand side using the binomial expansion; keeping

terms only in ∆Twe get

hs≈hs,0(1 + (βm−2αb)∆T),

which means the block will sink a distance hs−hs,0given by

hs,0(βm−2αb)∆T=hs,0(1.8×10−4/C◦)−2(23×10−6/C◦)(50 C◦) = (6.7×10−3)hs,0.

In order to ﬁnish we need to know how much of the block was submerged in the ﬁrst place. Since

the fraction submerged is equal to the ratio of the densities, we have

hs,0/hb,0=ρb/ρm= (2.7×103kg/m3)/(1.36×104kg/m3),

so hs,0= 3.97 cm, and the change in depth is 0.27 mm.

P21-14 The area of glass expands according to ∆Ag= 2αgAg∆T. The are of Dumet wire expands

according to

∆Ac+ ∆Ai = 2(αcAc+αiAi)∆T.

We need these to be equal, so

αgAg=αcAc+αiAi,

αgrg2=αc(rc2−ri2) + αiri2,

αg(rc2+ri2) = αc(rc2−ri2) + αiri2,

ri2

rc2=αc−αg

αc−αi

P21-15

P21-16 V2=V1(p1/p2)(T1/T2),so

V2= (3.47 m3)[(76 cm Hg)/(36 cm Hg)][(225 K)/(295 K)] = 5.59 m3.

275

P21-17 Call the containers one and two so that V1= 1.22 L and V2= 3.18 L. Then the initial

number of moles in the two containers are

n1,i=piV1

RT i

and n2,i=piV2

RT i

The total is

n=pi(V1+V2)/(RT i).

Later the temperatures are changed and then the number of moles of gas in each container is

n1,f=pfV1

RT 1,f

and n2,f=pfV2

RT 2,f

The total is still n, so

RV1

T1,f

+V2

T2,f=pi(V1+V2)

RT i

We can solve this for the ﬁnal pressure, so long as we remember to convert all temperatures to

Kelvins,

pf=pi(V1+V2)

TiV1

T1,f

+V2

T2,f−1

pf=(1.44 atm)(1.22L + 3.18 L)

(289 K) (1.22 L)

(289 K) +(3.18 L)

(381 K) −1

= 1.74 atm.

P21-18 Originally nA=pAVA/RTAand nB=pBVB/RTB;VB= 4VA. Label the ﬁnal state of

Aas Cand the ﬁnal state of Bas D. After mixing, nC=pCVA/RTAand nD=pDVB/RTB, but

PC=PDand nA+nB=nC+nD. Then

pA/TA+ 4pB/TB=pC(1/TA+ 4/TB),

pC=(5×105Pa)/(300 K) + 4(1×105Pa)/(400 K)

1/(300 K) + 4/(400 K)= 2.00×105Pa.

P21-19 If the temperature is uniform then all that is necessary is to substitute p0=nRT/V and

p=nRT/V ; cancel RT from both sides, and then equate n/V with nV.

P21-20 Use the results of Problem 15-19. The initial pressure inside the bubble is pi=p0+ 4γ/ri.

The ﬁnal pressure inside the bell jar is zero, sopf= 4γ/rf. The initial and ﬁnal pressure inside the

bubble are related by piri3=pfrf3= 4γrf2. Now for numbers:

pi= (1.01×105Pa) + 4(2.5×10−2N/m)/(2.0×10−3m) = 1.0105×105Pa.

and

rf=s(1.0105×105Pa)(2.0×10−3m)3

4(2.5×10−2N/m) = 8.99×10−2m.

P21-21

P21-22

276

E22-1 (a) n= (2.56 g)/(197 g/mol) = 1.30×10−2mol.

(b) N= (6.02×1023mol−1)(1.30×10−2mol) = 7.83×1021.

E22-2 (a) N=pV/kT = (1.01×105Pa)(1.00 m3)/(1.38×10−23J/K)(293 K) = 2.50×1025.

(b) n= (2.50×1025)/(6.02×1023mol−1) = 41.5 mol.Then

m= (41.5 mol)[75%(28 g/mol) + 25%(32 g/mol)] = 1.20 kg.

E22-3 (a) We ﬁrst need to calculate the molar mass of ammonia. This is

M=M(N) + 3M(H) = (14.0 g/mol) + 3(1.01 g/mol) = 17.0 g/mol

The number of moles of nitrogen present is

n=m/M r= (315 g)/(17.0 g/mol) = 18.5 mol.

The volume of the tank is

V=nRT/p = (18.5 mol)(8.31 J/mol ·K)(350 K)/(1.35×106Pa) = 3.99×10−2m3.

(b) After the tank is checked the number of moles of gas in the tank is

n=pV/(RT ) = (8.68×105Pa)(3.99×10−2m3)/[(8.31 J/mol ·K)(295 K)] = 14.1 mol.

In that case, 4.4 mol must have escaped; that corresponds to a mass of

m=nMr= (4.4 mol)(17.0 g/mol) = 74.8 g.

E22-4 (a) The volume per particle is V/N =kT/P , so

V/N = (1.38×10−23J/K)(285 K)/(1.01×105Pa) = 3.89×10−26m3.

The edge length is the cube root of this, or 3.39×10−9m. The ratio is 11.3.

(b) The volume per particle is V/NA, so

V/NA= (18×10−6m3)/(6.02×1023) = 2.99×10−29m3.

The edge length is the cube root of this, or 3.10×10−10m. The ratio is 1.03.

E22-5 The volume per particle is V/N =kT /P , so

V/N = (1.38×10−23J/K)(308 K)/(1.22)(1.01×105Pa) = 3.45×10−26m3.

The fraction actually occupied by the particle is

4π(0.710×10−10m)3/3)

(3.45×10−26m3)= 4.34×10−5.

E22-6 The component of the momentum normal to the wall is

py= (3.3×10−27kg)(1.0×103m/s) cos(55◦) = 1.89×10−24kg ·m/s.

The pressure exerted on the wall is

p=F

A=(1.6×1023/s)2(1.89×10−24kg ·m/s)

(2.0×10−4m2)= 3.0×103Pa.

277

E22-7 (a) From Eq. 22-9,

vrms =r3p

ρ.

Then

p= 1.23 ×10−3atm 1.01 ×105Pa

1 atm = 124 Pa

and

ρ= 1.32 ×10−5g/cm31kg

1000 g100 cm

1 m 3

= 1.32 ×10−2kg/m3.

Finally,

vrms =s3(1240 Pa)

(1.32 ×10−2kg/m3)= 531 m/s.

(b) The molar density of the gas is just n/V ; but this can be found quickly from the ideal gas

law as n

V=p

RT =(1240 Pa)

(8.31 J/mol ·K)(317 K) = 4.71 ×10−1mol/m3.

n/V =(1.32 ×10−2kg/m3)

(4.71 ×10−1mol/m3)= 28.0 g/mol.

But what gas is it? It could contain any atom lighter than silicon; trial and error is the way to go.

Some of my guesses include C2H4(ethene), CO (carbon monoxide), and N2. There’s no way to tell

which is correct at this point, in fact, the gas could be a mixture of all three.

E22-8 The density is ρ=m/V =nMr/V , or

ρ= (0.350 mol)(0.0280 kg/mol)/π(0.125 m/2)2(0.560 m) = 1.43 kg/m3.

The rms speed is

vrms =s3(2.05)(1.01×105Pa)

(1.43 kg/m3)= 659 m/s.

E22-9 (a) N/V =p/kT = (1.01×105Pa)/(1.38×10−23J/K)(273 K) = 2.68×1025/m3.

(b) Note that Eq. 22-11 is wrong; for the explanation read the last two paragraphs in the ﬁrst

column on page 502. We need an extra factor of √2, so πd2=V/√2Nλ, so

d=q1/√2π(2.68×1025/m3)(285×10−9m) = 1.72×10−10m.

E22-10 (a) λ=V/√2Nπd2, so

λ=1

√2(1.0×106/m3)π(2.0×10−10m)2= 5.6×1012m.

(b) Particles eﬀectively follow ballistic trajectories.

278

E22-11 We have v=fλ, where λis the wavelength (which we will set equal to the mean free

path), and vis the speed of sound. The mean free path is, from Eq. 22-13,

λ=kT

√2πd2p

f=√2πd2pv

kT =√2π(315×10−12m)2(1.02 ×1.01×105Pa)(343 m/s)

(1.38×10−23J/K)(291 K) = 3.88×109Hz.

E22-12 (a) p= (1.10×10−6mm Hg)(133 Pa/mm Hg) = 1.46×10−4Pa. The particle density is

N/V = (1.46×10−4Pa)/(1.38×10−23J/K)(295 K) = 3.59×1016/m3.

(b) The mean free path is

λ= 1/p(2)(3.59×1016/m3)π(2.20×10−10m)2= 130 m.

E22-13 Note that vav ∝√T, while λ∝T. Then the collision rate is proportional to 1/√T. Then

T= (300 K)(5.1×109/s)2

(6.0×109/s)2= 216 K.

E22-14 (a) vav = (65 km/s)/(10) = 6.5 km/s.

(b) vrms =p(505 km/s)/(10) = 7.1 km/s.

E22-15 (a) The average is

4(200 s) + 2(500 m/s) + 4(600 m/s)

4+2+4 = 420 m/s.

The mean-square value is

4(200 s)2+ 2(500 m/s)2+ 4(600 m/s)2

4+2+4 = 2.1×105m2/s2.

The root-mean-square value is the square root of this, or 458 m/s.

(b) I’ll be lazy. Nine particles are not moving, and the tenth has a speed of 10 m/s. Then the

average speed is 1 m/s, and the root-mean-square speed is 3.16 m/s. Look, vrms is larger than vav!

reference where vav = 0, then some of the individual speeds must be greater than zero, and some

will be less than zero. Squaring these speeds will result in positive, non-zero, numbers; the mean

square will necessarily be greater than zero, so vrms >0.

Only if all of the particles have the same speed will vrms=vav.

E22-16 Use Eq. 22-20:

vrms =s3(1.38×10−23J/K)(329 K)

(2.33×10−26kg + 3 ×1.67×10−27kg) = 694 m/s.

E22-17 Use Eq. 22-20:

vrms =s3(1.38×10−23J/K)(2.7 K)

(2 ×1.67×10−27kg) = 180 m/s.

279

E22-18 Eq. 22-14 is in the form N=Av2e−Bv2. Taking the derivative,

dv = 2Ave−Bv2−2ABv3e−Bv2,

and setting this equal to zero,

v2= 1/B = 2kT/m.

E22-19 We want to integrate

vav =1

NZ∞

N(v)v dv,

NZ∞

4πN m

2πkT 3/2v2e−mv2/2kT v dv,

= 4πm

2πkT 3/2Z∞

v2e−mv2/2kT v dv.

The easiest way to attack this is ﬁrst with a change of variables— let x=mv2/2kT , then kT dx =

mv dv. The limits of integration don’t change, since √∞=∞. Then

vav = 4πm

2πkT 3/2Z∞

2kT

mxe−xkT

mdx,

= 2 2kT

πm 1/2Z∞

xe−αxdx

The factor of αthat was introduced in the last line is a Feynman trick; we’ll set it equal to one when

we are ﬁnished, so it won’t change the result.

Feynman’s trick looks like

dα Ze−αxdx =Z∂

∂α e−αxdx =Z(−x)e−αxdx.

Applying this to our original problem,

vav = 2 2kT

πm 1/2Z∞

xe−αxdx,

=−d

dα22kT

πm 1/2Z∞

e−αxdx,

=−22kT

πm 1/2d

dα −1

αe−αx

∞

0,

=−22kT

πm 1/2d

dα 1

α,

=−22kT

πm 1/2−1

α2.

We promised, however, that we would set α= 1 in the end, so this last line is

vav = 2 2kT

πm 1/2

=r8kT

πm .

280

E22-20 We want to integrate

(v2)av =1

NZ∞

N(v)v2dv,

NZ∞

4πN m

2πkT 3/2v2e−mv2/2kT v2dv,

= 4πm

2πkT 3/2Z∞

v2e−mv2/2kT v2dv.

The easiest way to attack this is ﬁrst with a change of variables— let x2=mv2/2kT , then

p2kT/mdx =dv. The limits of integration don’t change. Then

(v2)av = 4πm

2πkT 3/2Z∞

02kT

m5/2

x4e−x2dx,

=8kT

√π m Z∞

x4e−x2dx

Look up the integral; although you can solve it by ﬁrst applying a Feynman trick (see solution to

Exercise 22-21) and then squaring the integral and changing to polar coordinates. I looked it up. I

found 3√π/8, so

(v2)av =8kT

√π m3√π/8 = 3kT/m.

E22-21 Apply Eq. 22-20:

vrms =p3(1.38×10−23J/K)(287 K)/(5.2×10−17kg) = 1.5×10−2m/s.

E22-22 Since vrms ∝pT/m, we have

T= (299 K)(4/2) = 598 K,

or 325◦C.

E22-23 (a) The escape speed is found on page 310; v= 11.2×103m/s. For hydrogen,

T= (2)(1.67×10−27kg)(11.2×103m/s)2/3(1.38×10−23J/K) = 1.0×104K.

For oxygen,

T= (32)(1.67×10−27kg)(11.2×103m/s)2/3(1.38×10−23J/K) = 1.6×105K.

(b) The escape speed is found on page 310; v= 2.38×103m/s. For hydrogen,

T= (2)(1.67×10−27kg)(2.38×103m/s)2/3(1.38×10−23J/K) = 460K.

For oxygen,

T= (32)(1.67×10−27kg)(2.38×103m/s)2/3(1.38×10−23J/K) = 7300K.

E22-24 (a) vav = (70 km/s)/(22) = 3.18 km/s.

(b) vrms =q(250 km2/s2)/(22) = 3.37 km/s.

281

E22-25 According to the equation directly beneath Fig. 22-8,

ω=vφ/L = (212 m/s)(0.0841 rad)/(0.204 m) = 87.3 rad/s.

E22-26 If vp=vrms then 2T2= 3T1, or T2/T1= 3/2.

E22-27 Read the last paragraph on the ﬁrst column of page 505. The distribution of speeds is

proportional to

v3e−mv2/2kT =v3e−Bv2,

taking the derivative dN/dv and setting equal to zero yields

dv = 3v2e−Bv2−2Bv4e−Bv2,

and setting this equal to zero,

v2= 3/2B= 3kT/m.

E22-28 (a) v=p3(8.31 J/mol ·K)(4220 K)/(0.07261 kg/mol) = 1200 m/s.

(b) Half of the sum of the diameters, or 273 pm.

λ= 1/√2(4.13×1025/m3)π(273×10−12m)2= 7.31×10−8m.

The collision rate is

(1200 m/s)/(7.31×10−8m) = 1.64×1010/s.

E22-29 The fraction of particles that interests us is

√π

(kT )3/2Z0.03kT

0.01kT

E1/2e−E/kT dE.

Change variables according to E/kT =x, so that dE =kT dx. The integral is then

√πZ0.03

0.01

x1/2e−xdx.

Since the value of xis so small compared to 1 throughout the range of integration, we can expand

according to

e−x≈1−xfor x1.

The integral then simpliﬁes to

√πZ0.03

0.01

x1/2(1 −x)dx =2

√π2

3x3/2−2

5x5/20.03

0.01

= 3.09×10−3.

E22-30 Write N(E) = N(Ep+). Then

N(Ep+)≈N(Ep) + dN(E)

dE Ep

+...

But the very deﬁnition of Epimplies that the ﬁrst derivative is zero. Then the fraction of [particles

with energies in the range Ep±0.02kT is

√π

(kT )3/2(kT /2)1/2e−1/2(0.02kT ),

or 0.04p1/2eπ = 9.68×10−3.

282

E22-31 The volume correction is on page 508; we need ﬁrst to ﬁnd d. If we assume that the

particles in water are arranged in a cubic lattice (a bad guess, but we’ll use it anyway), then 18

grams of water has a volume of 18×10−6m3, and

d3=(18×10−6m3)

(6.02×1023)= 3.0×10−29m3

is the volume allocated to each water molecule. In this case d= 3.1×10−10m. Then

b=1

2(6.02×1023)( 4

3π(3.1×10−10m)3) = 3.8 m3/mol.

E22-32 d3= 3b/2πNA, or

d=3

s3(32×10−6m3/mol)

2π(6.02×1023/mol) = 2.9×10−10m.

E22-33 ahas units of energy volume per square mole, which is the same as energy per mole times

volume per mole.

P22-1 Solve (1 −x)(1.429) + x(1.250) = 1.293 for x. The result is x= 0.7598.

P22-2

P22-3 The only thing that matters is the total number of moles of gas (2.5) and the number of

moles of the second gas (0.5). Since 1/5 of the total number of moles of gas is associated with the

second gas, then 1/5 of the total pressure is associated with the second gas.

P22-4 Use Eq. 22-11 with the appropriate √2 inserted.

λ=(1.0×10−3m3)

√2(35)π(1.0×10−2m)2= 6.4×10−2m.

P22-5 (a) Since λ∝1/d2, we have

=rλn

λa

=s(27.5×10−8m)

(9.90×10−8m) = 1.67.

(b) Since λ∝1/p, we have

λ2=λ1

= (9.90×10−8m) (75.0 cm Hg)

(15.0 cm Hg) = 49.5×10−8m.

λ2=λ1

= (9.90×10−8m) (233 K)

(293 K) = 7.87×10−8m.

283

P22-6 We can assume the molecule will collide with something. Then

1 = Z∞

Ae−crdr =A/c,

so A=c. If the molecule has a mean free path of λ, then

λ=Z∞

rce−crdr = 1/c,

so A=c= 1/λ.

P22-7 What is important here is the temperature; since the temperatures are the same then the

average kinetic energies per particle are the same. Then

2m1(vrms,1)2=1

2m2(vrms,2)2.

We are given in the problem that vav,2= 2vrms,1. According to Eqs. 22-18 and 22-20 we have

vrms =r3RT

M=r3π

8r8RT

πM =r3π

8vav.

Combining this with the kinetic energy expression above,

=vrms,2

vrms,12

= 2r3π

8!2

= 4.71.

P22-8 (a) Assume that the speeds are not all the same. Transform to a frame of reference where

vav = 0, then some of the individual speeds must be greater than zero, and some will be less

than zero. Squaring these speeds will result in positive, non-zero, numbers; the mean square will

necessarily be greater than zero, so vrms >0.

(b) Only if all of the particles have the same speed will vrms=vav.

P22-9 (a) We need to ﬁrst ﬁnd the number of particles by integrating

N=Z∞

N(v)dv,

=Zv0

Cv2dv +Z∞

(0) dv =CZv0

v2dv =C

3v3

Invert, then C= 3N/v3

(b) The average velocity is found from

vav =1

NZ∞

N(v)v dv.

Using our result from above,

vav =1

NZv0

03N

v2v dv,

0Zv0

v3dv =3

4=3

4v0.

284

As expected, the average speed is less than the maximum speed. We can make a prediction about

the root mean square speed; it will be larger than the average speed (see Exercise 22-15 above) but

smaller than the maximum speed.

rms =1

NZ∞

N(v)v2dv.

Using our results from above,

rms =1

NZv0

03N

v2v2dv,

0Zv0

v4dv =3

5=3

5v2

Then, taking the square root,

rms =r3

5v0

Is p3/5>3/4? It had better be.

P22-10

P22-11

P22-12

P22-13

P22-14

P22-15 The mass of air displaced by 2180 m3is m= (1.22 kg/m3)(2180 m3) = 2660 kg.The mass

of the balloon and basket is 249 kg and we want to lift 272 kg; this leaves a remainder of 2140 kg for

the mass of the air inside the balloon. This corresponds to (2140 kg)/(0.0289 kg/mol) = 7.4×104mol.

The temperature of the gas inside the balloon is then

T= (pV )/(nR) = [(1.01×105Pa)(2180 m3)]/[(7.4×104mol)(8.31 J/mol ·K) = 358 K.

That’s 85◦C.

P22-16

P22-17

285

E23-1 We apply Eq. 23-1,

H=kA∆T

∆x

The rate at which heat ﬂows out is given as a power per area (mW/m2), so the quantity given is

really H/A. Then the temperature diﬀerence is

∆T=H

∆x

k= (0.054 W/m2)(33,000 m)

(2.5 W/m·K) = 710 K

The heat ﬂow is out, so that the temperature is higher at the base of the crust. The temperature

there is then

710 + 10 = 720 ◦C.

E23-2 We apply Eq. 23-1,

H=kA∆T

∆x= (0.74 W/m·K)(6.2 m)(3.8 m) (44 C◦)

(0.32 m) = 2400 W.

E23-3 (a) ∆T/∆x= (136 C◦)/(0.249 m) = 546 C◦/m.

(b) H=kA∆T/∆x= (401 W/m·K)(1.80 m2)(546 C◦/m) = 3.94×105W.

T= (124◦C) −(546 C◦/m)(0.11 m) = 63.9◦C.

E23-4 (a) H= (0.040 W/m·K)(1.8 m2)(32 C◦)/(0.012 m) = 190 W.

(b) Since khas increased by a factor of (0.60)/(0.04) = 15 then Hshould also increase by a

factor of 15.

E23-5 There are three possible arrangements: a sheet of type 1 with a sheet of type 1; a sheet

of type 2 with a sheet of type 2; and a sheet of type 1 with a sheet of type 2. We can look back on

Sample Problem 23-1 to see how to start the problem; the heat ﬂow will be

H12 =A∆T

(L/k1)+(L/k2)

for substances of diﬀerent types; and

H11 =A∆T/L

(L/k1)+(L/k1)=1

A∆T k1

for a double layer if substance 1. There is a similar expression for a double layer of substance 2.

For conﬁguration (a) we then have

H11 +H22 =1

A∆T k1

L+1

A∆T k2

L=A∆T

2L(k1+k2),

while for conﬁguration (b) we have

H12 +H21 = 2 A∆T

(L/k1)+(L/k2)=2A∆T

L((1/k1) + (1/k2))−1.

We want to compare these, so expanding the relevant part of the second conﬁguration

((1/k1) + (1/k2))−1= ((k1+k2)/(k2k2))−1=k1k2

k1+k2

286

Then which is larger

(k1+k2)/2 or 2k1k2

k1+k2

If k1k2then the expression become

k1/2 and 2k2,

so the ﬁrst expression is larger, and therefore conﬁguration (b) has the lower heat ﬂow. Notice that

we get the same result if k1k2!

E23-6 There’s a typo in the exercise.

H=A∆T/R; since the heat ﬂows through one slab and then through the other, we can write

(T1−Tx)/R1= (Tx−T2)/R2. Rearranging,

Tx= (T1R2+T2R1)/(R1+R2).

E23-7 Use the results of Exercise 23-6. At the interface between ice and water Tx= 0◦C. Then

R1T2+R2T1= 0, or k1T1/L1+k2T2/L2= 0. Not only that, L1+L2=L, so

k1T1L2+ (L−L2)k2T2= 0,

L2=(1.42 m)(1.67 W/m·K)(−5.20◦C)

(1.67 W/m·K)(−5.20◦C) −(0.502 W/m·K)(3.98◦C) = 1.15 m.

E23-8 ∆Tis the same in both cases. So is k. The top conﬁguration has Ht=kA∆T/(2L). The

bottom conﬁguration has Hb=k(2A)∆T/L. The ratio of Hb/Ht= 4, so heat ﬂows through the

bottom conﬁguration at 4 times the rate of the top. For the top conﬁguration Ht= (10 J)/(2 min) =

5 J/min. Then Hb= 20 J/min. It will take

t= (30 J)/(20 J/min) = 1.5 min.

E23-9 (a) This exercise has a distraction: it asks about the heat ﬂow through the window, but

what you need to ﬁnd ﬁrst is the heat ﬂow through the air near the window. We are given the

temperature gradient both inside and outside the window. Inside,

∆T

∆x=(20◦C) −(5◦C)

(0.08 m) = 190 C◦/m;

a similar expression exists for outside.

From Eq. 23-1 we ﬁnd the heat ﬂow through the air;

H=kA∆T

∆x= (0.026 W/m·K)(0.6 m)2(190 C◦/m) = 1.8 W.

The value that we arrived at is the rate that heat ﬂows through the air across an area the size of

the window on either side of the window. This heat ﬂow had to occur through the window as well,

H= 1.8 W

answers the window question.

(b) Now that we know the rate that heat ﬂows through the window, we are in a position to ﬁnd

the temperature diﬀerence across the window. Rearranging Eq. 32-1,

∆T=H∆x

kA =(1.8 W)(0.005 m)

(1.0 W/m·K)(0.6 m)2= 0.025 C◦,

so we were well justiﬁed in our approximation that the temperature drop across the glass is very

small.

287

E23-10 (a) W= +214 J, done on means positive.

(b) Q=−293 J, extracted from means negative.

E23-11 (a) ∆Eint along any path between these two points is

∆Eint =Q+W= (50 J) + (−20 J) = 30 J.

Then along ibf W = (30 J) −(36 J) = −6 J.

(b) Q= (−30 J) −(+13 J) = −43 J.

(d) ∆Eintib = (22 J) −(10 J) = 12 J; while ∆Eintbf = (40 J) −(22 J) = 18 J. There is no work

done on the path bf, so

Qbf = ∆Eintbf −Wbf = (18 J) −(0) = 18 J,

and Qib =Qibf −Qbf = (36 J) −(18 J) = 18 J.

E23-12 Q=mL = (0.10)(2.1×108kg)(333×103J/kg) = 7.0×1012J.

E23-13 We don’t need to know the outside temperature because the amount of heat energy

required is explicitly stated: 5.22 GJ. We just need to know how much water is required to transfer

this amount of heat energy. Use Eq. 23-11, and then

m=Q

c∆T=(5.22 ×109J)

(4190 J/kg ·K)(50.0◦C−22.0◦C) = 4.45 ×104kg.

This is the mass of the water, we want to know the volume, so we’ll use the density, and then

V=m

ρ=(4.45 ×104kg)

(998 kg/m3)= 44.5 m3.

E23-14 The heat energy required is Q=mc∆T. The time required is t=Q/P . Then

t=(0.136 kg)(4190 J/kg ·K)(100◦C−23.5◦C)

(220 W) = 198 s.

E23-15 Q=mL, so m= (50.4×103J)/(333 ×103J/kg) = 0.151 kg is the mount which freezes.

Then (0.258 kg) −(0.151 kg) = 0.107 kg is the amount which does not freeze.

E23-16 (a) W=mg∆y; if |Q|=|W|, then

∆T=mg∆y

mc =(9.81 m/s2)(49.4 m)

(4190 J/kg ·K) = 0.112 C◦.

E23-17 There are three “things” in this problem: the copper bowl (b), the water (w), and the

copper cylinder (c). The total internal energy changes must add up to zero, so

∆Eint,b+ ∆Eint,w+ ∆Eint,c= 0.

As in Sample Problem 23-3, no work is done on any object, so

Qb+Qw+Qc= 0.

288

The heat transfers for these three objects are

Qb=mbcb(Tf,b−Ti,b),

Qw=mwcw(Tf,w−Ti,w) + Lvm2,

Qc=mccc(Tf,c−Ti,c).

For the most part, this looks exactly like the presentation in Sample Problem 23-3; but there is an

extra term in the second line. This term reﬂects the extra heat required to vaporize m2= 4.70 g of

water at 100◦C into steam 100◦C.

Some of the initial temperatures are speciﬁed in the exercise: Ti,b=Ti,w= 21.0◦C and Tf,b=

Tf,w=Tf,c= 100◦C.

(a) The heat transferred to the water, then, is

Qw= (0.223 kg)(4190 J/kg·K) ((100◦C) −(21.0◦C)) ,

+(2.26×106J/kg)(4.70×10−3kg),

= 8.44 ×104J.

This answer diﬀers from the back of the book. I think that they (or was it me) used the latent heat

of fusion when they should have used the latent heat of vaporization!

(b) The heat transfered to the bowl, then, is

Qw= (0.146 kg)(387 J/kg ·K) ((100◦C) −(21.0◦C)) = 4.46 ×103J.

Qc=−Qb−Qw=−(4.46 ×103J) −(8.44 ×104J) = −8.89 ×104J.

The initial temperature of the cylinder is then given by

Ti,c=Tf,c−Qc

mccc

= (100◦C) −(−8.89 ×104J)

(0.314 kg)(387 J/kg ·K) = 832◦C.

E23-18 The temperature of the silver must be raised to the melting point and then the heated

silver needs to be melted. The heat required is

Q=mL +mc∆T= (0.130 kg)[(105×103J/kg) + (236 J/kg ·K)(1235 K −289 K)] = 4.27×104J.

E23-19 (a) Use Q=mc∆T,m=ρV , and t=Q/P . Then

t=[maca+ρwVwcw]∆T

=[(0.56 kg)(900 J/kg·K) + (998 kg/m3)(0.64×10−3m3)(4190 J/kg·K)](100◦C−12◦C)

(2400 W) = 117 s.

(b) Use Q=mL,m=ρV , and t=Q/P . Then

t=ρwVwLw

P=(998 kg/m3)(0.640×10−3m3)(2256×103J/kg)

(2400 W) = 600 s

is the additional time required.

289

E23-20 The heat given oﬀ by the steam will be

Qs=msLv+mscw(50 C◦).

The hear taken in by the ice will be

Qi=miLf+micw(50 C◦).

Equating,

ms=mrmi

Lf+cw(50 C◦)

Lv+cw(50 C◦),

= (0.150 kg) (333×103J/kg) + (4190 J/kg·K)(50 C◦)

(2256×103J/kg) + (4190 J/kg·K)(50 C◦)= 0.033 kg.

E23-21 The linear dimensions of the ring and sphere change with the temperature change ac-

cording to

∆dr=αrdr(Tf,r−Ti,r),

∆ds=αsds(Tf,s−Ti,s).

When the ring and sphere are at the same (ﬁnal) temperature the ring and the sphere have the

same diameter. This means that

dr+ ∆dr=ds+ ∆ds

when Tf,s=Tf,r. We’ll solve these expansion equations ﬁrst, and then go back to the heat equations.

dr+ ∆dr=ds+ ∆ds,

dr(1 + αr(Tf,r−Ti,r)) = ds(1 + αs(Tf,s−Ti,s)) ,

which can be rearranged to give

αrdrTf,r−αsdsTf,s=ds(1 −αsTi,s)−dr(1 −αrTi,r),

but since the ﬁnal temperatures are the same,

Tf=ds(1 −αsTi,s)−dr(1 −αrTi,r)

αrdr−αsds

Putting in the numbers,

Tf=

(2.54533cm)[1−(23×10−6/C◦)(100◦C)]−(2.54000cm)[1−(17×10−6/C◦)(0◦C)]

(2.54000cm)(17×10−6/C◦)−(2.54533cm)(23×10−6/C◦),

= 34.1◦C.

No work is done, so we only have the issue of heat ﬂow, then

Qr+Qs= 0.

Where “r” refers to the copper ring and “s” refers to the aluminum sphere. The heat equations are

Qr=mrcr(Tf−Ti,r),

Qs=mscs(Tf−Ti,s).

Equating and rearranging,

ms=mrcr(Ti,r−Tf)

cs(Tf−Ti,s)

ms=(21.6 g)(387 J/kg·K)(0◦C−34.1◦C)

(900 J/kg·K)(34.1◦C−100◦C) = 4.81 g.

290

E23-22 The problem is compounded because we don’t know if the ﬁnal state is only water, only

ice, or a mixture of the two.

Consider ﬁrst the water. Cooling it to 0◦C would require the removal of

Qw= (0.200 kg)(4190 J/kg ·K)(0◦C−25◦C) = −2.095×104J.

Consider now the ice. Warming the ice to would require the addition of

Qi= (0.100 kg)(2220 J/kg ·K)(0◦C + 15◦C) = 3.33×103J.

The heat absorbed by the warming ice isn’t enough to cool the water to freezing. However, the ice

can melt; and if it does it will require the addition of

Qim = (0.100 kg)(333×103J/kg) = 3.33×104J.

This is far more than will be liberated by the cooling water, so the ﬁnal temperature is 0◦C, and

consists of a mixture of ice and water.

(b) Consider now the ice. Warming the ice to would require the addition of

Qi= (0.050 kg)(2220 J/kg ·K)(0◦C + 15◦C) = 1.665×103J.

The heat absorbed by the warming ice isn’t enough to cool the water to freezing. However, the ice

can melt; and if it does it will require the addition of

Qim = (0.050 kg)(333×103J/kg) = 1.665×104J.

This is still not enough to cool the water to freezing. Hence, we need to solve

Qi+Qim +micw(T−0◦C) + mwcw(T−25◦C) = 0,

which has solution

T=(4190 J/kg ·K)(0.200 kg)(25◦C) −(1.665×103J) + (1.665×104J)

(4190 J/kg ·K)(0.250 kg) = 2.5◦C.

E23-23 (a) c= (320 J)/(0.0371 kg)(42.0◦C−26.1◦C) = 542 J/kg ·K.

(b) n=m/M = (37.1 g)/(51.4 g/mol) = 0.722 mol.

E23-24 (1) W=−p∆V= (15 Pa)(4 m3) = −60 J for the horizontal path; no work is done during

the vertical path; the net work done on the gas is −60 J.

(2) It is easiest to consider work as the (negative of) the area under the curve; then W=

−(15 Pa + 5 Pa)(4 m3)/2 = −40 J.

(3) No work is done during the vertical path; W=−p∆V= (5 Pa)(4 m3) = −20 J for the

horizontal path; the net work done on the gas is −20 J.

E23-25 Net work done on the gas is given by Eq. 23-15,

W=−Zp dV.

But integrals are just the area under the curve; and that’s the easy way to solve this problem. In the

case of closed paths, it becomes the area inside the curve, with a clockwise sense giving a positive

value for the integral.

The magnitude of the area is the same for either path, since it is a rectangle divided in half by

a square. The area of the rectangle is

(15×103Pa)(6 m3) = 90×103J,

so the area of path 1 (counterclockwise) is -45 kJ; this means the work done on the gas is -(-45 kJ)

or 45 kJ. The work done on the gas for path 2 is the negative of this because the path is clockwise.

291

E23-26 During the isothermal expansion,

W1=−nRT ln V2

=−p1V1ln p1

During cooling at constant pressure,

W2=−p2∆V=−p2(V1−V2) = −p2V1(1 −p1/p2) = V1(p1−p2).

The work done is the sum, or

−(204×103Pa)(0.142 m3) ln (204×103Pa)

(101×103Pa) + (0.142 m3)(103 Pa) = −5.74×103J.

E23-27 During the isothermal expansion,

W=−nRT ln V2

=−p1V1ln V2

W=−(1.32)(1.01×105Pa)(0.0224 m3) ln (0.0153 m3)

(0.0224 m3)= 1.14×103J.

E23-28 (a) pV γis a constant, so

p2=p1(V1/V2)γ= (1.00 atm)[(1 l)/(0.5 l)]1.32 = 2.50 atm;

T2=T1(p2/p1)(V2/V1), so

T2= (273 K)(2.50 atm)

(1.00 atm)

(0.5 l)

(1 l) = 341 K.

(b) V3=V2(p2/p1)(T3/T2), so

V3= (0.5 l)(273 K)

(341 K) = 0.40 l.

W12 =(1.01×105Pa/atm)(1×10−3m3/l)

(1.32) −1[(2.5 atm)(0.5 l) −(1.0 atm)(1 l)] = 78.9 J.

During the cooling process,

W23 =−p∆V=−(1.01×105Pa/atm)(2.50 atm)(1×10−3m3/l)[(0.4 l) −(0.5 l)] = 25.2 J.

The net work done is W123 = 78.9 J + 25.2 J = 104.1 J.

E23-29 (a) According to Eq. 23-20,

pf=piViγ

Vfγ=(1.17 atm)(4.33 L)(1.40)

(1.06 L)(1.40) = 8.39 atm.

(b) The ﬁnal temperature can be found from the ideal gas law,

Tf=Ti

pfVf

piVi

= (310 K)(8.39 atm)(1.06 L)

(1.17 atm)(4.33 L) = 544 K.

W=1

(1.40) −1(8.39 ×1.01×105Pa)(1.06×10−3m3)

−(1.17 ×1.01×105Pa)(4.33×10−3m3),

= 966 J.

292

E23-30 Air is mostly diatomic (N2and O2), so use γ= 1.4.

(a) pV γis a constant, so

V2=V1γ

pp1/p2=V11.4

p(1.0 atm)/(2.3 atm) = 0.552V1.

T2=T1(p2/p1)(V2/V1), so

T2= (291 K)(2.3 atm)

(1.0 atm)

(0.552V1)

= 369 K,

or 96◦C.

(b) The work required for delivering 1 liter of compressed air is

W12 =(1.01×105Pa/atm)(1×10−3m3/l)

(1.40) −1[(2.3 atm)(1.0 l) −(1.0 atm)(1.0 l/0.552)] = 123 J.

The number of liters per second that can be delivered is then

∆V/∆t= (230 W)/(123 J/l) = 1.87 l.

E23-31 Eint,rot =nRT = (1 mol)(8.31 J/mol ·K)(298 K) = 2480 J.

E23-32 Eint,rot =3

2nRT = (1.5)(1 mol)(8.31 J/mol ·K)(523 K) = 6520 J.

E23-33 (a) Invert Eq. 32-20,

γ=ln(p1/p2)

ln(V2/V1)=ln(122 kPa/1450 kPa)

ln(1.36 m3/10.7 m3)= 1.20.

(b) The ﬁnal temperature is found from the ideal gas law,

Tf=Ti

pfVf

piVi

= (250 K)(1450×103Pa)(1.36 m3)

(122×103Pa)(10.7 m3)= 378 K,

which is the same as 105◦C.

n= [pV ]/[RT ] = [(1450×103Pa)(1.36 m3)]/[(8.31 J/mol ·K)(378 K)] = 628 mol.

(d) From Eq. 23-24,

Eint =3

2nRT =3

2(628 mol)(8.31 J/mol ·K)(250 K) = 1.96×106J

before the compression and

Eint =3

2nRT =3

2(628 mol)(8.31 J/mol ·K)(378 K) = 2.96×106J

after the compression.

(e) The ratio of the rms speeds will be proportional to the square root of the ratio of the internal

energies,

p(1.96×106J)/(2.96×106J) = 0.813;

we can do this because the number of particles is the same before and after, hence the ratio of the

energies per particle is the same as the ratio of the total energies.

293

E23-34 We can assume neon is an ideal gas. Then ∆T= 2∆Eint/3nR, or

∆T=2(1.34×1012eV)(1.6×10−19J/eV)

3(0.120 mol)(8.31 J/mol ·K) = 1.43×10−7J.

E23-35 At constant pressure, doubling the volume is the same as doubling the temperature. Then

Q=nCp∆T= (1.35 mol)7

2(8.31 J/mol ·K)(568 K −284 K) = 1.12×104J.

E23-36 (a) n=m/M = (12 g)/(28 g/mol) = 0.429 mol.

(b) This is a constant volume process, so

Q=nCV∆T= (0.429 mol)5

2(8.31 J/mol ·K)(125◦C−25◦C) = 891J.

E23-37 (a) From Eq. 23-37,

Q=ncp∆T= (4.34 mol)(29.1 J/mol ·K)(62.4 K) = 7880 J.

(b) From Eq. 23-28,

Eint =5

2nR∆T=5

2(4.34 mol)(8.31 J/mol ·K)(62.4 K) = 5630 J.

Ktrans =3

2nR∆T=5

2(4.34 mol)(8.31 J/mol ·K)(62.4 K) = 3380 J.

E23-38 cV=3

2(8.31 J/mol ·K)/(4.00 g/mol) = 3120 J/kg ·K.

E23-39 Each species will experience the same temperature change, so

Q=Q1+Q2+Q3,

=n1C1∆T+n2C2∆T+n3C3∆T,

Dividing this by n=n1+n2+n3and ∆Twill return the speciﬁc heat capacity of the mixture, so

C=n1C1+n2C2+n3C3

n1+n2+n3

E23-40 WAB = 0, since it is a constant volume process, consequently, W=WAB +WABC =−15 J.

But around a closed path Q=−W, so Q= 15 J. Then

QCA =Q−QAB −QBC = (15 J) −(20 J) −(0 J) = −5 J.

Note that this heat is removed from the system!

294

E23-41 According to Eq. 23-25 (which is speciﬁc to ideal gases),

∆Eint =3

2nR∆T,

and for an isothermal process ∆T= 0, so for an ideal gas ∆Eint = 0. Consequently, Q+W= 0 for

an ideal gas which undergoes an isothermal process.

But we know Wfor an isotherm, Eq. 23-18 shows

W=−nRT ln Vf

Then ﬁnally

Q=−W=nRT ln Vf

E23-42 Qis greatest for constant pressure processes and least for adiabatic. Wis greatest (in

magnitude, it is negative for increasing volume processes) for constant pressure processes and least

for adiabatic. ∆Eint is greatest for constant pressure (for which it is positive), and least for adiabatic

(for which is is negative).

E23-43 (a) For a monatomic gas, γ= 1.667. Fast process are often adiabatic, so

T2=T1(V1/V2)γ−1= (292 K)[(1)(1/10)]1.667−1= 1360 K.

(b) For a diatomic gas, γ= 1.4. Fast process are often adiabatic, so

T2=T1(V1/V2)γ−1= (292 K)[(1)(1/10)]1.4−1= 733 K.

E23-44 This problem cannot be solved without making some assumptions about the type of pro-

cess occurring on the two curved portions.

E23-45 If the pressure and volume are both doubled along a straight line then the process can

be described by

p=p1

The ﬁnal point involves the doubling of both the pressure and the volume, so according to the ideal

gas law, pV =nRT , the ﬁnal temperature T2will be four times the initial temperature T1.

Now for the exercises.

(a) The work done on the gas is

W=−Z2

p dV =−Z2

V dV =−p1

V1V2

2−V2

2

We want to express our answer in terms of T1. First we take advantage of the fact that V2= 2V1,

then

W=−p1

V14V2

2−V2

2=−3

2p1V1=−3

2nRT1

(b) The nice thing about ∆Eint is that it is path independent, we care only of the initial and

ﬁnal points. From Eq. 23-25,

∆Eint =3

2nR∆T=3

2nR (T2−T1) = 9

2nRT1

295

Q= ∆Eint −W=9

2nRT1+3

2nRT1= 6nRT1.

(d) If we deﬁne speciﬁc heat as heat divided by temperature change, then

c=Q

n∆T=6RT1

4T1−T1

= 2R.

E23-46 The work done is the area enclosed by the path. If the pressure is measured in units of

10MPa, then the shape is a semi-circle, and the area is

W= (π/2)(1.5)2(10MPa)(1×10−3m3) = 3.53×104J.

The heat is given by Q=−W=−3.53×104J.

E23-47 (a) Internal energy changes according to ∆Eint =Q+W, so

∆Eint = (20.9 J) −(1.01×105Pa)(113×10−6m3−63×10−6m3) = 15.9 J.

(b) T1=p1V1/nR and T2=p2V2/nR, but pis constant, so ∆T=p∆V/nR. Then

CP=Q

n∆T=QR

p∆V=(20.9 J)(8.31 J/mol ·K)

(1.01×105Pa)(113×10−6m3−63×10−6m3)= 34.4 J/mol ·K.

E23-48 Constant Volume

(a) Q= 3(3.15 mol)(8.31 J/mol ·K)(52.0 K) = 4080 J.

(b) W= 0.

Constant Pressure

(a) Q= 4(3.15 mol)(8.31 J/mol ·K)(52.0 K) = 5450 J.

(b) W=−p∆V=−nR∆T=−(3.15 mol)(8.31 J/mol ·K)(52.0 K) = −1360 J.

Adiabatic

(a) Q= 0.

(b) W= (pfVf−piVi)/(γ−1) = nR∆T/(γ−1) = 3(3.15 mol)(8.31 J/mol ·K)(52.0 K) = 4080 J.

P23-1 (a) The temperature diﬀerence is

(5 C◦/9 F◦)(72◦F− −20◦F) = 51.1 C◦.

The rate of heat loss is

H= (1.0 W/m·K)(1.4 m2)(51.1 C◦)/(3.0×10−3m) = 2.4×104W.

(b) Start by ﬁnding the Rvalues.

Rg= (3.0×10−3m)/(1.0 W/m·K) = 3.0×10−3m2·K/W,

Ra= (7.5×10−2m)/(0.026W/m·K) = 2.88m2·K/W.

Then use Eq. 23-5,

H=(1.4 m2)(51.1 C◦)

2(3.0×10−3m2·K/W) + (2.88m2·K/W) = 25 W.

Get double pane windows!

296

P23-2 (a) H= (428 W/m·K)(4.76×10−4m2)(100 C◦)/(1.17 m) = 17.4 W.

(b) ∆m/∆t=H/L = (17.4 W)/(333×103J/kg) = 5.23×10−5kg/s, which is the same as 188 g/h.

P23-3 Follow the example in Sample Problem 23-2. We start with Eq. 23-1:

H=kAdT

dr ,

H=k(4πr2)dT

dr ,

Zr2

Hdr

4πr2=ZT2

kdT,

4pi 1

r1−1

r2=k(T1−T2),

Hr2−r1

r1r2= 4πk(T1−T2),

H=4πk(T1−T2)r1r2

r2−r1

P23-4 (a) H= (54×10−3W/m2)4π(6.37×106m)2= 2.8×1013W.

(b) Using the results of Problem 23-3,

∆T=(2.8×1013W)(6.37×106m−3.47×106m)

4π(4.2 W/m·K)(6.37×106m)(3.47×106m) = 7.0×104C◦.

Since T2= 0◦C, we expect T1= 7.0×104C◦.

P23-5 Since H=−kA dT/dx, then H dx =−aT dT .His a constant, so integrate both side

according to

ZH dx =−ZaT dT,

HL =−a1

2(T2

2−T2

1),

H=aA

2L(T2

1−T2

2).

P23-6 Assume the water is all at 0◦C. The heat ﬂow through the ice is then H=kA∆T/x; the

rate of ice formation is ∆m/∆t=H/L. But ∆m=ρA∆x, so

∆x

∆t=H

ρAL =k∆T

ρLx ,

(1.7 W/m·K)(10 C◦)

(920 kg/m3)(333×103J/kg)(0.05 m) = 1.11×10−6m/s.

That’s the same as 0.40 cm/h.

P23-7 (a) Start with the heat equation:

Qt+Qi+Qw= 0,

297

where Qtis the heat from the tea, Qiis the heat from the ice when it melts, and Qwis the heat

from the water (which used to be ice). Then

mtct(Tf−Tt,i) + miLf+mwcw(Tf−Tw,i) = 0,

which, since we have assumed all of the ice melts and the masses are all equal, can be solved for Tf

Tf=ctTt,i+cwTw,i−Lf

ct+cw

=(4190J/kg ·K)(90◦C) + (4190J/kg ·K)(0◦C) −(333×103J/kg)

(4190J/kg ·K) + (4190J/kg ·K) ,

= 5.3◦C.

(b) Once again, assume all of the ice melted. Then we can do the same steps, and we get

Tf=ctTt,i+cwTw,i−Lf

ct+cw

=(4190J/kg ·K)(70◦C) + (4190J/kg ·K)(0◦C) −(333×103J/kg)

(4190J/kg ·K) + (4190J/kg ·K) ,

=−4.7◦C.

So we must have guessed wrong when we assumed that all of the ice melted. The heat equation

then simpliﬁes to

mtct(Tf−Tt,i) + miLf= 0,

and then

mi=mtct(Tt,i−Tf)

=(0.520 kg)(4190J/kg ·K)(90◦C−0◦)

(333×103J/kg) ,

= 0.458 kg.

P23-8 c=Q/m∆T=H/(∆m/∆t)∆T. But ∆m/∆t=ρ∆V/∆t. Combining,

c=H

(∆V/∆t)ρ∆T=(250 W)

(8.2×10−6m3/s)(0.85×103kg/m3)(15 C◦)= 2.4×103J/kg ·K.

P23-9 (a) n=NA/M , so

=(2256×103J/kg)

(6.02×1023/mol)/(0.018 kg/mol) = 6.75×10−20J.

(b) Eav =3

2kT , so



Eav

=2(6.75×10−20J)

3(1.38×10−23J/K)(305 K) = 10.7.

P23-10 Qw+Qt= 0, so

Ct∆Tt+mwcw(Tf−Ti) = 0,

Ti=(0.3 kg)(4190 J/kg ·m)(44.4◦C) + (46.1 J/K)(44.4◦C−15.0◦C)

(0.3 kg)(4190 J/kg ·m) = 45.5◦C.

298

P23-11 We can use Eq. 23-10, but we will need to approximate cﬁrst. If we assume that the

line is straight then we use c=mT +b. I approximate mfrom

m=(14 J/mol ·K) −(3 J/mol ·K)

(500 K) −(200 K) = 3.67×10−2J/mol.

Then I ﬁnd bfrom those same data points,

b= (3 J/mol ·K) −(3.67×10−2J/mol)(200 K) = −4.34 J/mol ·K.

Then from Eq. 23-10,

Q=nZTf

c dT,

=nZTf

(mT +b)dT,

=nhm

2T2+bT iTf

=nm

2(Tf2−Ti2) + b(Tf−Ti),

= (0.45mol) (3.67×10−2J/mol)

2((500 K)2−(200 K)2)

+ (−4.34 J/mol ·K)(500 K −200 K)) ,

= 1.15×103J.

P23-12 δQ =nCδT , so

Q=nZC dT,

=n(0.318 J/mol ·K2)T2/2−(0.00109 J/mol ·K3)T3/3−(0.628 J/mol ·K)T90 K

50 K ,

=n(645.8 J/mol).

Finally,

Q= (645.8 J/mol)(316 g)/(107.87 g/mol) = 189 J.

P23-13 T V γ−1is a constant, so

T2= (292 K)(1/1.28)(1.40)−1= 265 K

P23-14 W=−Rp dV , so

W=−ZnRT

V−nb −an2

V2dV,

=−nRT ln(V−nb)−an2

V

=−nRT ln Vf−nb

Vi−nb −an21

Vf−1

Vi.

299

P23-15 When the tube is horizontal there are two regions ﬁlled with gas, one at p1,i,V1,i; the

other at p2,i,V2,i. Originally p1,i=p2,i= 1.01×105Pa and V1,i=V2,i= (0.45 m)A, where Ais the

cross sectional area of the tube.

When the tube is held so that region 1 is on top then the mercury has three forces on it: the

force of gravity, mg; the force from the gas above pushing down p2,fA; and the force from the gas

below pushing up p1,fA. The balanced force expression is

p1,fA=p2,fA+mg.

If we write m=ρlmAwhere lm= 0.10 m, then

p1,f=p2,f+ρglm.

Finally, since the tube has uniform cross section, we can write V=Al everywhere.

(a) For an isothermal process pili=pflf, where we have used V=Al, and then

p1,i

l1,i

l1,f−p2,i

l2,i

l2,f

=ρglm.

But we can factor out p1,i=p2,iand l1,i=l2,i, and we can apply l1,f+l2,f= 0.90 m. Then

l1,f−1

0.90 m −l1,f

=ρglm

pili

Put in some numbers and rearrange,

0.90 m −2l1,f= (0.294 m−1)l1,f(0.90 m −l1,f),

which can be written as an ordinary quadratic,

(0.294 m−1)l1,f2−(2.265)l1,f+ (0.90 m) = 0

The solutions are l1,f= 7.284 m and 0.421 m. Only one of these solutions is reasonable, so the

mercury shifted down 0.450 −0.421 = 0.029 m.

(b) The math is a wee bit uglier here, but we can start with piliγ=pflfγ, and this means that

everywhere we had a l1,fin the previous derivation we need to replace it with l1,fγ. Then we have

l1,fγ−1

(0.90 m −l1,f)γ=ρglm

piliγ.

This can be written as

(0.90 m −l1,f)γ−l1,fγ−= (0.404 m−γ)l1,fγ(0.90 m −l(0.1,f)γ,

which looks nasty to me! I’ll use Maple to get the answer, and ﬁnd l1,f= 0.429, so the mercury

shifted down 0.450 −0.429 = 0.021 m.

Which is more likely? Turn the tube fast, and the adiabatic approximation works. Eventually

the system will return to room temperature, and then the isothermal approximation is valid.

P23-16 Internal energy for an ideal diatomic gas can be written as

Eint =5

2nRT =5

2pV,

simply by applying the ideal gas law. The room, however, has a ﬁxed pressure and volume, so the

internal energy is independent of the temperature. As such, any energy supplied by the furnace

leaves the room, either as heat or as expanding gas doing work on the outside.

300

P23-17 The speed of sound in the iodine gas is

v=fλ = (1000 Hz)(2 ×0.0677 m) = 135 m/s.

Then

γ=Mv2

RT =n(0.127 kg/mol)(135 m/s)2

(8.31 J/mol ·K)(400 K) =n(0.696).

Since γis greater than one, n≥2. If n= 2 then γ= 1.39, which is consistent; if n= 3 then

γ= 2.08, which is not consistent.

Consequently, iodine gas is diatomic.

P23-18 W=−Q=mL = (333×103J/kg)(0.122 kg) = 4.06×104J.

P23-19 (a)

Process AB

Q=3

2(1.0 mol)(8.31 J/mol ·K)(300 K) = 3740 J.

W= 0.

∆Eint =Q+W= 3740 J.

Process BC

Q= 0.

W= (pfVf−piVi)/(γ−1) = nR∆T/(γ−1) = (1.0 mol)(8.31 J/mol ·K)(−145 K)/(1.67 −1) =

−1800 J

∆Eint =Q+W=−1800 J.

Process AB

Q=5

2(1.0 mol)(8.31 J/mol ·K)(−155 K) = −3220 J.

W=−p∆V=−nR∆T=−(1.0 mol)(8.31 J/mol ·K)(−155 K) = 1290 J.

∆Eint =Q+W= 1930 J.

Cycle

Q= 520 J; W=−510 J (rounding error!); ∆Eint = 10 J (rounding error!)

P23-20

P23-21 pf= (16.0 atm)(50/250)1.40 = 1.68 atm.The work done by the gas during the expansion

W= [piVi−pfVf]/(γ−1),

=(16.0 atm)(50×10−6m3)−(1.68 atm)(250×10−6m3)

(1.40) −1(1.01×105Pa/atm),

= 96.0 J.

This process happens 4000 times per minute, but the actual time to complete the process is half of

the cycle, or 1/8000 of a minute. Then P= (96 J)(8000)/(60 s) = 12.8×103W.

301

E24-1 For isothermal processes the entropy expression is almost trivial, ∆S=Q/T, where if Q

is positive (heat ﬂow into system) the entropy increases.

Then Q=T∆S= (405 K)(46.2 J/K) = 1.87×104J.

E24-2 Entropy is a state variable and is path independent, so

(a) ∆Sab,2= ∆Sab,1= +0.60 J/K,

(b) ∆Sba,2=−∆Sab,2=−0.60 J/K,

E24-3 (a) Heat only enters along the top path, so

Qin =T∆S= (400 K)(0.6 J/K−0.1 J/K) = 200 J.

(b) Heat leaves only bottom path, so

Qout =T∆S= (250 K)(0.1 J/K−0.6 J/K) = −125 J.

Since Q+W= 0 for a cyclic path,

W=−Q=−[(200 J) + (−125 J)] = −75 J.

E24-4 (a) The work done for isothermal expansion is given by Eq. 23-18,

W=−(4.00 mol)(8.31 J/mol ·K)(410 K) ln 3.45V1

=−1.69×104J.

(b) For isothermal process, Q=−W, then

∆S=Q/T = (1.69×104J)/(410 K) = 41.2 J/K.

E24-5 (a) We want to ﬁnd the heat absorbed, so

Q=mc∆T= (1.22 kg)(387 J/mol ·K) ((105◦C) −(25.0◦C)) = 3.77×104J.

(b) We want to ﬁnd the entropy change, so, according to Eq. 24-1,

∆S=ZTf

=ZTf

mc dT

=mc ln Tf

The entropy change of the copper block is then

∆S=mc ln Tf

= (1.22 kg)(387 J/mol ·K) ln (378 K)

(298 K) = 112 J/K.

E24-6 ∆S=Q/T =mL/T , so

∆S= (0.001 kg)(−333×103J/kg)/(263 K) = −1.27J/K.

302

E24-7 Use the ﬁrst equation on page 551.

n=∆S

Rln(Vf/V i)=(24 J/K)

(8.31 J/mol ·K) ln(3.4/1.3) = 3.00 mol.

E24-8 ∆S=Q/T c−Q/T h.

(a) ∆S= (260 J)(1/100 K −1/400 K) = 1.95 J/K.

(b) ∆S= (260 J)(1/200 K −1/400 K) = 0.65 J/K.

(d) ∆S= (260 J)(1/360 K −1/400 K) = 0.0722 J/K.

E24-9 (a) If the rod is in a steady state we wouldn’t expect the entropy of the rod to change.

Heat energy is ﬂowing out of the hot reservoir into the rod, but this process happens at a ﬁxed

temperature, so the entropy change in the hot reservoir is

∆SH=QH

=(−1200 J)

(403 K) =−2.98 J/K.

The heat energy ﬂows into the cold reservoir, so

∆SC=QH

=(1200 J)

(297 K) = 4.04 J/K.

The total change in entropy of the system is the sum of these two terms

∆S= ∆SH+ ∆SC= 1.06 J/K.

(b) Since the rod is in a steady state, nothing is changing, not even the entropy.

E24-10 (a) Qc+Ql= 0, so

mccc(T−Tc) + mlcl(T−Tl) = 0,

which can be solved for Tto give

T=(0.05 kg)(387 J/kg ·K)(400 K) + (0.10 kg)(129 J/kg ·K)(200 K)

(0.05 kg)(387 J/kg ·K) + (0.10 kg)(129 J/kg ·K) = 320 K.

(b) Zero.

∆S= (0.05 kg)(387 J/kg ·K) ln (320 K)

(400 K) + (0.10 kg)(129 J/kg ·K) ln (320 K)

(200 K) = 1.75 J/K.

E24-11 The total mass of ice and water is 2.04 kg. If eventually the ice and water have the same

mass, then the ﬁnal state will have 1.02 kg of each. This means that 1.78 kg −1.02 kg = 0.76 kg of

water changed into ice.

(a) The change of water at 0◦C to ice at 0◦C is isothermal, so the entropy change is

∆S=Q

T=−mL

T=(0.76 kg)(333×103J/kg)

(273 K) =−927 J/K.

(b) The entropy change is now +927 J/K.

303

E24-12 (a) Qa+Qw= 0, so

maca(T−Ta) + mwcw(T−Tw) = 0,

which can be solved for Tto give

T=(0.196 kg)(900 J/kg ·K)(380 K) + (0.0523 kg)(4190 J/kg ·K)(292 K)

(0.196 kg)(900 J/kg ·K) + (0.0523 kg)(4190 J/kg ·K) = 331 K.

That’s the same as 58◦C.

(b) ∆S=mc ln Tf/T i, so

∆Sa= (0.196 kg)(900 J/kg ·K) ln (331 K)

(380 K) =−24.4 J/K.

(0.0523 kg)(4190 J/kg ·K) ln (331 K)

(292 K) = 27.5 J/K.

(d) ∆S= (27.5 J/K) + (−24.4 J/K) = 3.1 J/K.

E24-13 (a) e= 1 −(36.2 J/52.4 J) = 0.309.

(b) W=Qh−Qc= (52.4 J) −(36.2 J) = 16.2 J.

E24-14 (a) Qh= (8.18 kJ)/(0.25) = 32.7 kJ, Qc=Qh−W= (32.7 kJ) −(8.18 kJ) = 24.5 kJ.

(b) Qh= (8.18 kJ)/(0.31) = 26.4 kJ, Qc=Qh−W= (26.4 kJ) −(8.18 kJ) = 18.2 kJ.

E24-15 One hour’s worth of coal, when burned, will provide energy equal to

(382×103kg)(28.0×106J/kg) = 1.07×1013J.

In this hour, however, the plant only generates

(755×106W)(3600 s) = 2.72×1012J.

The eﬃciency is then

e= (2.72×1012J)/(1.07×1013J) = 25.4%.

E24-16 We use the convention that all quantities are positive, regardless of direction. WA= 5WB;

Qi,A= 3Qi,B; and Qo,A= 2Qo,B. But WA=Qi,A−Qo,A, so

5WB= 3Qi,B−2Qo,B,

or, applying WB=Qi,B−Qo,B,

5WB= 3Qi,B−2(Qi,B−WB),

3WB=Qi,B,

WB/Qi,B= 1/3 = eB.

Then

eA=WA

Qi,A

=5WB

3Qi,B

3=5

304

E24-17 (a) During an isothermal process W=−Q=−2090 J. The negative indicates that the

gas did work on the environment.

(b) The eﬃciency is e= 1 −(297 K)/(412 K) = 0.279.Then

Qo=Qi(1 −e) = (2090 J)[1 −(0.279)] = 1510 J.

Since this is rejected heat it should actually be negative.

the environment.

E24-18 1−e=Tc/T h, or Tc=Th(1 −e). The diﬀerence is

∆T=Th−Tc=The,

so Th= (75 C◦)/(0.22) = 341 K, and

Tc= (341 K)[(1 −(0.22)] = 266 K.

E24-19 The BC and DA processes are both adiabatic; so if we could ﬁnd an expression for work

done during an adiabatic process we might be almost done. But what is an adiabatic process? It is

a process for which Q= 0, so according to the ﬁrst law

∆Eint =W.

But for an ideal gas

∆Eint =nCV∆T,

as was pointed out in Table 23-5. So we have

|W|=nCV|∆T|

and since the adiabatic paths BC and DA operate between the same two isotherms, we can conclude

that the magnitude of the work is the same for both paths.

E24-20 (a) To save typing, assume that all quantities are positive. Then

e1= 1 −T2/T1,

W1=e1Q1, and Q2=Q1−W1. Not only that, but

e2= 1 −T3/T2,

and W2=e2Q2. Combining,

e=W1+W2

=e1Q1+e2(Q1−W1)

=e1+e2(1 −e1) = e1+e2−e1e2,

e= 1 −T2

+ 1 −T3

T2−1 + T2

+T3

T2−T3

= 1 − −T3

(b) e= 1 −(311 K)/(742 K) = 0.581.

E24-21 (a) p2= (16.0 atm)(1/5.6)(1.33) = 1.62 atm.

(b) T2=T1(1/5.6)(1.33)−1= (0.567)T1, so

e= 1 −(0.567) = 0.433.

305

E24-22 (a) The area of the cycle is ∆V∆p=p0V0, so the work done by the gas is

W= (1.01×105Pa)(0.0225 m3) = 2270 J.

(b) Let the temperature at abe Ta. Then

Tb=Ta(Vb/Va)(pb/pa) = 2Ta.

Let the temperature at cbe Tc. Then

Tc=Ta(Vc/Va)(pc/pa) = 4Ta.

Consequently, ∆Tab =Taand ∆Tbc = 2Ta. Putting this information into the constant volume and

constant pressure heat expressions,

Qab =3

2nR∆Tab =3

2nRTa=3

2paVa,

and

Qbc =5

2nR∆Tbc =5

2nR2Ta= 5paVa,

so that Qac =13

2p0V0, or

Qac =13

2(1.01×105Pa)(0.0225 m3) = 1.48×104J.

(d) ec= 1 −(Ta/4Ta) = 0.75.

E24-23 According to Eq. 24-15,

K=TL

TH−TL

=(261 K)

(299 K) −(261 K) = 6.87

Now we solve the question out of order.

(b) The work required to run the freezer is

|W|=|QL|/K = (185 kJ)/(5.70) = 32.5 kJ.

(a) The freezer will discharge heat into the room equal to

|QL|+|W|= (185 kJ) + (32.5 kJ) = 218 kJ.

E24-24 (a) K=|QL|/|W|= (568 J)/(153 J) = 3.71.

(b) |QH|=|QL|+|W|= (568 J) + (153 J) = 721 J.

E24-25 K=TL/(TH−TL); |W|=|QL|/K =|QL|(TH/TL−1).

(a) |W|= (10.0 J)(300 K/280K −1) = 0.714 J.

(b) |W|= (10.0 J)(300 K/200K −1) = 5.00 J.

(d) |W|= (10.0 J)(300 K/50K −1) = 50.0 J.

E24-26 K=TL/(TH−TL); |W|=|QL|/K =|QL|(TH/TL−1).Then

|QH|=|QL|+|W|=|QL|(TH/TL) = (0.150 J)(296 K/4.0 K) = 11 J.

306

E24-27 We will start with the assumption that the air conditioner is a Carnot refrigerator.

K=TL/(TH−TL); |W|=|QL|/K =|QL|(TH/TL−1).For fun, I’ll convert temperature to the

absolute Rankine scale! Then

|QL|= (1.0 J)/(555◦R/530◦R−1) = 21 J.

E24-28 The best coeﬃcient of performance is

Kc= (276 K)/(308 K −276 K) = 8.62.

The inventor claims they have a machine with

K= (20 kW −1.9kW)/(1.9 kW) = 9.53.

Can’t be done.

E24-29 (a) e= 1 −(258 K/322 K) = 0.199. |W|= (568 J)(0.199) = 113 J.

(b) K= (258 K)/(322 K −258 K) = 4.03. |W|= (1230 J)/(4.03) = 305 J.

E24-30 The temperatures are distractors!

|W|=|QH|−|QL|=|QH| − K|W|,

|W|=|QH|/(1 + K) = (7.6 MJ)/(1 + 3.8) = 1.58 MJ.

Then P= (1.58 MJ)/(3600 s) = 440 W.

E24-31 K= (260 K)/(298 K −260 K) = 6.8.

E24-32 K= (0.85)(270K)/(299K−270K) = 7.91. In 15 minutes the motor can do (210 W)(900 s) =

1.89×105J of work. Then

|QL|=K|W|= (7.91)(1.89×105J) = 1.50×106J.

E24-33 The Carnot engine has an eﬃciency

= 1 −T2

=|W|

|Q1|.

The Carnot refrigerator has a coeﬃcient of performance

K=T4

T3−T4

=|Q4|

|W|.

Lastly, |Q4|=|Q3| − |W|. We just need to combine these three expressions into one. Starting with

the ﬁrst, and solving for |W|,

|W|=|Q1|T1−T2

Then we combine the last two expressions, and

T3−T4

=|Q3|−|W|

|W|=|Q3|

|W|−1.

307

Finally, combine them all,

T3−T4

=|Q3|

|Q1|

T1−T2−1.

Now, we rearrange,

|Q3|

|Q1|=T4

T3−T4

+ 1T1−T2

=T3

T3−T4T1−T2

= (1 −T2/T1)/(1 −T4/T3).

E24-34 (a) Integrate:

ln N!≈ZN

ln x dx =Nln N−N+ 1 ≈Nln N−N.

(b) 91, 752, and about 615,000. You will need to use the Stirling approximation extended to a

double inequality to do the last two:

√2πnn+1/2e−n+1/(12n+1) < n!<√2πnn+1/2e−n+1/(12n).

E24-35 (a) For this problem we don’t care how the particles are arranged inside a section, we

only care how they are divided up between the two sides.

Consequently, there is only one way to arrange the particles: you put them all on one side, and

you have no other choices. So the multiplicity in this case is one, or w1= 1.

(b) Once the particles are allowed to mix we have more work in computing the multiplicity.

Using Eq. 24-19, we have

w2=N!

(N/2)!(N/2)! =N!

((N/2)!)2

S=kln w

For part (a), with a multiplicity of 1, S1= 0. Now for part (b),

S2=kln N!

((N/2)!)2!=kln N!−2kln(N/2)!

and we need to expand each of those terms with Stirling’s approximation.

Combining,

S2= = k(Nln N−N)−2k((N/2) ln(N/2) −(N/2)) ,

=kNlnN −kN −kN ln N+kN ln 2 + kN,

=kN ln 2

Finally, ∆S=S2−S1=kN ln 2.

(d) The answer should be the same; it is a free expansion problem in both cases!

308

P24-1 We want to evaluate

∆S=ZTf

nCVdT

=ZTf

nAT 3dT

=ZTf

nAT 2dT ,

=nA

3Tf3−Ti3.

Into this last expression, which is true for many substances at suﬃciently low temperatures, we

substitute the given numbers.

∆S=(4.8 mol)(3.15×10−5J/mol ·K4)

3(10 K)3−(5.0 K)3= 4.41×10−2J/K.

P24-2

P24-3 (a) Work is only done along path ab, where Wab =−p∆V=−3p0∆V0. So Wabc =−3p0V0.

(b) ∆ERbc =3

2nR∆Tbc, with a little algebra,

∆Eintbc =3

2(nRTc−nRTb) = 3

2(pcVc−pbVb) = 3

2(8 −4)p0V0= 6p0V0.

∆Sbc =3

2nR ln(Tc/Tb),with a little algebra,

∆Sbc =3

2nR ln(pc/pb) = 3

2nR ln 2.

P24-4 (a) For an isothermal process,

p2=p1(V1/V2) = p1/3.

For an adiabatic process,

p3=p1(V1/V2)γ=p1(1/3)1.4= 0.215p1.

For a constant volume process,

T3=T2(p3/p2) = T1(0.215/0.333) = 0.646T1.

(b) The easiest ones ﬁrst: ∆Eint12 = 0, W23 = 0, Q31 = 0, ∆S31 = 0. The next easier ones:

∆Eint23 =5

2nR∆T23 =5

2nR(0.646T1−T1) = −0.885p1V1,

Q23 = ∆Eint23 −W23 =−0.885p1V1,

∆Eint31 =−∆Eint23 −∆Eint12 = 0.885p1V1,

W31 = ∆Eint31 −Q31 = 0.885p1V1.

309

Finally, some harder ones:

W12 =−nRT1ln(V2/V1) = −p1V1ln(3) = −1.10p1V1,

Q12 = ∆Eint12 −W12 = 1.10p1V1.

And now, the hardest:

∆S12 =Q12/T1= 1.10nR,

∆S23 =−∆S12 −∆S31 =−1.10nR.

P24-5 Note that TA=TB=TC/4 = TD.

Process I: ABC

(a) QAB =−WAB =nRT0ln(VB/VA) = p0V0ln 2. QBC =3

2nR(TC−TB) = 3

2(pCVC−pBVB) =

2(4p0V0−p0V0) = 4.5p0V0.

(b) WAB =−nRT0ln(VB/VA) = −p0V0ln 2. WBC = 0.

2nR(TC−TA) = 3

2(pCVC−pAVA) = 3

2(4p0V0−p0V0) = 4.5p0V0.

(d) ∆SAB =nR ln(VB/VA) = nR ln 2; ∆SBC =3

2nR ln(TC/TB) = 3

2nR ln 4 = 3nR ln 2.Then

∆SAC = 4nR.

Process II: ADC

(a) QAD =−WAD =nRT0ln(VD/VA) = −p0V0ln 2. QDC =5

2nR(TC−TD) = 5

2(pCVC−

pDVD) = 5

2(4p0V0−p0V0) = 10p0V0.

(b) WAB =−nRT0ln(VD/VA) = p0V0ln 2. WDC =−p∆V=−p0(2V0−V0/2) = −3

2p0V0.

2nR(TC−TA) = 3

2(pCVC−pAVA) = 3

2(4p0V0−p0V0) = 4.5p0V0.

(d) ∆SAD =nR ln(VD/VA) = −nR ln 2; ∆SDC =5

2nR ln(TC/TD) = 5

2nR ln 4 = 5nR ln 2.Then

∆SAC = 4nR.

P24-6 The heat required to melt the ice is

Q=m(cw∆T23 +L+ci∆T12),

= (0.0126 kg)[(4190 J/kg ·K)(15 C◦) + (333×103J/kg) + (2220 J/kg ·K)(10 C◦)],

= 5270 J.

The change in entropy of the ice is

∆Si=m[cwln(T3/T2) + L/T2+ciln(T2/T1)],

= (0.0126kg)[(4190J/kg·K) ln(288/273) + (333×103J/kg)/(273K),

+(2220J/kg·K) ln(273/263)],

= 19.24 J/K

The change in entropy of the lake is ∆Sl= (−5270 J)/(288 K) = 18.29 J/K. The change in entropy

of the system is 0.95 J/kg.

P24-7 (a) This is a problem where the total internal energy of the two objects doesn’t change,

but since no work is done during the process, we can start with the simpler expression Q1+Q2= 0.

The heat transfers by the two objects are

Q1=m1c1(T1−T1,i),

Q2=m2c2(T2−T2,i).

Note that we don’t call the ﬁnal temperature Tfhere, because we are not assuming that the two

objects are at equilibrium.

310

We combine these three equations,

m2c2(T2−T2,i) = −m1c1(T1−T1,i),

m2c2T2=m2c2T2,i+m1c1(T1,i−T1),

T2=T2,i+m1c1

m2c2

(T1,i−T1)

As object 1 “cools down”, object 2 “heats up”, as expected.

(b) The entropy change of one object is given by

∆S=ZTf

mc dT

T=mc ln Tf

and the total entropy change for the system will be the sum of the changes for each object, so

∆S=m1c1ln T1

Ti,1

+m2c2ln T2

Ti,2

Into the this last equation we need to substitute the expression for T2in as a function of T1. There’s

no new physics in doing this, just a mess of algebra.

remembering that T2is a function, not a variable. Then

d(∆S)

dT1

=m1c1

+m2c2

dT2

dT1

We’ve saved on algebra, but now we need to evaluate dT2/dT1. Starting with the results from part

(a),

dT2

dT1

dT1T2,i+m1c1

m2c2

(T1,i−T1),

=−m1c1

m2c2

Now we collect the two results and write

d(∆S)

dT1

=m1c1

+m2c2

T2−m1c1

m2c2,

=m1c11

T1−1

T2.

We could consider writing T2out in all of its glory, but what would it gain us? Nothing. There is

actually considerably more physics in the expression as written, because...

(d) ...we get a maximum for ∆Swhen d(∆S)/dT1= 0, and this can only occur when T1=T2

according to the expression.

P24-8 Tb= (10.4×1.01×105Pa)(1.22 m3)/(2 mol)(8.31 J/mol ·K) = 7.71×104K.Maybe not so

realistic? Tacan be found after ﬁnding

pc=pb(Vb/Vc)γ= (10.4×1.01×105Pa)(1.22/9.13)1.67 = 3.64×104Pa,

Then

Ta=Tb(pa/pb) = (7.71×104K)(3.64×104/1.05×106) = 2.67×103K.

311

Similarly,

Tc=Ta(Vc/Va) = (2.67×103K)(9.13/1.22) = 2.00×104K.

(a) Heat is added during process ab only;

Qab =3

2nR(Tb−Ta) = 3

2(2 mol)(8.31 J/mol ·K)(7.71×104K−2.67×103K) = 1.85×106J.

(b) Heat is removed during process ca only;

Qca =5

2nR(Ta−Tc) = 5

2(2 mol)(8.31 J/mol ·K)(2.67×103K−2.00×104K) = −0.721×106J.

(d) e=W/Qab = (1.13×106)/(1.85×106) = 0.611.

P24-9 The pV diagram for this process is Figure 23-21, except the cycle goes clockwise.

(a) Heat is input during the constant volume heating and the isothermal expansion. During

heating,

Q1=3

2nR∆T=3

2(1 mol)(8.31 J/mol ·K)(600 K −300 K) = 3740 J;

During isothermal expansion,

Q2=−W2=nRT ln(Vf/V i) = (1 mol)(8.31 J/mol ·K)(600 K) ln(2) = 3460 J;

so Qin = 7200 J.

(b) Work is only done during the second and third processes; we’ve already solved the second,

W2=−3460 J;

W3=−p∆V=paVc−paVa=nR(Tc−Ta) = (1 mol)(8.31 J/mol ·K)(600 K −300 K) = 2490 J;

So W=−970 J.

P24-10 (a) Tb=Ta(pb/pa) = 3Ta;

Tc=Tb(Vb/Vc)γ−1= 3Ta(1/4)0.4= 1.72Ta;

pc=pb(Vb/Vc)γ= 3pa(1/4)1.4= 0.430pa;

Td=Ta(Va/Vd)γ−1=Ta(1/4)0.4= 0.574Ta;

pd=pa(Va/Vd)γ=pa(1/4)1.4= 0.144pa.

(b) Heat in occurs during process ab, so Qi=5

2nR∆Tab = 5nRTa; Heat out occurs during

process cd, so Qo=5

2nR∆Tcd = 2.87nRTa. Then

e= 1 −(2.87nRTa/5nRTa) = 0.426.

P24-11 (c) (VB/VA)=(pA/pB) = (0/0.5) = 2. The work done on the gas during the isothermal

compression is

W=−nRT ln(VB/VA) = −(1 mol)(8.31 J/mol ·K)(300 K) ln(2) = −1730 J.

Since ∆Eint = 0 along an isotherm Qh= 1730 J.

The cycle has an eﬃciency of e= 1 −(100/300) = 2/3. Then for the cycle,

W=eQh= (2/3)(1730 J) = 1150 J.

312

Instructor Solutions Manual For Physics Volume 1

Instructor%20Solutions%20Manual%20for%20Physics%20Volume%201

Instructor%20Solutions%20Manual%20for%20Physics%20Volume%201

Instructor%20Solutions%20Manual%20for%20Physics%20Volume%201

Navigation menu

Versions of this User Manual:

Views

Navigation