Manual
User Manual:
Open the PDF directly: View PDF ![]() .
.
Page Count: 4

The G.R.B. code
J´erˆome Guilet, Rapha¨el Raynaud, Matteo Bugli
24 juillet 2018
1 R´esum´e
Pour produire des courbes de lumi`ere associ´ees `a la coalescence de deux ´etoiles `a neu-
trons, le code G.R.B. utilise un mod`ele adapt´e des travaux de Sun et al. (2017) qui sup-
pose que l’objet compact form´e est un magn´etar qui perd de l’´energie par un ph´enom`ene
de freinage magn´etique induit par la composante dipolaire du champ magn´etique de
l’´etoile. L’´evolution temporelle de la fr´equence de rotation de l’´etoile est d´etermin´ee par
le couple magn´etique du rayonnement du dipˆole
Ω(t) = Ω0
(1 + t/τem)1/2,(1)
o`u τem = 3c3I/(B2R6Ω2
0) est le temps caract´eristique de ralentissement, I,Ret B´etant
le moment d’inertie, le rayon et le champ magn´etique dipolaire de l’´etoile `a neutrons.
On suppose que le rayonnement X est produit par dissipation interne dans le vent du
magn´etar et ´emis de fa¸con isotrope (Zhang, 2013). La luminosit´e en rayons X est alors
d´etermin´ee par la luminosit´e de ralentissement du dipˆole, modulo un facteur d’efficacit´e η
LX=ηLdip(t) = ηB2R6Ω4(t)
6c3.(2)
Le magn´etar central r´esultant de la fusion de deux ´etoiles `a neutrons, il faut ´egalement
tenir compte de la pr´esence d’´ejecta opaques r´esiduels qui vont d’abord absorber ce
rayonnement dans certaines directions et le r´e-´emettre avec un spectre de corps noir.
Le calcul des courbes de lumi`ere dans la zone o`u le rayonnement est initialement pi´eg´e
requiert donc de d´eterminer l’´evolution dynamique des ejecta. On utilise dans ce cas le
mod`ele de Yu et al. (2013), qui prend en compte l’injection d’´energie par le magn´etar
central et le chauffage additionel par d´esint´egration d’´el´ements radioactifs.
Enfin, pr´ecisons que cette approche ph´enom´enologique permet de comparer simple-
ment diff´erentes ´equations d’´etat qui d´eterminent le rayon R, le moment d’inertie Iet
la p´eriode critique de rotation du magn´etar en de¸c`a de laquelle l’objet s’effondre en
trou noir. Cette p´eriode Pest reli´ee `a la masse maximale d’une ´etoile `a neutrons par la
relation (Lasky et al., 2014)
Mmax =MTOV(1 + αP β),(3)
1

o`u la masse maximale d’une ´etoile statique MTOV et les exposants αet βsont donn´es
par les mod`eles d’´equation d’´etat (Ai et al., 2018). Lorsque l’´etoile s’effondre en trou
noir du fait du ralentissement de sa rotation, on suppose que la luminosit´e due au vent
du magn´etar s’arrˆete abruptement.
2 Equations
We consider a magnetar of radius R, mass M, moment of inertia Iand surface mag-
netic field B, surrounded by an accretion disc with initial mass Mdisc and radius Rdisc.
The time evolution of the magnetar angular frequency Ω will be determined both by the
magnetic and accretion torques Ndip and Nacc, defined respectively by
Ndip =−µ2Ω3
6c3,(4)
Nacc =n(Ω) (GMrm)1/2˙
M , (5)
where µ=BR3is the dipole moment and rmthe Alfv´en radius
rm=µ4/7(GM)−1/7˙
M−2/7.(6)
Depending of the values of the Alfv´en radius and the corotation radius rc=GM/Ω21/3,
the system will be accreting or expelling material: if rm< rc, the system is accreting
and the accretion torque spins up the magnetar, whereas for rm> rcthe magnetar
loses angular momentum with the expelled material — the so-called propeller regime
(Gompertz et al., 2014). The change of sign of the accretion torque is handle by the
following prefactor
n(Ω) =
1−rm
rc3/2
rm> R
1−Ω
ΩK
rm< R
,(7)
where ΩK=pGM/R3.
— BAR MODE INSTA
— accretion rate
— ejecta
2
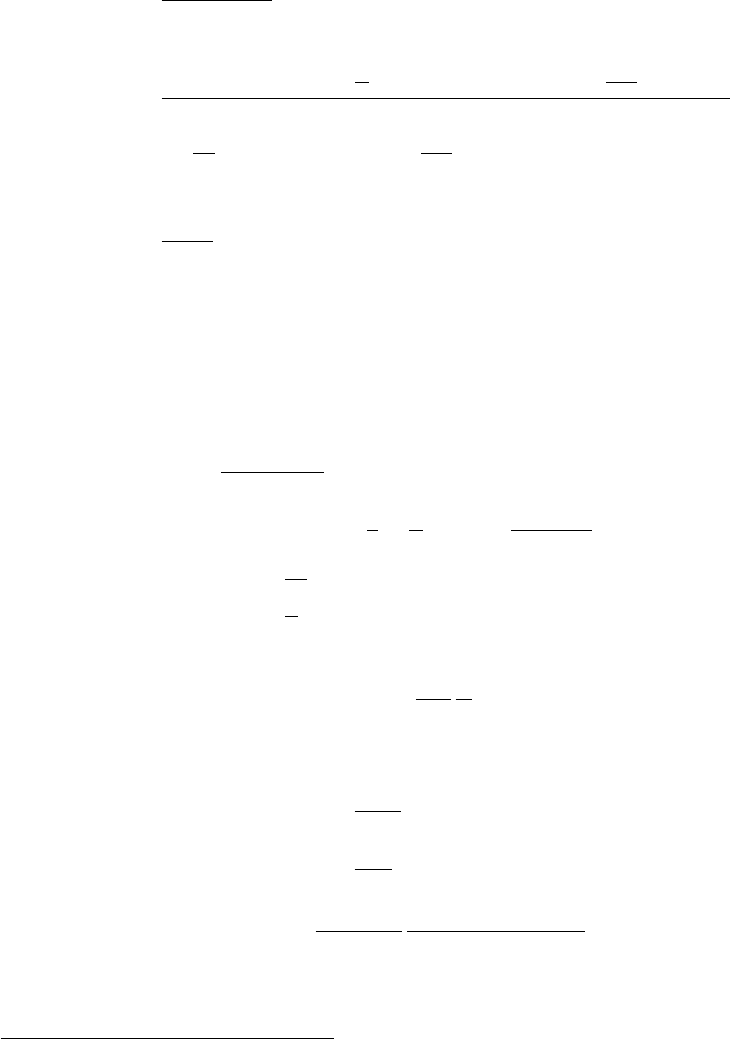
The complete ODE system to be integrated in time is then (Sun et al., 2017)
˙
Ω = Ndip +Nacc
I,(8)
˙
t0=D(Γ) ,(9)
˙
Γ = (Ldip +Lra −Le)−Γ
D(ξLdip +Lra −Le)+ΓDE0
int
3V0(4πR2βc)
Mejc2+E0
int
,(10)
˙
E0
int =Dh1
D2(ξLdip +Lra −Le)−E0
int
3V0(4πR2βc)i,(11)
˙
V0= 4πR2βcD,(12)
˙
R=βc
1−β.(13)
In the above system, Dis the Doppler factor defined by D= [Γ(1 −βcos θ)]−1with
β(Γ) = (1−Γ−2)−1/2and ξan efficiency parameter defining the fraction of the spin-down
energy that is used to heat the ejecta. The different luminosities that enter the equations
are the dipole spin-down luminosity Ldip, the co-moving radiative heating luminosity Lra
and the co-moving bolometric emission luminosity of the heated electrons Le, respectively
Ldip =B2R6Ω(t)4
6c3,(14)
Lra = 4 ×1049Mej,-2 1
2−1
πarctan t0−1.3 s
0.11 s 1.3
D2,(15)
L0
e=(cE0
int Γ
τR t<tτ=1
cE0
int Γ
Rt≥tτ=1
.(16)
Optical depth of the ejecta
τ=κMej
V0
R
Γ(17)
Blackbody spectrum
T0=
E0
int
aV 0τ1/4
τ > 1
E0
int
aV 01/4
τ≤1
,(18)
νLbb =8π2D2R2
h3c2
(hν/D)4
exp (hν/DkT 0)−1.(19)
where aand kare respectively the blackbody radiation constant and the Boltzmann
constant 1.
1. In CGS units, we have a= 7.5646 ×10−15 erg cm−3K−4and k= 1.380 658 ×10−16 erg/K.
3
The main output of the code are the X-ray luminosities for both the free and trapped
zones
LX,free(t) = ηLdip(t),(20)
LX,trapped(t) = e−τηLdip(t) + Z6 keV
0.3 keV
νLbbdν . (21)
where one needs to introduce the factor ηto parametrize the efficiency at which the
dipole spin-down luminosity is converted to X-ray luminosity.
— magnetar collapse
The code has been benchmark against the results of Gompertz et al. (2014).
Bibliography
Ai, S., Gao, H., Dai, Z.-G., et al. 2018, ArXiv e-prints, arXiv:1802.00571
Gompertz, B. P., O’Brien, P. T., & Wynn, G. A. 2014, Monthly Notices of the Royal
Astronomical Society, 438, 240
Lasky, P. D., Haskell, B., Ravi, V., Howell, E. J., & Coward, D. M. 2014, Physical Review
D, 89, 047302
Sun, H., Zhang, B., & Gao, H. 2017, The Astrophysical Journal, 835, 7
Yu, Y.-W., Zhang, B., & Gao, H. 2013, The Astrophysical Journal, 776, L40
Zhang, B. 2013, The Astrophysical Journal, 763, L22
4